Complete Solutions to TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS) of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| OSWAAL PUBLICATION | TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS) |
| Number Of Questions | 16 |
| Academic Year | 2023-24 |
In the trapezium ABCD, `AB || DC` and `Delta ARD` - `Delta BEC`. Then Prove that AD = BC.

View SolutionIf a straight line divdes two sides of a triangle proportionally, then the straight line a parallel to third sides, (Converse of Thales theorem). Prove.
View SolutionIn a trapezium, prove that the line joining the midpoints of non - parallel sides are parallel to the parallel sides of the trapezium.
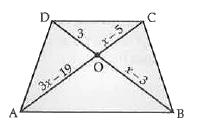
View SolutionIn a trapezium ABCD, `AB || CD`
If `OA = 3x - 19`
`OC = 3 - 5`
`BO = x - 3` and `OD = 3`, find `x`
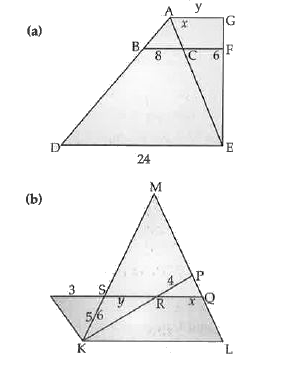
View SolutionFind the unknown values is each of the following figures. All lengths given in centimetres. (Measures are not in scale)
(a)
 (C)
(C)
View SolutionIn `Delta ABC`, D and E are points in the sides AB and AC respectively such that `DE || BC` If `AD = 6 cm`, `DB = 9 cm`, and `AE = 8 cm` find AC.
View SolutionE and F are points on the sides PQ and PR respectively of `Delta` PQR. For each of the following cases, state whether EF || QR:
(i) `PE=3.9 cm, EQ=3 cm,PF=3.6 cm,FR=2.4cm`
(ii) `PE=4 cm,QE=4.5 cm,PF=8 cm,RF=9 cm`
(iii) `PQ=1.28cm,PR=2.56 cm,PE=0.18cm,PF=0.36cm`
View SolutionIn the figure, `PC || QK` and `BC || HK`. If `AQ = 6 cm`, `QH = 4 cm`, `HP = 5 cm` and `KC = 18 cm`. Find AK and PB.
View SolutionIn the figure, `PR || RC` and `QR || BD`. Prove that `PQ || CD`.
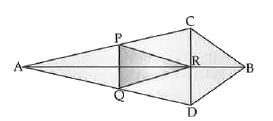
View SolutionIn `Delta ABC`, `DE || BC` and `CD || EF`. Prove that
`AD^(2) = AF xx AB`
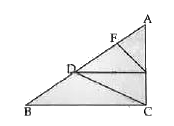
View SolutionIf the diagonals of a quadrilateral divide each other proportionally, then prove that the quadrilateral is a trapezium.
View SolutionIn the given figure, `(SP)/(SQ)= (PT)/(TR)` and `/_ PST` = `/_PRQ`. Prove that PQR is an isosceles triangle.
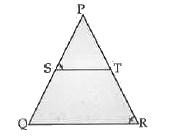
View SolutionIn a `Delta ABC`, let P and Q be points on AB and AC respectively such that `PQ || BC`. Prove that the median AD bisects PQ.
View SolutionThrought the mid-point M of the sides of a parallelogram ABCD, the line BM is drawn intersecting AC at L, and AD produced to E. Prove that `EL = 2 BL`.
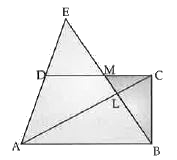
View SolutionProve that the two madians of a triangle divide each other in the ratio `2 : 1`
View SolutionIn the given figure `/_ ABD = /_BDC` and `CD = 4AB`. Show that `BD =5BE`.
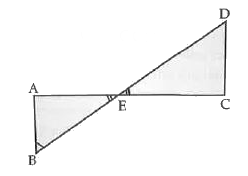
View Solution
TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS) questions and complete solutions for chapter TRIANGLES of OSWAAL PUBLICATION MATHS (KANNADA ENGLISH) of Class 10
All Exercises from OSWAAL PUBLICATION Class 10 MATHS TRIANGLES
- TEXTBOOK CORNER (EXERCISE 2.1)
- TEXTBOOK CORNER (EXERCISE 2.2)
- TEXTBOOK CORNER (EXERCISE 2.3)
- TEXTBOOK CORNER (EXERCISE 2.4)
- TEXTBOOK CORNER (EXERCISE 2.5)
- TEXTBOOK CORNER (EXERCISE 2.6)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (MULTIPLE CHOICE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (LONG ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-3 AREA OF SIMILAR TRIANGLES (MULTIPLE CHOICE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (LONG ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-4 PYTHAGOREAN TRIPLETS (MULTIPLE CHOICE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-5 PYTHAGORAS THEOREM (LONG ANSWER TYPE QUESTIONS)
- TOPIC-5 PYTHAGORAS THEOREM (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-5 PYTHAGORAS THEOREM (SHORT ANSWER TYPE QUESTIONS)