Complete Solutions to Torque , Torque Equation And Equilibrium Of A Rigid Body of chapter ROTATIONAL DYNAMICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | ROTATIONAL DYNAMICS |
| A2Z | Torque , Torque Equation And Equilibrium Of A Rigid Body |
| Number Of Questions | 29 |
| Academic Year | 2023-24 |
A couple produces.
View SolutionWhen a torque acting upon a system is zero, which of the following will be constant ?
View SolutionWhen a steady torque (net force is zero) is acting on a body, the body.
View SolutionThe force `7 hat i+ 3 hat j - 5 hat k` acts on a particle whose position vector is `hat i- hat j + hat k`. What is the torque of a given force about the origin ?
View SolutionA wheel of radius `20 cm` has four forces applied to it as shown in fig. Then, the torque produced by these forces about `O` is.
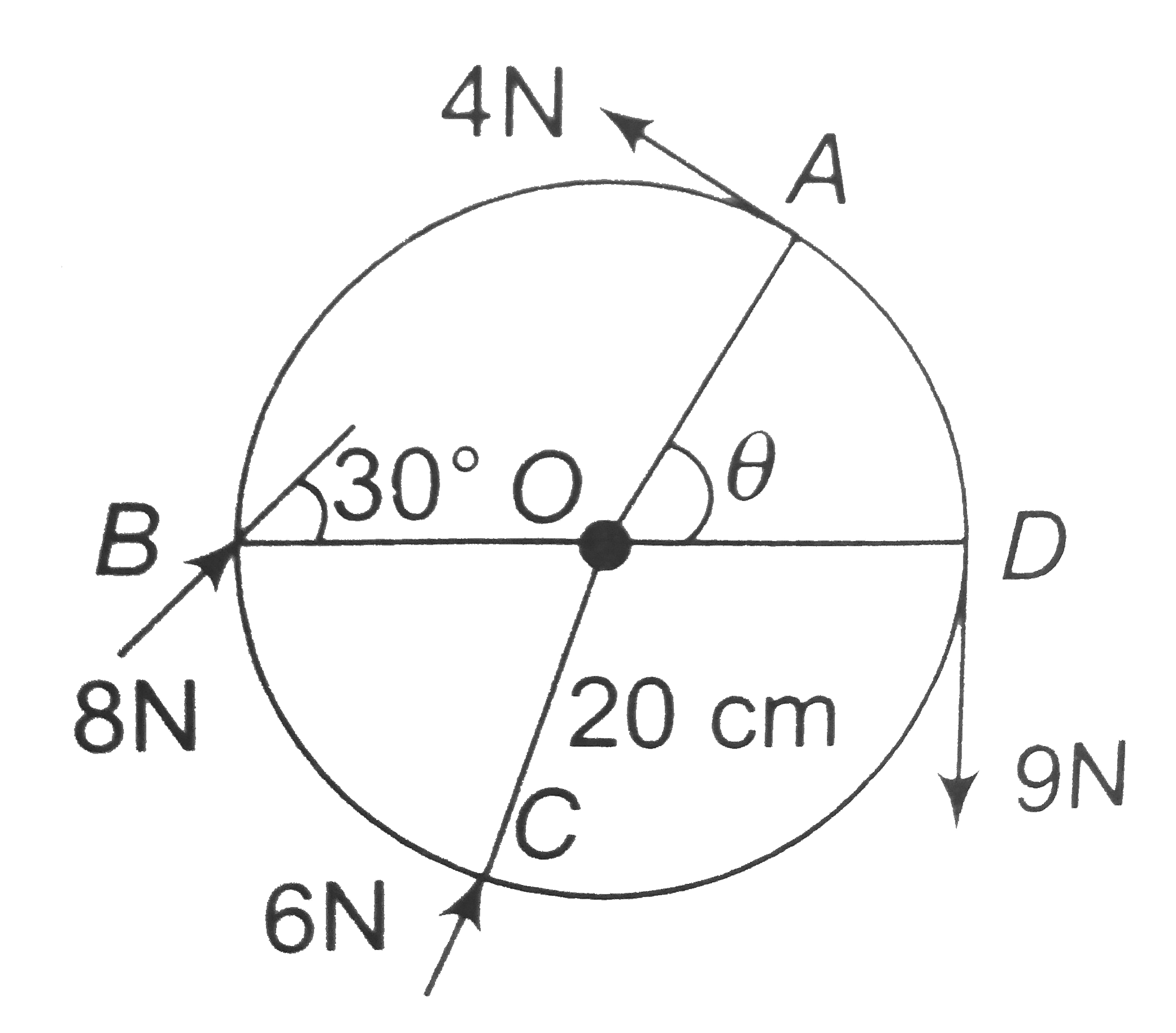 .
.
View SolutionFigure shows a lamina in `x-y` plane. Two axes `z` and `z'` pass perpendicular to its plane. A force `F` acts in the plane of lamina at point `P` as shown. Which of the following statements is incorrect ?
(The point `P` is closer to `z'-axis` than the z-axis).
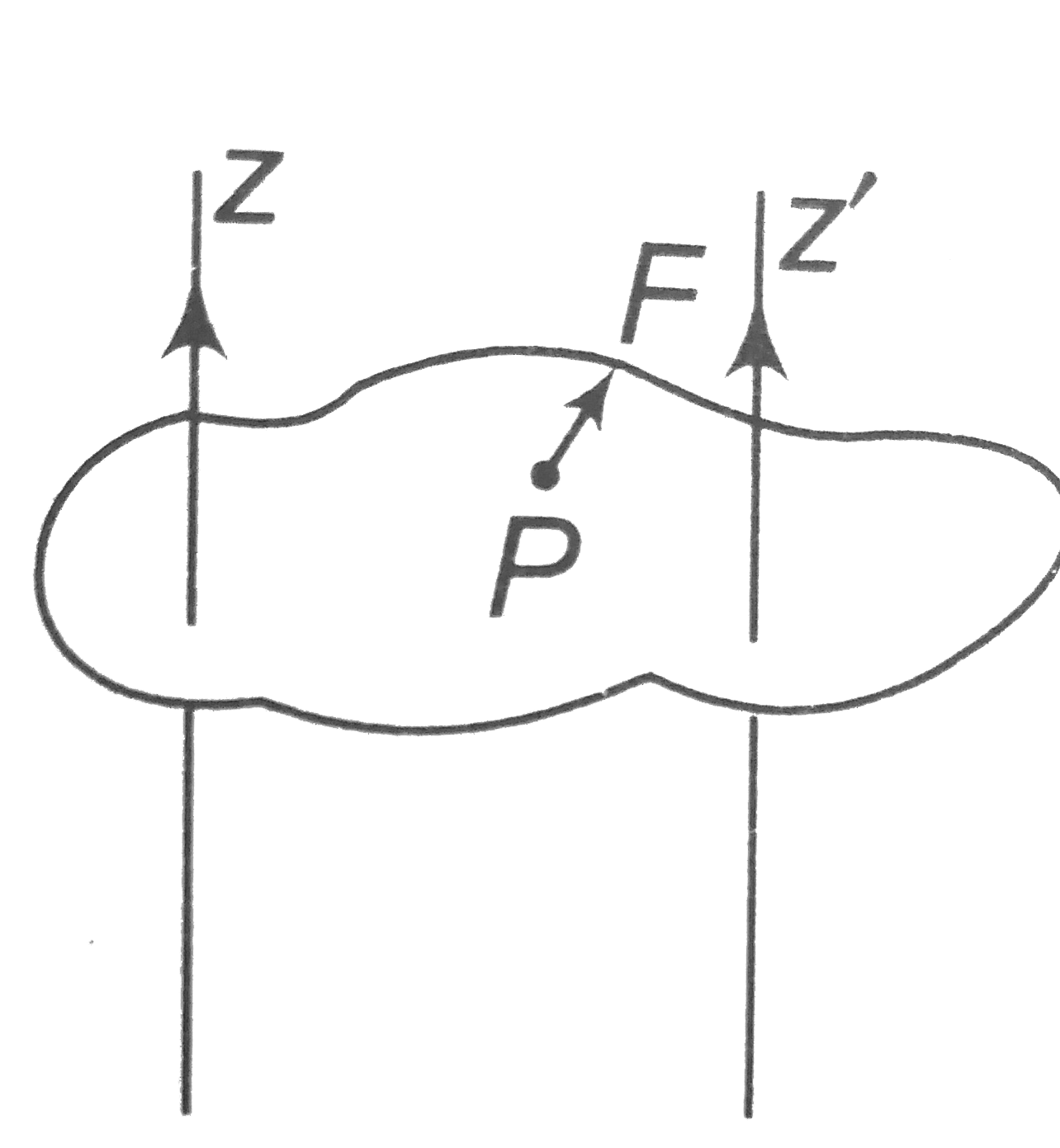 .
.
View SolutionA rigid rod of length `2 L` is acted upon by some forces. All forces labelled `F` have the same magnitude. Which cases have a non-zero net torque acting on the rod about its centre ?
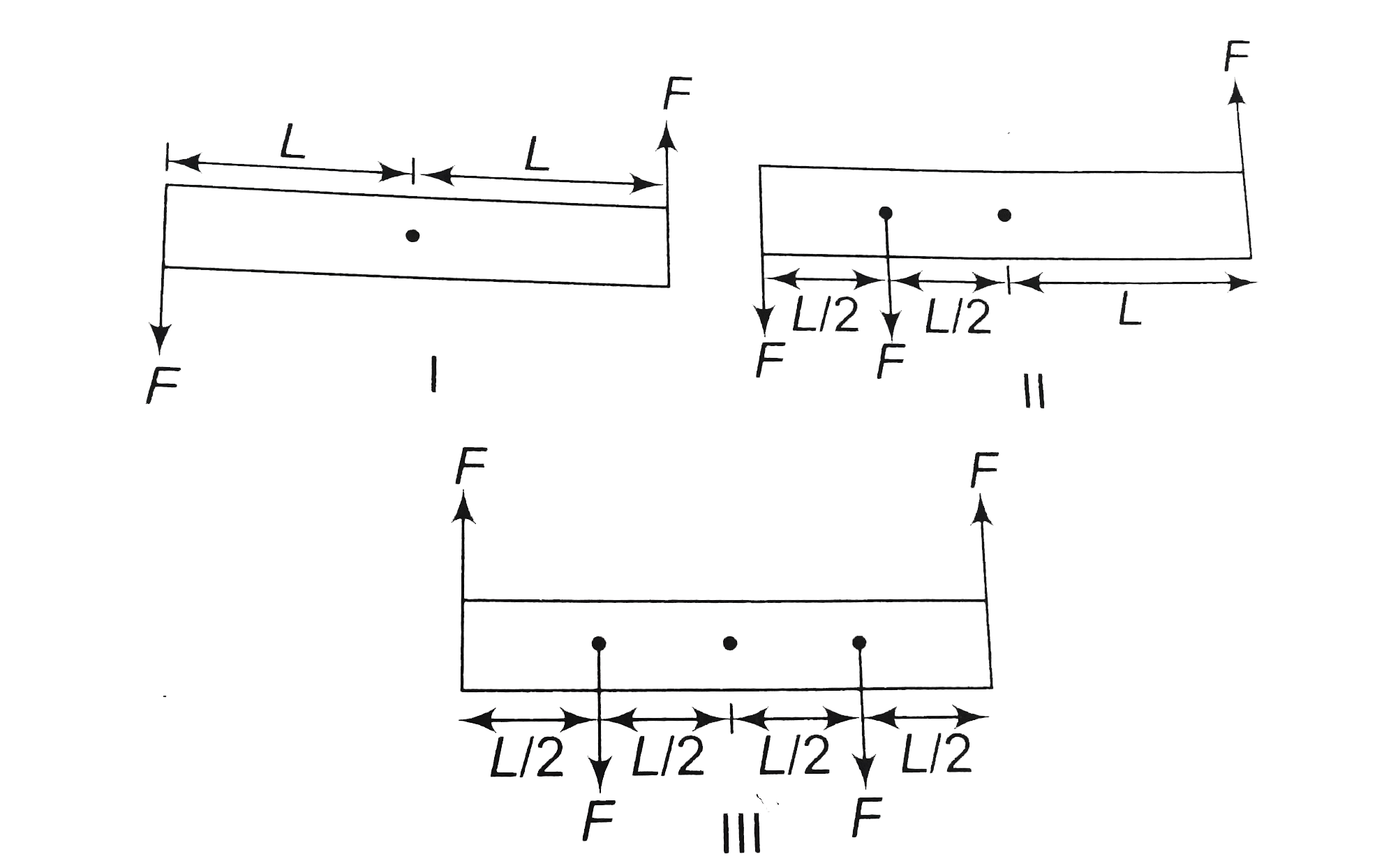 .
.
View SolutionA uniform rod of length `1 m` mass `4 kg` is supports on two knife-edges placed `10 cm` from each end. A `60 N` weight is suspended at `30 cm` from one end. The reactions at the knife edges is.
View SolutionA metre stick is balanced on a knife edge at its centre. When two coins, each of mass `5 g` are put one on one of the other at the `12 cm` mark, the stick is found to balanced at `45 cm`. The mass of the metre stick is.
View SolutionA non-uniform bar of weight `W` and weight `L` is suspended by two strings of neigligible weight as shown in figure. The angles made by the strings with the vertical are `theta_1` and `theta_2` respectively.
The distance `d` of the centre of gravity of the bar from left end is.
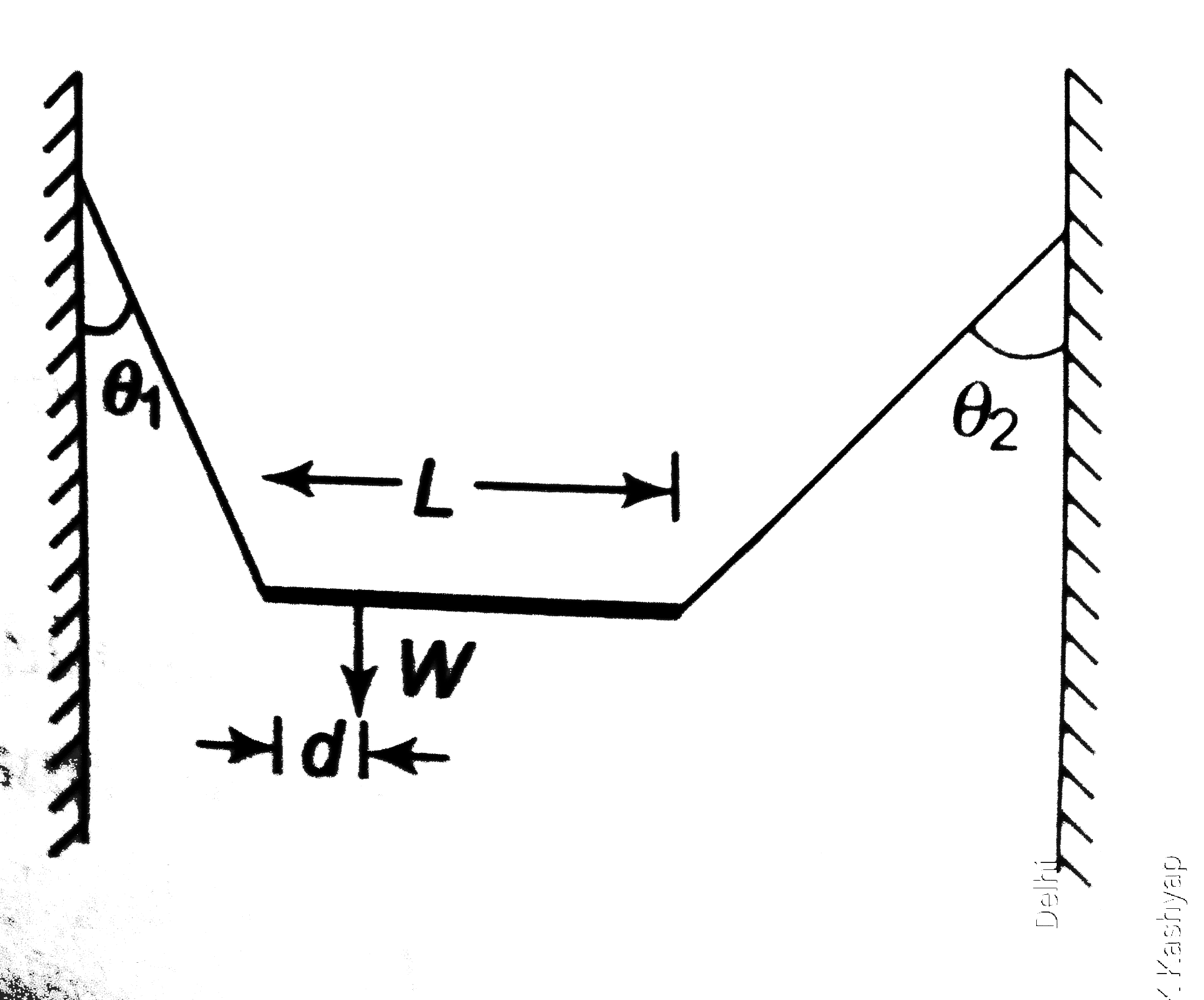 .
.
View SolutionA uniform cube of side a and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point that is directly above the centre of the face, at a height 3a/4 above the base. The minimum value of F which the cube begins to tip about the edge is ....(Assume that the cube does not slide).
View SolutionA triangular set square of angles `30^@, 60^@, 90^@` and of negligible mass is suspended freely from the right angled corner and weights are hung at the two corners. If the hypotenuse of the set square sets horizontally, then the ratio of the weights `W_1//W_2` is.
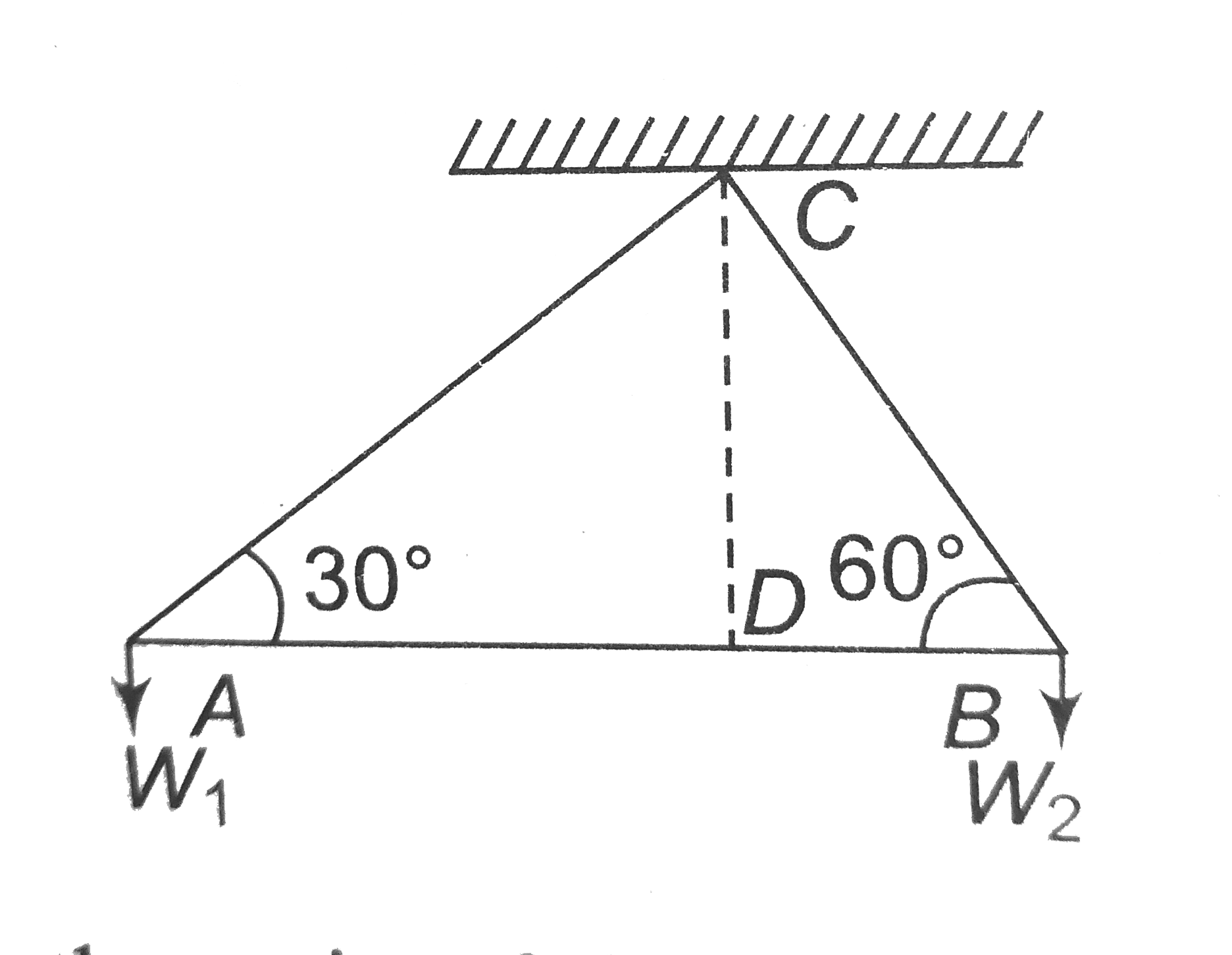 .
.
View SolutionA rigid massless rod `AB` of length `1 m` is placed horizontally on two rigid supports at its ends as showm in figure. A weight `10 N` is hung from a point `C` at a distance `30 cm` from `A`. Find the reactions at the supports `A` and `B` respectively.
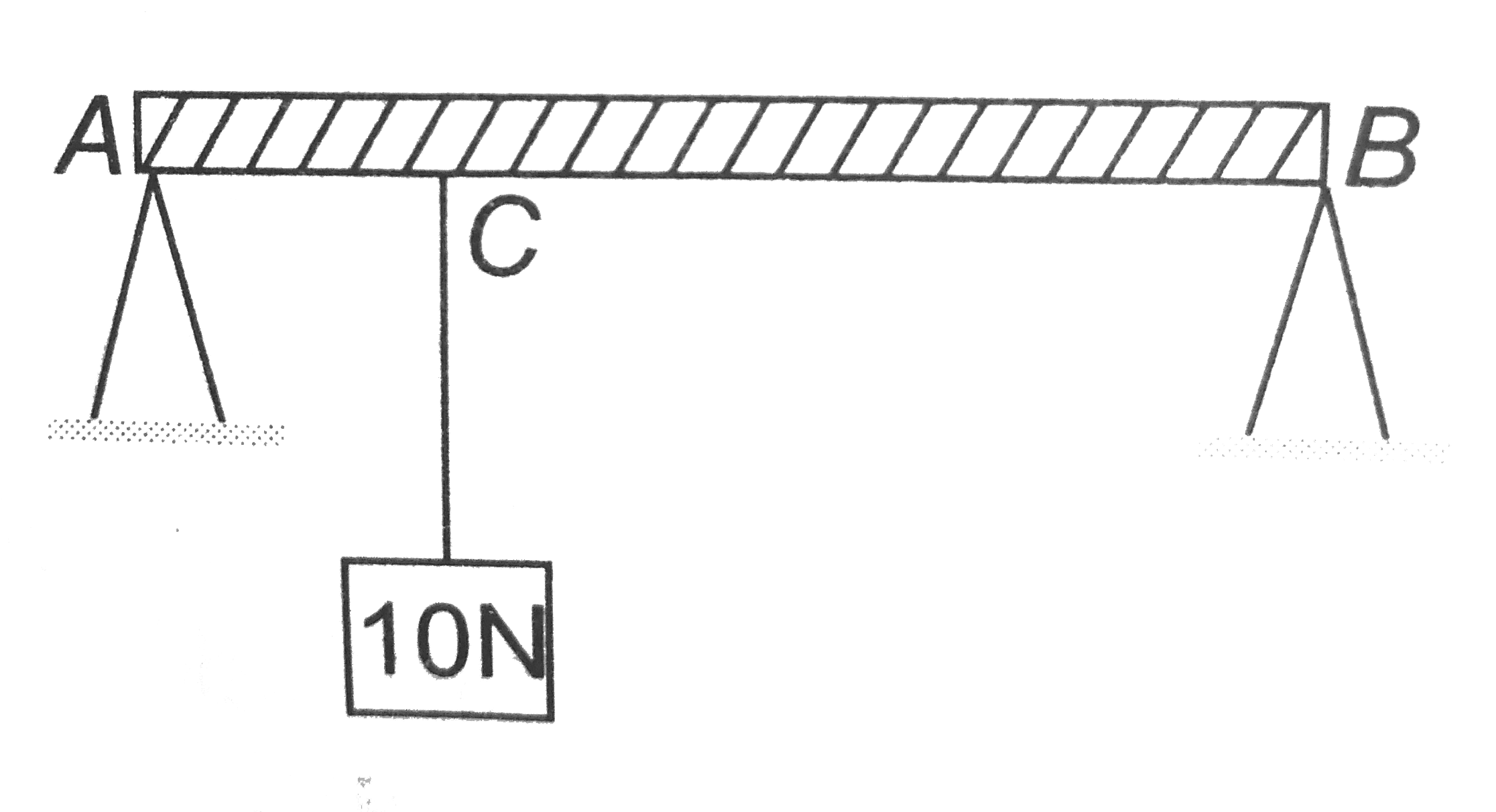 .
.
View SolutionSolve the above question if rod has a weight `20 N` uniformly distributed over its length.
View SolutionA rigid mass less rod of length `L` is hinged at its one end a weight `W` is hung at a distance `l(lt L)` from this end.
What is force `P` should be applied upwards at the other end so that the rod remains in equilibrium horizontally ?
 .
.
View SolutionIn previous problem, what is the reaction force on the hinge ?
View SolutionWhere must a `800 N` weight be hung on a uniform horizontal `100 N` pole of length `L` so that a body at one end supports one-third as much as a man at the other end ?
View SolutionA unifrom horizontal `200 N` beam `AB` length `L` has two weights hanging from it, `300 N` at `L//3` from end `A` and `400 N` at `3L//4` from the same end. What single additional force acting on the beam will produced equilibrium ?
View SolutionA weightless rod is acted on by upward parallel forces of `2 N` and `4N` ends `A` and `B` respectively. The total length of the rod `AB=3m`. To keep the rod in equilibrium a fo rce of `6 N` should act in the following manner :
View SolutionA false balance has equal arms. An object weights `W_1` when placed in one pan and `W_2` when placed in the other pan. The true weight `W` of the object is.
View SolutionThe beam and pans of a balance have negligible mass. An object weight `W_1` when placed in one pan and `W_2` when placed in the other pan. The weights `W` of the object is.
View SolutionIn first figure a meter stick, half of which is wood and the other half steel is pivoted at the wooden end at `A` and a force is applied at the steel and at `O`. On second figure the stick is pivoted at the steel end at `O` and the same force is applied at the wooden end at `A`. The angular acceleration.
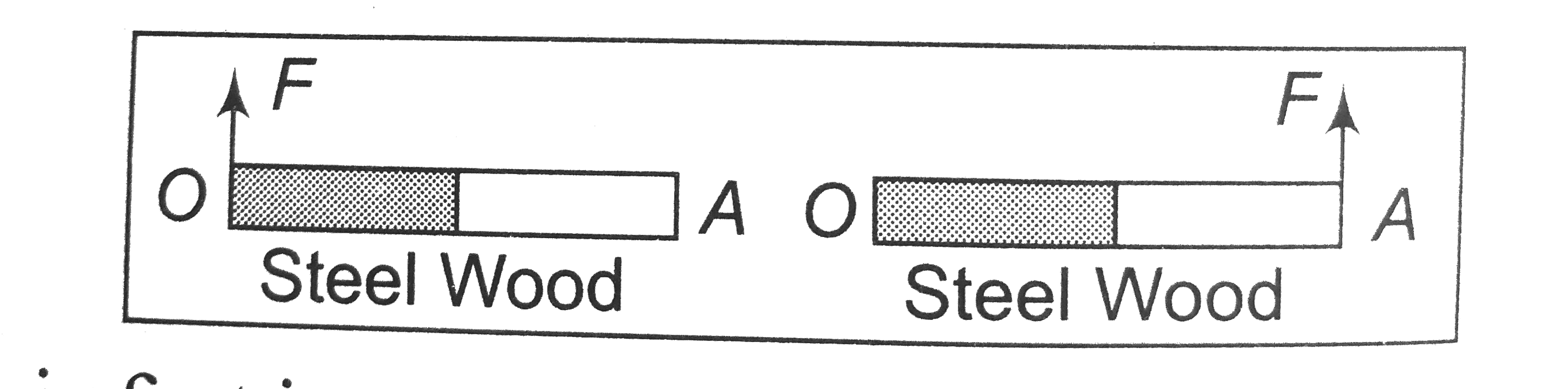 .
.
View SolutionA ring starts from rest and acquires an angular speed of `10 rads^-1` in `2 s`. The mass of the ring is `500 gm` and its radius is `20 cm`. The torque on the ring is.
View SolutionA wheel of moment of inertia `2.0 xx 10^3 kgm^2` is rotating at uniform angular speed of `4 rads^-1`. What is the torque required to stop it in one second.
View SolutionThe moment of inertia of an angular wheel shown in figure is `3200 kgm^2`. If the inner radius is `5 cm`, and the outer radius is `20 cm`, and the wheel is acted upon by the forces shown, then the angular acceleration of the wheel is.
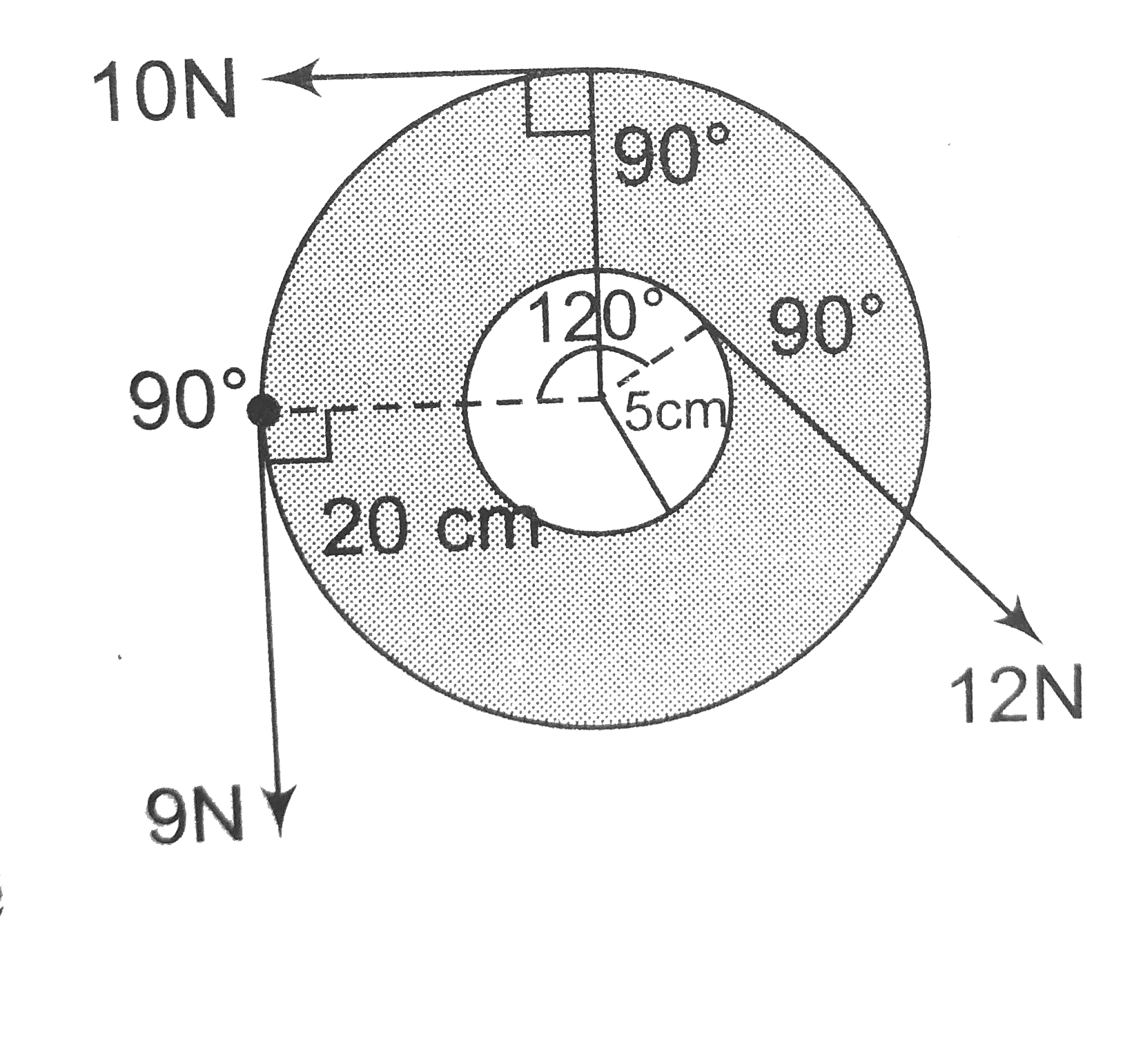 .
.
View SolutionA uniform disc of mass `M` and radius `R` is mounted on an axle supported in frictionless bearings. A light cord is wrapped around the rim of the disc and a steady downward pull `T` is exerted on the cord. The angular acceleration of the disc is
View SolutionIn previous problem, the tangential acceleration of a point on the rim is :
View SolutionIn `Q.137`, if we hang a body of mass `m` from the cord, the tangential acceleration of the disc is :
View SolutionIn `Q.137`, the tension in the cord in the above problem is :
View Solution
Torque , Torque Equation And Equilibrium Of A Rigid Body questions and complete solutions for chapter ROTATIONAL DYNAMICS of A2Z PHYSICS (HINGLISH) of Class 11
All Exercises from A2Z Class 11 PHYSICS ROTATIONAL DYNAMICS
- AIIMS Questions
- Angular Momentum And Its Conservation
- Assertion Reasoning
- Centre Of Mass
- Chapter Test
- Displacement , Velocity And Acceleration Of Centre Of Mass
- Moment Of Inertia
- NEET Questions
- Problems Based On Mixed Concepts
- Rotation And Translation Combined And Rolling Motion
- Rotational Kinematics
- Torque , Torque Equation And Equilibrium Of A Rigid Body
- Work And Energy In Case Of Rotation