Complete Solutions to Matching type of chapter FORCE ANALYSIS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | FORCE ANALYSIS |
| ANURAG MISHRA | Matching type |
| Number Of Questions | 13 |
| Academic Year | 2023-24 |
A motorcycle moves around a vertical circle with a constants speed under the influence of the force of gravity `vec(W)`, friction between wheel and track `vec(f)` and normal reaction between wheel track `vec(N)` :
`{:(,"Column-1",,"Column-2"),("(A)","Constant magnitude","(P)",vec(N)),("(B)","Directed towards centre when value in non-zero","(Q)",vec(N)+vec(f)),("(C)","Total reaction force by track","(R)",vec(f)+vec("w")),("(D)","When motion is along vertical the value is zero","(S)",vec(N)+vec("w")+vec(f)):}`
View SolutionA block is projected with an initial velocity `v_("Block")` on a long conveyor belt moving with velocity `v_("Block")` (at that instant)having constant acceleration `a_("Belt")`. Mark the correct option regarding friction after long time (friction coefficient between block and belt `= mu`). If :
`{:(,"Column-1",,"Column-2"),("(A)",v_("Block")=2v_("Belt")and a_("Belt")=0,"(P)","zero"),("(B)",v_("block")=2v_("Belt")and a_("Belt")gt mug,"(Q)",f_(s)"static friction" (0 lt f_(s) lt f_(L))),("(C)",v_("Block")=2v_("Belt")and a_("Belt")=mug,"(R)",f_(L)"limiting friction"),("(D)",v_("Block")=2v_("Belt")and a_("Belt")lt mug,"(S)",f_(K) "kinetic friction"):}`
View SolutionThe inclined surface is rough `mu = (1)/(2)`. For different values of m and M system slides down or up the plane or remains stationary. Match the appropriate entries of column-1 with those of column-2
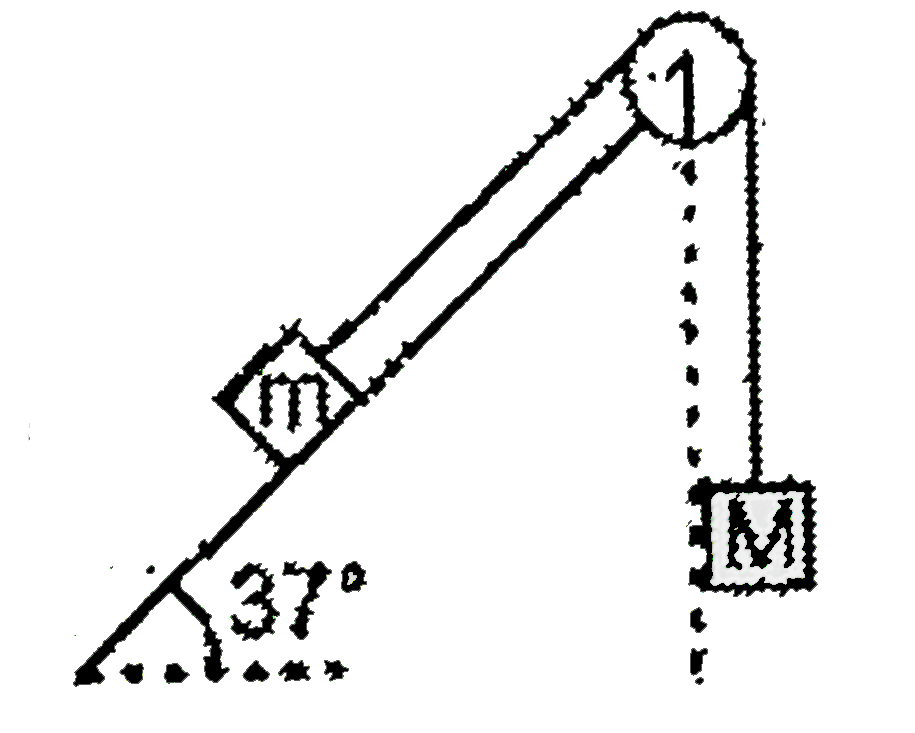
`{:(,"Column-1",,"Column-2"),("(A)","Minimum value of " (m)/(M) "so theta m slides down","(P)",(5)/(3)),("(B)","Minimum valie of " (M)/(m)"so that m slides up","(Q)",1),("(C)","Value of" (m)/(M) "so that friction force on m is zero","(R)",(3)/(5)),("(D)",underset("and acceleration of M")"Ratio of vertical component of acceleration of m","(S)",5):}`
View SolutionA river is flowing with speed 3 km/hr west to east. A man swims with speed 5 km/hr in still water. Man is at south bank of the river. Match the column-1 with direction of velocities of man w.r.t. ground in column-2
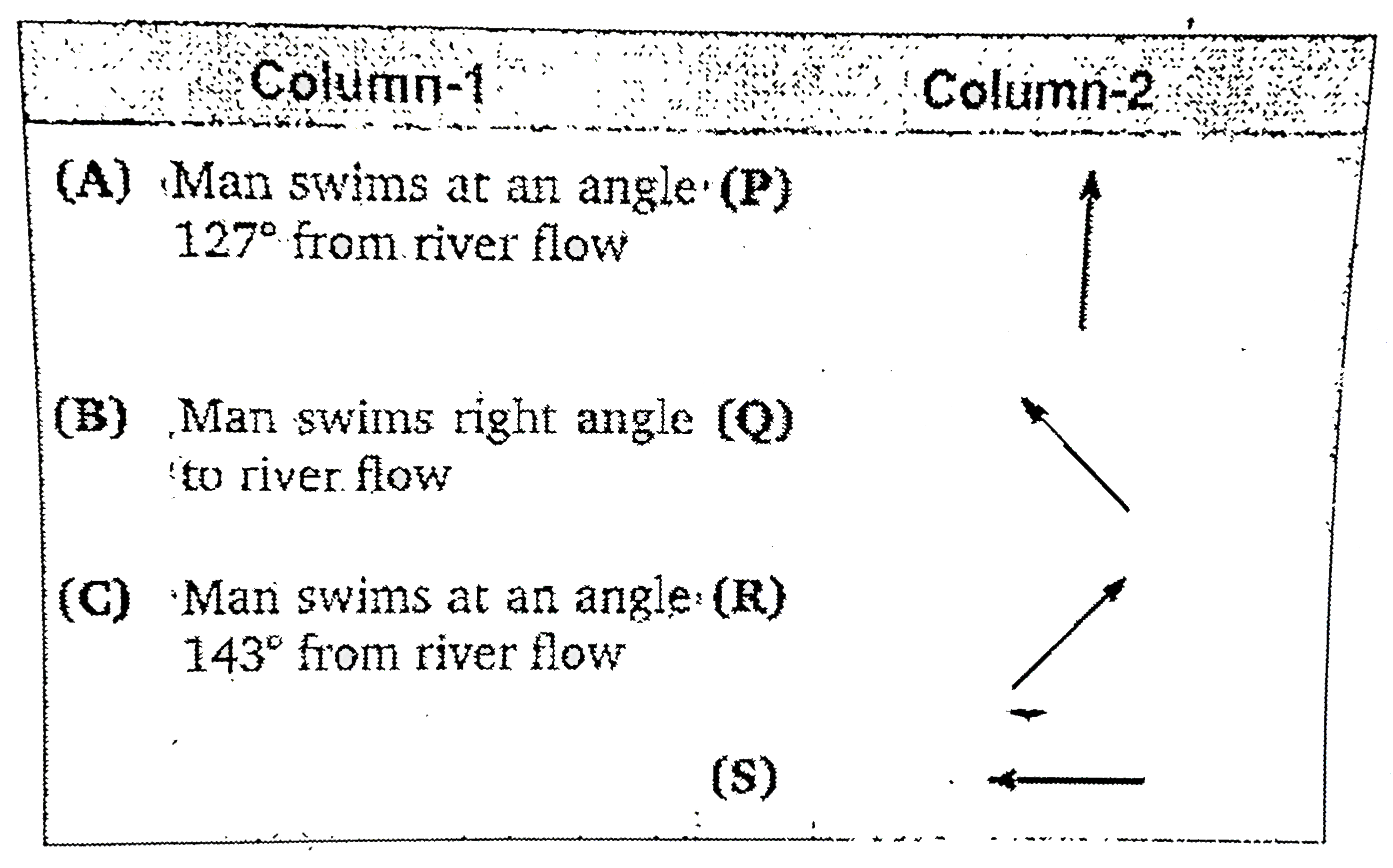
View SolutionA particle is moving on a straight line. It is initially at rest
`v=` instantaneous velocity
P = instantaneous power S = displacement
F= force t = time
Match the possible expression of the quantities in column-1 with the situation in column-2
`{:(,"Column-1",,"Column-2"),("(A)",v^(3) prop S,"(P)","P = constant"),("(B)",v^(2) prop t,"(Q)",P prop v),("(C)",v^(2) prop S,"(R)","F = constant"),("(D)",v prop t,"(S)",F prop (1)/(v)),(,,"(T)",P prop t):}`
View SolutionMatch the column:

`|{:(,"Column -1",,"Column -2"),((A),theta=37^(@),(P),f" is upwards"),((B),theta=45^(@),(Q),f" is downwards"),((C),theta=53^(@),(R),f" is static"),(,,(S),f" is kinetic"):}|`
View SolutionLift can move in y-axis as well as along x-axis. A ball of mass m is attached to ceiling of lift with inetensible light rope and box of mass mis placed against a wall as shown in figure. Neglect friction everywhere.

`|{:(,"Column -1",,"Column -2"),((A),"In figure lift is moving along x-axis",(P),"zero"),(,"then, value of T may be",,),((B),"Lift moving toward right along",(Q),gt mg),(,"x-axis with decreasing speed then",,),(,"value of N may be",,),((C),"Lift is moving in upward direction",(R),lt mg),(,"(y-axis) then value of T may be",,),((D),"Lift is moving in downward",(S),=mg),(,"direction with constant velocity",,),(,"then value of T may be",,):}|`
View SolutionIn the diagram strings, springs and the pulley are light and ideal. The system is in equilibrium with the strings taut `(T gt 0)`, match the column. Masses are equal.
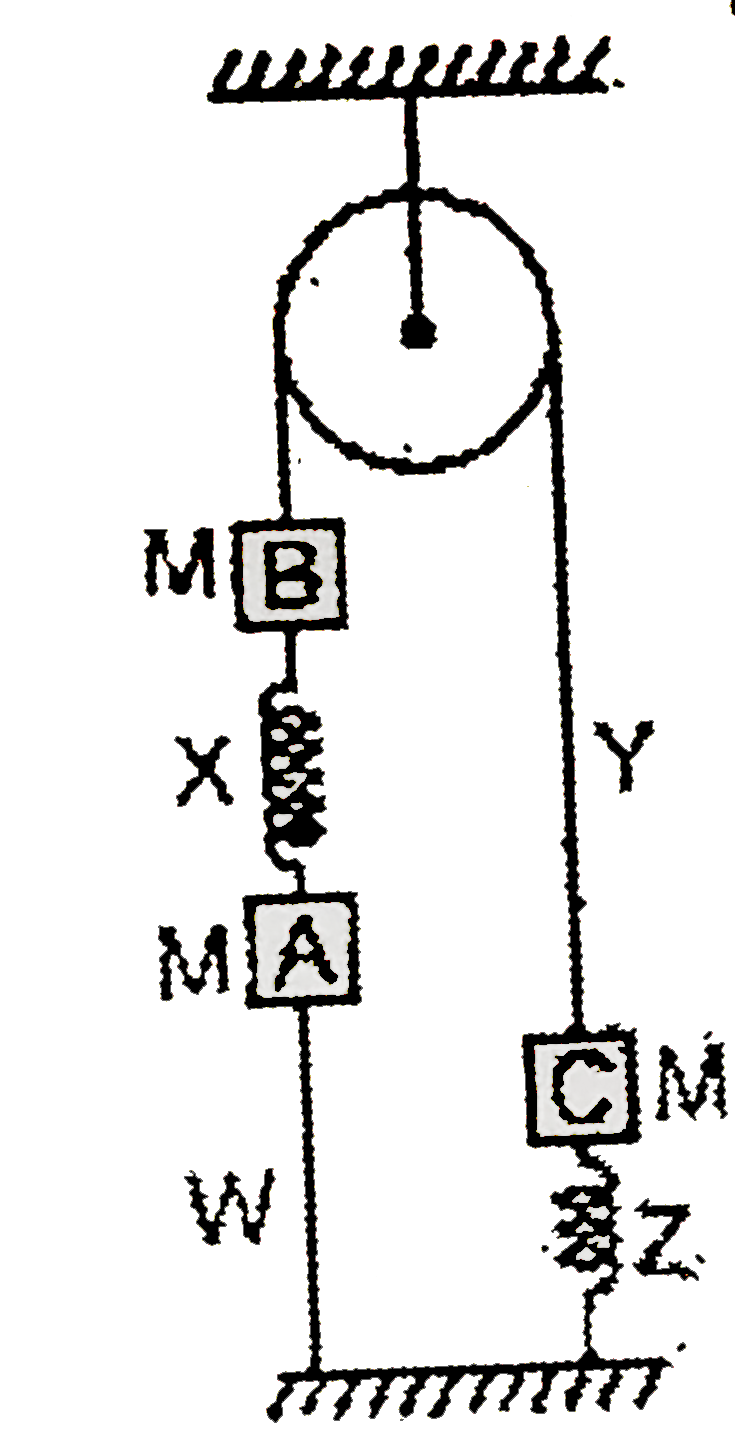
`|{:(,"Column -1",,"Column -2"),((A),"Just after string W breaks",(P),a_(A)=0),((B),"Just after spring X breaks",(Q),a_(B)=0),((C),"Just after string Y breaks",(R),a_(C)=0),((D),"Just after spring Z breaks",(S),a_(B)=a_(C)):}|`
View SolutionIn the situation shown, all surfaces are frictionless and triangular wedge is free to move. In column-2, the direction of certain vectors are shown. Match the direction of quantities in column-1 with possible vector in column-2.
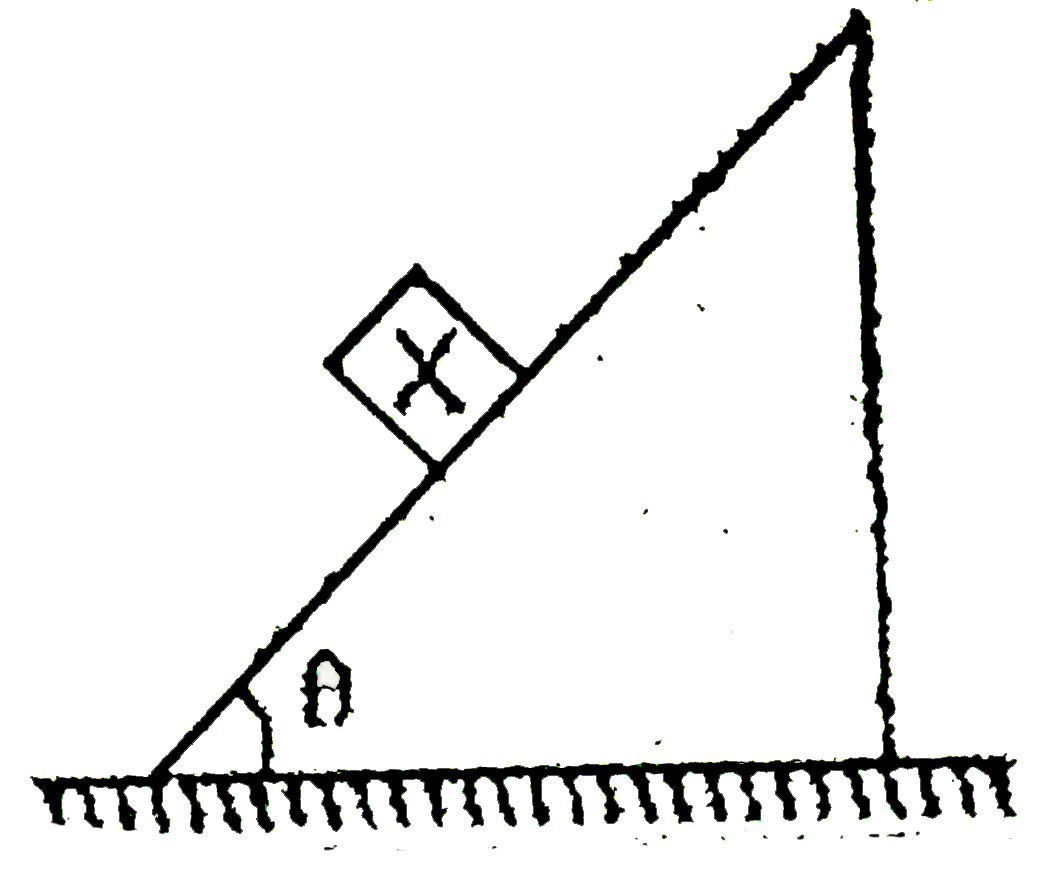

View SolutionSee the diagrams carefully in Column-1 and match each with the obeying relation (S) in column-2. The string is massless, inextensible and pulley is frictionless in each case. a=g/3, m= mass of block T = tension in a given string, `a_("pulley") =` acceleration of movable pulley in each case, acceleration due to gravity is g.

View SolutionA block is placed on a rough horizontal surface. A constant force F is acting on the block as shown in the figure.
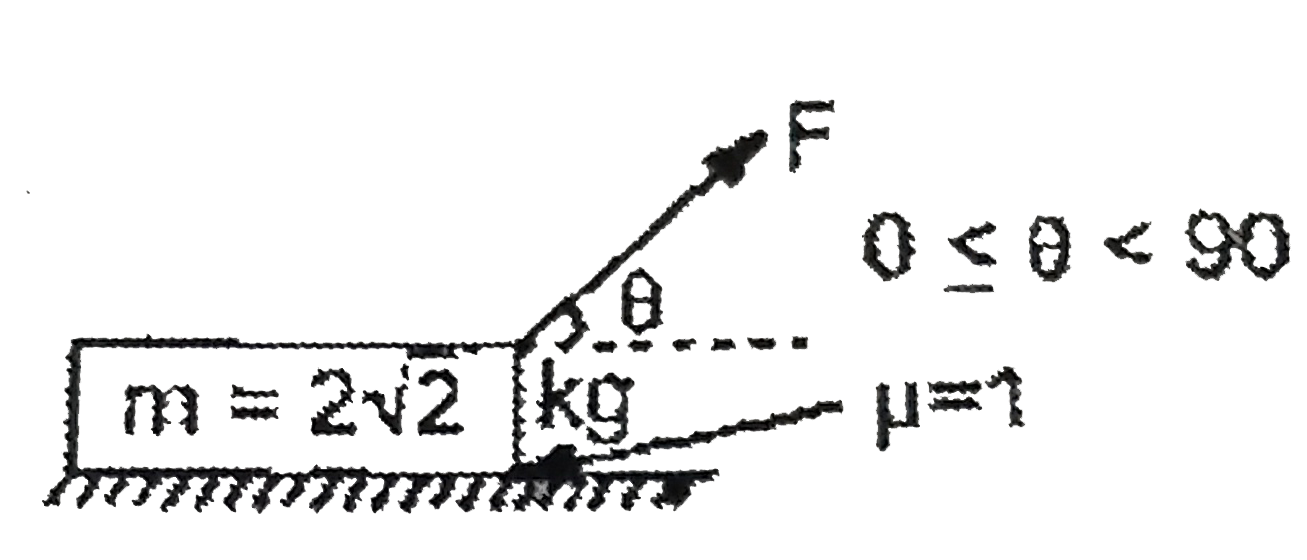
Column-1 gives the magnitude of force F and column-2 gives information about friction acting on the block. Match the entries in column-1 to all possible entries in column-2.
View SolutionColumn-1 shows certain siruations and column-2 shows information about forces.
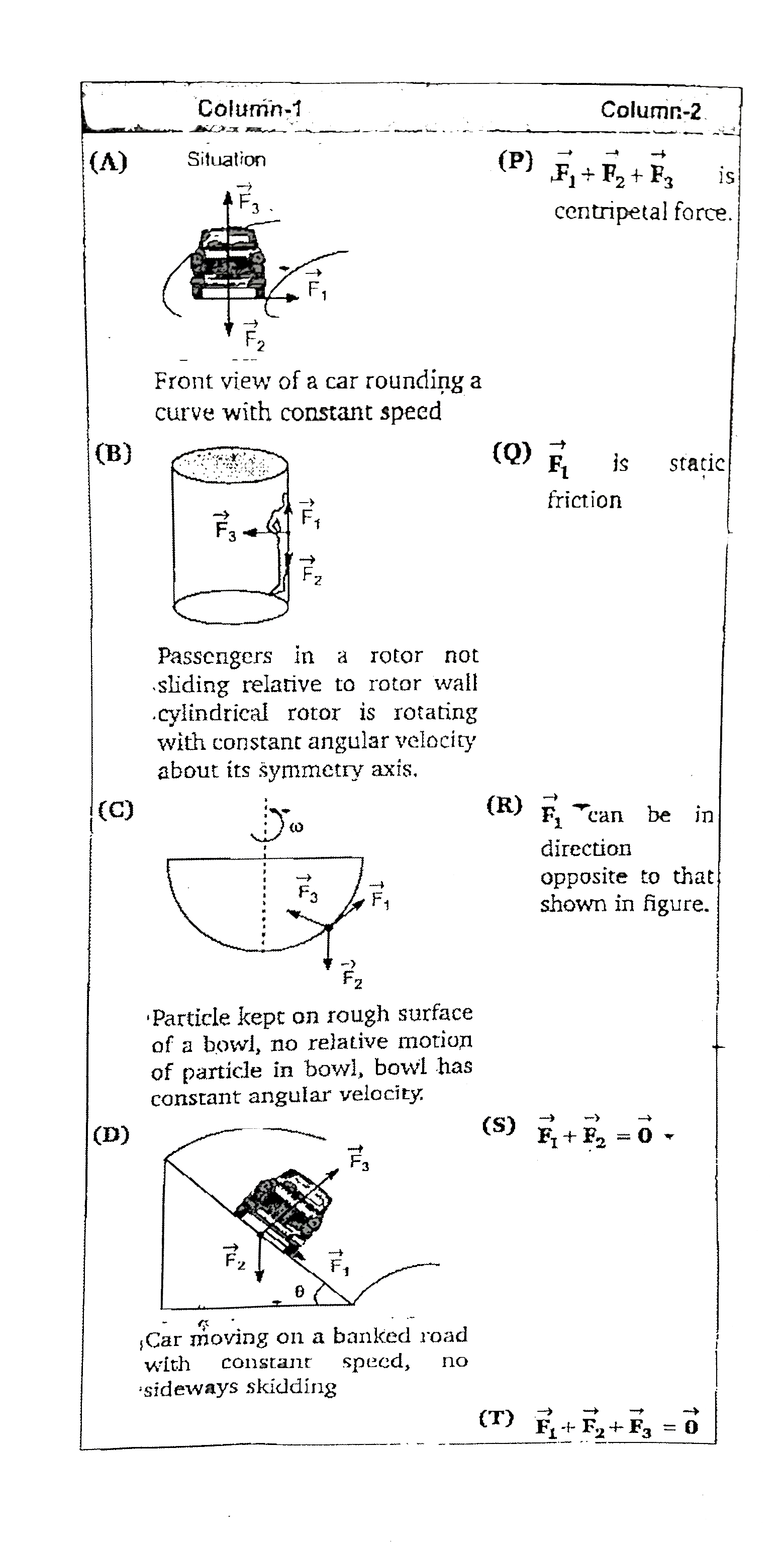
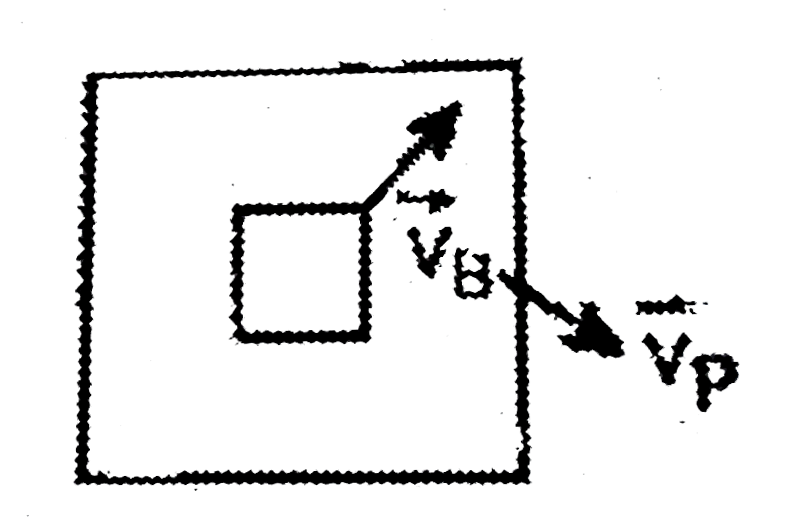
View SolutionFind unit vector in direction of friction force acting on block
`vecv_p=7hati-2hatj, vecv_B=3hati+hatj`
View Solution
Matching type questions and complete solutions for chapter FORCE ANALYSIS of SHREE BALAJI PHYSICS (HINGLISH) of Class 11