Complete Solutions to Level 2 Subjective of chapter WORK, ENERGY & POWER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK, ENERGY & POWER |
| DC PANDEY | Level 2 Subjective |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
Two block of masses `m_(1)` and `m_(2)` connected by a light spring rest on a horixontal plane. The cofficient of friction between the block and the surface is equal to `mu`. What minimum constant force has to be applied in the horizontal direction to the block of mass `m_(1)` in order to shift the other block?
View SolutionThe flexible bicycle type chain of length `(pir)/2` and mass per unit length `rho` is released from rest with `theta =0^@` In the smooth circular channel and falls through the hole in the supporting surface, Determine the velocity v of the chain as the last link leaves the slot.
 .
.
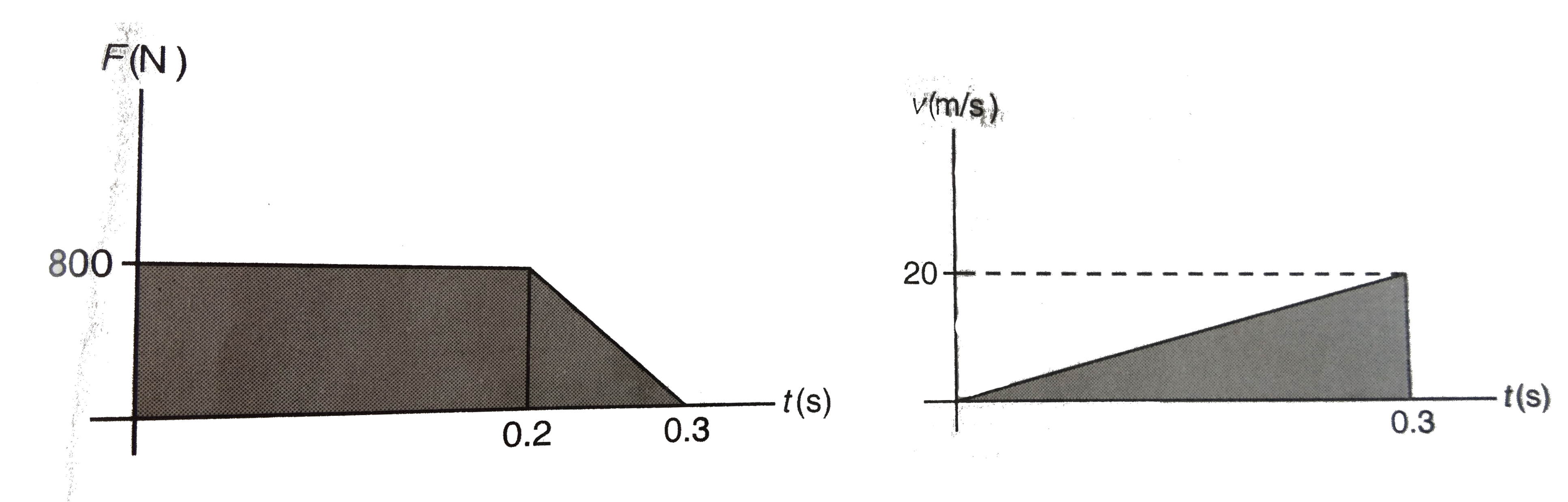
View SolutionA baseball having a mass of `0.4 kg` is thrown such that the force acting on it varies with time as shown in the first graph. The correspondeng velocity time gragh is shown in the second graph. Detemine the power applied as a functon time and the work done till `t=0.3 s`.
View SolutionA chain (AB) of length `l` loaded in a smooth horizontal table so that its fraction of length h hangs freely and toucher the surface of the table with its end B. At a certain moment ,the end A of the chain is set free. With what velocity will this end the chain slip out of the table ?
 .
.
View SolutionThe block shown in the figure is acted on by a sping with spring constant (k) and (a) weak frictional force of constant of constant magitude (f.) The block is pulled a distance `x_(0)` from equilibrium position and then then then fren released. It oscillates many times ultimately comes to rest.
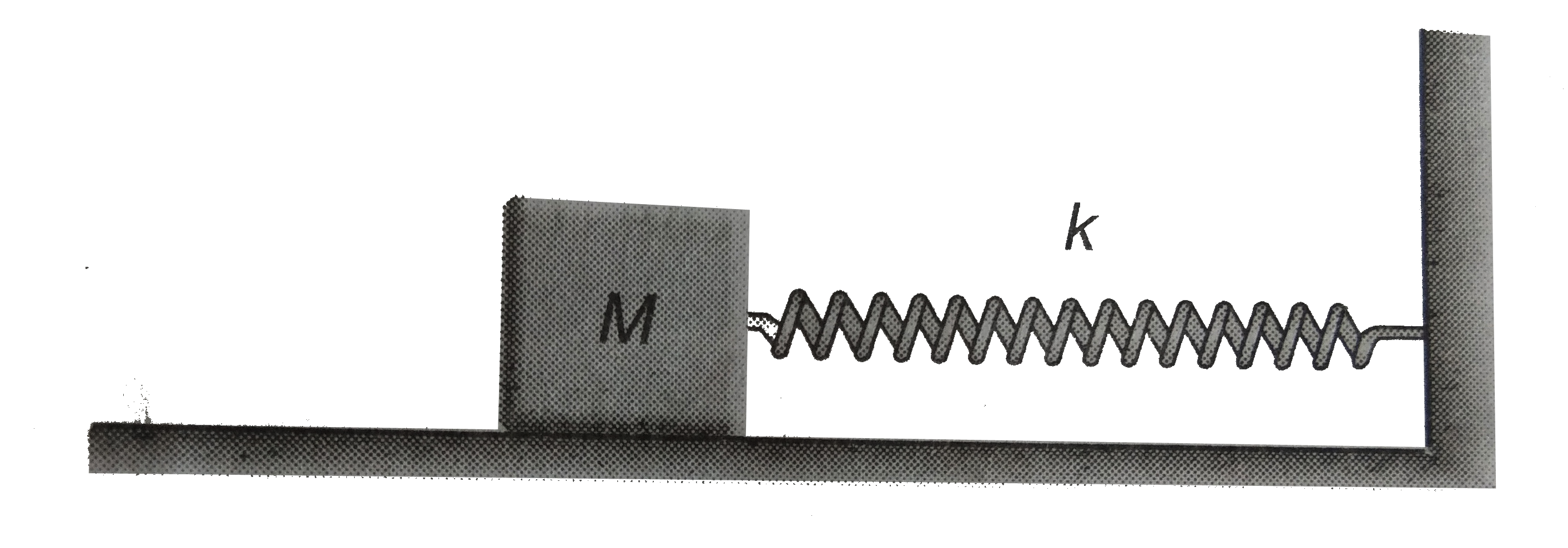
(a) Show that the decrease of amplitude is the same for each cycle of lscillation.
(b) Find the number of cycles the mass oscillates before coming to rest.
View SolutionA spring mass system is held at rest with the spring relaxed at a height (H) above the ground. Determine the minimum value of (H) so that the systen has a tendency to rebound after hitting the ground. Given that the coefficient of restitution between `(m_(2))` and ground is zero.
 .
.
View SolutionA block of mass m moving at a speed v compresses a spring throgh a distance x before its speed is halved. Find the spring constant of the spring.
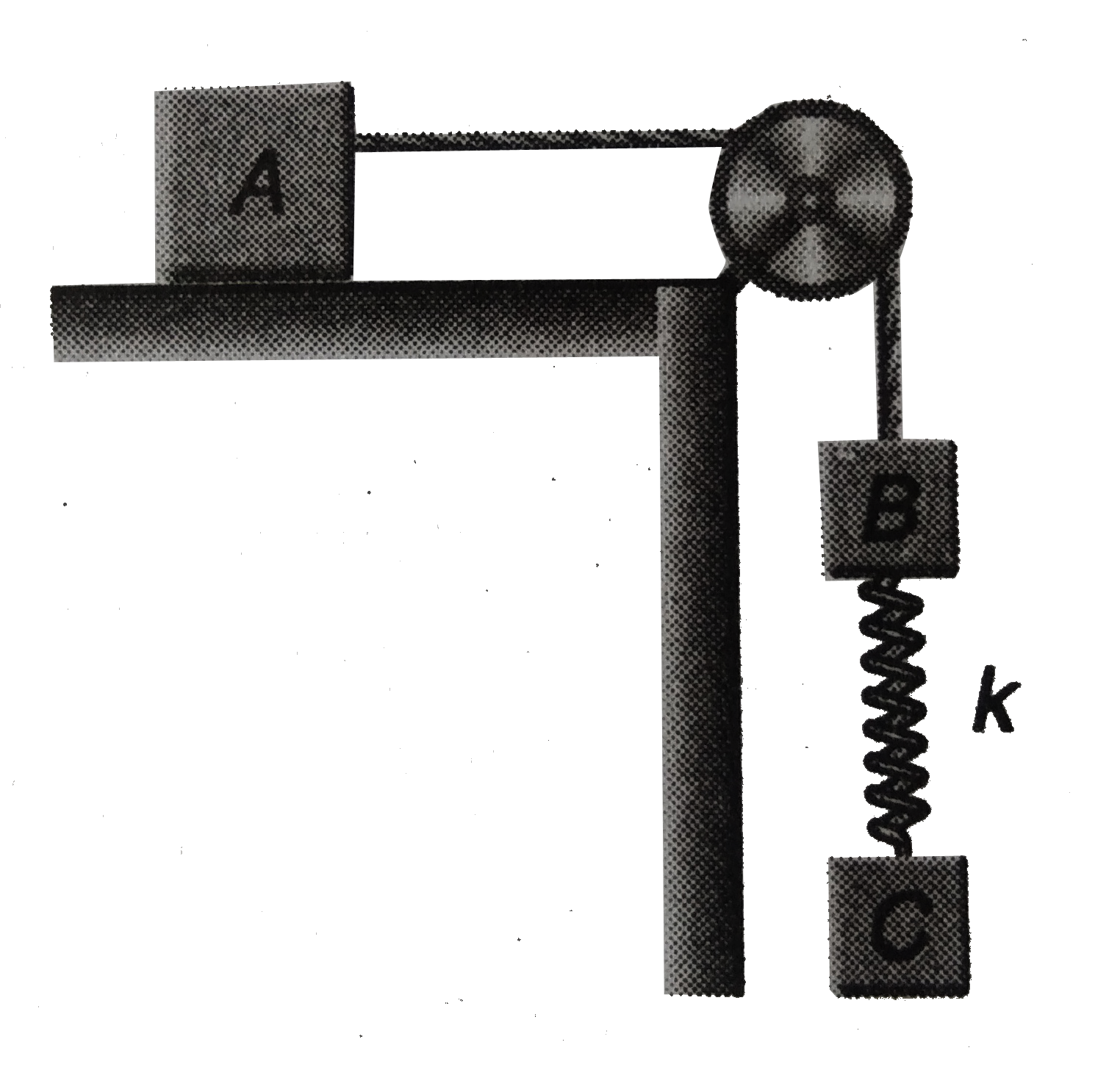
View SolutionIn the figure shown masses of the blocks A, B and C are 6kg, 2kg and 1kg respectively. Mass of the spring is negligibly small and its stiffness is 1000 N//m. The coefficient of friction between the block A and the table is `mu =0.8`. Initially block C is held such that spring is in relaxed position. The block is released from rest. Find `(g=10 m//s^(2))`.
View SolutionA body of mass m slides down a plane inclined at an angle `alpha`. The coefficient of friction is `mu`. Find the rate at which kinetic plus gravitational potential is dissipated at any time t.
View SolutionA particle moving in a straight line is acted upon by a force which works at a constant rate and changes its velocity from (u and v ) over a distance x. Prove that the taken in it is
`3/2 (u+v)x/(u^(2)+v^(2)+uv)` .
View SolutionA chain of length l and mass m lies on the surface of a smooth sphere of radius R>l with one end tied to the top of the sphere.
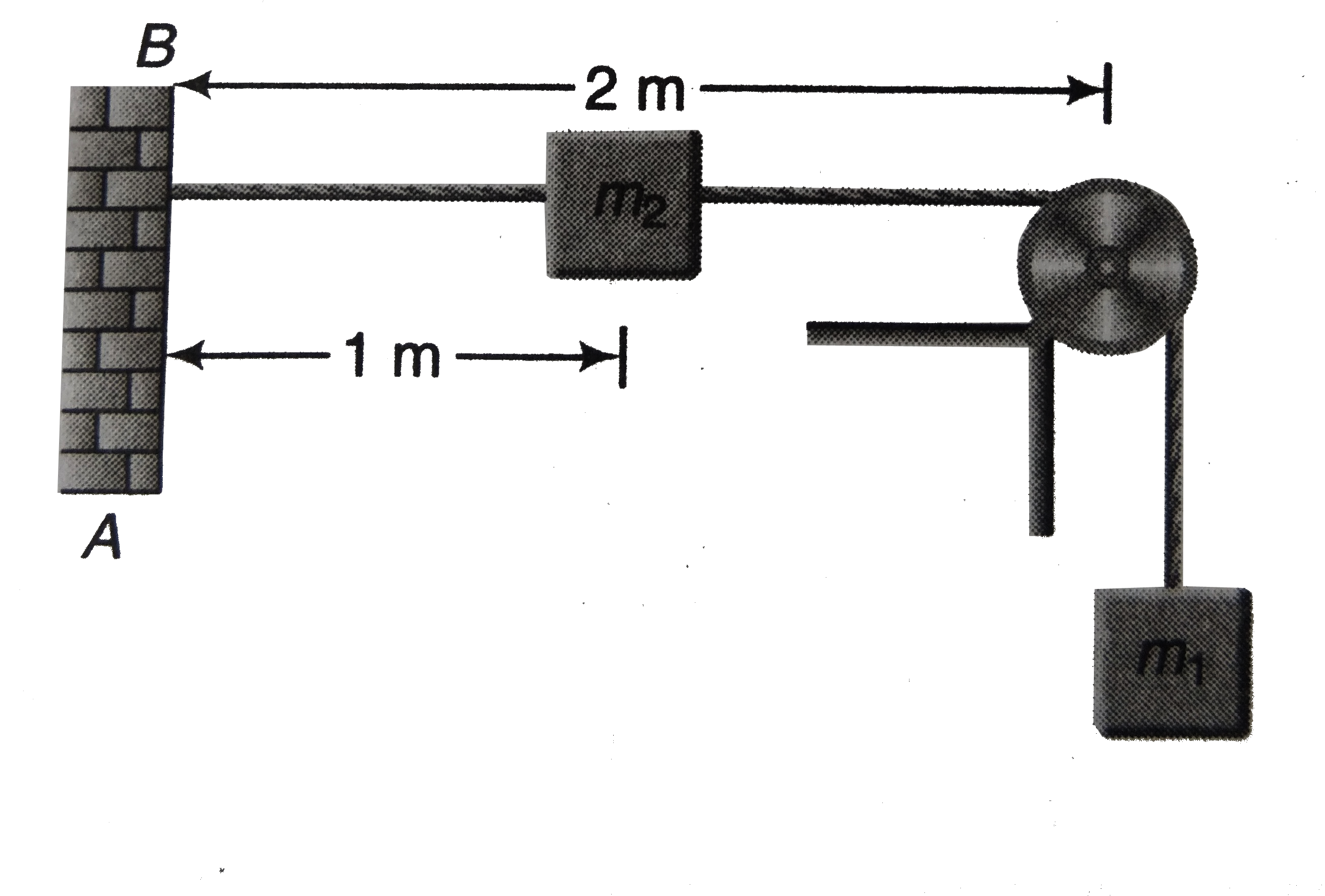
View SolutionFind the speed of both the blocks at the moment the block `m_(2)` hits the wall AB, after the blocks are released from rest. Given that `m_(1) =0.5 kg` and `m_(2) =2 kg, (g=10 m//s^(2))`
 .
.
View SolutionA block of mass M slides along a horizontal table with speed `v_(0)`. At `x=0`, it hits a spring with spring constant k and begins to experience a friction force. The coefficient of friction is variable and is given by `mu=bx`, where b is a positive constant. Find the loss in mechanical energy when the block has first come momentarily to rest.
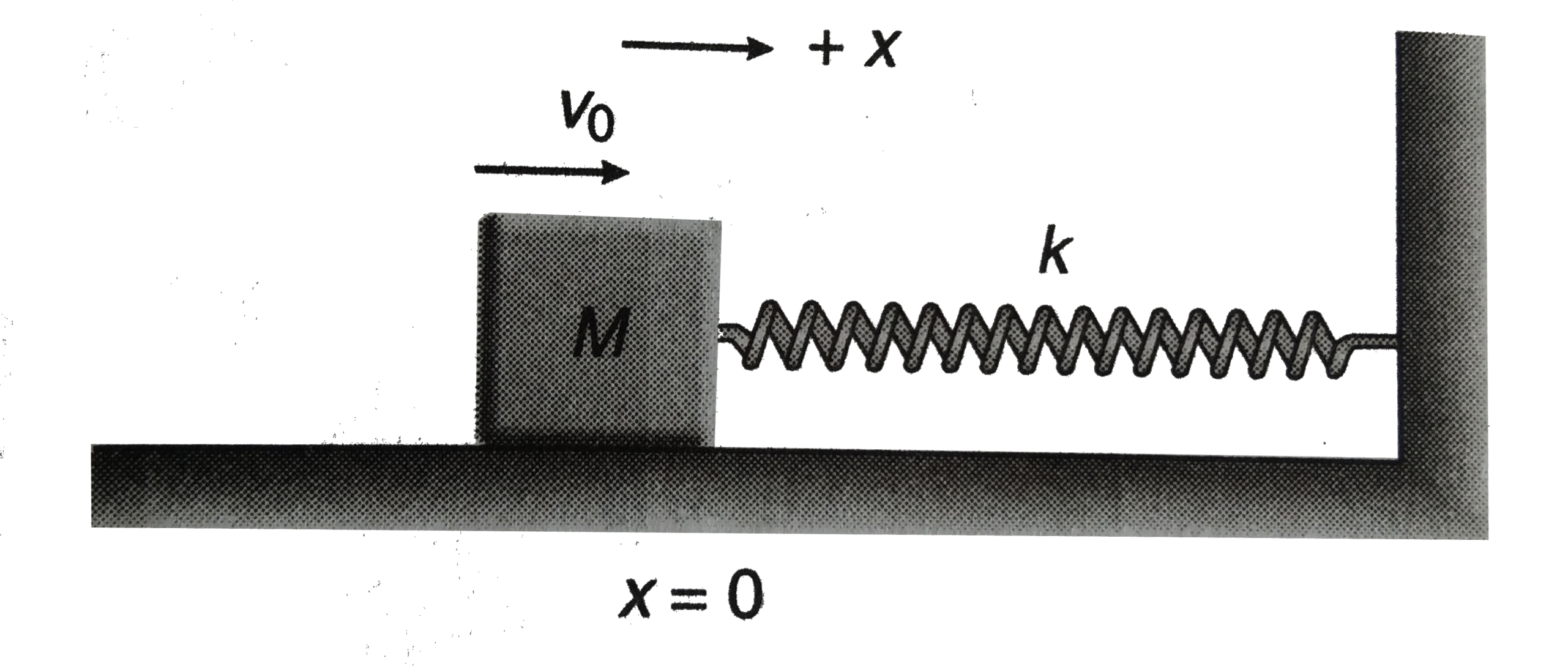 .
.
View SolutionA small block of ice with mass `0.120 kg` is placed against a horizontal compressed spring mounted on a horizonta table top that is `1.90 m` above the floor. The spring has a force constant `k=2300 M//m` and is initially compressed `0.045 m`. The mass of the spring is negligible. The spring is releaded and the block slock slides along the table, goes off the edge and travels to the floor. If there is negligible friction between the ice and the table, what is the speed of the block of ice when it reaches the floor. `(g=9.8 m//s^(2))`
 .
.
View SolutionA `0.500 kg` block is attached to a spring with length `0.60m` and force constant `k=40.0 N//m`. The mass of the spring is negligible. You pull the block to the right along the surface with a constant horizontal force `F=20.0 N`. (a) What is the block's speed when the block reaches point B,which is `0.25 m` to the right of right of point A? (b) What the block reaches point B, you let go off the block. In the subsequent motion, how close does the block get to the wall where the lift end of the spring is attached? Neglect size of block and friction.
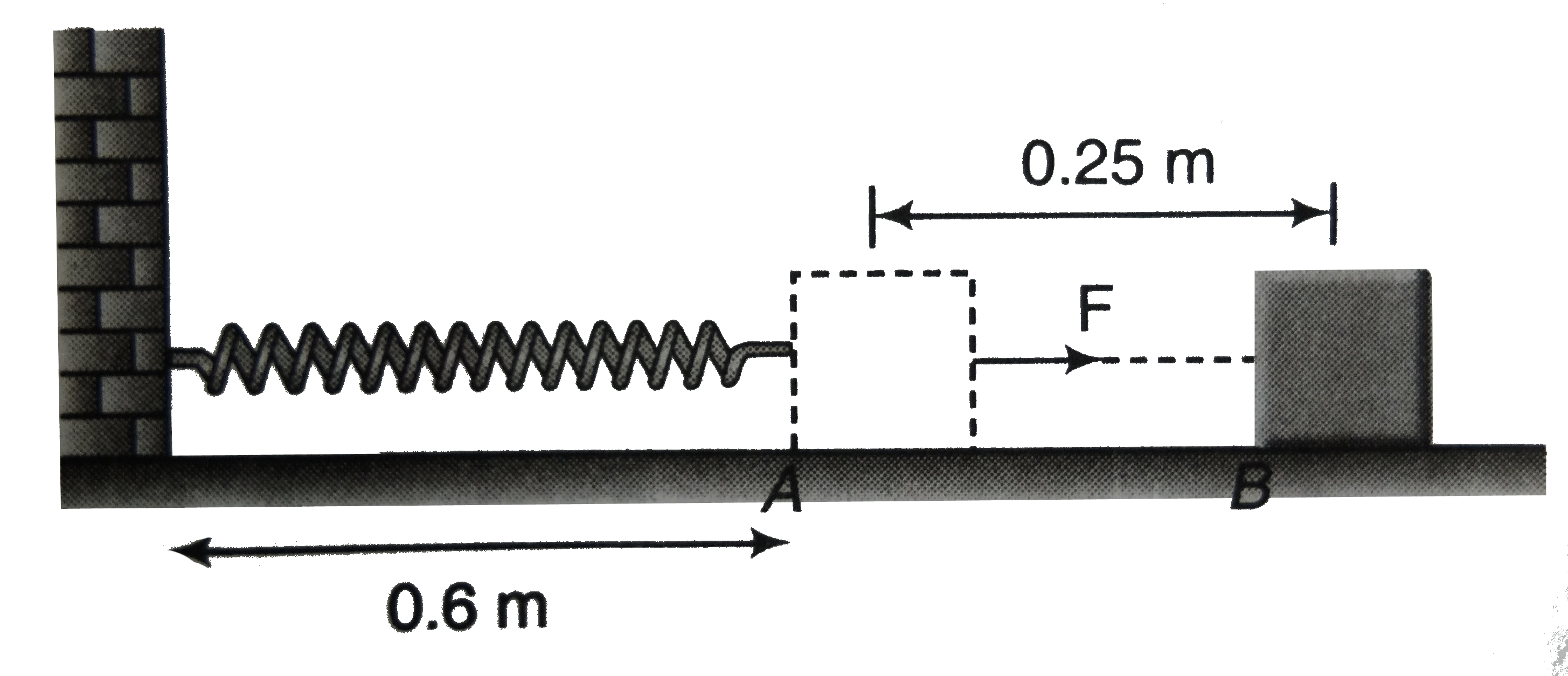 .
.
View Solution
Level 2 Subjective questions and complete solutions for chapter WORK, ENERGY & POWER of DC PANDEY ENGLISH of Class 11