Complete Solutions to Solved Examples of chapter WORK, ENERGY & POWER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK, ENERGY & POWER |
| DC PANDEY | Solved Examples |
| Number Of Questions | 12 |
| Academic Year | 2023-24 |
In the figure shown, all surfaces are smooth and force constant of spring is `10N//m`. Block of mass (2 kg) is attaced with the spring. The spring is compressed by (2m) and then released. Find the maximum distance `d` travelled by the block over the inclined plane. Take `g=10m//s^(2)`.
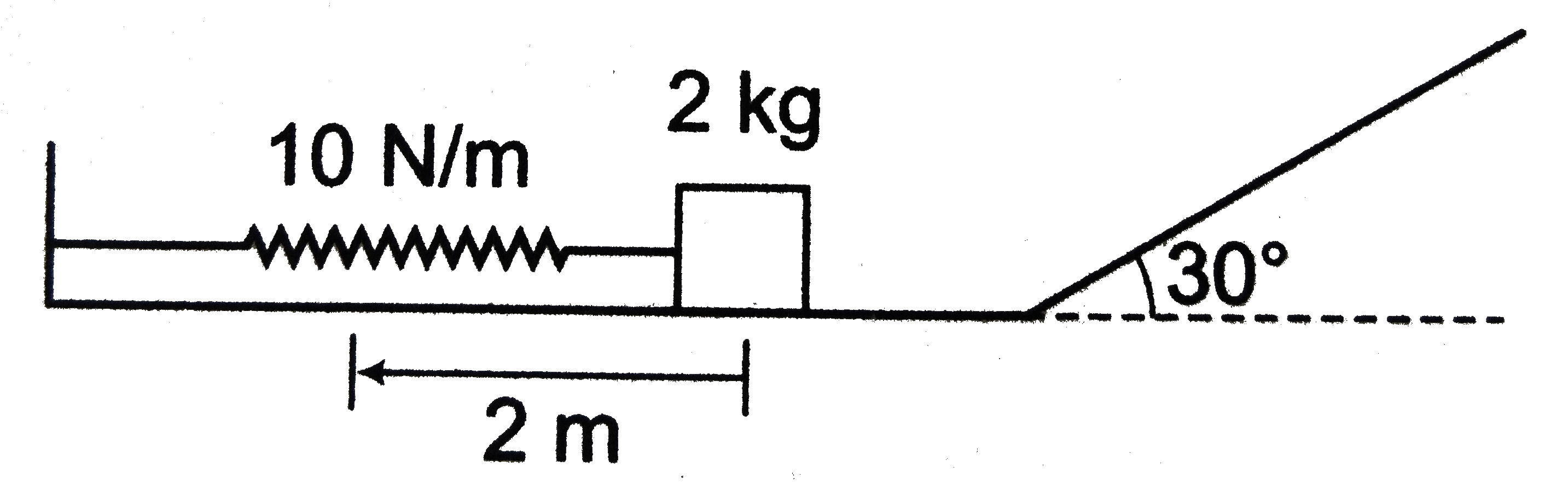 .
.
View SolutionA smooth narrow tube in the form of an arc AB of a circle of centre O and radius r is fixed so that A is vertically above O and OB is horizontal. Particles P of mass m and Q of mass 2 m with a light inextensible string of length (pi r//2) connecting them are placed inside the rube with P at A and Q at B and released from rest. Assuming the string remains taut during motion, find the speed of particles when P reaches B.
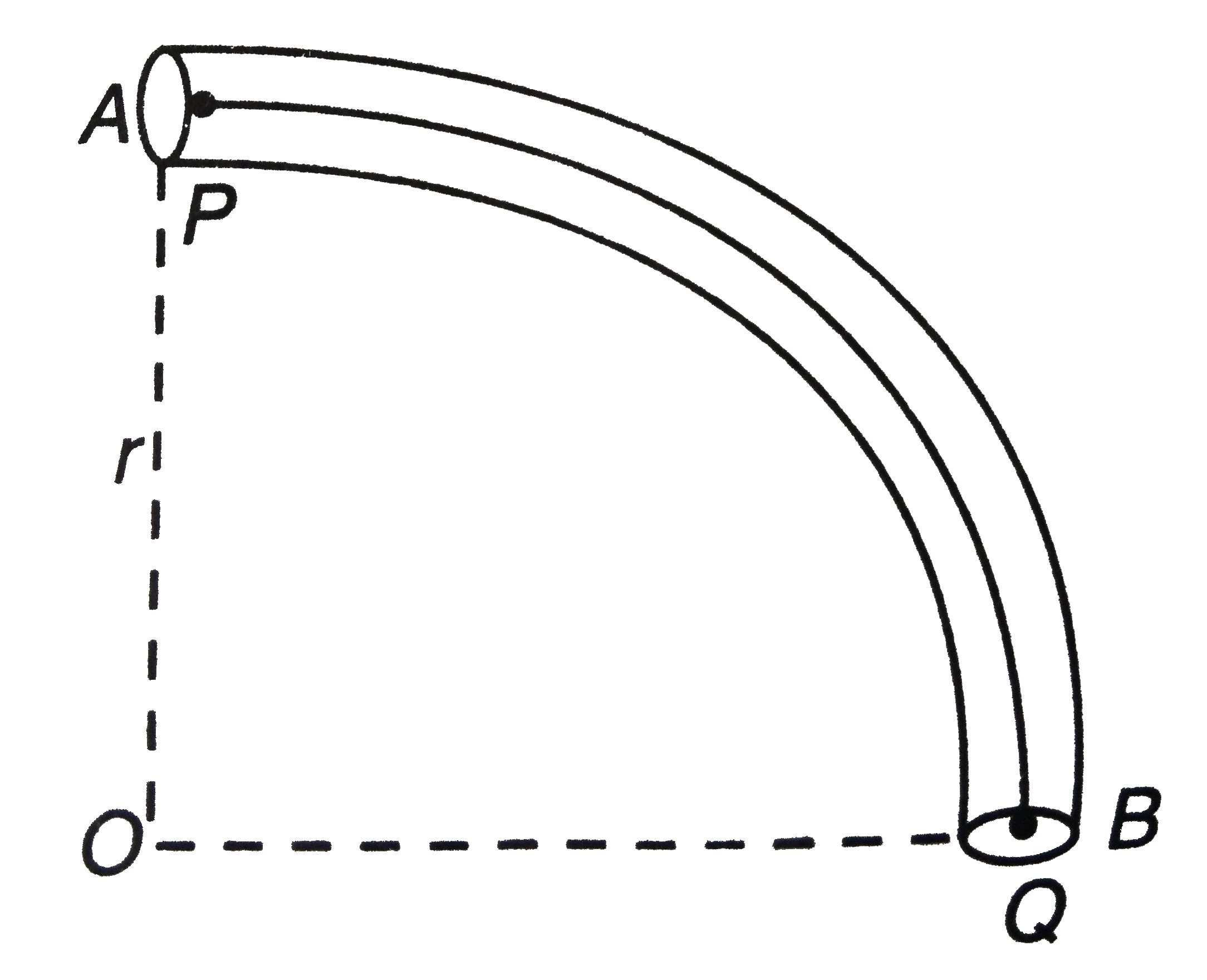 .
.
View SolutionOne end of a light spring of natural length d and spring constant k is fixed on a rigid wall and the other is attached to a smooth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the wall. Initially the spring makes an angle of `37^(@)` with the horizontal as shown in fig. When the system is released from rest, find the speed of the ring when the spring becomes horizontal.
[ sin `37^(@)` = 3/5]
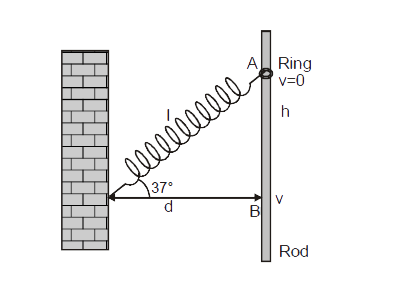
View SolutionIn the adjoining figure, block A is of mass (m) and block B is of mass 2m. The spring has force constant k. All the surfaces are smooth and the system is released form rest with spring unstretched. Find the maximum extension in the spring and acceleration of block B at time of maximum extension
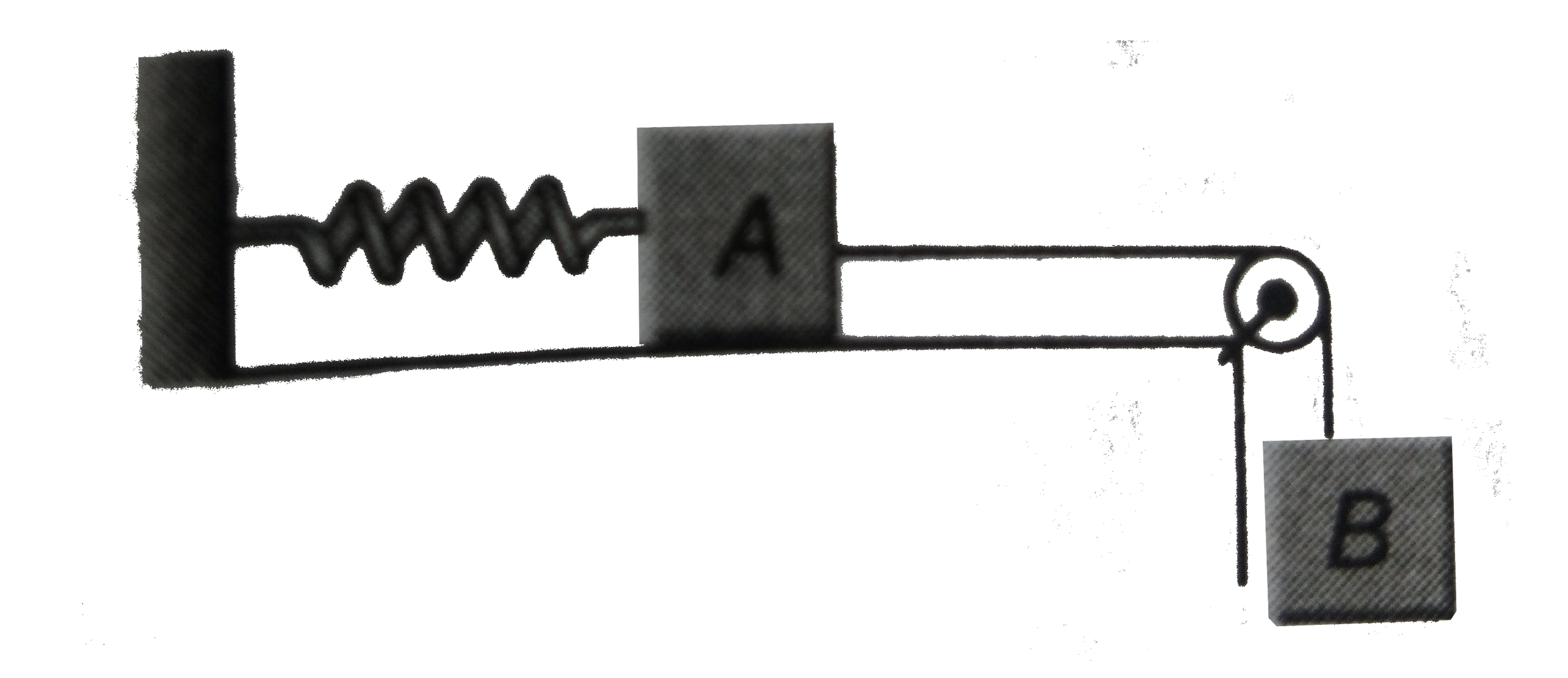 .
.
View Solution .
.
In the figure shown, `AB = BC = 2m`. Friction coefficient everywhere is `mu=0.2.` Find the maximum compression of the spring.
View SolutionA particle slides along a track with elevated ends and a flat central part as shown in figure. The flat part has a length `l=3m`. The curved portions of the track are frictionless. For the flat part, the coefficient of kinetic friction is `mu_k=0.2`. The particle is released at point A which is at height `h=1.5m` above the flat part of the track. Where does the particle finally come to rest?

View SolutionA `0.5kg` block slides from the point A on a horizontal track with an initial speed `3 m//s` towards a weightless horizontal spring of length `1m` and force constant `2N//m.` The part AB of the track is frictionless and the part BC has the confficient of static and kinetic friction as `0.20` respectively. If the distancences AB and BD are `2m` and `2.14m` respectively, find total distance through which the block moves before it comes to rest completely. `(g=10 m//s^(2) ).
View SolutionA body is displaced from origin to `(2m, 4m)` under the following two forces:
(a) `F=(2^hati + 6^hatj)N`, a constant force
(b) `F(2x^hati + 3y^(2)hatj)N`
Find work done by the given forces in both cases.
View SolutionA force `F=-k(^hati + x^hatj)` (where k is a positive constant) acts on a particle moving in the `x-y` plane. Starting from the origin, the particle is taken along the positive x-axis to the point `(a,0)` and then parallel to the y-axis to the point `(a,a)`. The total work done by the force F on the particle is
(a) `-2ka^(2)` , (b) `2ka^(2) , (c)`-ka^(2)` , (d) `ka^(2)`
View SolutionA body is displaced from prigin to (1m,1m) by force
`F=(2yhati + 3x^(2)hatj)` along two paths
(a) `x=y` (b) `y=x^(2)`
Find the work done along both paths.
View SolutionA particle, which is constrained to move along x-axis, is subjected to a force in the some direction which varies with the distance x of the particle from the origin an `F (x) =-kx + ax^(3)`. Here, k and a are positive constants. For `x(ge0,` the functional form of the potential energy (u) U of the U (x) the particle is.
(a)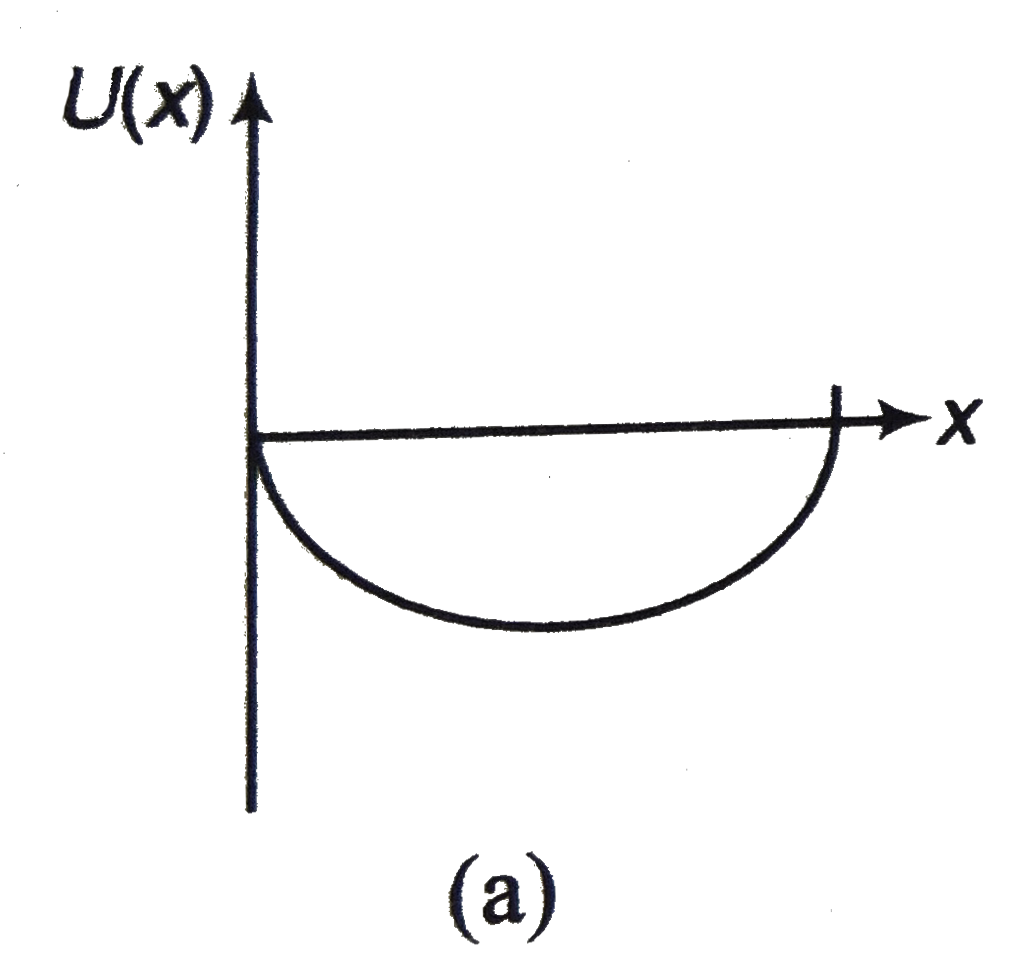 , (b)
, (b) 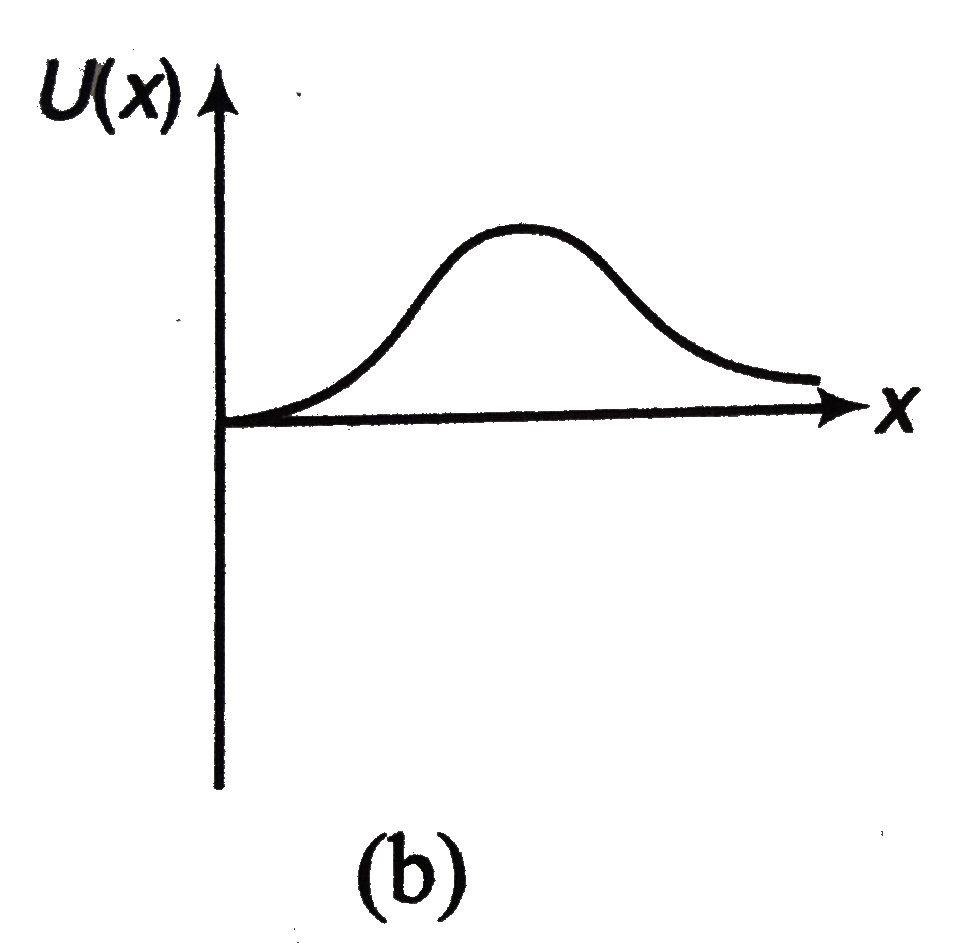 , (c)
, (c) 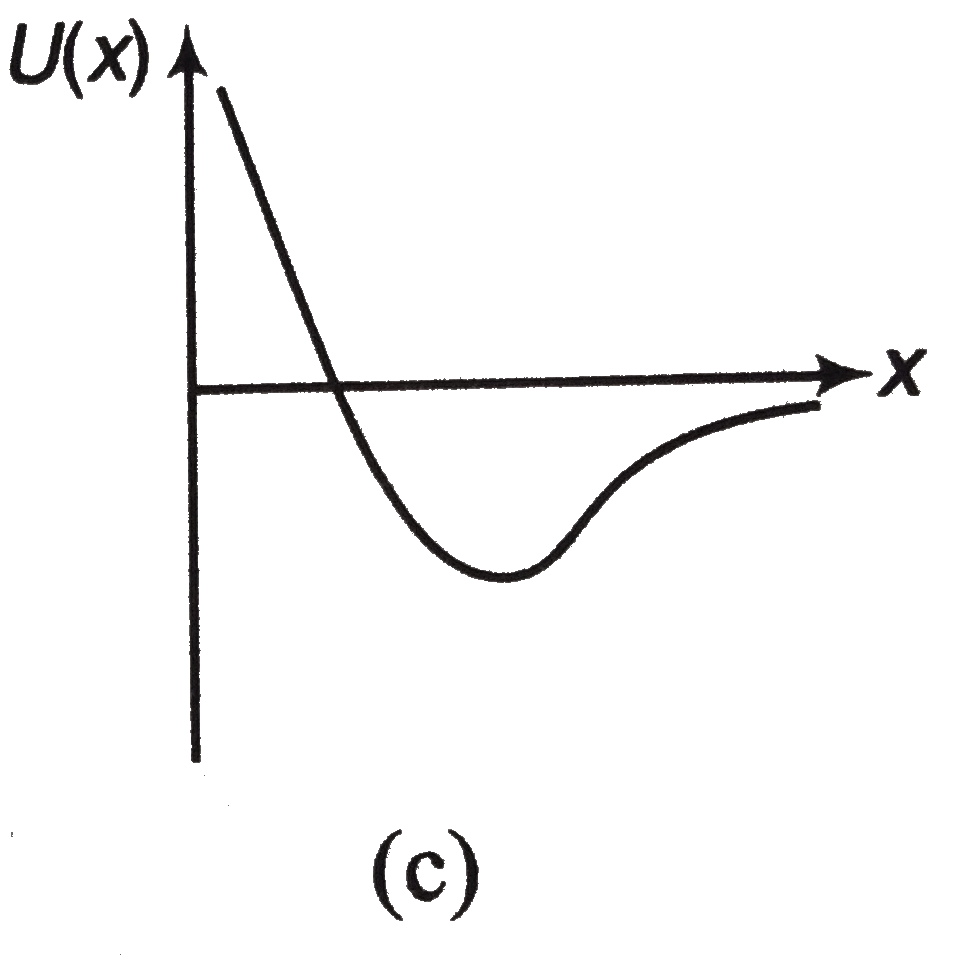 , (d)
, (d) 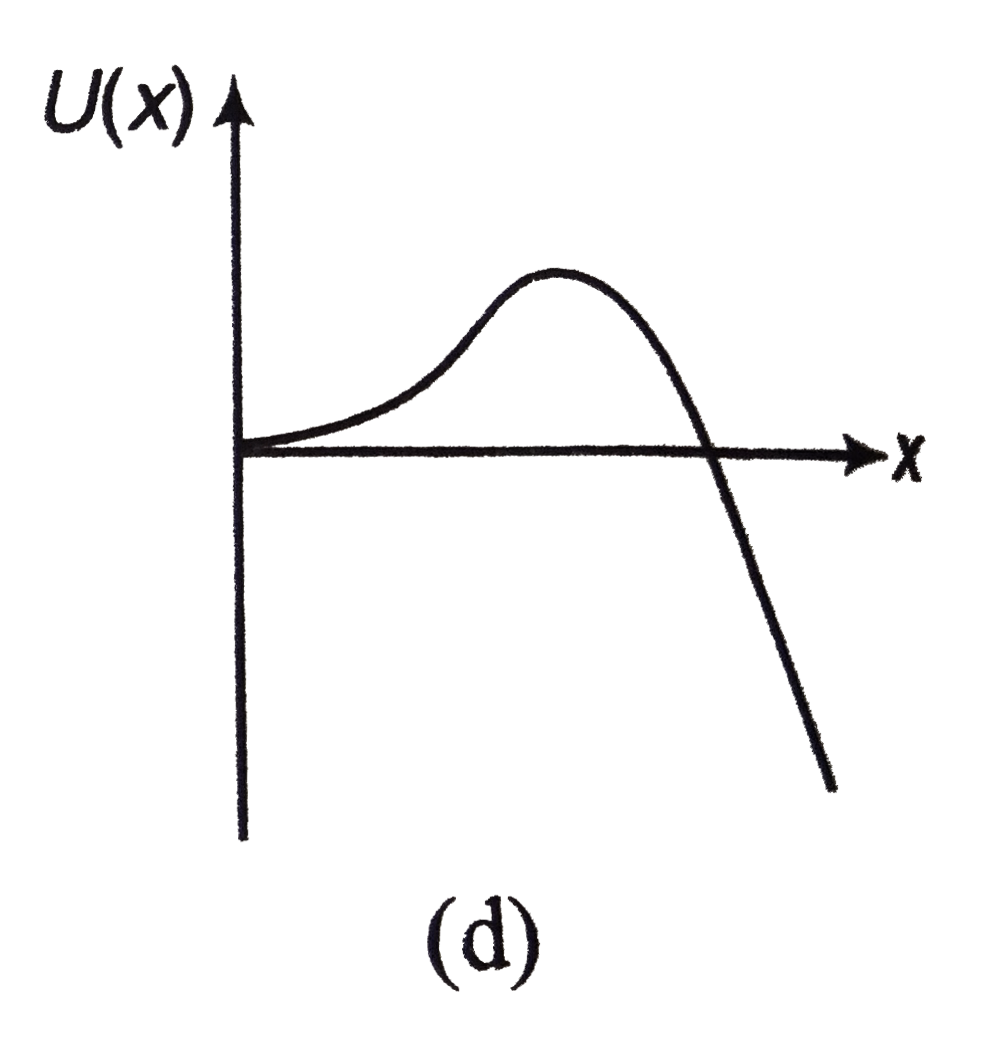 .
.
View SolutionA particle is placed at the origin and a force F=Kx is acting on it (where k is a positive constant). If `U_((0))=0`, the graph of `U (x)` verses x will be (where U is the potential energy function.)
View Solution
Solved Examples questions and complete solutions for chapter WORK, ENERGY & POWER of DC PANDEY ENGLISH of Class 11