Complete Solutions to Level 2 Objective of chapter WORK, ENERGY & POWER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WORK, ENERGY & POWER |
| DC PANDEY | Level 2 Objective |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
A bead of mass `1/2 kg` starts from rest from A to move in a vertical place along a smooth fixed quarter ring of radius `5 m`, under the action of a constant horizontal force `f=5 N` as shown. The speed of bead as it reaches the point (B) is [Take `g=10 ms^(-2)`]
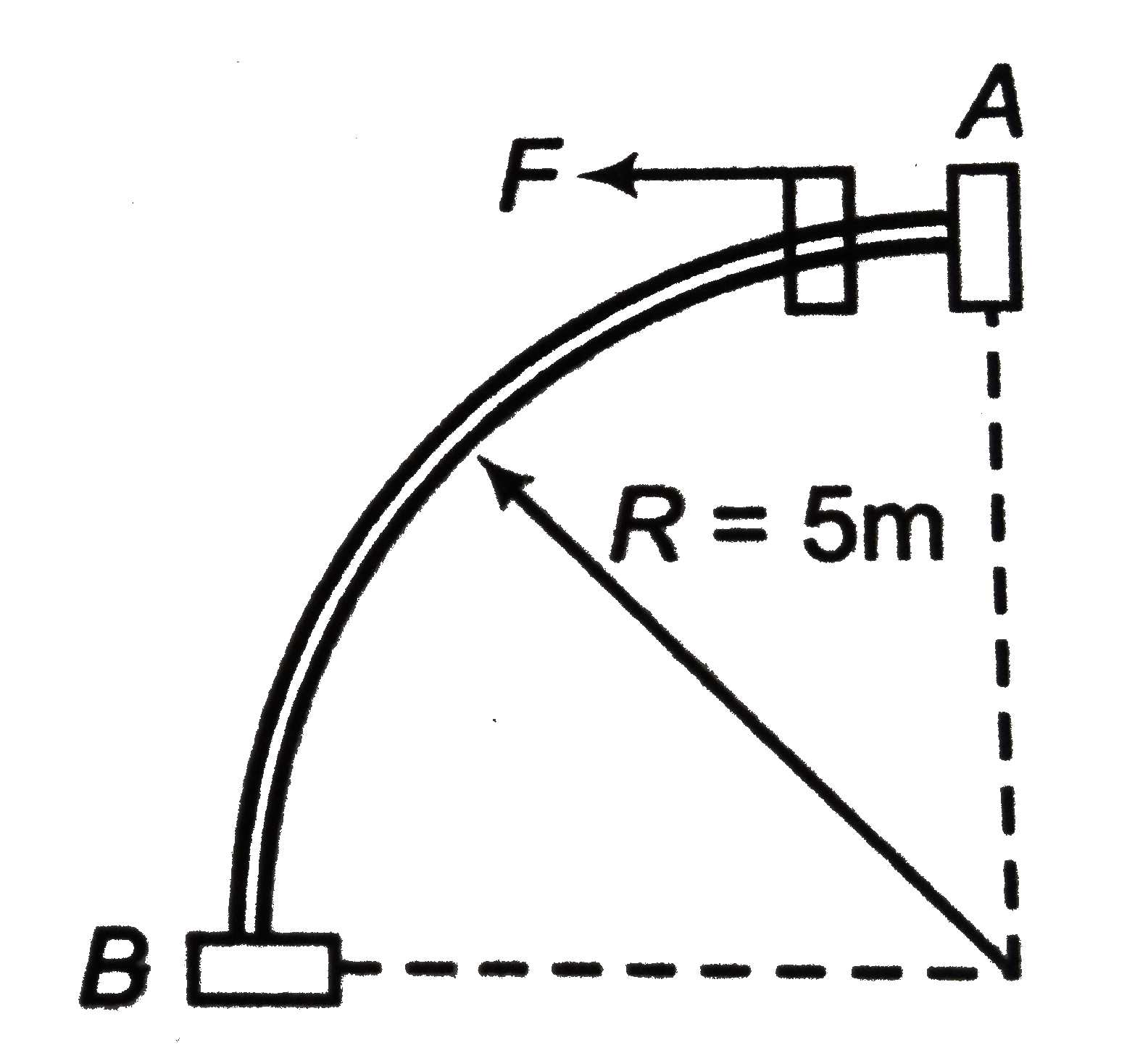
View SolutionA car of mass m is accelerating on a level smooth road under the action of a single F. The power delivered to the car is constant and equal to P. If the velocity of the car at an instant is v, then after travelling how much distance it becomes double?
 .
.
View SolutionAn ideal massless spring `S` can compressed `1.0` m in equilibrium by a force of `100 N`. This same spring is placed at the bottom of a friction less inclined plane which makes an angle `theta =30^@` with the horizontal. A (10 kg) mass (m) is released from rest at the top of the incline and and is brought to rest momentarily after compressing the spring by `2 m. if `g =10 ms^(-1)`, what is the speed of just before it touches the spring?
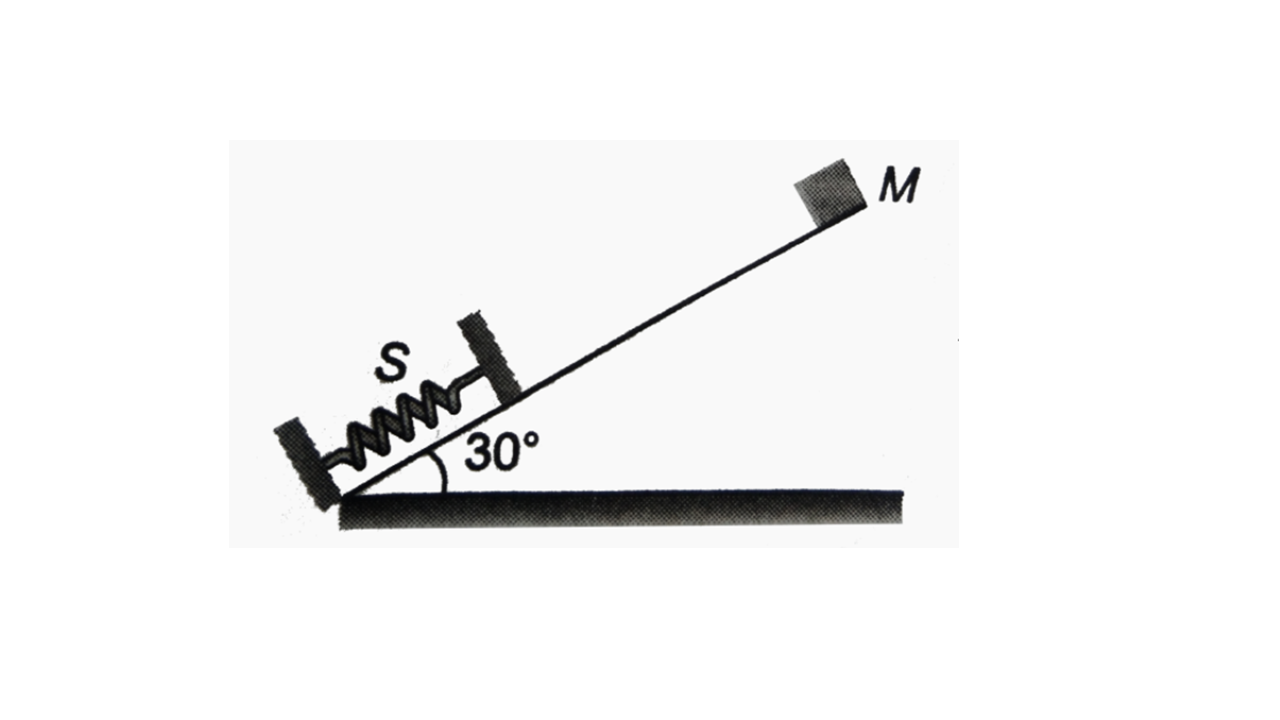 .
.
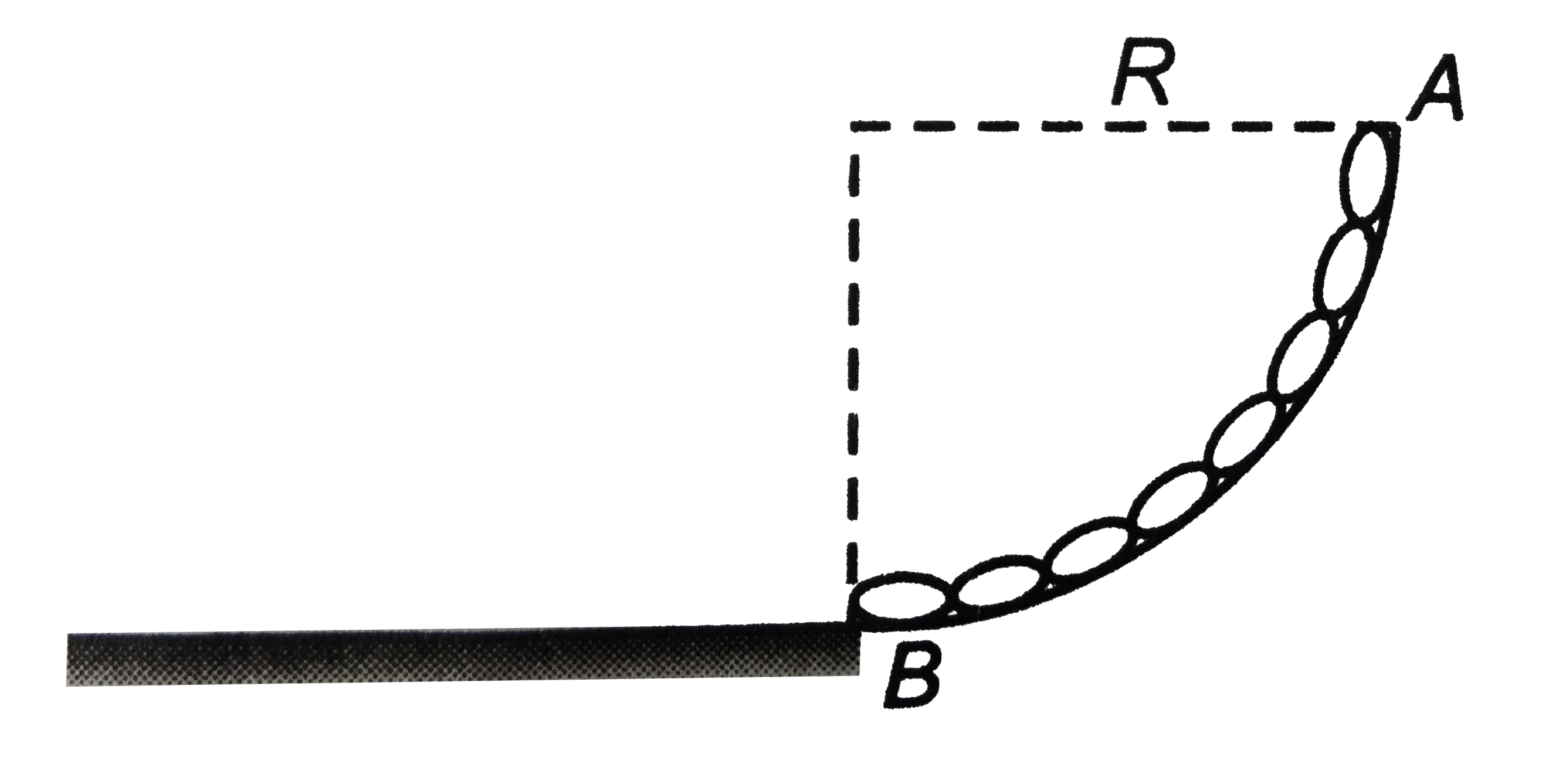
View SolutionA smooth chain (AB) of mass (m) rests against a surface in the form of a quarter of circle of radius R. If it is released from rest, the form of a quarter of a circle of radius R. If it is releaded from, the velocity of the chain after it comes over the horizontal part of the surface is .
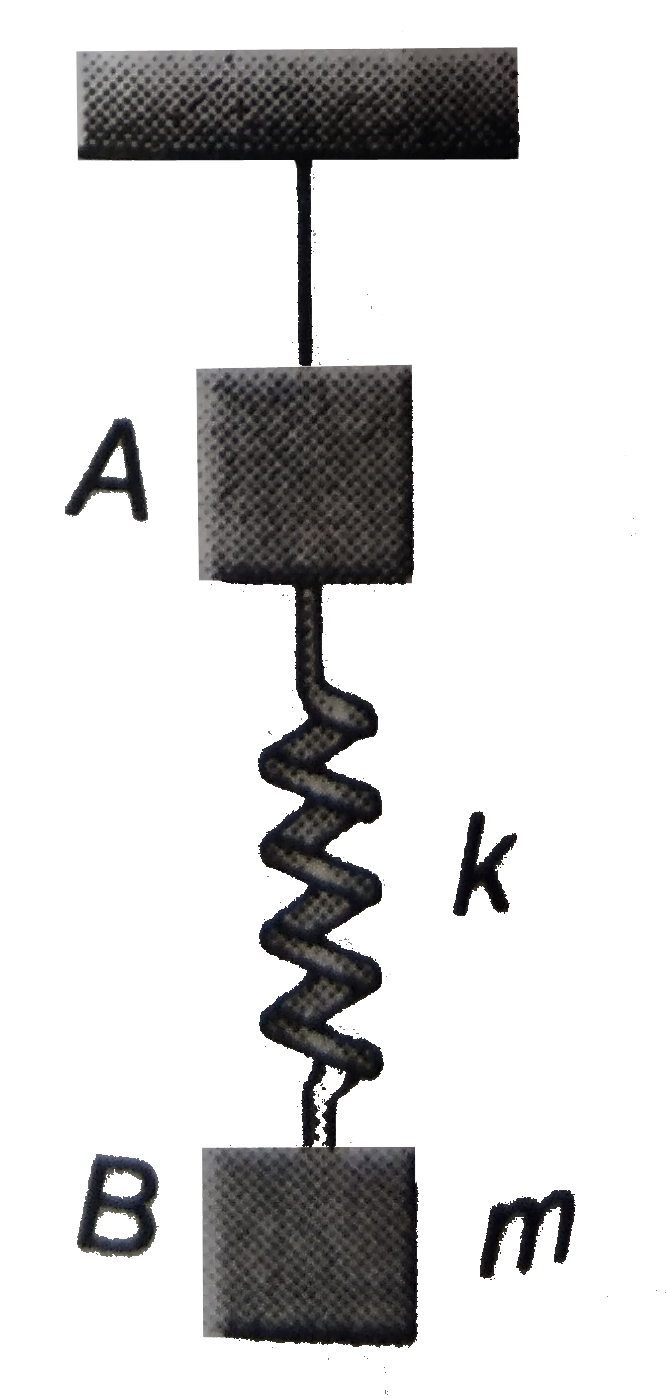
View SolutionInitially the system shown in figure is in equilibrium. At the moment, the string is cut the downward of `a_(1)` and `a_(2)` are.
 .
.
View SolutionIn the diagram shown, the block A and B are of the same mass M and the mass of the block C is `(M_(1))`. Friction is present only under the block A. the whole system is suddenly released from the state of rest. The minimum coefficient of friction on block A to keep it in the state of rest is equal to .

View SolutionSystem shown in figure is in equilibrium, find the magnitude of net change in the string tension between two masses just after, when one of the springs in cut, Mass of both the blocks is same and equal to m spring constant of both springs is k.
 .
.
View SolutionA body is moving is down an inclined plane of slope `37^@` the coefficient of friction between the body and the plane varies as `mu=0.3x`, where x is the distance traveled down the plane by the body. The body will have maximum speed. `(sin 37^@ =(3)/(5))` . a) at x = 1.16 m b) at x = 2m c) at bottom most point of the plane d) at x = 2.5 m
View SolutionThe given plot shows the variation of U, the potential energy of interaction between two particles with the distance separating them r,
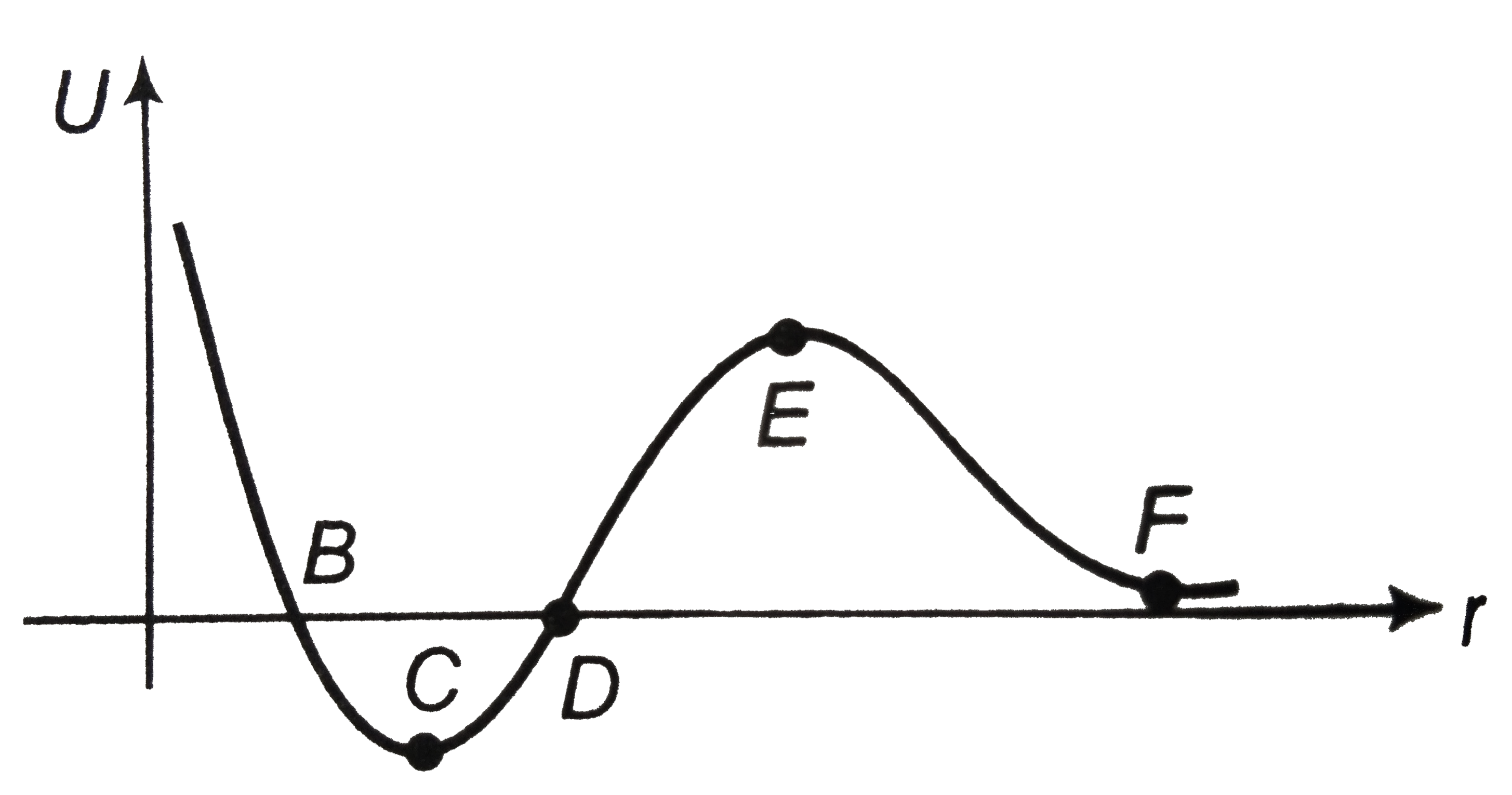
View SolutionA particle is projected at `t=0` from a point on the ground with certain velocity at an angle with the horizontal. The power of gravitation force is plotted against time. Which of the following is the best representation?
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is `mu` then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionA block of mass (m) slides along the track with kinetic friction mu. A man pulls the block through a rope which makes an angle `theta` with the horizontal as shown in the figure. The block moves with constant speed v. Power dlivered by man is
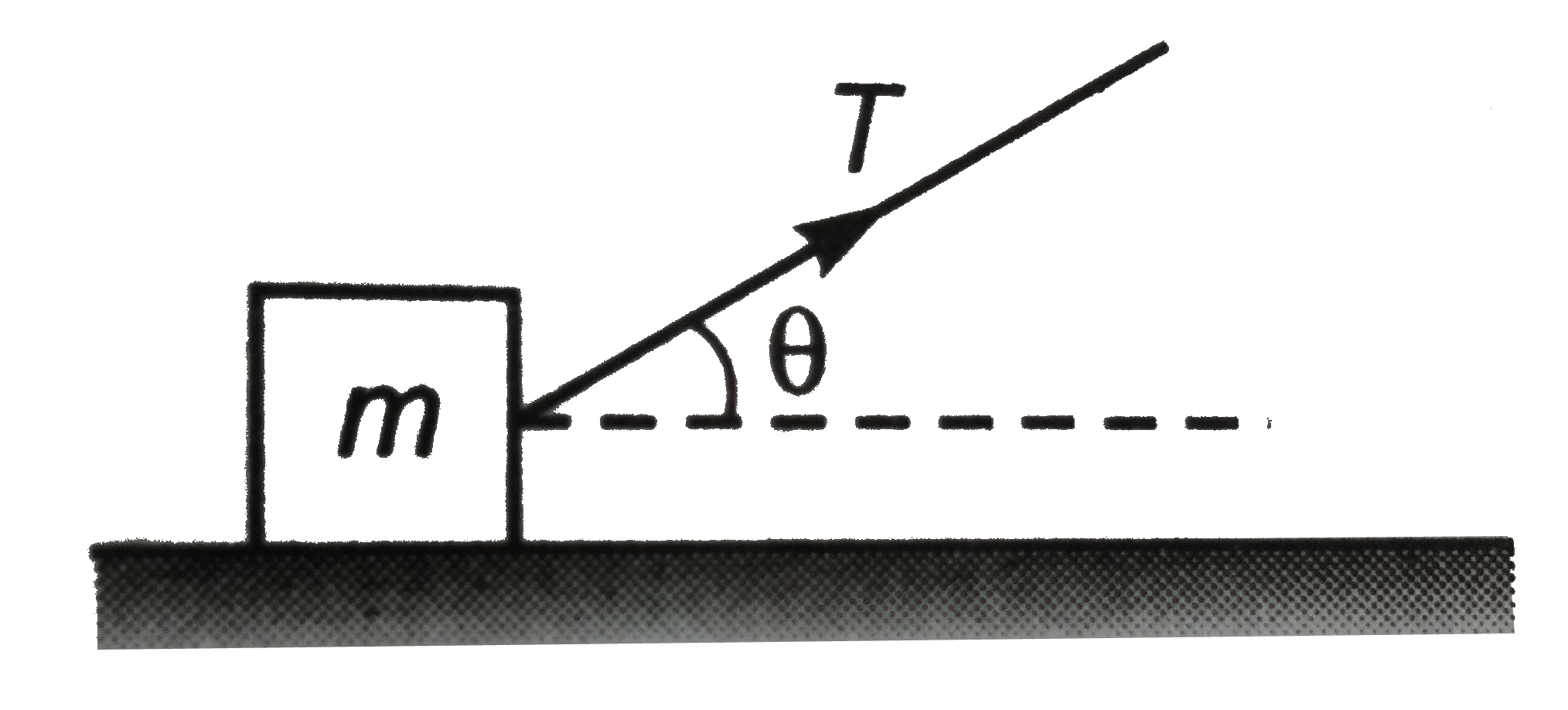 .
.
View SolutionThe potential energy `phi` in joule of a particle of mass `1 kg` moving in x-y plane obeys the law, `phi=3x + 4y`. Here, x and y are in metres. If the particle is at rest at `(6m, 8m)` at time 0, then the work done by conservative force on the particle from the initial position to the instant when it crosses the x-axis is . a) 25 J b) 25 J c) 50 J d) - 50 J
View SolutionThe force acting on a body moving along x-axis variation of the particle particle shown in the figure. The body is in stable equilibrium at
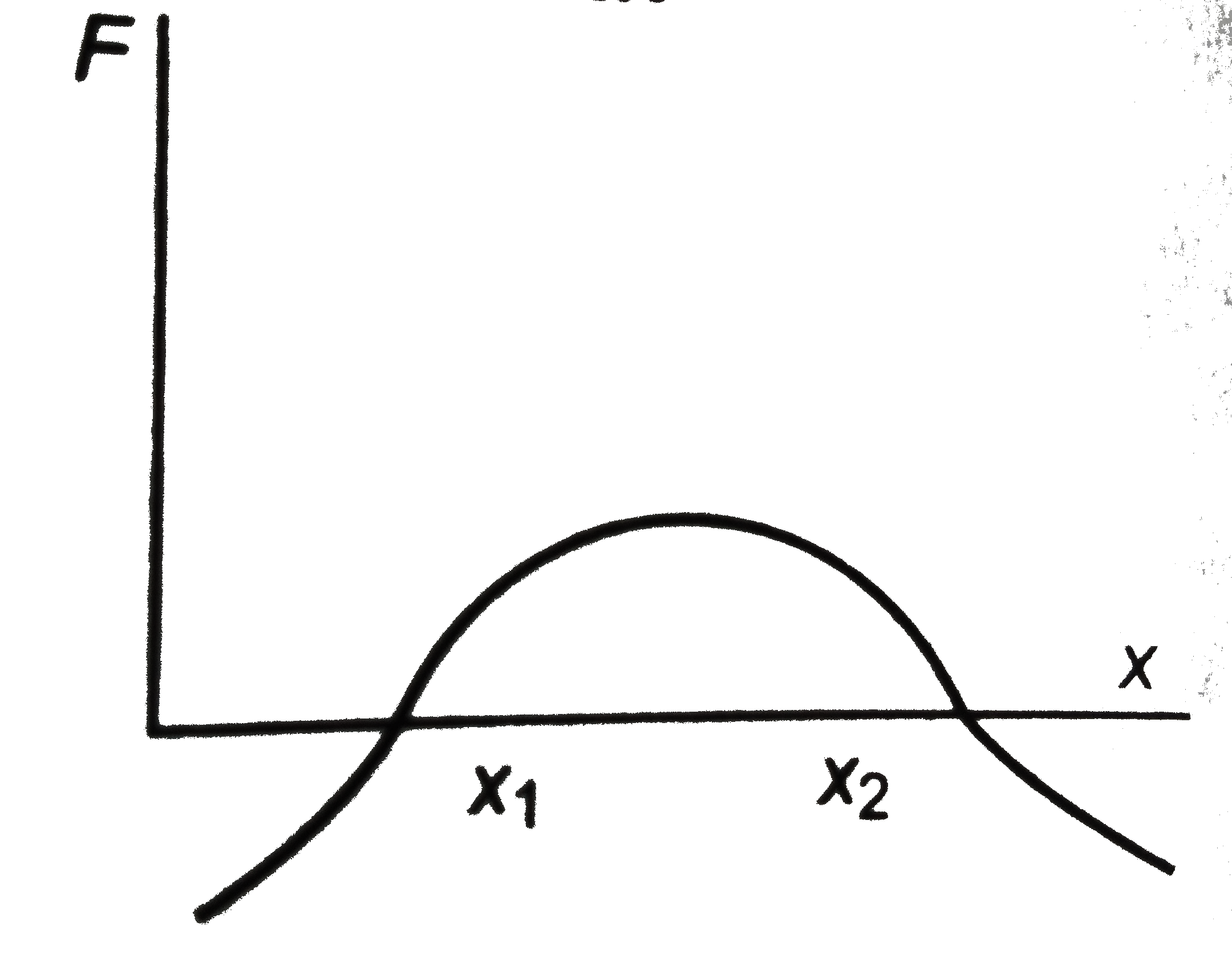 .
.
View SolutionA small mass slides down an inclined plane of inclination `theta` with the horizontal the coefficient of friction is `mu = mu_(0)x`, where x is the distance through which the mass slides down. Then the distance covered by the mass before it stops is
View SolutionTwo light vertical springs with equal natural length and spring constants `k_(1)` and `k_(2)` are separated by a distance `l`. Their upper ends are rigidly connected and the lower ends are connected to `A` and `B` of a light horizontal rod `AB`. A vertically downwards force `F` is applied at point `C` on the rod. `AB` will remain horizontal in equilibrium if the distance `AC` is
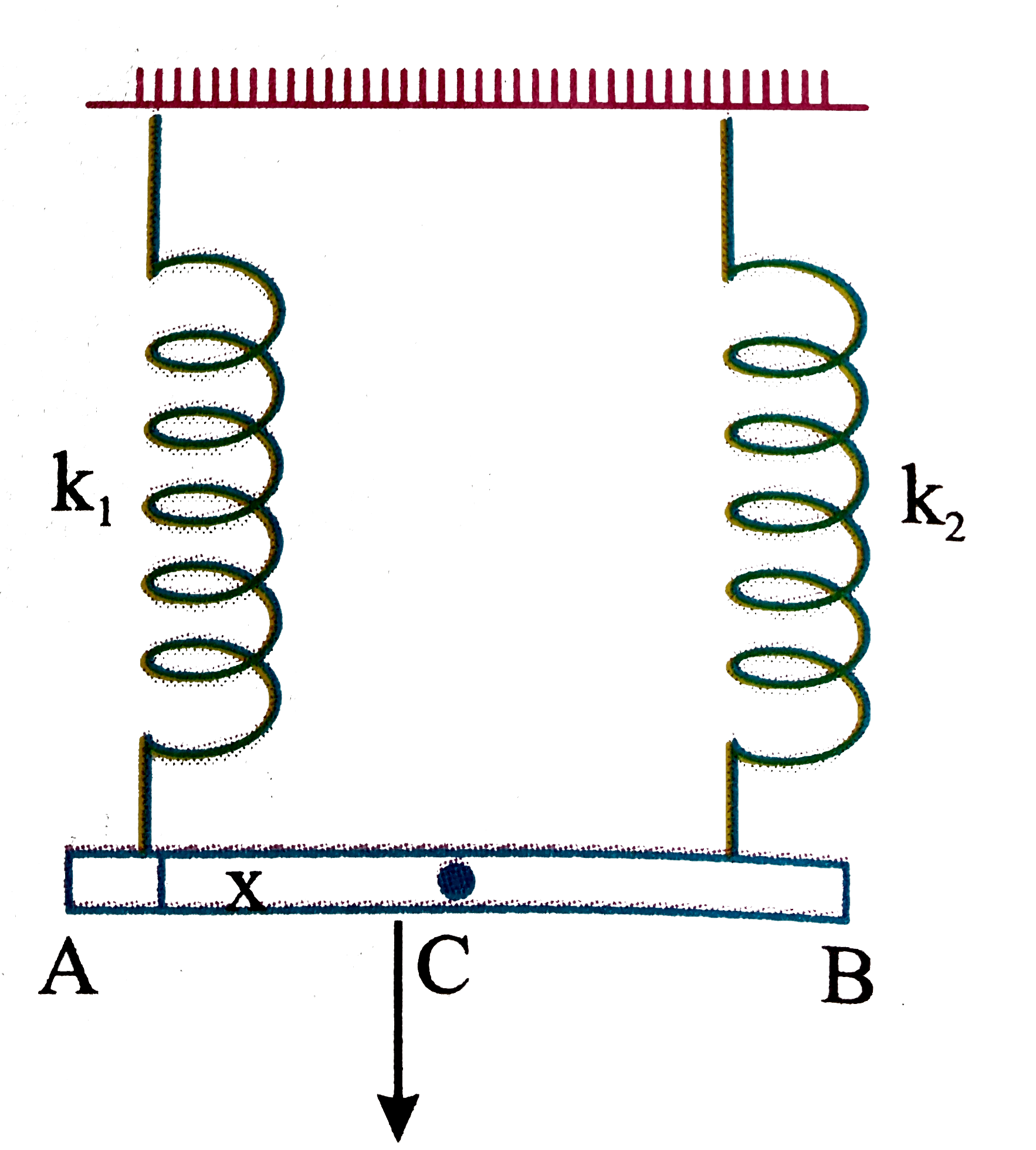
View SolutionA block of mass `1kg` slides down a curved track which forms one quadrant of a circle of radius `1m` as shown in figure. The speed of block at the bottom of track is `v=2 ms^(-1)`. The work done by the of friction is
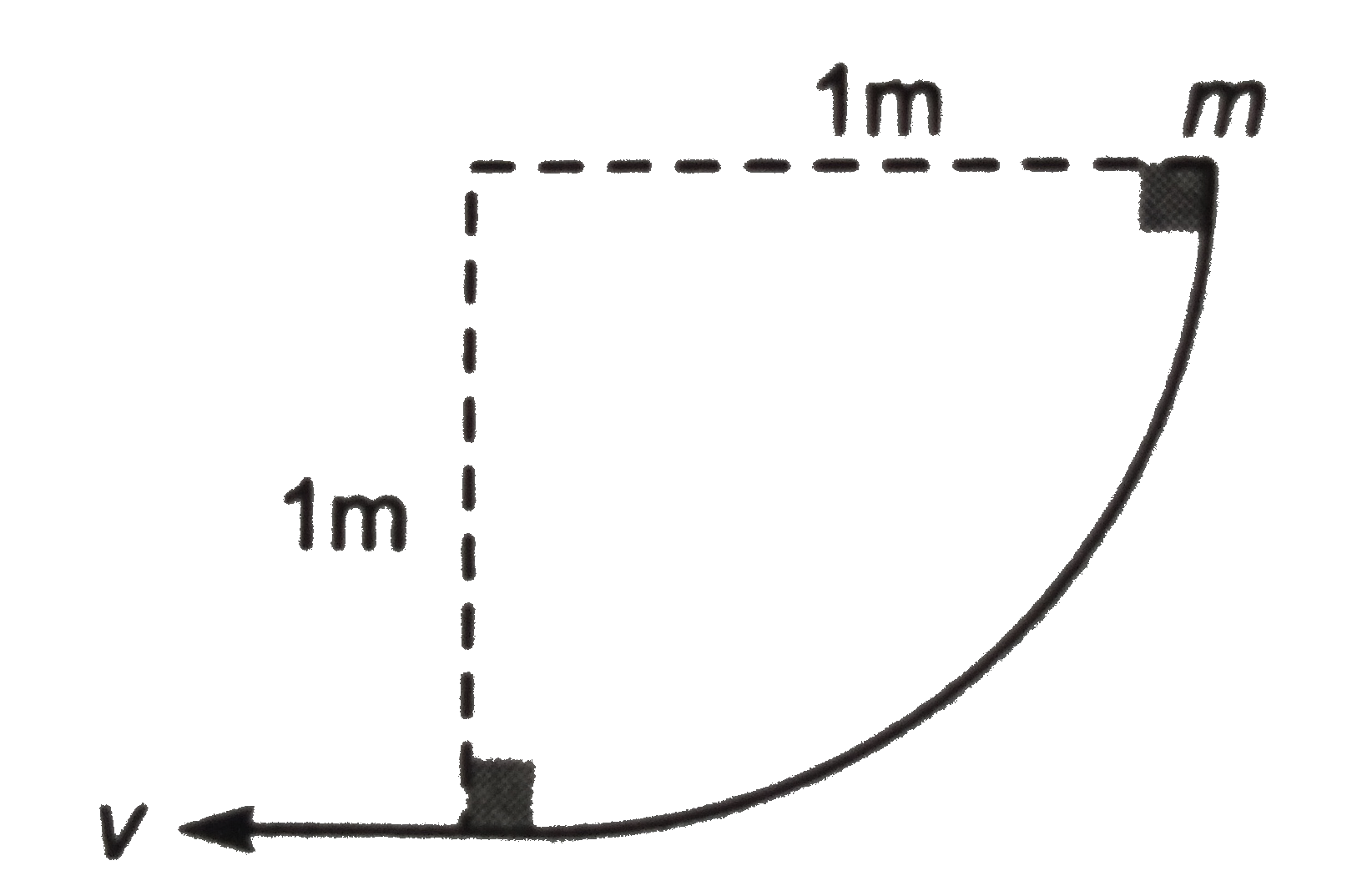 .
.
View SolutionThe potential energy function for a diatomic molecule is `U(x) =(a)/(x^(12)) - (b)/(x^(6))`. In stable equilibrium, the distance between the particles is .
View SolutionA rod mass (M) hinged at (O) is kept in equilibrium with a spring of stiffness (k) as shown in figure. The potential energy stored in the spring is .

View SolutionIn the figure. `(m_2) (< m_(1))` are joined together by a pulley. When the mass `(m_(1))` is released from the height `h` above the floor, it strikes the floor with a speed .

View SolutionA particle free to move along x-axis is acted upon by a force `F=-ax+bx^(2) whrte a and b are positive constants. `, the correct variation of potential energy function U(x) is best represented by.
View SolutionEqual net forces act on two different block (A) and (B) masses (m) and 4(m) respectively For same displacement, identify the correct statement.
View SolutionThe potential energy function of a particle in the x-y plane is given by `U =k(x+y)`, where (k) is a constant. The work done by the conservative force in moving a particlae from (1,1) to (2,3) is .
View SolutionA vertical spring is fixed to one of its end and a massless plank pland fitted to other end, A block is released from a height (h) as shown, spring is in relaxed position then choose the correct statement.
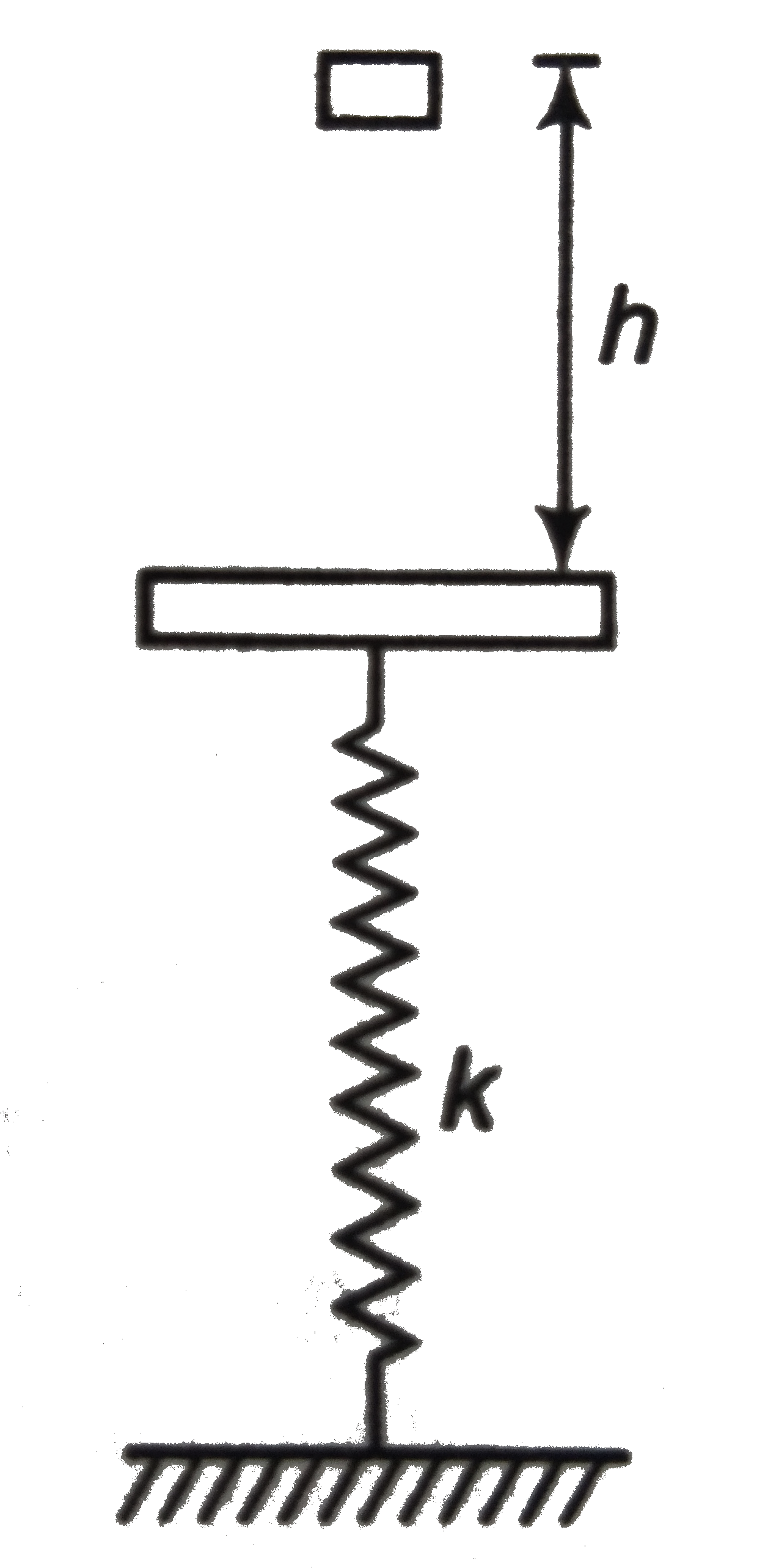 .
.
View SolutionA uniform chain of length of length `pir` lies inside a smooth semicircular tube (AB) of radius f. Assuming a slight disturbance to start the chain in motion, the velocity it will emerge from the end (B) of the tube will be

View SolutionA block of mass m is connected to a spring of force constant k. Initially the block is at rest and the spring has natural length. A constant force F is applied hrizontally towards right. The maximum speed of the block will be (there is no friction between block and the surface)

View SolutionTwo blocks are connected to an ideal spring of stiffness `200 N//m`. At a certain moment, the two block are moving in opposite diretion with speeds `4 ms_(1)` and ' 6 ms_(1) ' the instantaneous elongation of the spring `10 cm`, The rate at which the spring energy `((kx^(2)/(2))` is increasing is: a) 500 J/s b) 400 J/s c) 200 J/s d) 100 J/s
View SolutionA block (A) of mass `45kg` is placed on another block (B) of mass `123 kg`. Now block (B) is displaced by external agent by `50 cm` horizontally towards right. During the same time block (A) just reaches to the left end of block (B), Initial and final positions are shown in figures . the work done on block (A) in ground frame is
 ,
,
View SolutionA block of mass `10 kg` is released on a fixed wedge inside a cart which is moving with constant velocity `10 ms^(1)` towards right. There is no relative motion between block and cart. Then work done by mormal reaction on block in two seconds from ground frame will be (g=10 ms^(1))
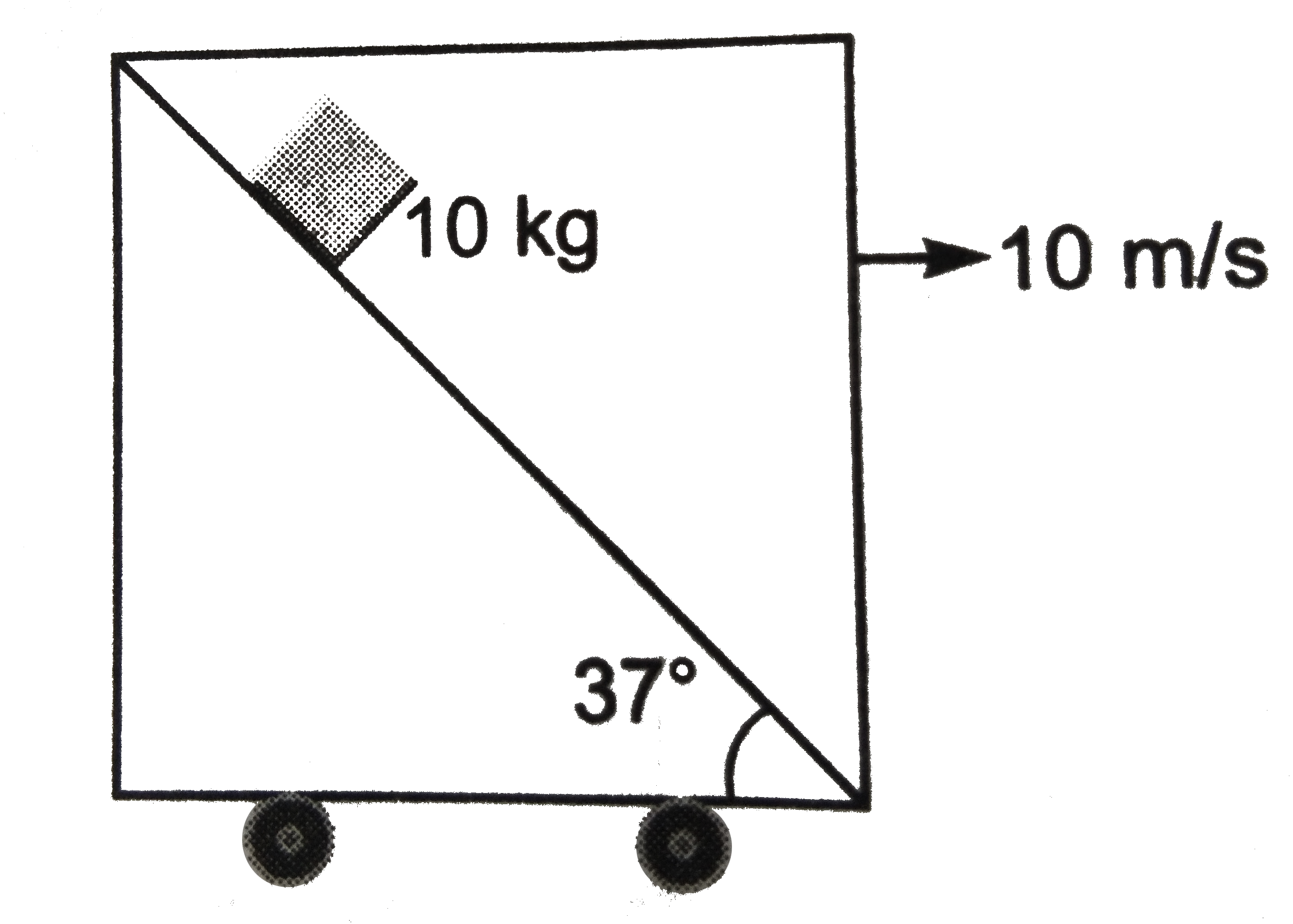
View SolutionA block tied between identical springs is in equilibrium. If upper spring is cut, then the acceleration of the block just after cut is `5 ms^(1)` Now if instead of upper string lower spring is cut, then the acceleration of the block just after the cut will be (Take `g=10 m//s^(2)`).
View Solution
Level 2 Objective questions and complete solutions for chapter WORK, ENERGY & POWER of DC PANDEY ENGLISH of Class 11