Complete Solutions to Subjective of chapter FLUID MECHANICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | FLUID MECHANICS |
| DC PANDEY | Subjective |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
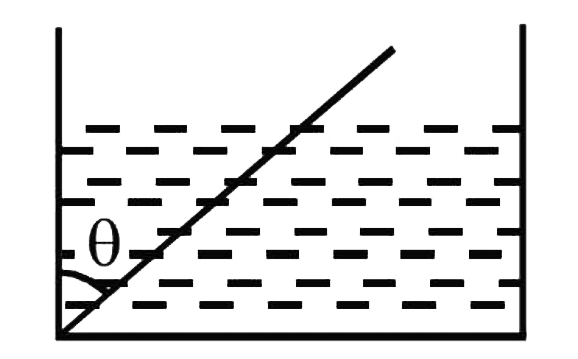
A wooden plank of length 1m and uniform cross-section is hinged at one end to the bottom of a tank as shown in fig. The tank is filled with water upto a hight 0.5m. The specific gravity of the plank is 0.5. Find the angle `theta` that the plank makes with the vertical in the equilibrium position. (Exclude the case `theta=theta^@`)
View SolutionA cubical block of wood of edge 3 cm floats in water. The lower surface of the cube just touches the free end of a vertical spring fixed at the bottom of the pot. Find the maximum weight that can be put on the block without wetting it. Density of wood =`800 kgm^-3` and spring constant of the spring `=50Nm^-1 Take g=10ms^-2`.
 ,
,
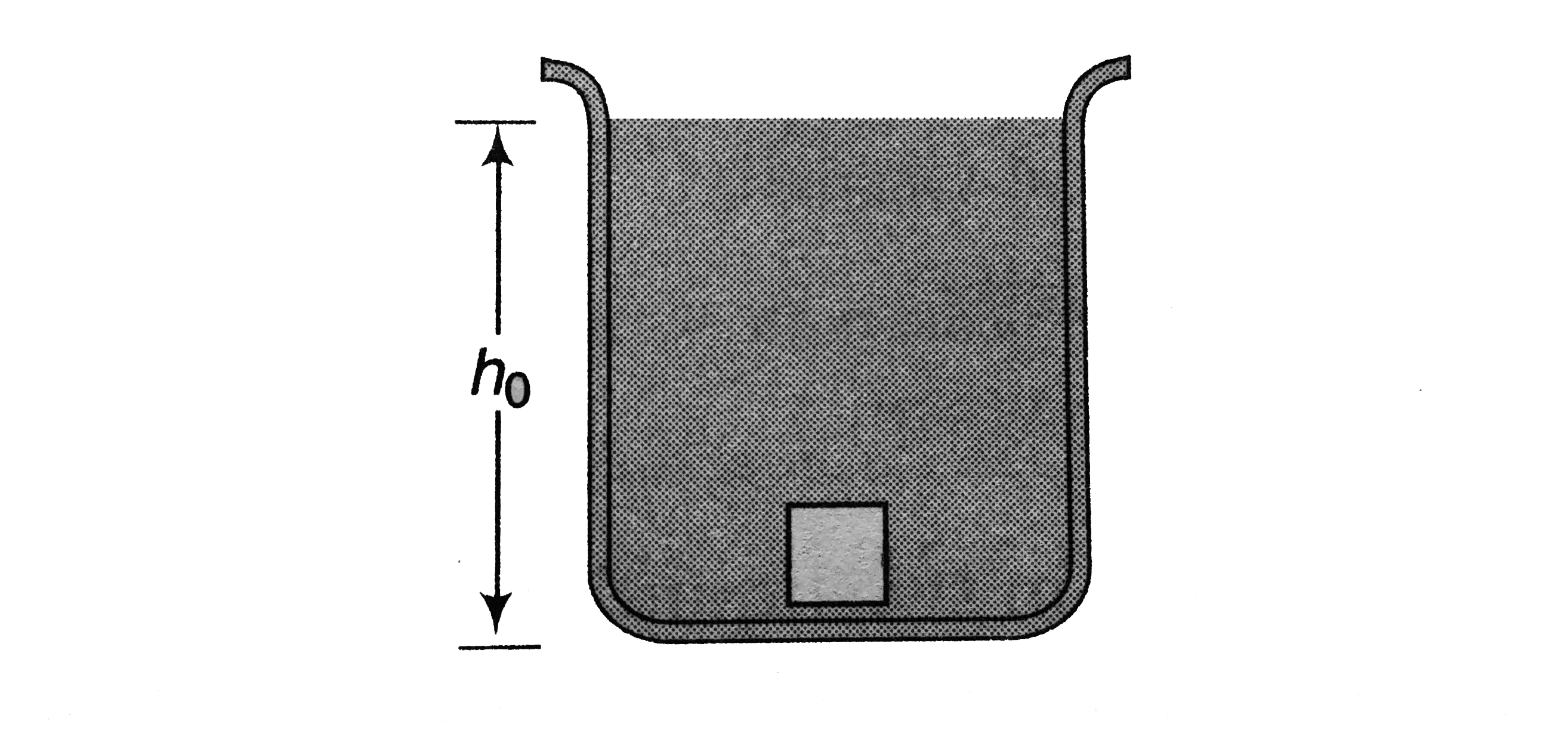
View SolutionFigure shown a container having liquid of variable density. The density of liquid veriesas `rho=rho_(0)(4-(3h)/(h_(0)))`. Here, `h_(0)` and `rho_(0)` are constants and h is measured from bottom of the container. A solid block of small dimensions whose density is `(5)/(2) rho_(0)` and mass m is released from bottom of the tank. Prove that the block will execute simple harmonic motion. Find the frequency of oscillation.
 .
.
View Solution_S01_030_Q01.png)
A cylinderical tank 1 m in radius rests on a plaform 5 m high. Initially the tank is filled with water upto a height of 5m a plug whose area is `10^(-4)cm^(2)` is removed from an orifice on the side of the tank at the bottom.
Calculate (a). Initial speed with which the water flows from the orifice
(b). Initial speed with which the water strikes the ground.
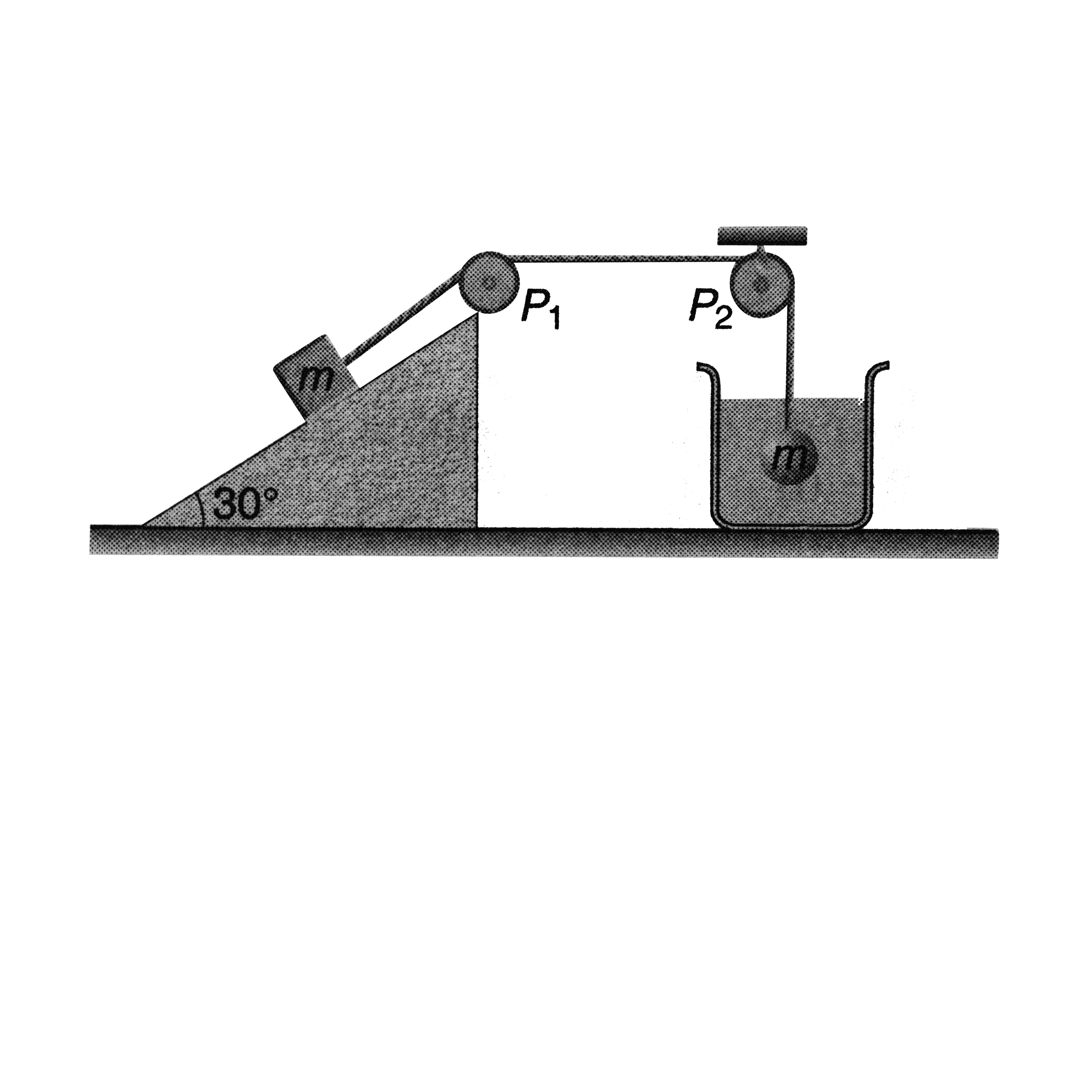
View SolutionA block of mass m is kept over a fixed smooth wedge. Block is attached to a sphere of same mass through fixed massles pullies `P_(1)` and `P_(2)`, sphere is dipped inside the water as shown. If specfic gravity of material of sphere is 2. Find the acceleration of sphere.
View SolutionA cubic body floats on mercury with 0.25 fraction of its volume below the surface. What fraction of the volume of the body will be immeresed in the mercury if a layer of water poured on top of the mercury covers the body conpletely.
View SolutionA siphon tube is discharging a liquid of specific gravity 0.9 from a reservoir as shown in the figure.
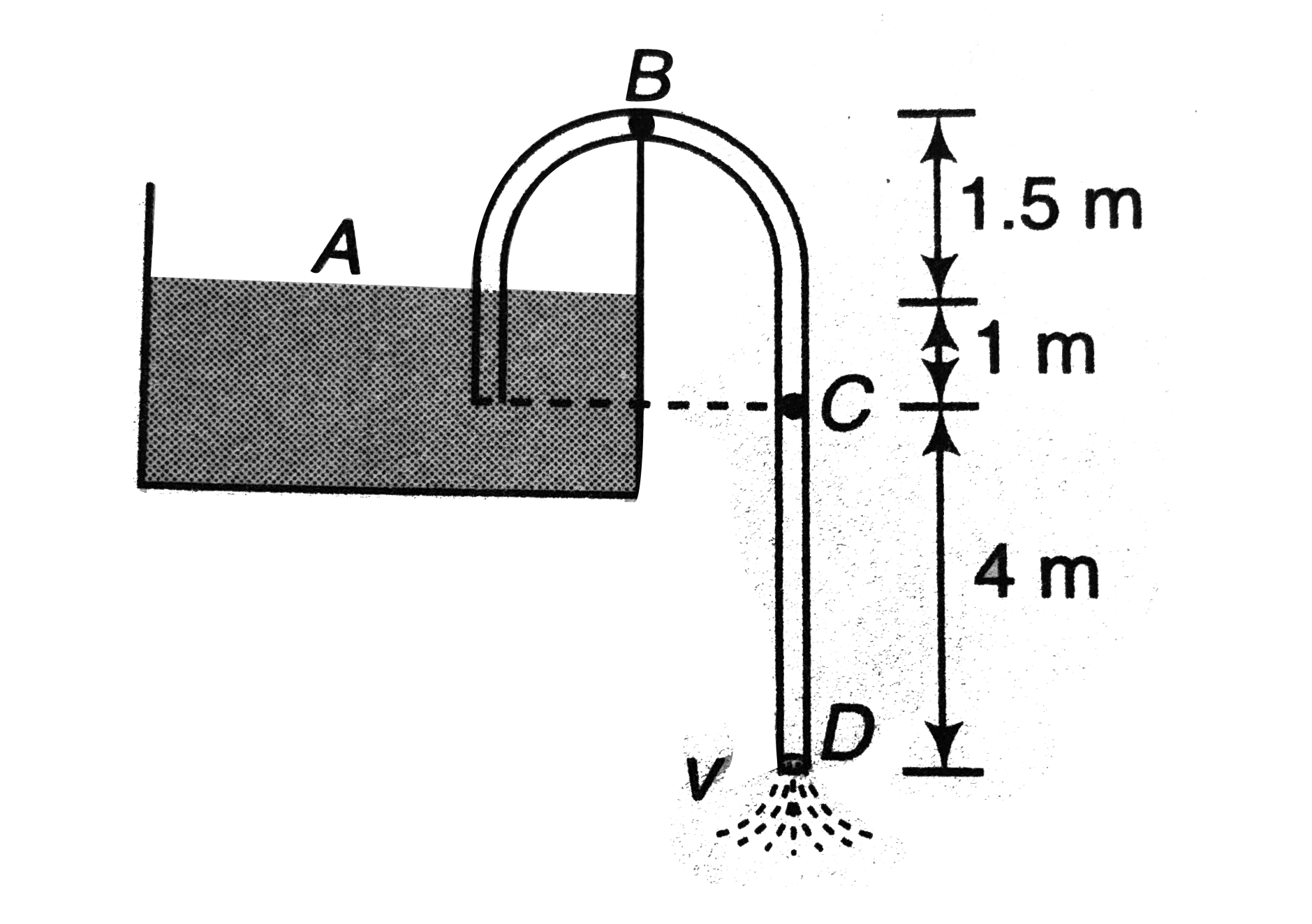
(a) Find the velocity of the liquid through the siphon.
(b) Find the pressure at the highest point B.
(c ) Find the pressure at point C.
View SolutionA long cylindrical tank of cross-sectional area `0.5m^(2)` is filled with water. It has a small hole at a height `50cm` from the bottom. A movable piston of cross-sectional area almost equal to `0.5m^(2)` is fitted on the top of the tank such that it can slide in the tank freely. A load of `20 kg` is applied on the top of the water by piston, as shown in the figure. Calculate the speed of the water jet with which it hits the surface when piston is 1m above the bottom. (Ignore the mass of the piston)

View SolutionA spring is attached to the bottom of an swimming pool, with the axis of the spring oriented vertically. An 8.00 kg block of wood `(rho=840 kh//m^(3))` is fixed to the top of the spring and compressed it. Then the pool is filled with water, completely covering the block. the spring is now observed to be stretched twice as much as it had been conpressed. Determine the percentage of the block's total volume that is hollow. Ignore any air in the hollow space.
View SolutionA rectangular tank of height 10m filled with water, is placed near the bottom of a plane inclined at an angle `30^(@)` with horizontal. At height h form bottom a small hole is made (as shown in figure) such that the stream coming out from hole, strikes the inclined plane normally. Calculate h.
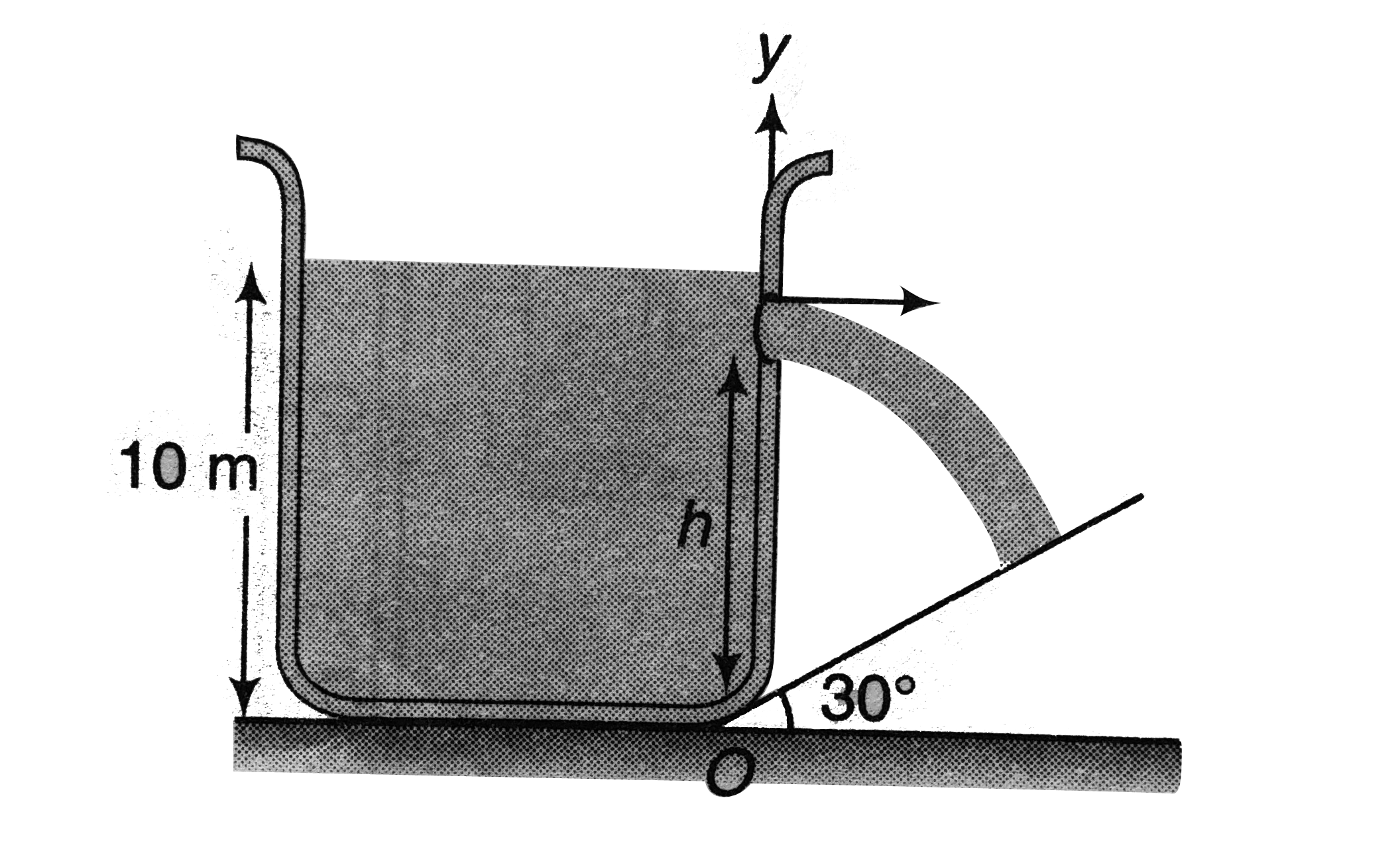
View SolutionA ball of density d is dropped on to a horizontal solid surface. It bounces elastically from the surface and returns to its original position in a time `t_1`. Next, the ball is released and it falls through the same height before striking the surface of a liquid of density of `d_L`
(a) If `dltd_L`, obtain an expression (in terms of d, `t_1` and `d_L`) for the time `t_2` the ball takes to come back to the position from which it was released.
(b) Is the motion of the ball simple harmonic?
(c) If `d=d_L`, how does the speed of the ball depend on its depth inside the liquid? Neglect all frictional and other dissipative forces. Assume the depth of the liquid to be large.
View SolutionThere is an air bubble of radius 1.0mm in a liquid of surface tension 0.075Nm−1 and density 103kgm−3. The bubble is at a depth of 10.0cm below the free surface of a liquid. By what amount is the pressure inside the bubble greater than the atmospheric pressure?
View SolutionA metal sphere of radius 1 mm and mass 50 mg falls vertically in glycerine. Find (a) the viscous force exerted by the glycerine on the sphere when the speed of the sphere is 1 cm s-1, (b) the hydrostatic force exerted by the glycerine on the sphere and (c) the terminal velocity with which the sphere will move down without acceleration. Density of glycerine `=1260kgm^-3` and its coefficient of viscosity at room temperature = 8.0 poise.
View SolutionA wire forming a loop is dipped into soap solution and taken out so that a film of soap solution is formed. A loop of 6.28 cm long thread is gently put on the film and the film is pricked with a needle inside the loop. The thread loop takes the shape of a circle. Find the tension in the thread. Surface tension of soap solution `=0.030Nm^-1`.
View SolutionA cylindrical vessel is filled with water upto a height of 1m. The cross-sectional area of the orifice at the bottom is `(1//400)` that of the vessel.
(a) What is the time required to empty the tank through the orifice at the bottom?
(b) What is the time required for the same amount of water to flow out if the water level in tank is maintained always at a height of 1m from orifice?
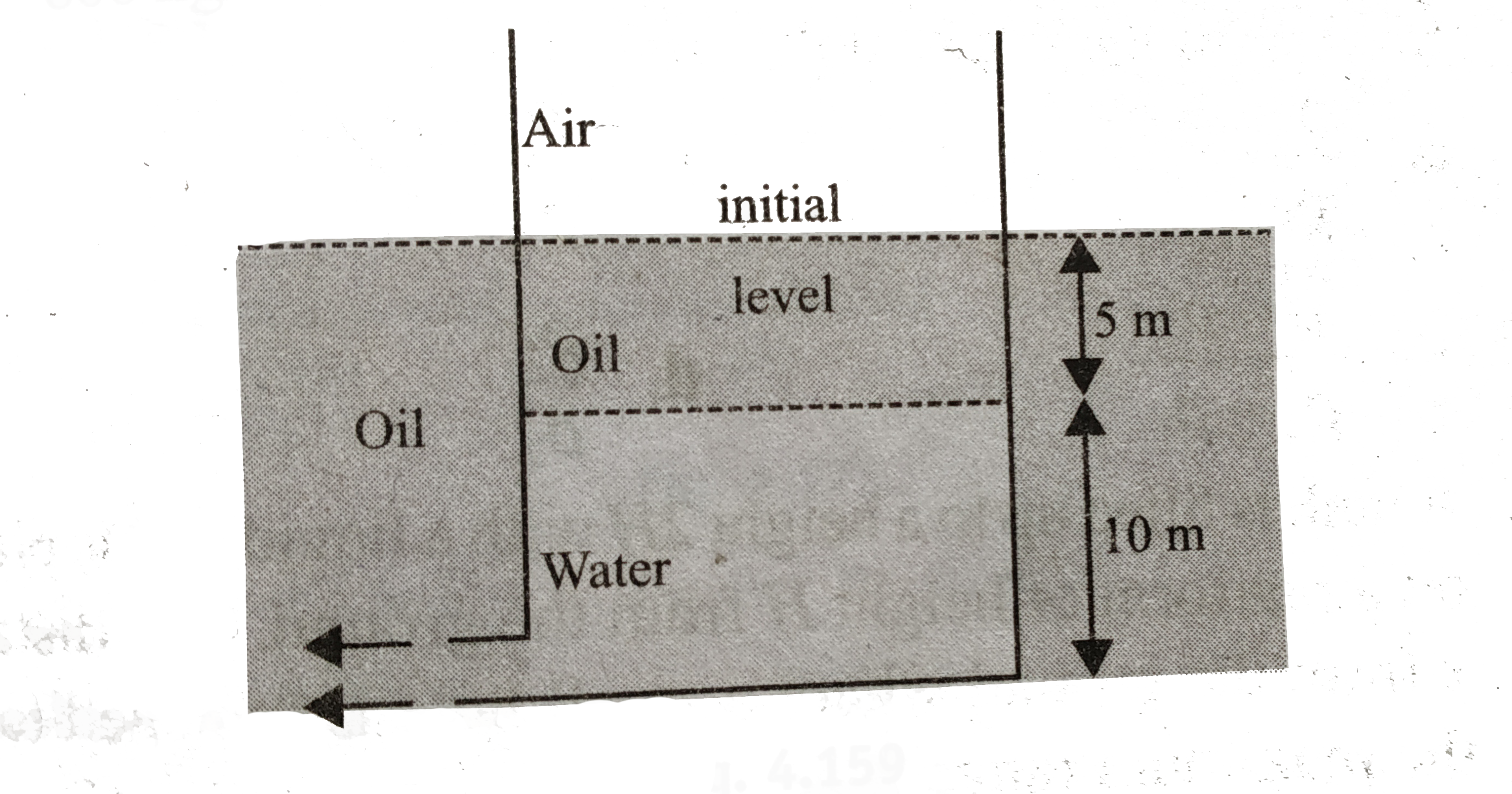
View SolutionA tank with a small orifice contains oil on top of water. It is immersed in a large tank of the same oil. Water flows through the hole. Assuming the level of oil outside the tank above orifice does not change.
a. What is the velocity of this flow initially?
b. When the flow stops, what would be the position of the oil-water interface in the tank?
c. Determine the time at which the flow stops. Density,of oil `800kg//m^(3)`.
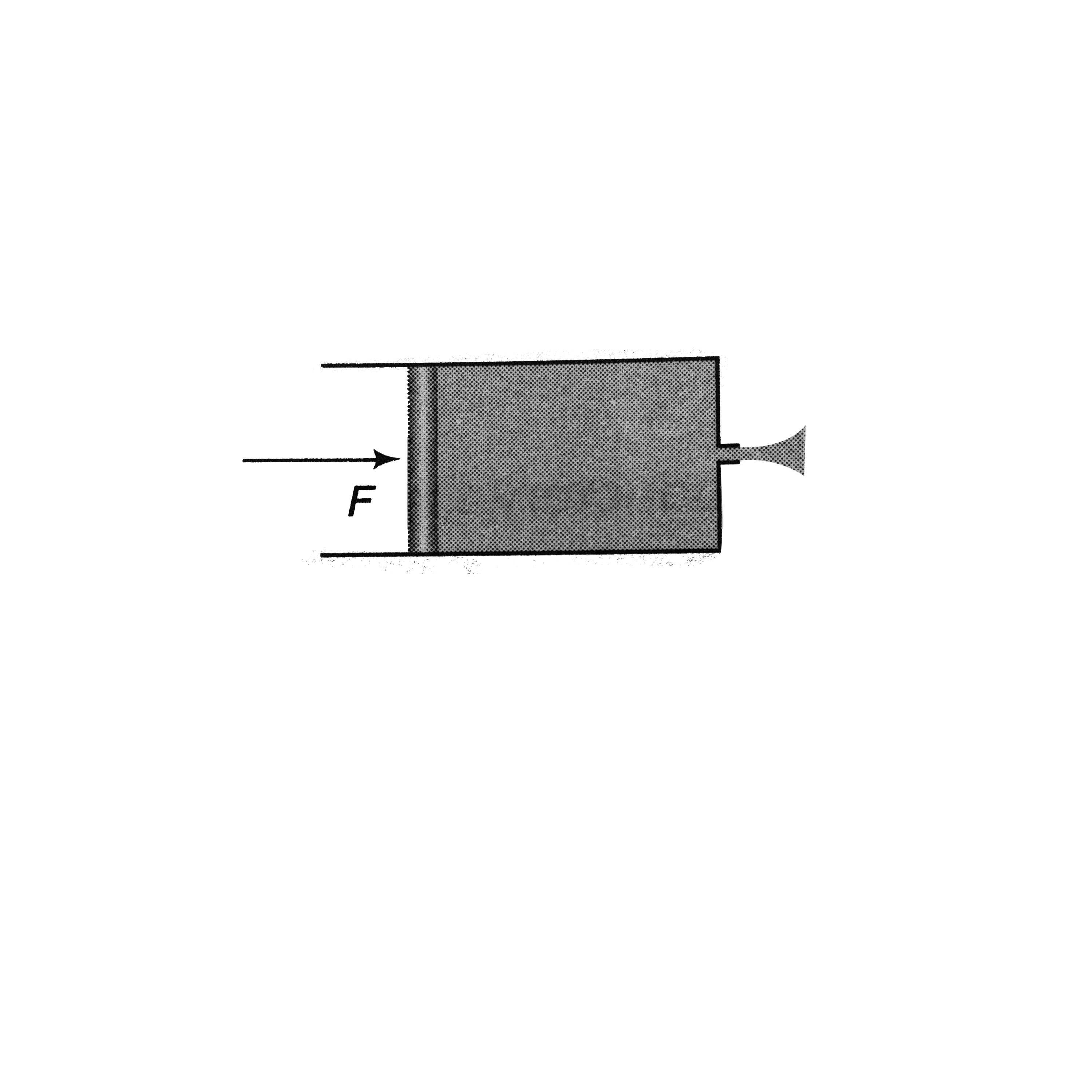
View SolutionWhat work should be done in order to squeeze all water from a horizontally located cylinder (figure) during the time t by means of a constant force acting on the piston? The volume of water in the cylinder is equal to V, the cross-sectional area of the orifice is s, with s being considerably less than the piston area. The friction and viscosity are negligibly small. Density of water is `rho`
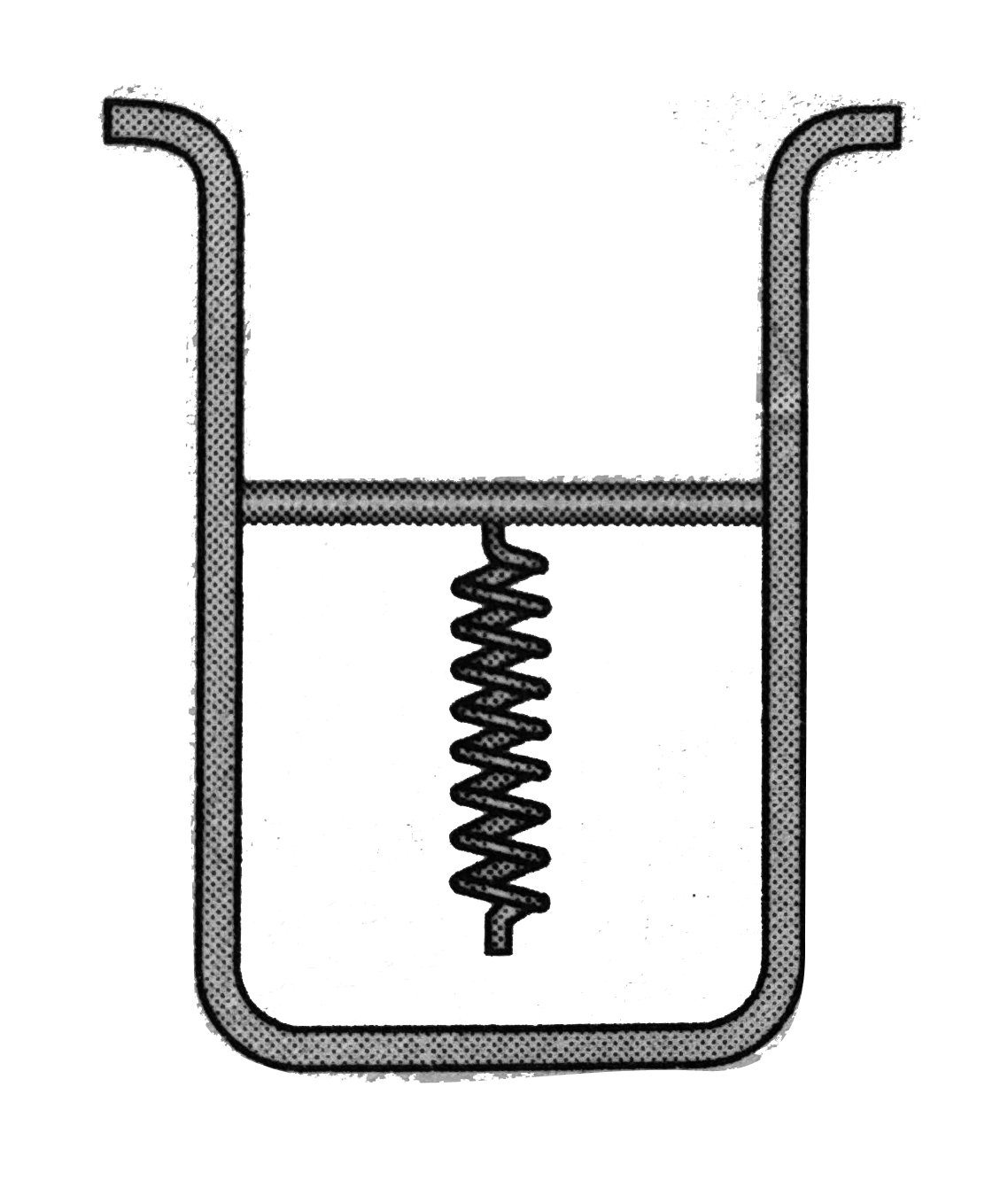
View SolutionA cylinder is fitted with a piston, beneath which is a spring, as in the figure. The cylinder is open at the top. Friction is absent. The spring is `3600 N//m`. The piston has a negligible mass and a radius of 0.025m (a) when air beneath the piston is completely pumped out, how much does the atmospheric pressure cause the spring to compress? (b) How much work does the atmospheric pressure do in compressing the spring?
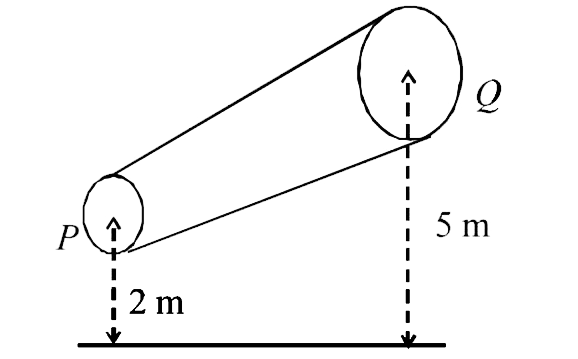
View SolutionA non-viscous liquid of constant density `1000kg//m^3` flows in a streamline motion along a tube of variable cross section. The tube is kept inclined in the vertical plane as shown in Figure. The area of cross section of the tube two point P and Q at heights of 2 metres and 5 metres are respectively `4xx10^-3m^2` and `8xx10^-3m^2`. The velocity of the liquid at point P is `1m//s`. Find the work done per unit volume by the pressure and the gravity forces as the fluid flows from point P to Q.
View SolutionA glass plate of length `10 cm`, breadth `1.54 cm` and thickness `0.20 cm` weigh `8.2 g` in air. It is held vertically with the long side horizontal and the lower half under water. Find the apparent weight of the plate. Surface tension of water `=7.3xx10^(-2)N//m` and `=9.8ms^(-12)`
View SolutionTwo narrow bores of diameters 3.0mm and 6.0 mm are joined together to form a U-shaped tube open at both ends. If th U-tube contains water, what is the difference in its levels in the two limbs of the tube? Surface tension of water at the temperature of the experiment is `7.3xx10^(-2)Nm^(-1)`. Take the angle of contact to be zero. and density of water to be `1.0xx10^(3)kg//m^(3)`.
`(g=9.8 ms^(-2))`
View SolutionTwo identical soap bubbles each of radius r and of the same surface tension `T` combine to form a new soap bubble of radius `R`. The two bubbles contain air at the same temperature. If the atmospheric pressure is `p_(0)` then find the surface tension T of the soap solution in terms of `p_(0)`, r and `R`. Assume process is isothermal.
View SolutionA soap bubble of radius `'r'` and surface tension `'T'` is given a potential of `'V'` volt. If the new radiys `'R'` of the bubble is related to its initial radius by equation.
`P_(0)[R^(3) - r^(3)] + [lambda T [R^(2) - r^(2)] - epsilon_(0) V^(2)R//2 = 0`, where `P_(0)` is the atmospheric pressure. Then find `lambda`
View SolutionA soap bubble of radius r and surface tension 'T' is given a potential of `V` volt. Show that the new radius 'R' of the bubble is related to its intial radius by eqation,
`p_(0)[R^(3)-r^(3)]+4T[R^(2)-r^(2)]0-epsilon_(0)V^(2)R//2=0`
where , `p_(0)` is the atmosphere pressure.
View Solution
Subjective questions and complete solutions for chapter FLUID MECHANICS of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY Class 11 PHYSICS FLUID MECHANICS
- Assertion and Reason
- B) Medical entrance special format question
- Check point 13.1
- Check point 13.2
- Check point 13.3
- Check point 13.4
- Example
- Example 13.1
- Example 13.10
- Example 13.11
- Example 13.12
- Example 13.13
- Example 13.14
- Example 13.15
- Example 13.16
- Example 13.17
- Example 13.18
- Example 13.19
- Example 13.2
- Example 13.20
- Example 13.21
- Example 13.22
- Example 13.23
- Example 13.24
- Example 13.25
- Example 13.26
- Example 13.27
- Example 13.28
- Example 13.29
- Example 13.3
- Example 13.30
- Example 13.31
- Example 13.32
- Example 13.33
- Example 13.34
- Example 13.35
- Example 13.4
- Example 13.5
- Example 13.6
- Example 13.7
- Example 13.8
- Example 13.9
- Exercise16.1
- Exercise16.2
- Exercise16.3
- Exercise16.4
- Exercise16.5
- Exercise16.6
- Exercise16.7
- Exercise16.8
- Introductory Exercise
- Level 1 Assertion And Reason
- Level 1 Single Correct
- Level 1 Subjective
- Level 2 Comprehension Based
- Level 2 More Than One Correct
- Level 2 Single Correct
- Match the columns
- Medical entranes gallery
- Miscellaneous Examples
- Solved Examples
- Subjective
- Subjective Question
- Taking it together