Complete Solutions to SECTION-B (ADDITIONAL EXERCISE) of chapter OSCILLATIONS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | OSCILLATIONS |
| KUMAR PRAKASHAN | SECTION-B (ADDITIONAL EXERCISE) |
| Number Of Questions | 9 |
| Academic Year | 2023-24 |
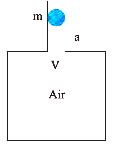
An air chamber of volume V has a neck area of cross section a into which a ball of mass m just fits and can move up and down without any friction (Fig.14.27). Show that when the ball is pressed down a little and released , it executes SHM. Obtain an expression for the time period of oscillations assuming pressure-volume variations of air to be isothermal [see Fig. 14.27].
View SolutionYou are riding in an automobile of mass 3000 kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15 cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by 50% during one complete oscillation. Estimate the values of the spring constant k.
View SolutionYou are riding in an automobile of mass 3000 kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15 cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by 50% during one complete oscillation. Estimate the values of the damping constant b for the spring and shock absorbar system of one wheel, assuming that each wheel supports 750 kg.
View SolutionShow that for a particle in linear SHM the average kinetic energy over a period of oscillation equals the average potential energy over the same period.
View SolutionA circular disc of mass 10 kg is suspended by a wire attached to its centre. The wire is twisted by rotating the disc and released. The period of torsional oscillations is found to be `1.5s`. The radius of the disc is 15 cm. Determine the torsional spring constant of the wire. (Torsional spring constant `alpha` is defined by the relation `J= -alpha theta`, where J is the restoring couple and `theta` the angle of twist).
View SolutionA body describes simple harmonic motion with an amplitude of 5 cm and a period of `0.2s`. Find the acceleration and velocity of the body when the displacement is 5 cm.
View SolutionA body describes simple harmonic motion with an amplitude of 5 cm and a period of `0.2s`. Find the acceleration and velocity of the body when the displacement is 3 cm.
View SolutionA body describes simple harmonic motion with an amplitude of 5 cm and a period of `0.2s`. Find the acceleration and velocity of the body when the displacement is 0 cm.
View SolutionA mass attached to a spring is free to oscillate, with angular velocity `omega`, in a horizontal plane without friction or damping. It is pulled to a distance `x_0` and pushed towards the centre with a velocity `v_0` at time t=0. Determine the amplitude of the resulting oscillaters `omega, x_(0)" and "v_(0)`. [Hint : Start with the equation `x= a cos (omega t+theta)` and note that the initial velocity is negative.]
View Solution
SECTION-B (ADDITIONAL EXERCISE) questions and complete solutions for chapter OSCILLATIONS of KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) of Class 11
All Exercises from KUMAR PRAKASHAN Class 11 PHYSICS OSCILLATIONS
- QUESTION PAPER (SECTION - A)
- QUESTION PAPER (SECTION - B)
- QUESTION PAPER (SECTION - C)
- QUESTION PAPER (SECTION - D)
- SECTION-A (QUESTIONS- ANSWERS)
- SECTION-A (TRY YOURSELF)
- SECTION-B (ADDITIONAL EXERCISE)
- SECTION-B (NUMERICAL FROM DARPAN BASED ON TEXTBOOK)
- SECTION-B (NUMERICAL FROM TEXTUAL EXERCISE)
- SECTION-B (NUMERICAL FROM TEXTUAL ILLUSTRATIONS)
- SECTION-C (OBJECTIVE QUESTIONS ASSERTION OR REASION)
- SECTION-C (OBJECTIVE QUESTIONS FILL IN THE BLANK)
- SECTION-C (OBJECTIVE QUESTIONS MATCHING)
- SECTION-C (OBJECTIVE QUESTIONS TRUE OR FALSE)
- SECTION-C (OBJECTIVE QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION LONG ANSWER QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION MCQs MORE THAN ONE OPTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION MCQs)
- SECTION-D (NCERT EXEMPLAR SOLUTION SHORT ANSWER QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION VERY SHORT ANSWER QUESTIONS)
- SECTION-E (MCQs ASKED IN GUJARAT BOARD AND COMPETITIVE EXAMS)
- SECTION-F (QUESTIONS FROM MODULE SIMPLE QUESTIONS)