Complete Solutions to SINGLE ANSWER QUESTIONS of chapter NEWTONS LAWS OF MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | NEWTONS LAWS OF MOTION |
| NARAYANA | SINGLE ANSWER QUESTIONS |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
The car A is used to pull a load `B` with the pulley arrangement shown. If A has a forwed pulley arraangement shown. If A has a forward velcotiy `v_(A)` determine an experssion for the upward velcotiy `v_(B)` of the load in terms of `V_(A)` and `theta, theta` is angle between string and horizontal
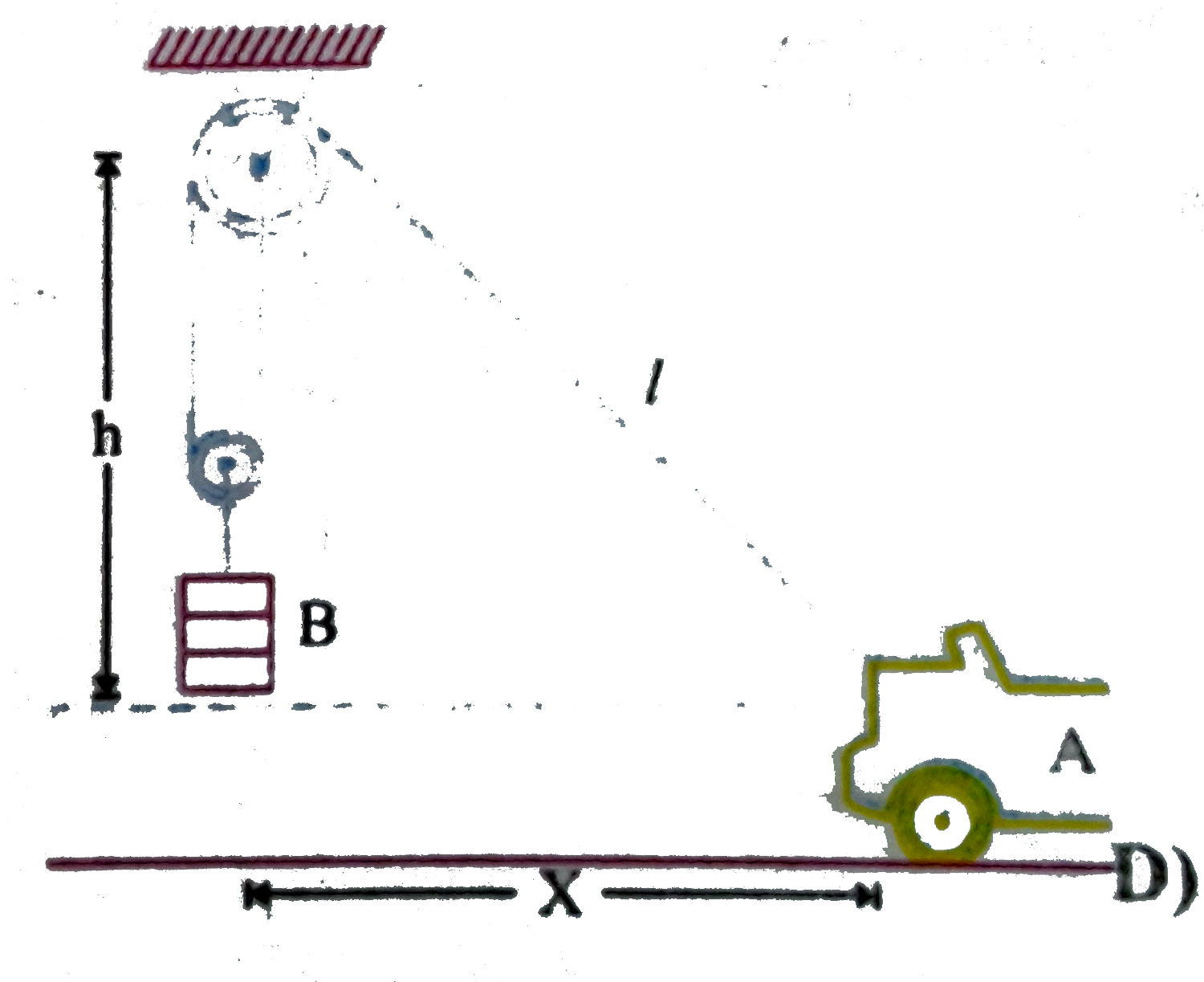 .
.
View SolutionIdentify the relationship which governs the velocities of the four cylinders. Assume all velocities as positive downward
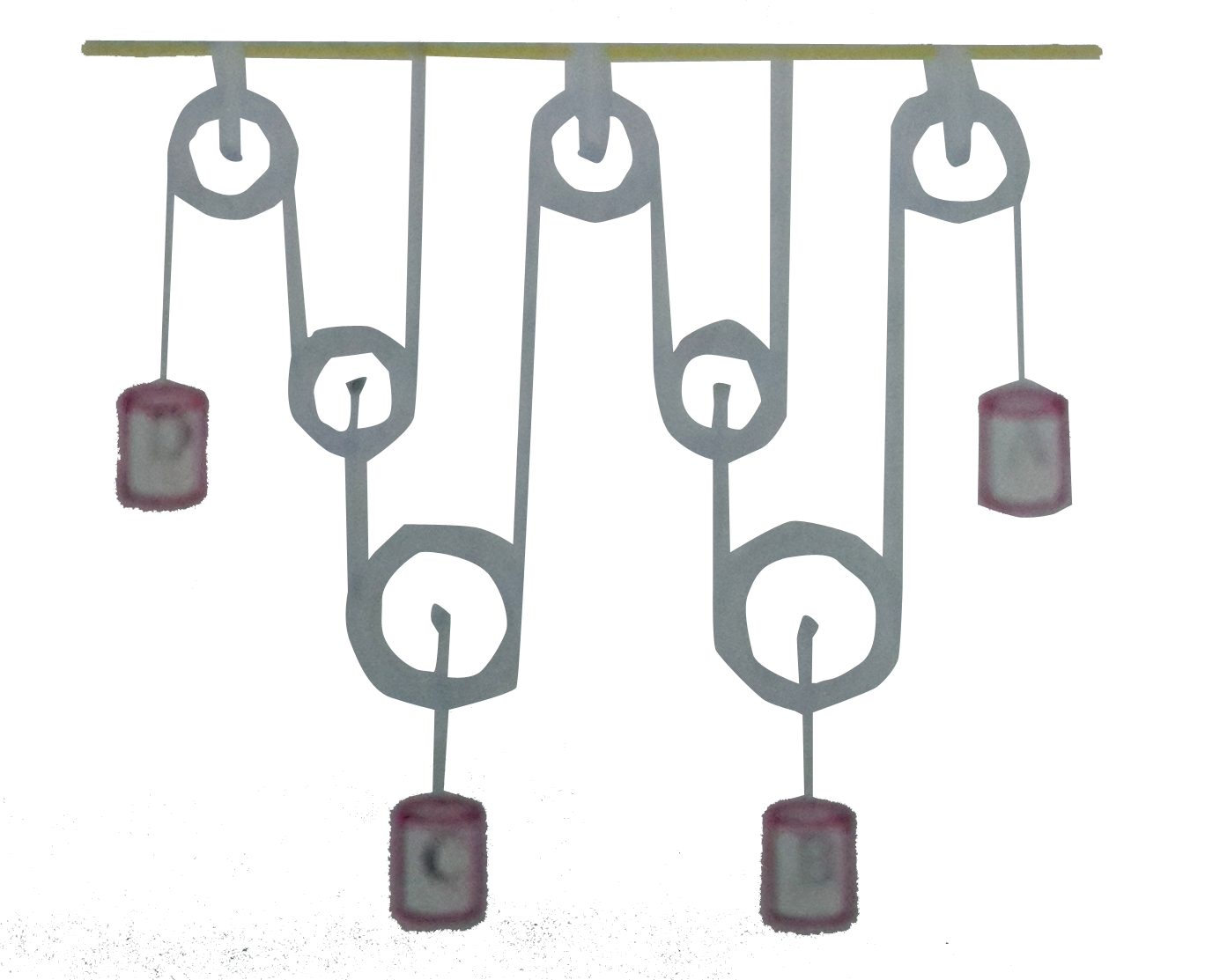 .
.
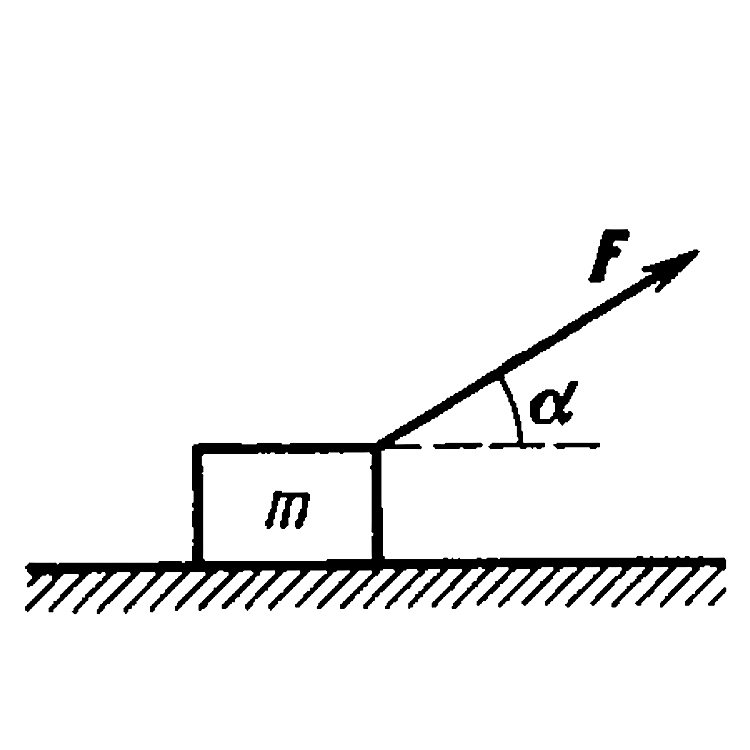
View SolutionAt the moment `t=0` the force `F=at` is applied to a small body of mass `m` resting on a smooth horizontal plane (a is constant).
The permanent direction of this force forms an angle `alpha` with the horizontal (figure). Find:
(a) the velocity of the body at the moment of its breaking off the plane,
(b) the distance traversed by the body up to this moment.
View SolutionThe vertical displacement of a block A in meter is given by `y=t^(2)//4` where t is in second. The downward acceleration `a_(B)` of a block B ( in `m//s^(2)`) is

View SolutionFind the acceleration of block `B` realtive to the ground if the block A moves to the left with an acceleration `a_(0)`
 .
.
View SolutionUnder to action of force `P` the constant acceleration of block `B` is `3ms^(-2)` to the right At the instant when the velcoity of `B` is `2ms^(-1)` to the right determine the absolute velocity of point `C` of the cable
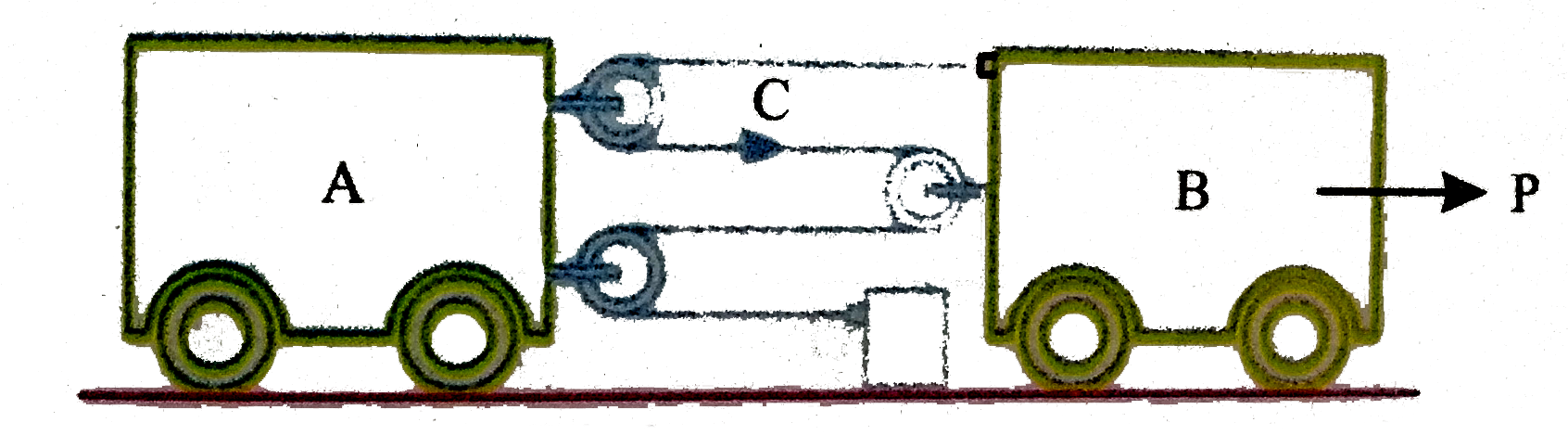 .
.
View SolutionBlock B has mass m and is released from rest when it is on top of wedge A, which has a mass 3m. Determine the tension in cord CD needed to hold the wedge from moving while B is sliding down A. Neglect friction.

View SolutionFind the acceleration of the body of mass `m_(2)` in the arrangement shown in figure. If the mass`m_(2)` is `eta` time great as the mass `m_(1)` and the angle that the inclined plane forms with the horizontal is equal to `theta`. The masses of the pulley and threads, as well as the friction, are assumed to be negligible.
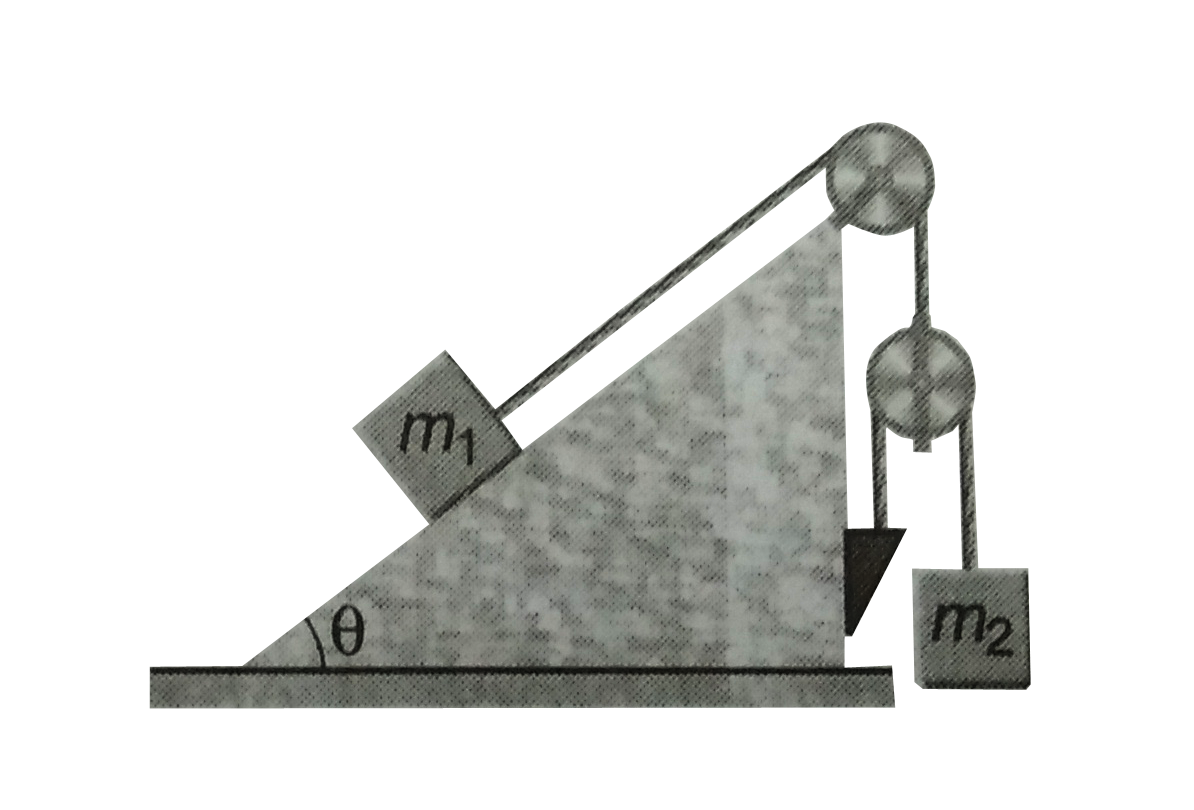
View SolutionIf `A` and `B` moves with acceleration a block c moves up with acceleration b calculate acceleration of `D` with respective `A`.
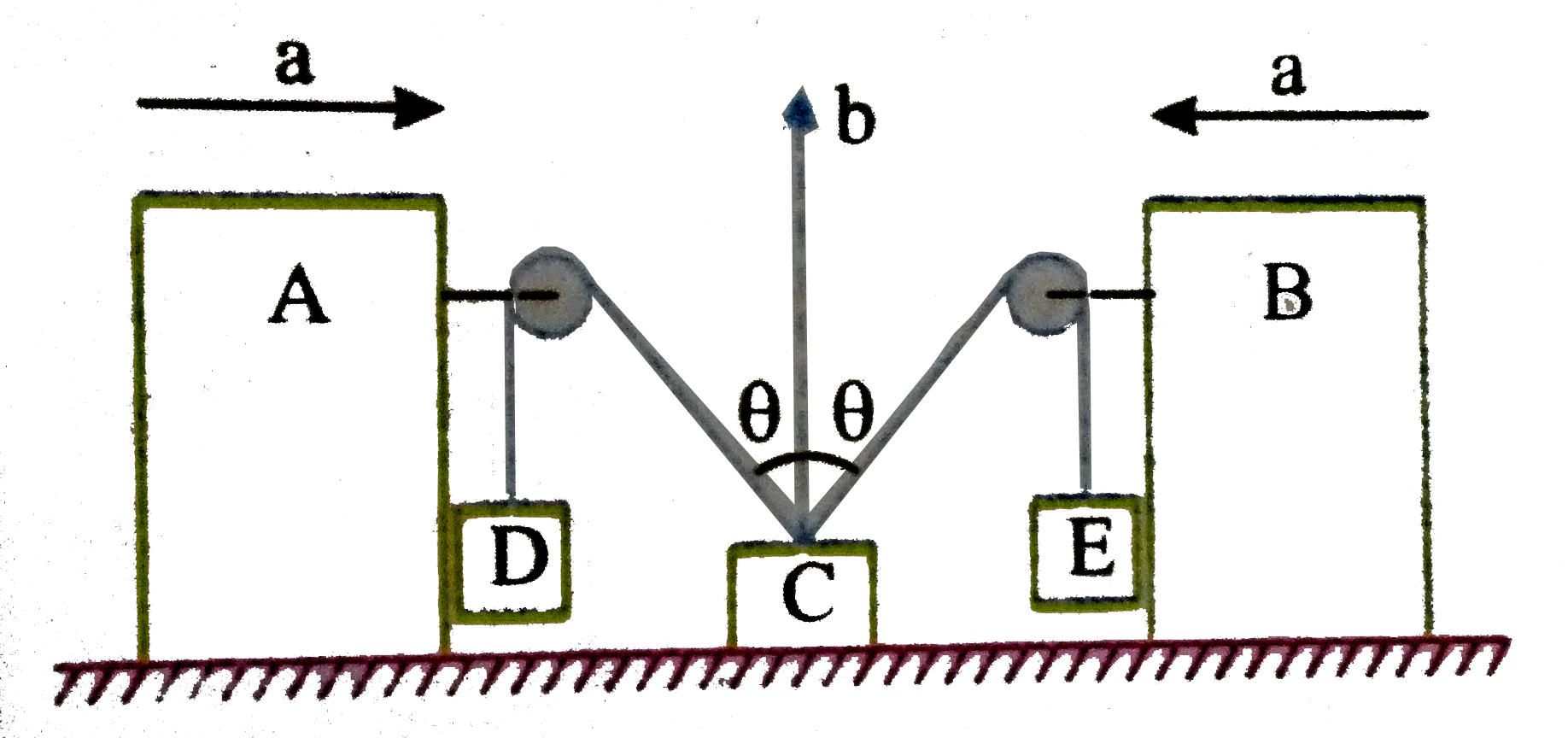 .
.
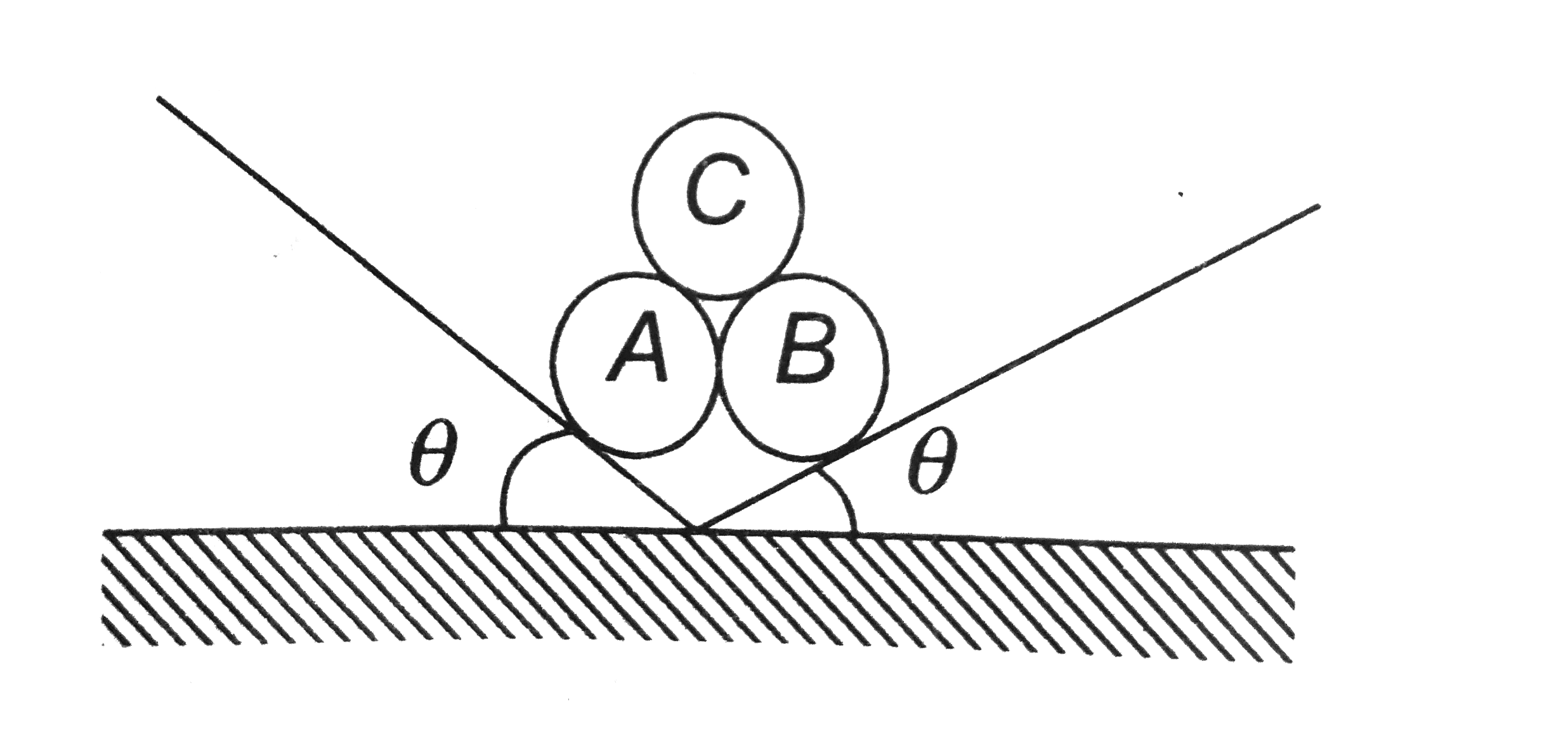
View SolutionThree identical rigid circular cylinder `A` `B` and `C` are arrenged on smooth inclined surfaces as shown in figure. The laest value of theta that prevent the arrangement from collapes is.
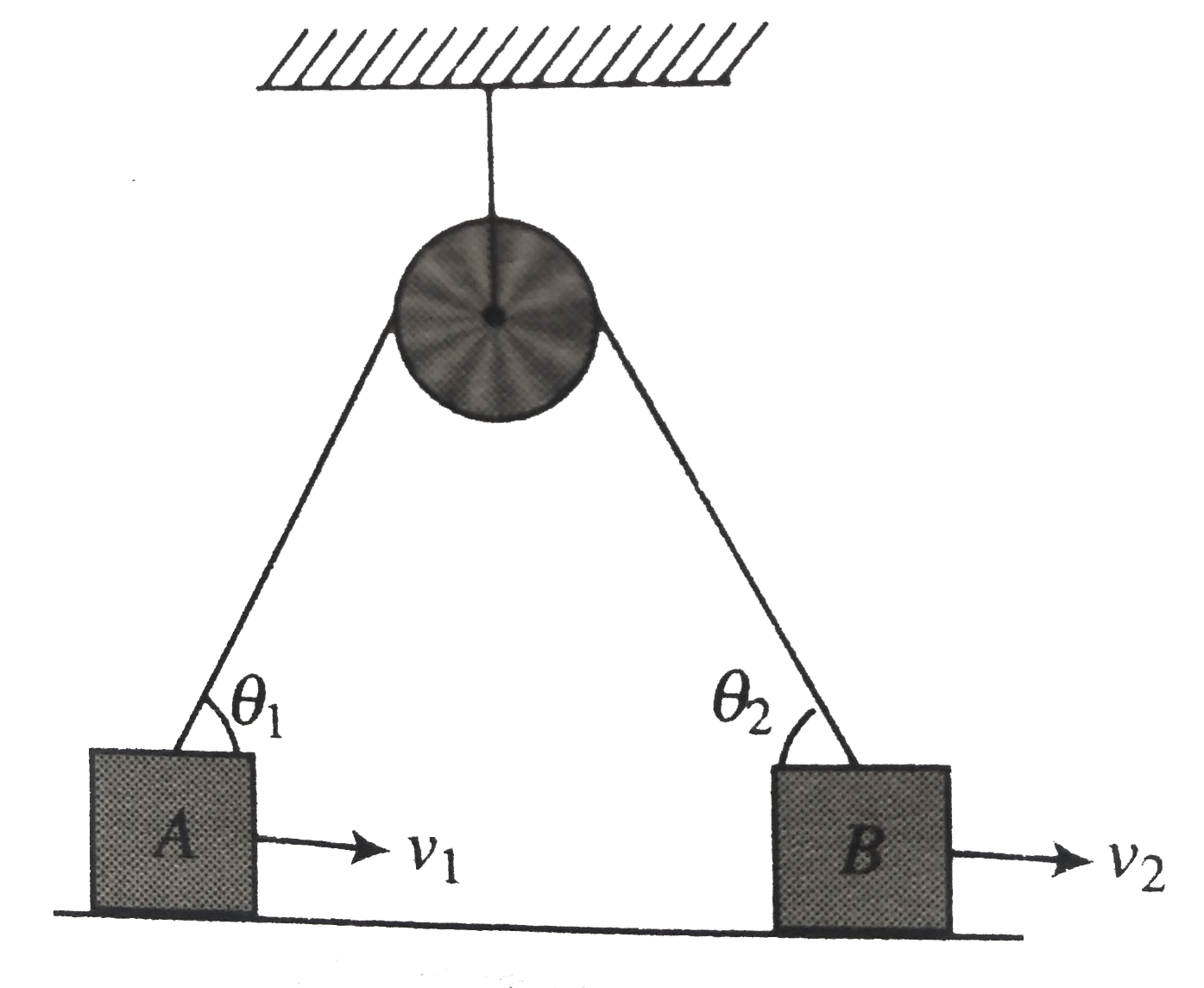
View SolutionIn fig., blocks A and B move with velocities `v_(1)` and `v_(2)` along horizontal direction. Find the ratio of `v_(1)//v_(2)`
View SolutionIn the arrangements shown, the pulleys, strings and springs are weightless and the systems can move freely without friction The extension of spring in `1` is `x_(1)` and that in `2` is `x_(2)` Then
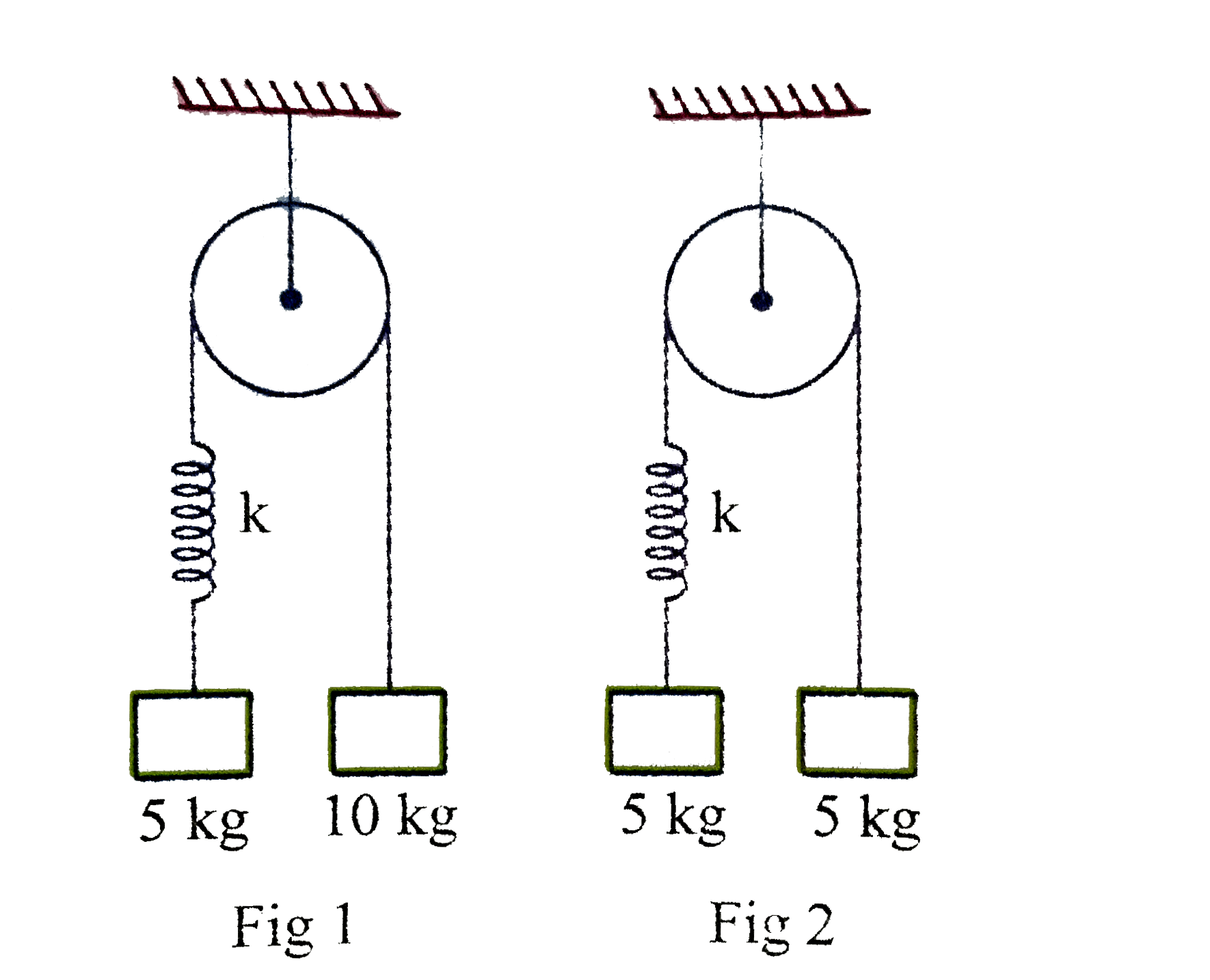 .
.
View SolutionFigure shows a system of four pulleys with two masses `m_(A) =3kg` and `m_(B)=4 kg`. At an instant force acting on block A if block `B` is going up at an acceleration of `3 m//s^(2)` and pulley `Q` is going down at an acceleration of `1 m//s^(2)` is
 .
.
View SolutionIf `A` and `B` moves with acceleration a as shown in diagram calculate accelration of `C` with respect to `B`
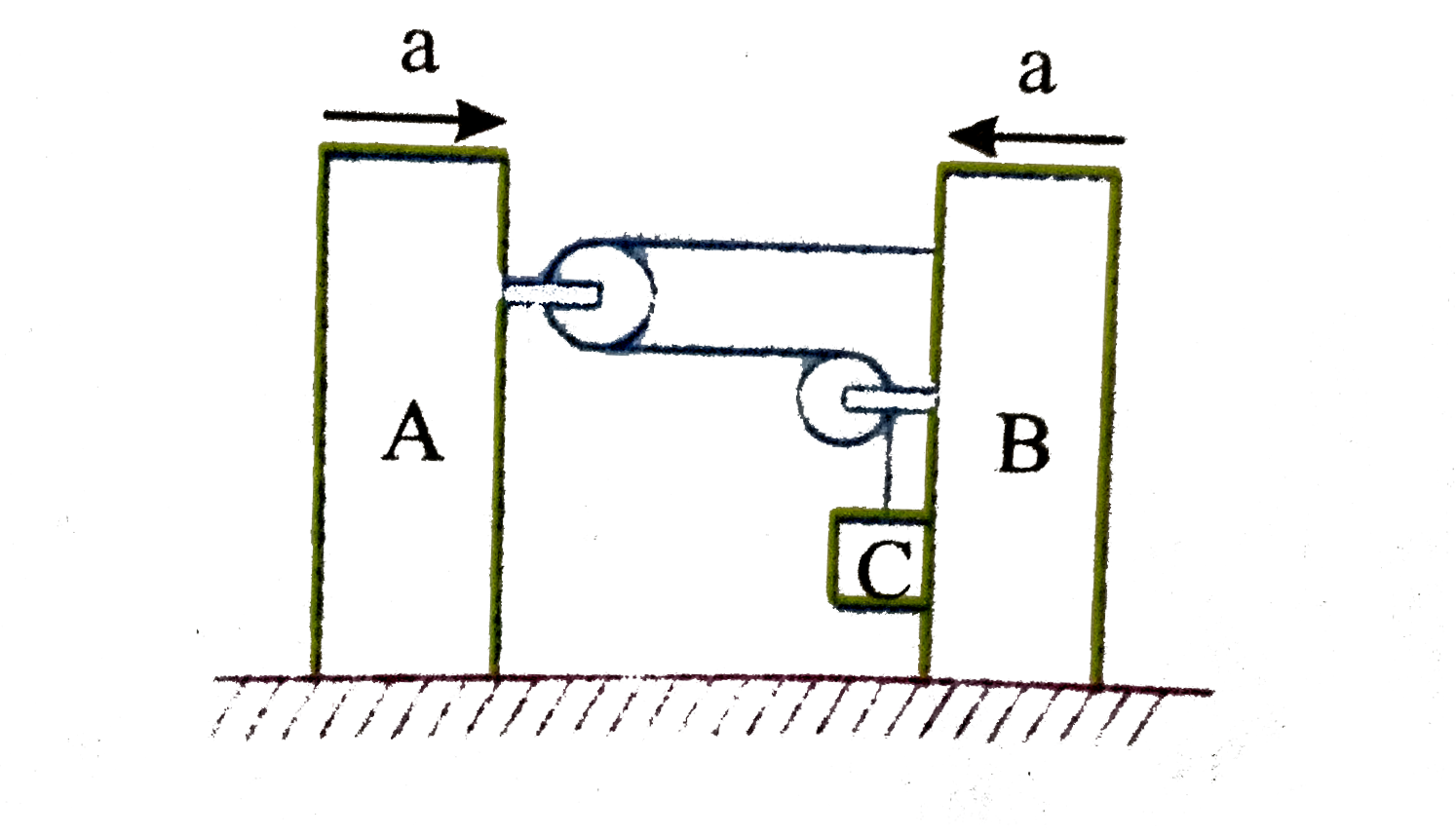 .
.
View SolutionIn the small block m is kept on planck of mass `M` and a force `F` is applied on planck as shown in diagram then which of the following statements is/are correct
 .
.
View SolutionA particle of mass m starts moving at `t =0` due to force `F =F_(0) sin omegat` where `F_(0)` and `omega` are constant Then correct statement is//are .
View SolutionFrom the given, choose the correct option
 .
.
View SolutionIn the diagram shown, the acceleration of the block `B` as shown in relative to the block A and relative to ground is `a_(BA)` and `a_(BG)` respectively. If the block A is moving towards left with an acceleration `a_(0)` then
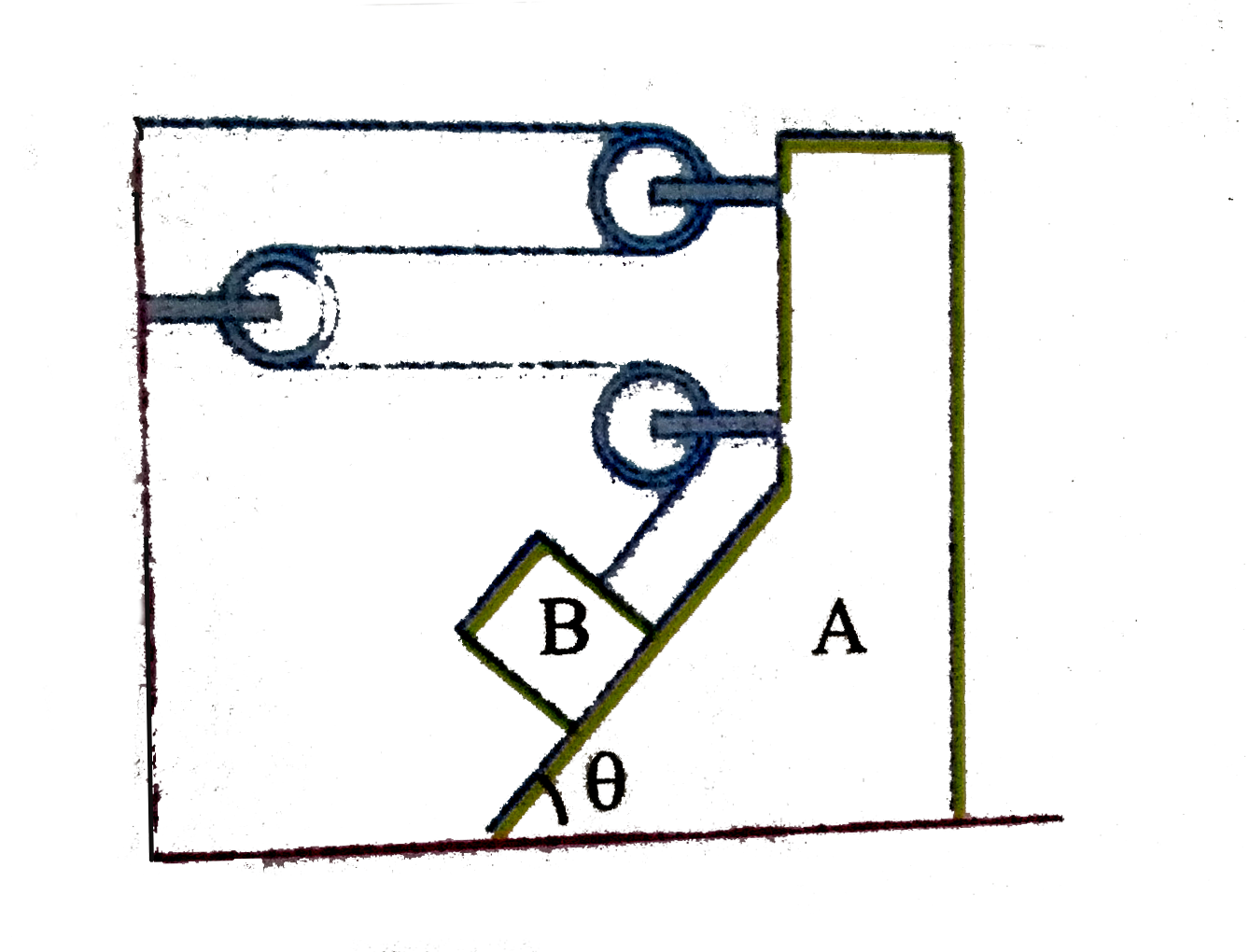 .
.
View SolutionIn the pulley system shown the movable pulleys `A,B` and `C` have mass m each, `D` and `E` are fixed pulleys. The strings are vertical light and inextensible Then
 .
.
View SolutionA block of mass m is placed on a wedge The wedge can be accelerated in four manners marked as `(1),(2),(3)` and (4) as shown. If the normal reactions in situation `(1),(2),(3)` and (4) are `N_(1),N_(2),N_(3)` and `N_(4)` respectively and acceleration with which the block slides on the wedge in situation are `b_(1),b_(2),b_(3)` and `b_(4)` respectively then

View SolutionA body of mass `m =18kg` is placed on an inclined plane the angle of inclination is `alpha =37^(@)` and is attached to the top end of the slope with a thread which is parallel to the slope. Then the plane slope is moved with a horizontal acceleration of a. Friction is negligible.
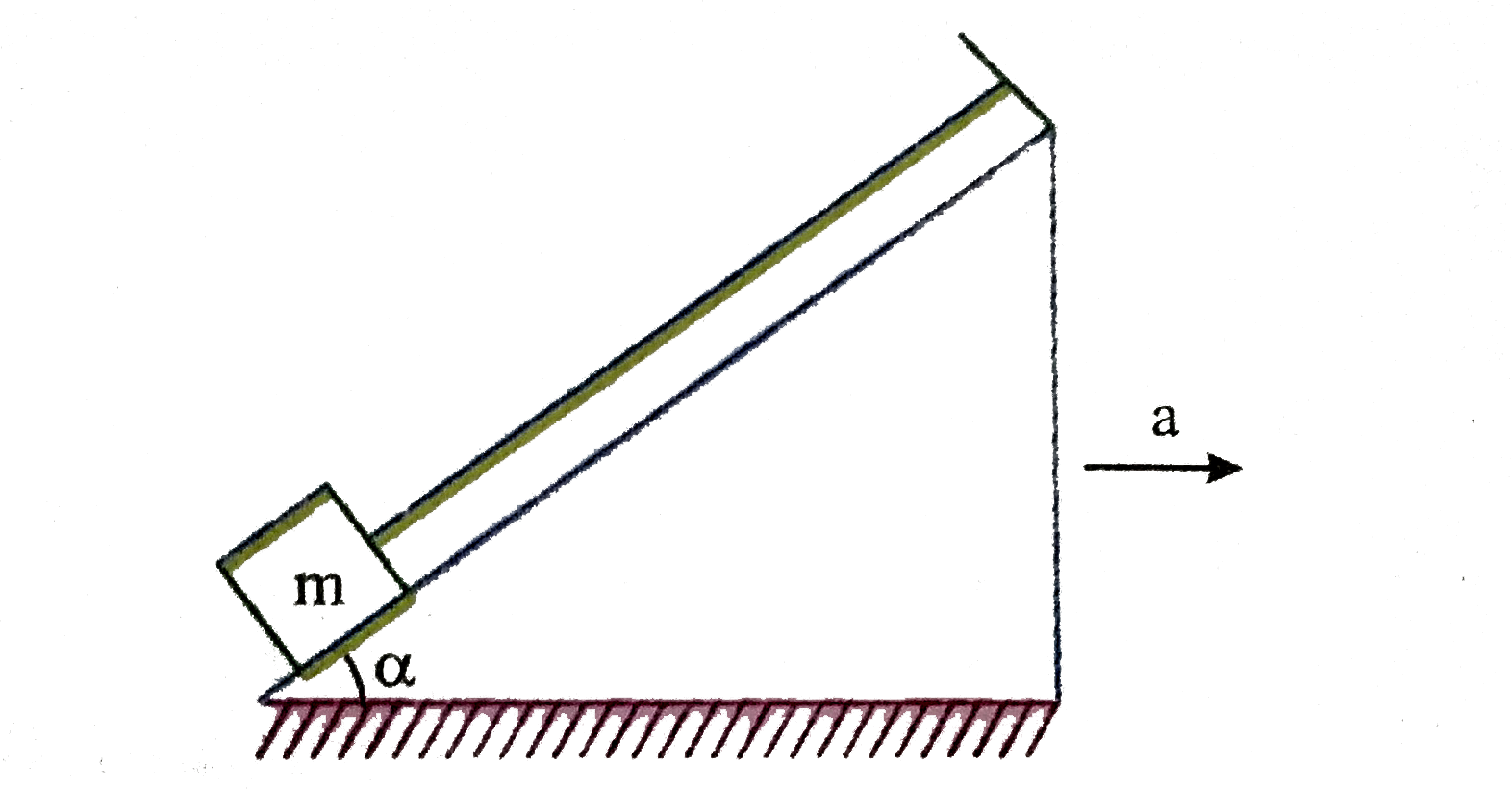
The tension in thread in the above question is .
View SolutionA body of mass `m =18kg` is placed on an inclined plane the angle of inclination is `alpha =37^(@)` and is attached to the top end of the slope with a thread which is parallel to the slope. Then the plane slope is moved with a horizontal acceleration of a. Friction is negligible.
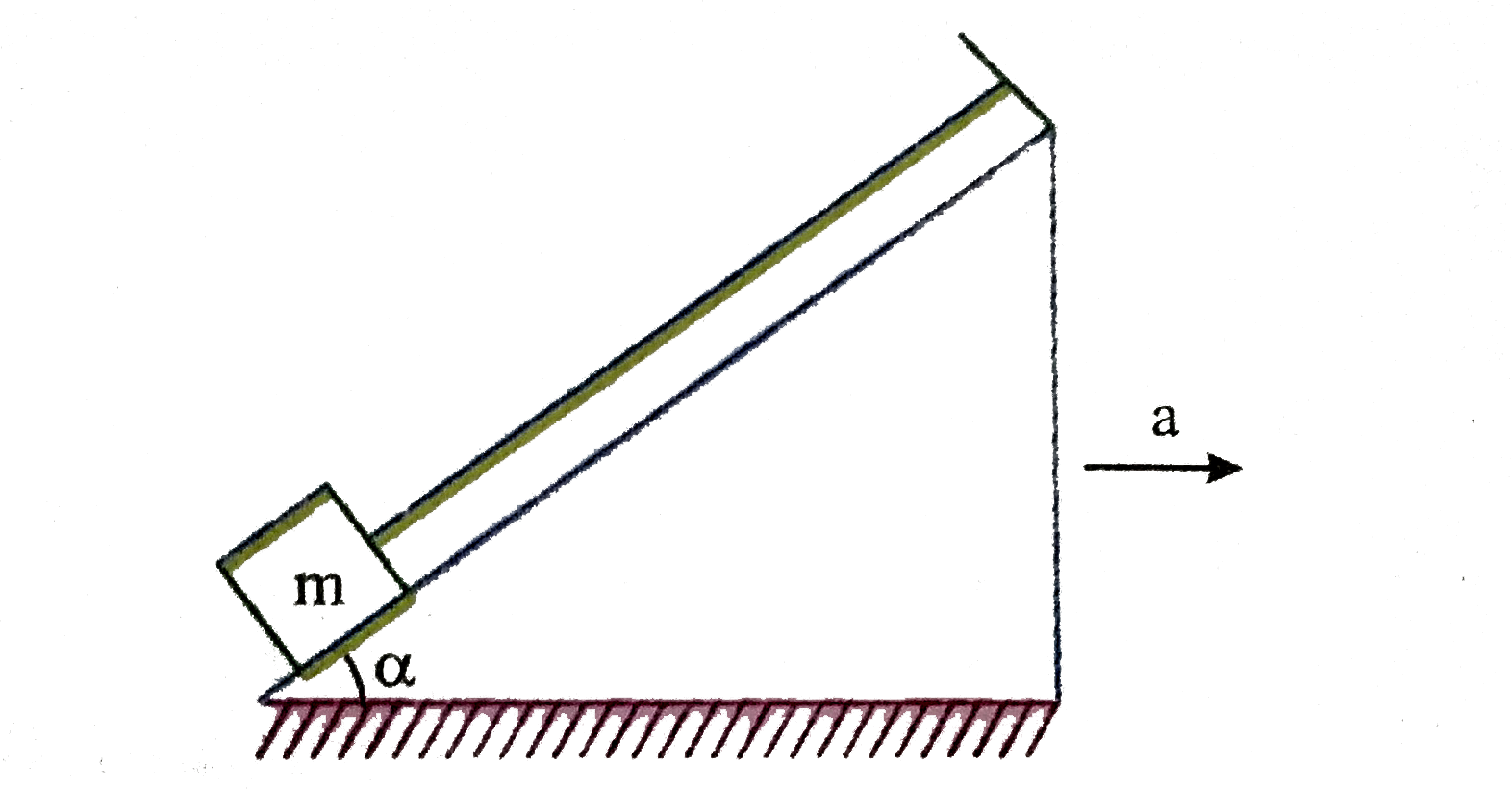
At what acceleration will the body lose contact with plane .
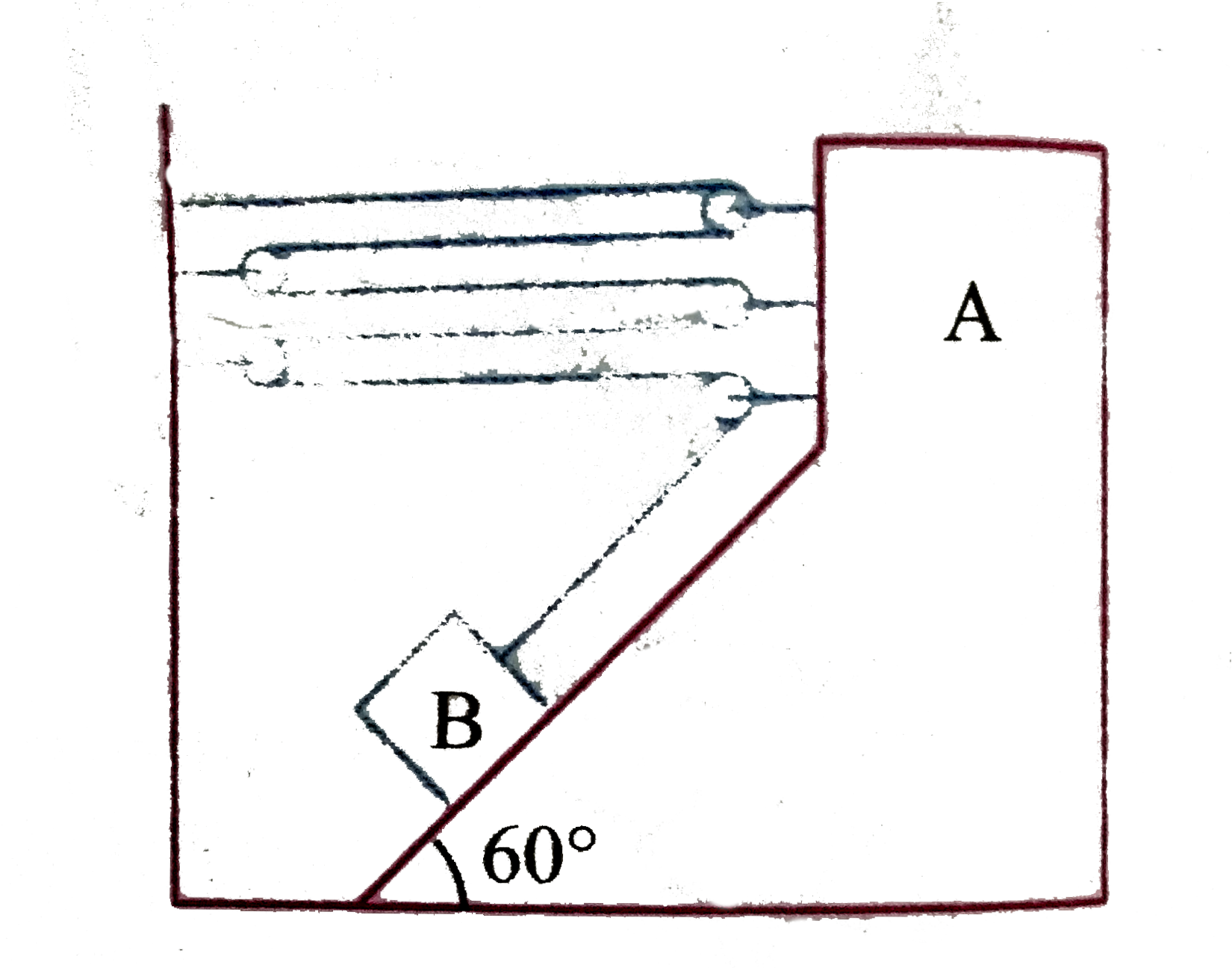
View SolutionTwo smooth block are placed at a smooth corner as shown in fig. Both the bloks are having mass m. We apply a force F on the block m. Block A presses block B in the normal direction, due to which pressing force on vertical wall will increase, and pressing force on the horizontal wall decreases, as we increases `F(theta = 37^(@)` with horizontal).
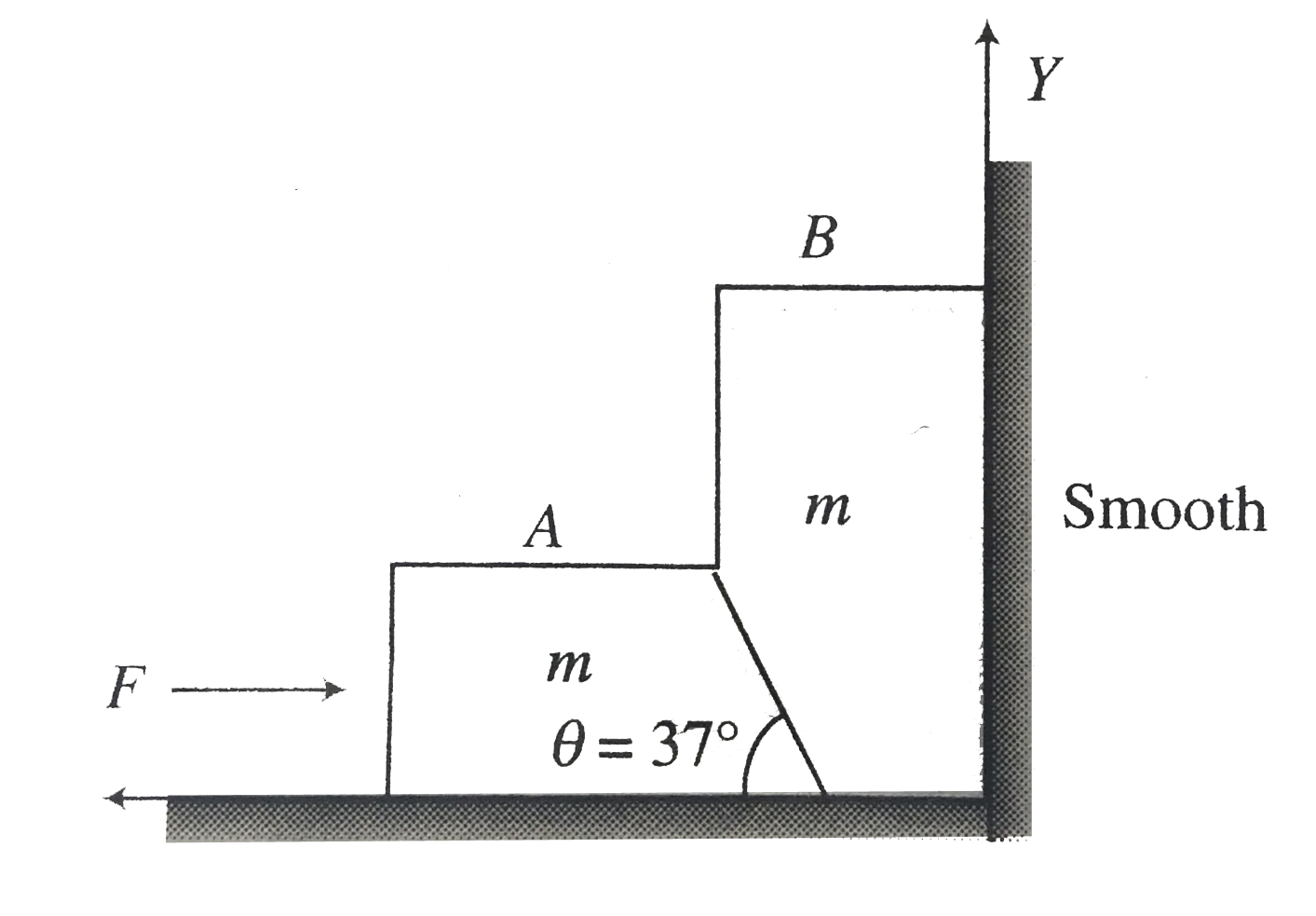
As soon as the pressing force on the horizontal wall by block B become zero, it will lose contact with ground. If the value of F further increases, block B will accelerate in the upward direction and simulaneously block A will towards right.
What is the minimum value of F to lift block B from ground?
View SolutionTwo smooth block are placed at a smooth corner as shown in fig. Both the bloks are having mass m. We apply a force F on the block m. Block A presses block B in the normal direction, due to which pressing force on vertical wall will increase, and pressing force on the horizontal wall decreases, as we increases `F(theta = 37^(@)` with horizontal).
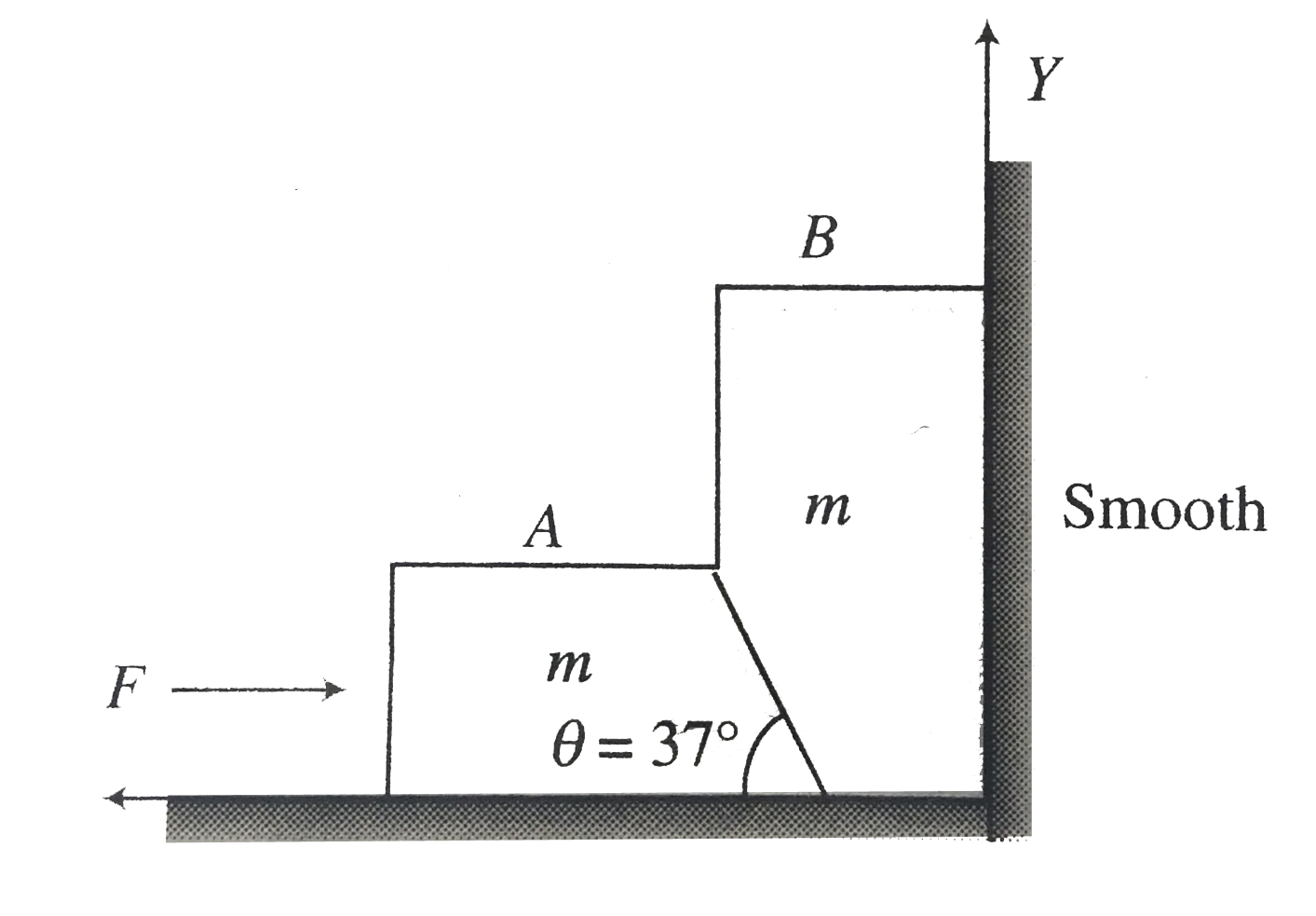
As soon as the pressing force on the horizontal wall by block B become zero, it will lose contact with ground. If the value of F further increases, block B will accelerate in the upward direction and simulaneously block A will towards right.
If both the blocks are stationary, the force exerted by ground of block A is
View SolutionTwo smooth block are placed at a smooth corner as shown in fig. Both the bloks are having mass m. We apply a force F on the block m. Block A presses block B in the normal direction, due to which pressing force on vertical wall will increase, and pressing force on the horizontal wall decreases, as we increases `F(theta = 37^(@)` with horizontal).
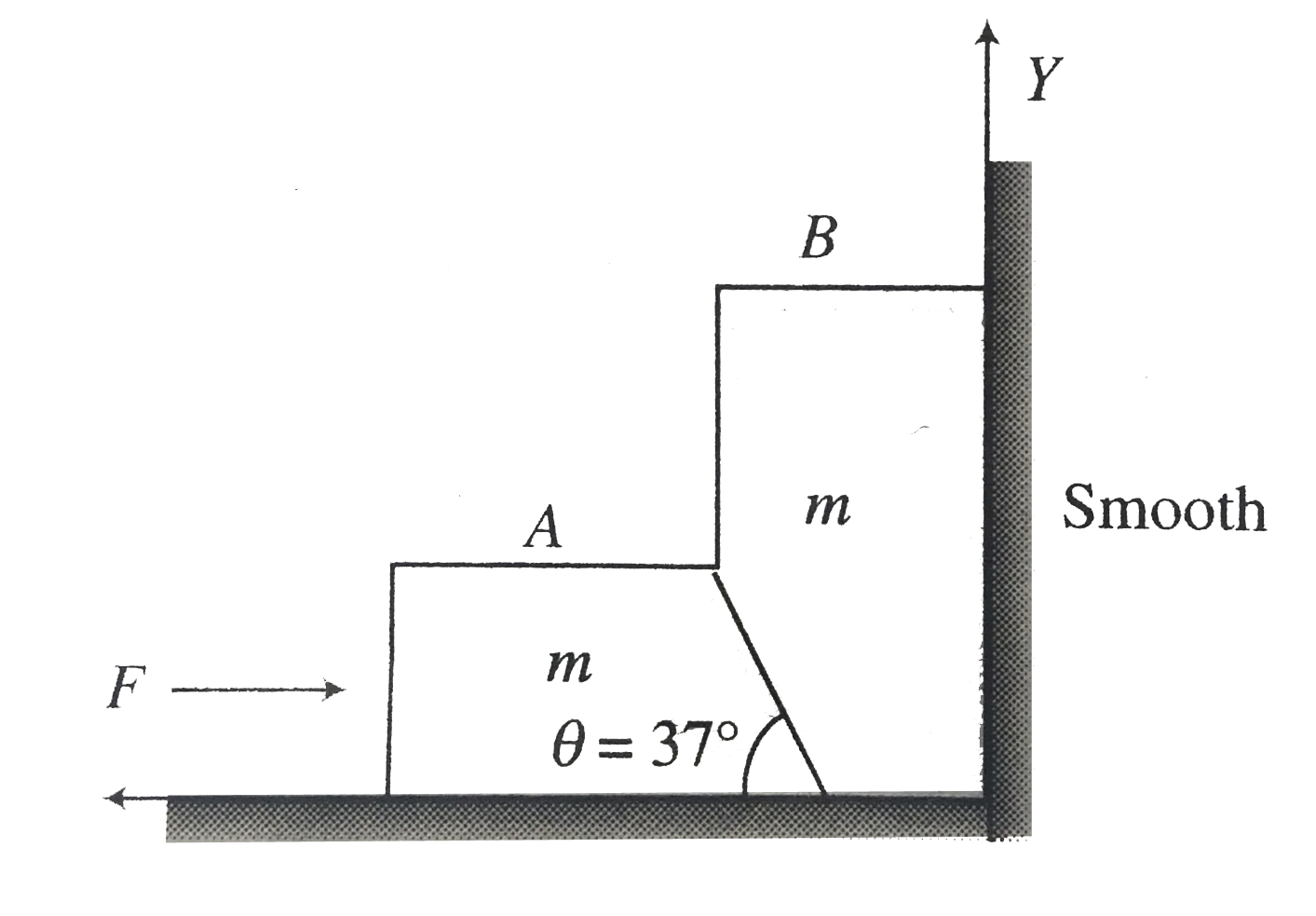
As soon as the pressing force on the horizontal wall by block B become zero, it will lose contact with ground. If the value of F further increases, block B will accelerate in the upward direction and simulaneously block A will towards right.
If the acceleration of block A is a rightwards, then the acceleration of block B will be
View SolutionIn the figure heavy mass m moves down the smooth surface of a wedge making an angle `alpha` with the horizontal. The wedge at rest `t =0` is on a smooth surface. The mass of the wedge is `M` the direaction of motion of the mass m makes an angle `beta` with the horizontal then, `"Tan" beta` is
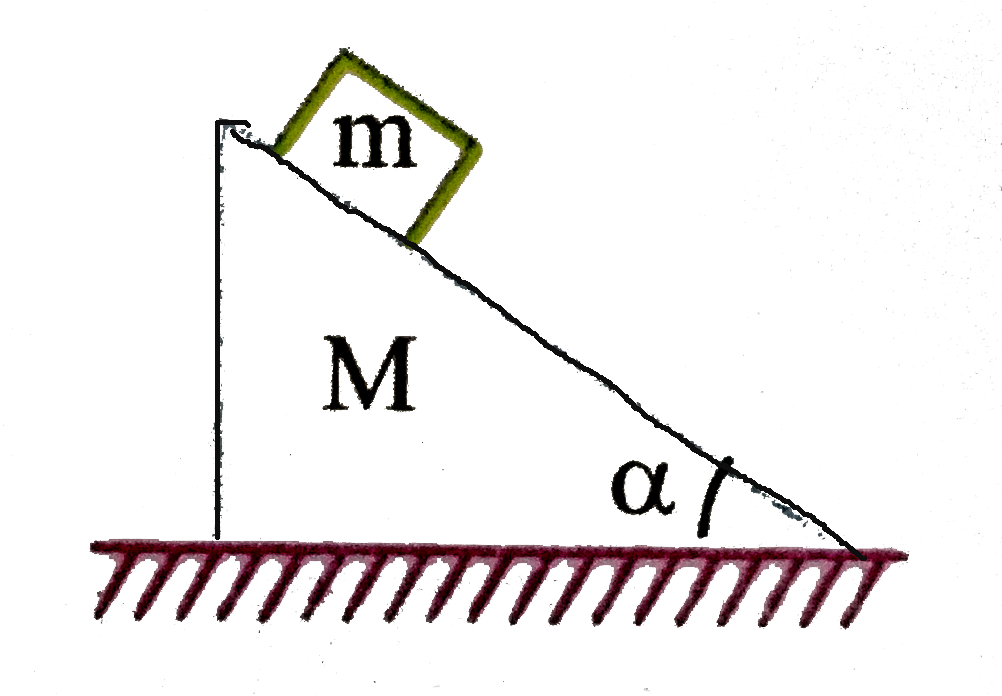 .
.
View SolutionA weightless inextensible rope rests on a stationary wedge forming an angle `alpha` with a horizontal One end of the rope is fixed to the wall to point A.A small load is attached to the rope at point `B` The wedge starts moving to the right with a constant acceleration a The acceleration of the load is given by
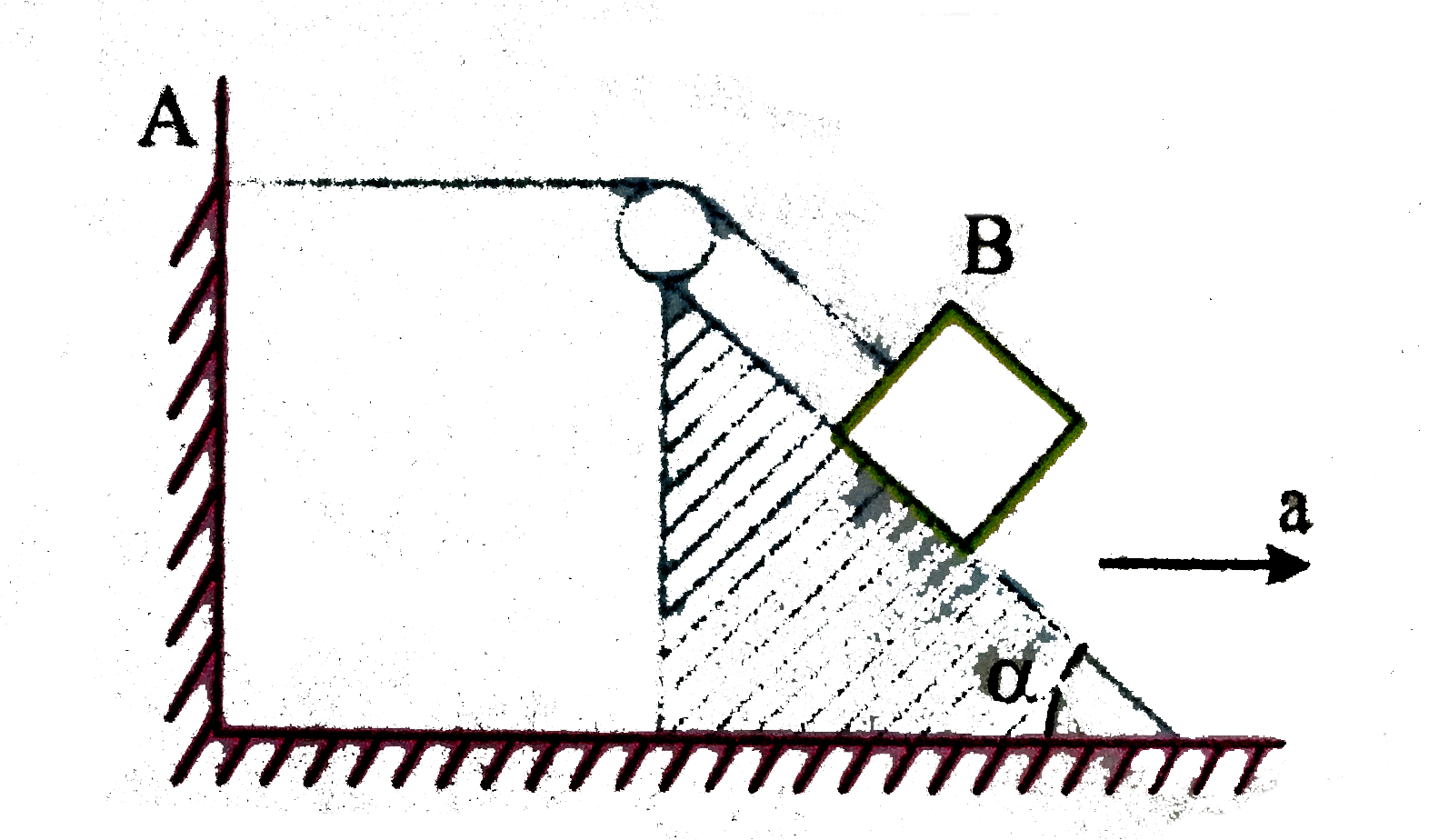 .
.
View SolutionBlock is attached to system of springs. Calculate equivalent spring constant.
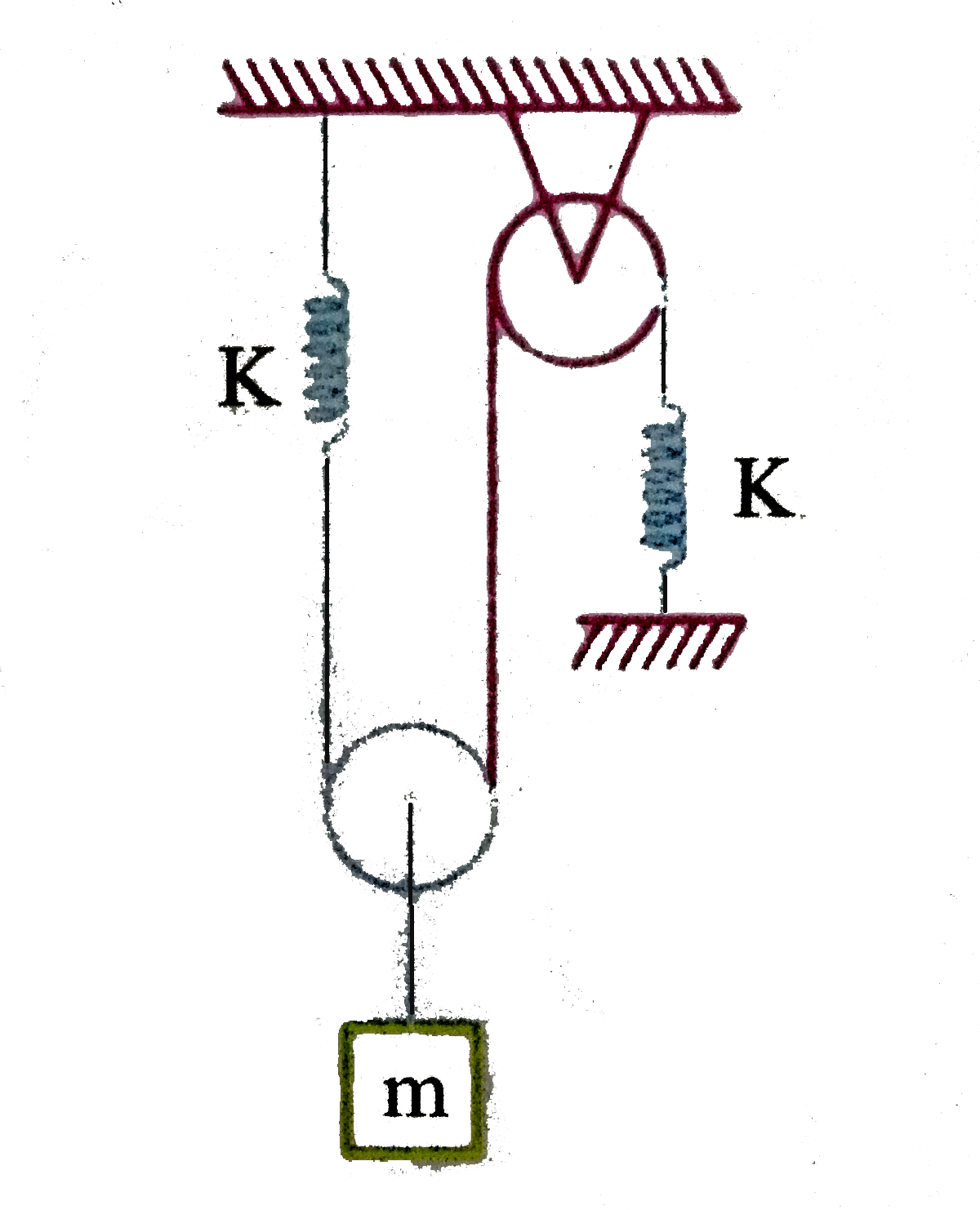 .
.
View SolutionBlock A and C starts from rest and move to the right with acceleration `a_(A)=12tms^(-2)` and `a_(C )=3ms^(-2)`. Here t is in second. The time when block B again comes to rest is
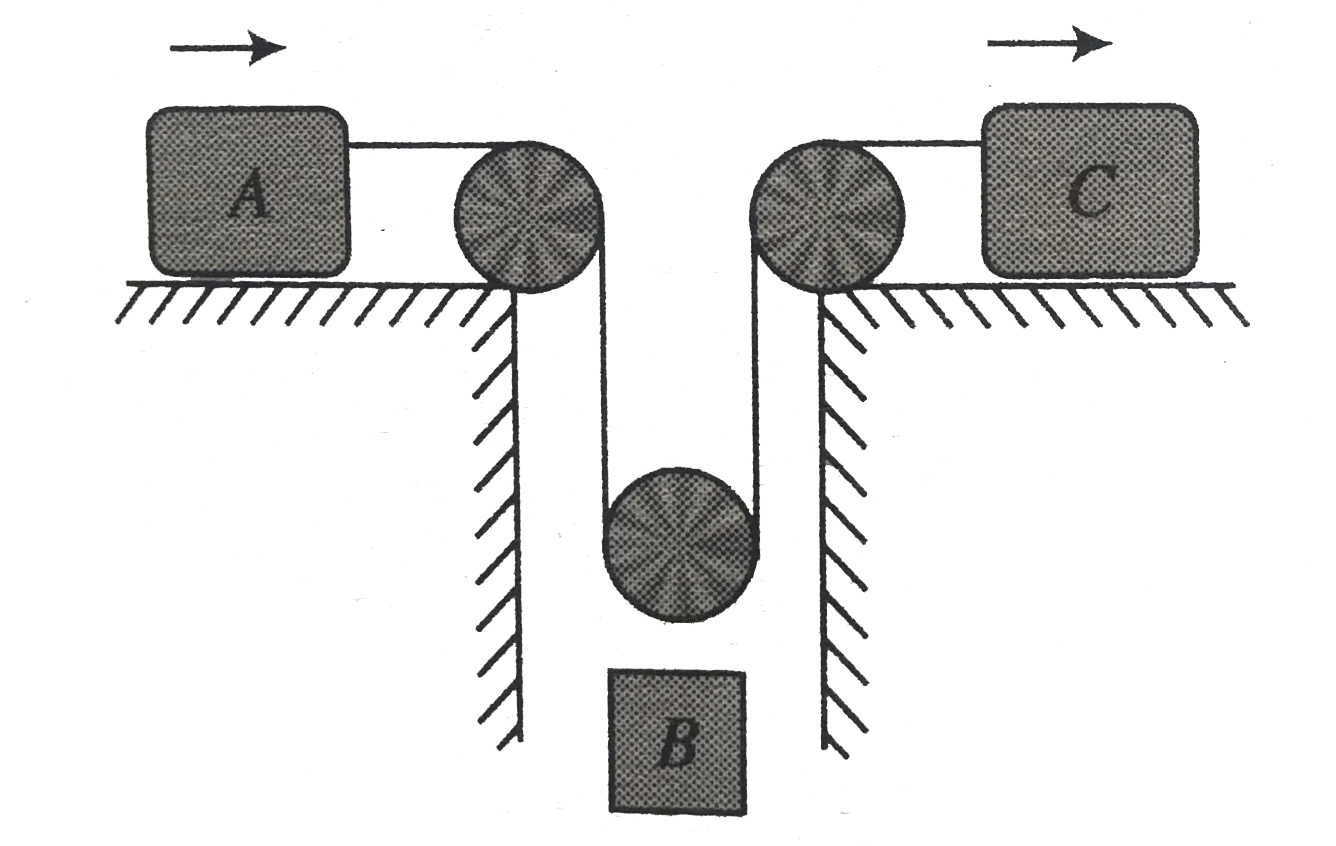
View SolutionIn the arrangement shown in fig. `m_(1)=1kg, m_(2)=2 kg`. Pulleys are massless and strings are light. For what value of M, the mass `m_(1)` moves with constant velocity.

View SolutionFind equivalent spring constant for the system
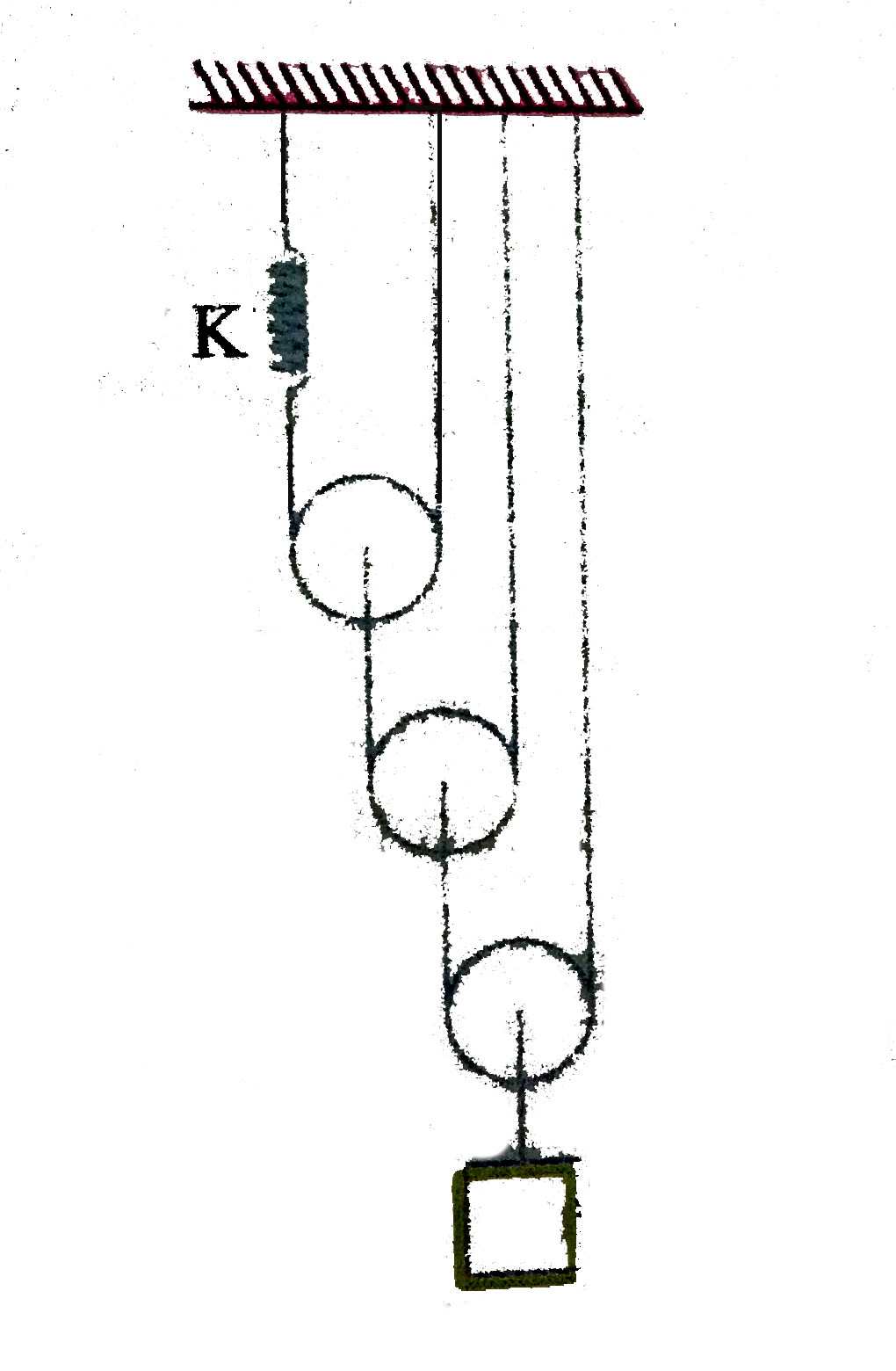 .
.
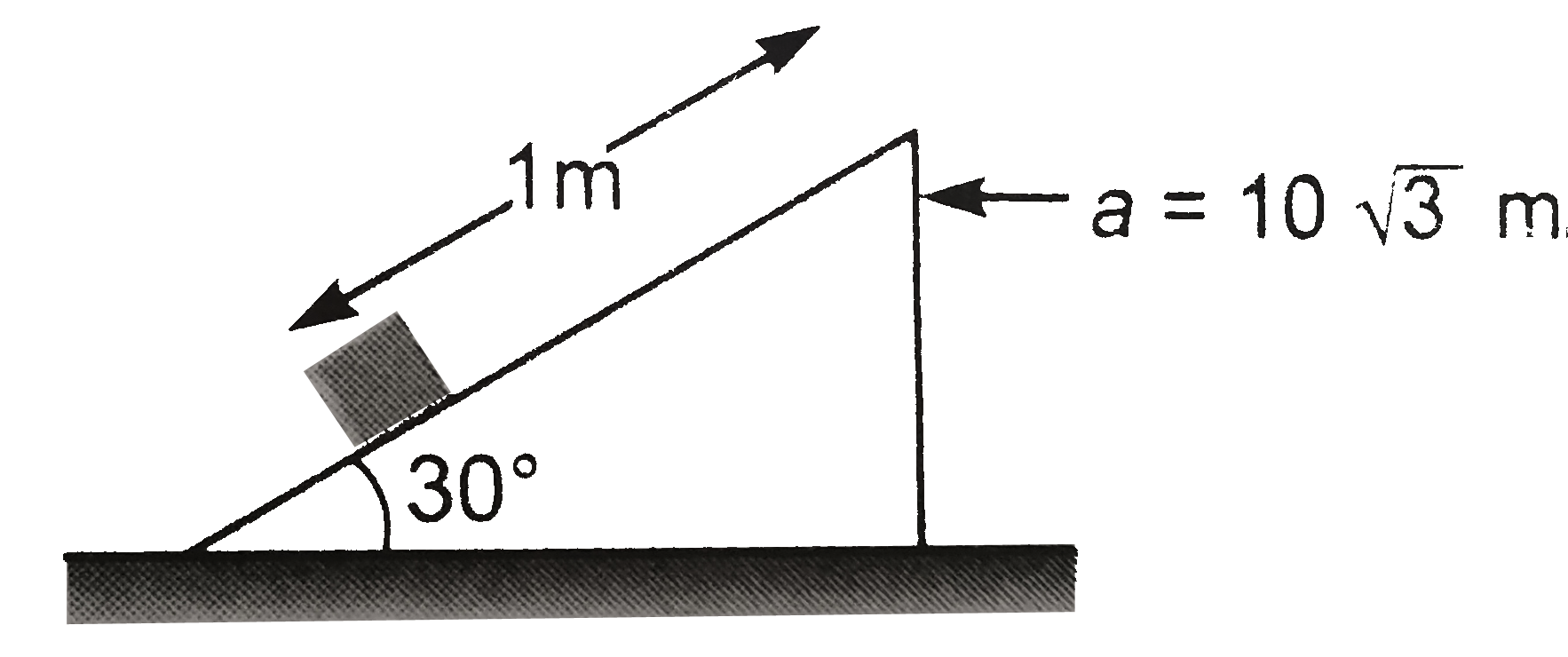
View SolutionIn the figure the wedge is pushed with an acceleration of `sqrt3 m//s ^(2)`. It is seen that the block start climbing up on the smooth inclined face of wedge . What will be the time taken by the block to reach the top?
View SolutionIn the above diagram system is in equilibrium if applied force `F` is doubled how much mass less block will more towards right before new equilibrium is achieved
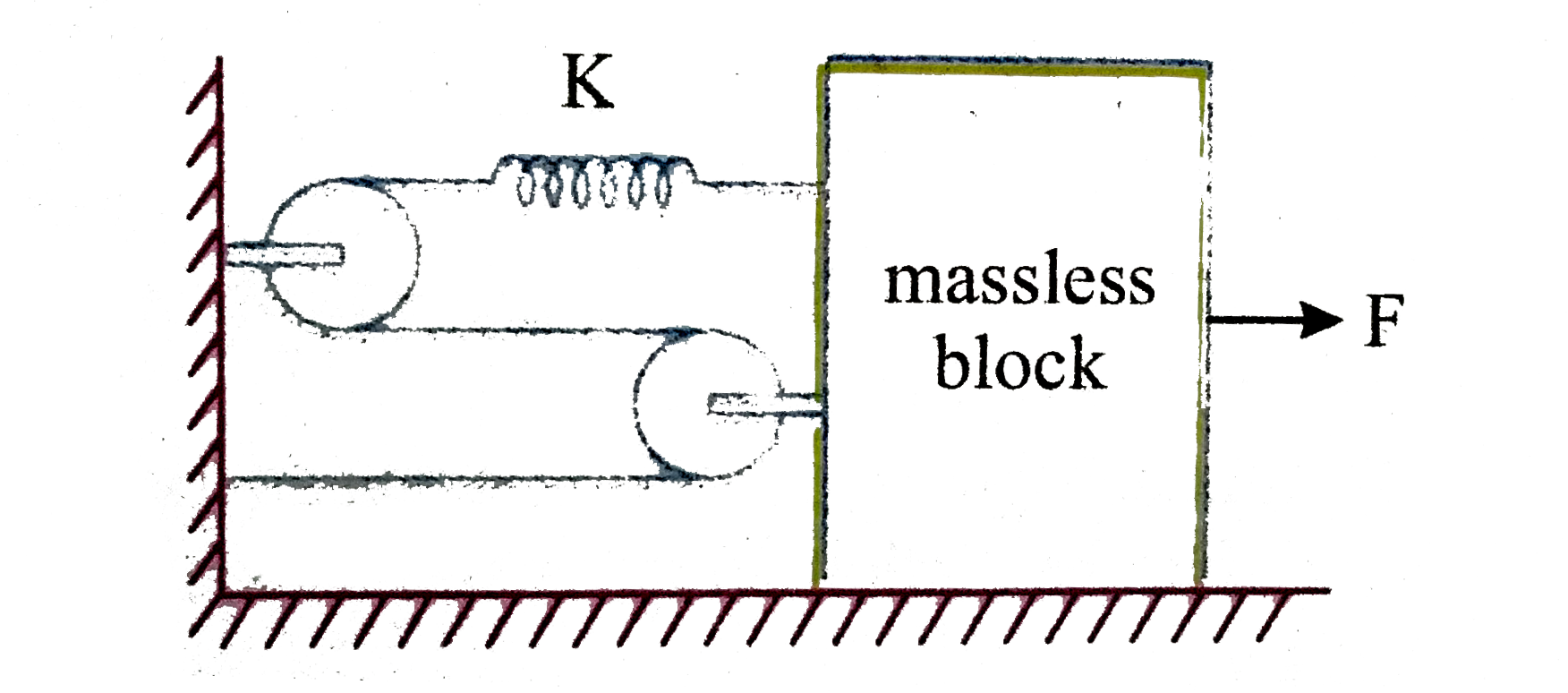 .
.
View SolutionIn the above diagram all surface friction less what horizontal force has to be applied on wedge such in equilibrium steady state sping is compressed by `(mg sin theta)/(K)`
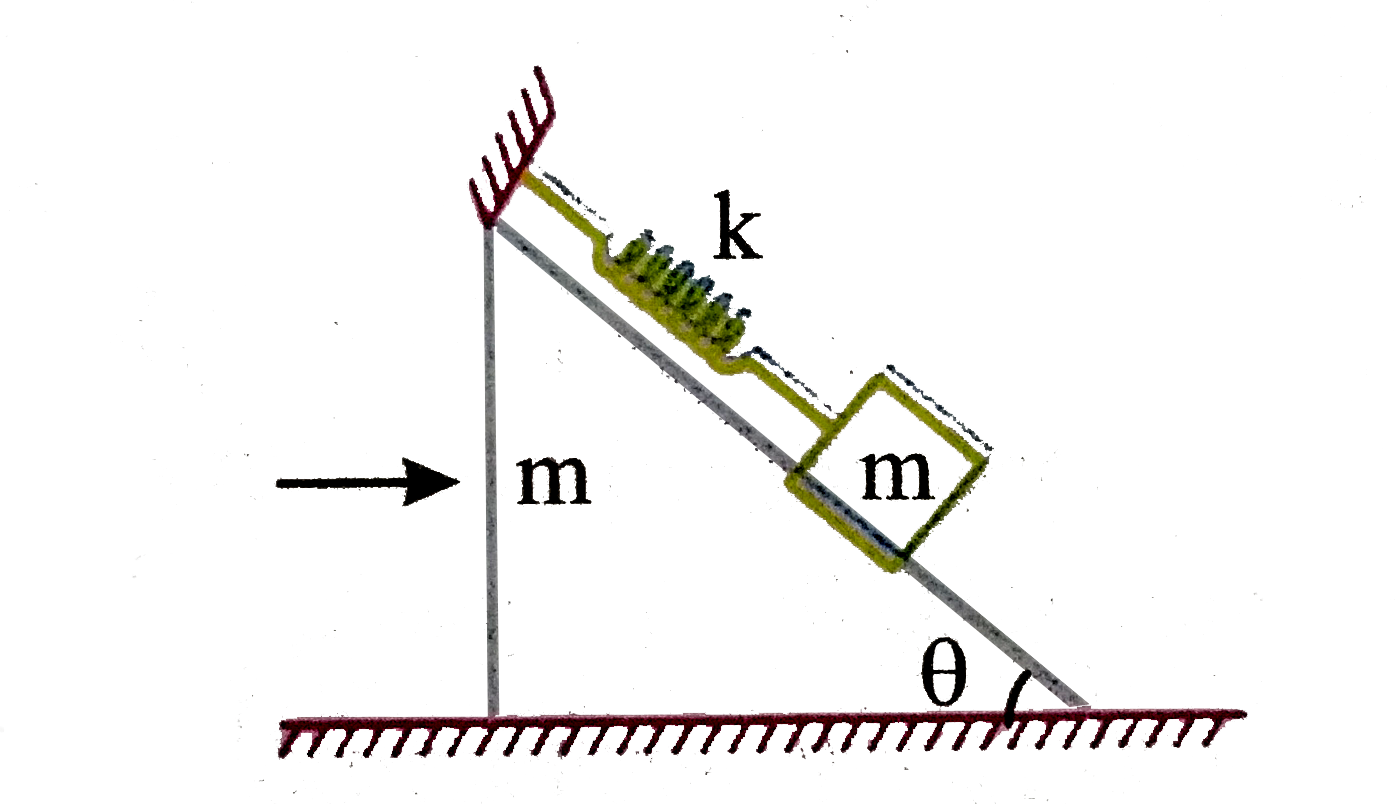 .
.
View SolutionIf the above diagram initially there is no elongation in spring if the block is displaced towards right by `x_(0)`. Calculate the elongation of spring A.
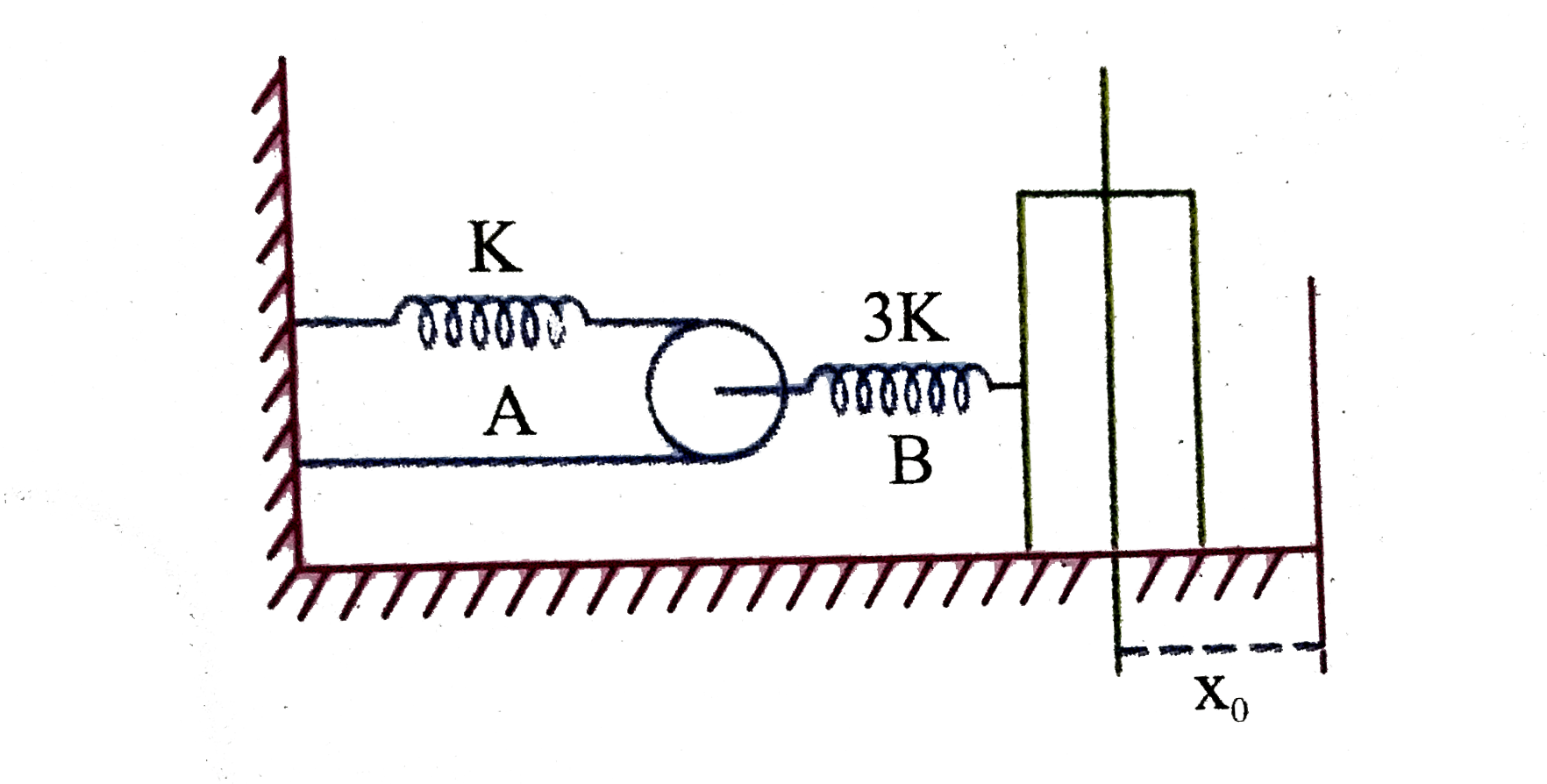 .
.
View Solution
SINGLE ANSWER QUESTIONS questions and complete solutions for chapter NEWTONS LAWS OF MOTION of NARAYANA PHYSICS (HINGLISH) of Class 11