Complete Solutions to EXERCISE - I(H.W) of chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | SYSTEM OF PARTICLES AND ROTATIONAL MOTION |
| NARAYANA | EXERCISE - I(H.W) |
| Number Of Questions | 63 |
| Academic Year | 2023-24 |
The distance of centre of mass from 'O ' is

View SolutionParticles of masses `1kg` and `3kg` are at `2i + 5j + 13k)m` and `(-6i + 4j - 2k)m` then instantaneous position of their centre of mass is
View SolutionThree identical spheres , each of mass 1 kg are kept as shown in figure given below , touching each other , with their centres on a straight line .If their centres are marked P,Q,R respectively , the distance of centre of mass of the system from P is :

View SolutionA particle of mass `m` is thrown horizontally from the top of a tower and anoher particle of mass `2m` is thrown vertically upward. The acceleration of centre of mass is
View SolutionTwo blocks of masses 5kg and 2kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse provides a velocity of 7m/s to the heavier block in the direction of the lighter block. The velocity of the centre of mass is :-
View SolutionTwo paricle A and B initially at rest, move towards each other under mutual force of attraction. At the instant when the speed of A is V and the speed of B is 2V, the speed of the centre of mass of the system is
View SolutionA man of mass `m` moves on a plank of mass `M` with a constant velocity `u` with respect to the plank, as shown in figure.
a.If the plank rests on smooth horizontal surface, determine the velocity of the plank.
b If the man travels a distance `L` with respect to the plank, find the distance travelled by the plank with respect to the ground.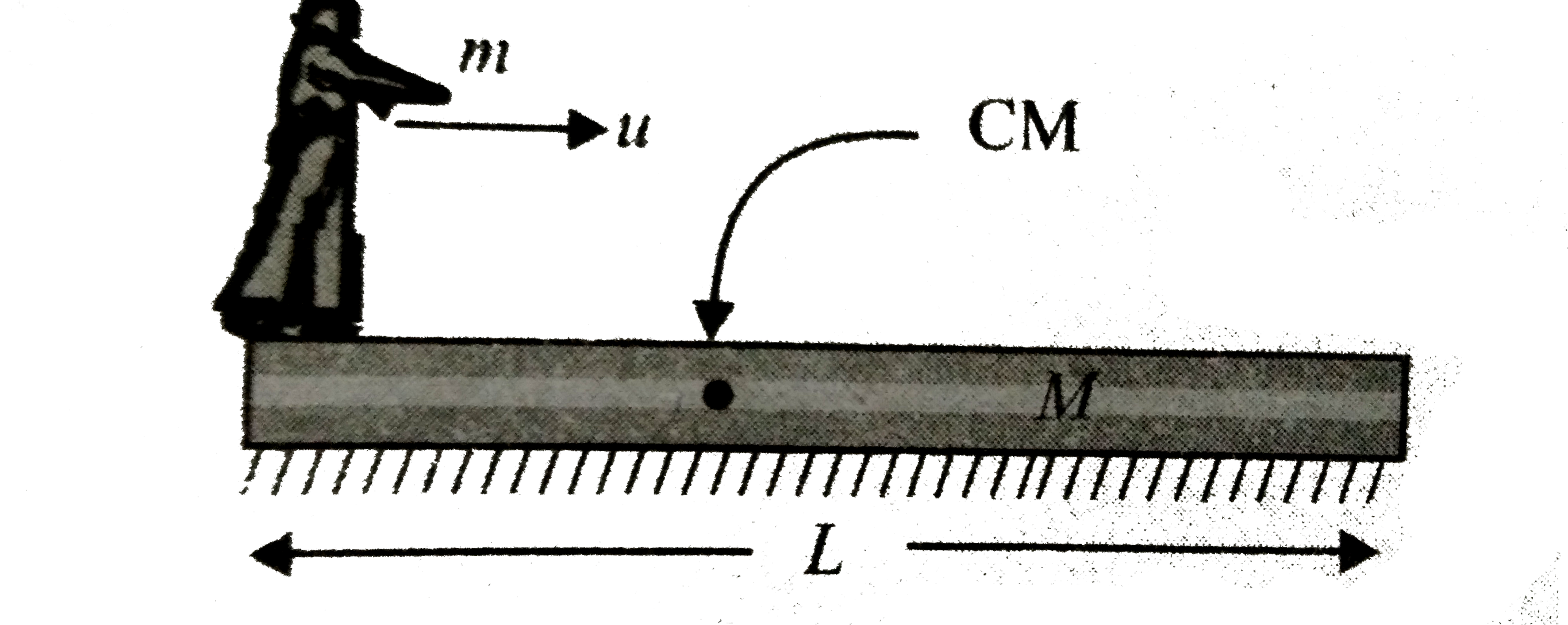
View SolutionTwo bodies of masses `5kg` and `3kg` are moving towards each other with `2ms^(-1)` and `4ms^(-1)` respectively. Then velocity of centre of mass is
View SolutionTwo particles of masses `4kg` and `6kg` are separated by a distance of `20cm` and are moving towards each other under mutual force of attraction, the position of the point where they meet is
View SolutionTwo objects of masses `200g` and `500g` have velocities of `10i m//s` and `(3i + 5j) m//s` respectively. The velocity of their centre of mass is
View SolutionThe unit vector perpendicular to `vec A = 2 hat i + 3 hat j + hat k` and ` vec B = hat i - hat j + hat k` is
View SolutionIf `vec A= 3i + j +2k` and `vec B = 2i - 2j +4k` and `theta` is the angle between the two vectors, then `sin theta` is equal to
View SolutionThe angular velocity of the seconds hand in a watch is
View SolutionThe angular displacement of a particle is given by `theta = t^3 + 2t +1`, where `t` is time in seconds. Its angular acceleration at `t=2s` is
View SolutionA particle is moving with uniform speed `0.5 m//s` along a circle of radius `1m` then the angular velocity of particle is (in `rad s^(-1)`)
View SolutionA wheel is making revolutions about its ais with unifrom angular acceleration. Starting from rest, ilt reaches 100 rev/sec in 4 seconds. Find the angular acceleration. Find the angle rotated during these four seconds.
View SolutionThe shaft of a motor car rotates at constant angular frequancy of 3000 revolutions //min .The angle through which it has turned in one second in radians is
View SolutionA particle P is moving in a circle of radius 'a' with a uniform speed V.C I the centre of the circle and AB is diameter . Then angular velocities of P about A and C in the ratio .

View SolutionInitial angular velocity of a wheel is ` 2 rad//s` .It rotates with a constant angular acceleration of `3.5 rad//s^(2)` .Its angular displacement in 2 s is
View SolutionA stationary wheel starts rotating about its own axis at constant angular acceleration. If the wheel completes `50` rotations in first `2` seconds, then the number of rotations mades by it in next two seconds is
View SolutionA disc of radius 0.1 m starts from rest with an angular acceleration of ` 4.4 rad//s^(2)` .Then linear velocity of the point on its after 5 s is
View SolutionShow that `overset rarr(a). (overset rarr(b) xx overset rarr(c ))` is equal in magnitude to the volume of the parallelopiped formed on the three vectiors, `overset rarr(a), overset rarr(b) and overset rarr(c )`.
View SolutionA force is applied on a door at point P making an angle `theta ` with position vector `bar(r )` , by partical observation , give the value of theta for which on has to exert minimum force to rotate the door ?
View SolutionA meter scale weighs 50 gms and carries 20 gm at one end , the scale balances when it is suspende at a distance x cm from other end . Then x I equal to
View SolutionA wire of mass `m` and length `l` is bent in the form of circular ring. The moment of inertia of the ring about its axis is
View SolutionThe radius of gyration of a body about an axis at a distance of `4cm` from its centre of mass is `5cm`. The radius of gyration about a parallel axis through centre of mass is
View SolutionIf `I` moment of inertia of a thin circular plate about an axis passing through tangent of plate in its plane. The moment of inertia of same circular plate about an axis perpendicular to its plane and passing through its centre is
View SolutionMoment of inertia of a hoop suspended from a peg about the peg is
View SolutionThe moment of inertia of a solid sphere about an axis passing through its centre is `0.8kgm^2`. The moment of inertia of another solid sphere whose mass is same as mass of first sphere, but the density is `8` times density of first sphere, about an axis passing through its centre is
View SolutionThe ratio of moments of inertia of solid sphere about axes passing through its centre and tangent respectively is
View SolutionThree identical masses, each of mass `1kg,` are placed at the corners of an equilateral triangle of side `l`. Then the moment of inertia of this system about an axis along one side of the triangle is
View SolutionThe radius of gyration of a body about an axis at a distance of `4cm` from its centre of mass is `5cm`. The radius of gyration about a parallel axis through centre of mass is
View SolutionThe diameter of a flywheel is increased by 1% keeping the mass same. Increase in its moment of inertia about the central axis is
View SolutionThe variation of moment of inertia I of a solid sphere of constant mass with radius R is given by
View SolutionFour particles each of mass `m` are placed at the corners of a square of side length `l`. The radius of gyration of the system about an axis perpendicular to the plane of square and passing through its centre is
View SolutionIn the above problem the moment of inertia of four bodies about an axis perpendicular to the plane of frame and passing through a corner is
View SolutionIn the above problem the moment of inertia of four bodies about an axis perpendicular to the plane of frame and passing through a corner is
View SolutionIn the above problem the moment of inertia of four bodies about an axis passing through any side of frame is
View SolutionMoment of inertia of a solid sphere about its diameter is `I_(0)` . Then moment of inertia about an axis parallel to its diameter at a distance equal to half of its radius is
View SolutionA uniform rod of mass m is bent into the form of a semicircle of radius R. The moment of inertia of the rod about an axis passing through A and perpendicular to the plane of the paper is

View SolutionA disc of moment of inertia `2 kg m^(2)` is acted upon by a constant torque of 40 Nm. If it is initially at rest, then the time taken by it to acquire an angular velocity 100 rad/s will be
View SolutionA circular disc of mass `4kg` and of radius `10cm` is rotating about its natural axis at the rate of `5 rad//sec`. Its angular momentum is
View Solution`vecF = a hat i + 3hat j+ 6 hat k` and `vec r = 2hat i-6hat j -12 hat k`. The value of `a` for which the angular momentum is conserved is
View SolutionA particle of mass `m` is rotating in a plane in circular path of radius `r`. Its angular momentum is `L`. The centripetal force acting on the particle is
View SolutionA mass is whirled in a circular path with a constant angular velocity and its angular momentum is L. If the string is now halved keeping the angular velocity same, the angular momentum is
View SolutionIf a uniform solid sphere of diameter 0.2 m and mass 10 kg is rotated about its diameter with an angular velocity of 2 rad/s, then the its angular momentum in kg `m^(2)//s` will be
View SolutionA child is standing with folded hands at the center of a platform rotating about its central axis. The kinetic energy of the system is `K`. The child now stretches his arms so that the moment of inertia of the system doubles. The kinetic energy of the system now is
View SolutionA constant torque acting on a uniform circular disc changes its angular mkomentum from L to 4L/3 in 2 seconds. Then the magnitude of the torque applied is
View SolutionIf the mass of earth and radius suddenly become `2` times and `1//4th` of the present value, the length of the day becomes
View SolutionA uniform circular disc of radius `R` is rotating about its own axis with moment of inertia `I` at an angular velocity `omega` if a denser particle of mass `m` is gently attached to the rim of disc than its angular velocity is
View SolutionA ballet dancer spins about a vertical axis at 60 rpm with his arms closed. Now he stretches his arms such that M.I. Increases by `50%`. The new speed of revolution is
View SolutionA metallic circular wheel is rotating about its own axis without friction. If the radius of wheel expands by `0.2%`, percentage change in its angular velocity
View SolutionA wheel at rest has M.I. `2/(pi^2) kgm^2`. It is rotated by a `60W` motor for one minute. The number of rotations made by the wheel in one minute is
View SolutionThe shaft of a motor is making 1260rpm. The torque supplied by the motor is `100Nm`. The power of motor is (in `KW`)
View SolutionA thin ring of mass `1kg` and radius `1m` is rolling at a speed of `1ms^(-1)`. Its kinetic energy is
View SolutionA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
View SolutionIf a sphere of mass `2kg` and diameter `10cm` is rolling at speed of `5ms^(-1).` Its rotational kinetic energy is
View SolutionA solid sphere and a solid cylinder having the same mass and radius, rolls down the same incline. The ratio of their acceleration will be
View SolutionA solid cylinder of mass `M` and radius `R` rolls down an inclined plane of height `h` without slipping. The speed of its centre when it reaches the bottom is.
View SolutionWhen a solid sphere is rolling along level surface the percentage of its total kinetic energy that is translational is
View SolutionThe speed of a uniform solid cylinder after rolling down an inclined plane of vertical height H, from rest without sliding is :-
View SolutionA ring is allowed to roll down on an incline of `1` in `10` without slipping. The acceleration of its centre of mass is
View SolutionA solid sphere and a spherical shell roll down an incline from rest from same height. The ratio of times taken by them is
View Solution
EXERCISE - I(H.W) questions and complete solutions for chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of NARAYANA PHYSICS (HINGLISH) of Class 11