Complete Solutions to EXERCISE - III of chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | SYSTEM OF PARTICLES AND ROTATIONAL MOTION |
| NARAYANA | EXERCISE - III |
| Number Of Questions | 43 |
| Academic Year | 2023-24 |
The moment of inertia of a uniform circular disc of radius `R` and mass `M` about an axis passing from the edge of the disc and normal to the disc is.
View SolutionA uniform rod length I and mass m is free to rotate in a vertical plane about A . The rod initially in horizontal position and it is released . The intial angular acceleration of the rod is ( Moment of inertia of rod about A is `(ml^(2))/3`)

View SolutionThe radius of a pulley is 20 cm and its moment of inertia is `0.32 kg - m^(2)` . A block of mass 2 kg is suspended from the pulley with the help of massless string as shown in the figure . If the block is released from rest . Then determine the acceleration of the block .

View SolutionA wheel has angular acceleration of `3.0 rad//s^2` and an initial angular speed of `2.00 rad//s`. In a tine of `2 s` it has rotated through an angle (in radian) of
View SolutionA uniform rod AB of length I and mass m is free to rotate about pont A . The rod is released from rest in the horizontal position . Given that the moment of inertia of the rod about A is `(ml^(2))/3 ` , the intial angular acceleration of the rod will be

View SolutionA particle of mass 'm ' moves in the XY plane with a velocity 'v' along the straight line AB. If the angular momentum of the particle with respect to origin O is `L_(A)` when it is A and `L_(B)` when it is at B then :

View SolutionThe ratio of the radii of gyration of a circular disc to that of a circular ring, each of same mass and radius, around their respective axes is.
View SolutionA thin rod of length `L` and mass `M` is bent at its midpoint into two halves so that the angle between them is `90^@`. The moment of inertia of the bent rod about an axis passing through the bending point and perpendicular to the plane defined by the two halves of the rod is.
View SolutionThree identical rings of mass m and radius r are placed as shown in figure . Find moment of inertia of the system about asis XX ?

View SolutionA disc is rotating with angular velocity `(omega )` about its axis (Without any translation push ) on a smooth surface . Find the direction and magnitude of velocity at points A and B .

View SolutionA thin circular ring of mass `M` and radius `R` is rotating in a horizontal plane about an axis vertical to its plane with a constant angular velocity `omega`. If two objects each of mass `m` be attached gently to the opposite ends of a diameter of the ring, the ring will then rotate with an angular velocity
View SolutionTwo blocks of mass `1kg` and `3 kg` have position v ectors ` hat(i) + 2 hat(j) + hat(k)` and `3 hat(i) - 2 hat(j) + hat(k)` , respectively . The center of mass of this system has a position vector.
View SolutionFrom a circular disc of radius R and 9M , a small disc of mass M and radius `(R )/(3)` is removed concentrically .The moment of inertia of the remaining disc about and axis perpendicular to the plane of the disc and passing through its centre is
View SolutionA solid cylinder and a hollow cylinder, both of the same mass and same external diameter are released from the same height at the same time on an inclined plane. Both roll down without slipping. Which one will reach the bottom first ?
View Solution(1) Centre of gravity (C.G.) of a body is the point at which the weight of the body acts,
(2) Centre of mass coincides with the centre of gravity if the earth is assumed to have infinitely large radius,
(3) To evaluate the gravitational field intensity due to any body at an external point, the entire mass of the body can be cosidered to be concentrated at its C.G..,
(4) The radius of gyration of any body rotating about ab axis is the length of the perpendicular dropped from thr C.G. the body to the axis. which one of the following paries of statements is correct ?
View SolutionA thin circular ring of mass M and radius r is rotating about its axis with a constant angular velocity `omega`, Two objects, each of mass m, are attached gently to the opposite ends of a diameter of the ring. The wheel now rotates with an angular velocity `omega=`
View SolutionA circular disc of moment of inertia `I_(t)` is rotating in a horizontal plane about its symmetry axis with a constant angular velocity `omega_(i)`. Another disc of moment of inertia `I_(b)` is dropped co-axially onto the rotating disc. Initially, the second disc has zero angular speed. Eventually, both the discs rotate with a constant angular speed `omega_(f)`. Calculate the energy lost by the initially rotating disc due to friction.
View SolutionTwo particle which are initially at rest move towards each other under the action of their internal attraction. If their speeds are `v` and `2 v` at any instant, then the speed of centre of mass of the system will be
View SolutionThe instantaneous angular position of a point on a rotating wheel is given by the equation
`theta(t) = 2t^(3) - 6 t^(2)`
The torque on the wheel becomes zero at
View SolutionThe moment of inertia of a thin uniform rod of mass `M` and length `L` about an axis passing through its mid-point and perpendicular to its length is`I_0`. Its moment of inertia about an axis passing through one of its ends perpendicular to its length is.
View SolutionA small mass attached to a string rotates on frictionless table top as shown . If the tension is the string is increase by pulling the string causing the radius of the circular motion to decrease by a factor of 2 , the kinetic energy of the mass will

View SolutionA circular platform is mounted on a frictionless vertical axle. Its radius `R = 2m` and its moment of inertia about the axle is `200 kg m^(2)`. It is initially at rest. A `50 kg` man stands on the edge at the platform and begins to walk along the edge at the speed of `1ms^(-1)` relative to the ground. Time taken by the man to complete one revolution is :
View SolutionThe moment of inertia of a uniform circular disc is maximum about an axis perpendicular to the sisc and passing through

View SolutionThree masses are placed on the x-axis : `300 g` at origin. `500 g` at `x = 40 cm` and `400 g` at `x = 70 cm`. The distance of the centre of mass from the origin is.
View SolutionWhen a mass is rotating in a plane about a fixed point, its angular momentum is directed along.
View SolutionTwo persons of masses `55 kg` and `65 kg` respectively are at the opposite ends of a boat. The length of the boat is `3.0 m` and weights `100 kg`. The `55 kg` man walks up to the `65 kg` man and sits with him. If the boat is in still water the centre of mass of the system shifts by.
View SolutionABC is an equilateral triangle with O as its centre , `bar(F)_(1),bar(F)_(2) and bar(F)_(3)` represent three forces acting along the sides AB, BC and AC respectively . If the total torque about O is zero then the magnitude of `bar_(F)_(3)` is

View SolutionA rod PQ of mass M and length L is hinged at end P . The rod is kept horizontal by a massless string tied to pont Q as shown in figure . When string is cut , the intial angular acceleration of the rod is

View Solution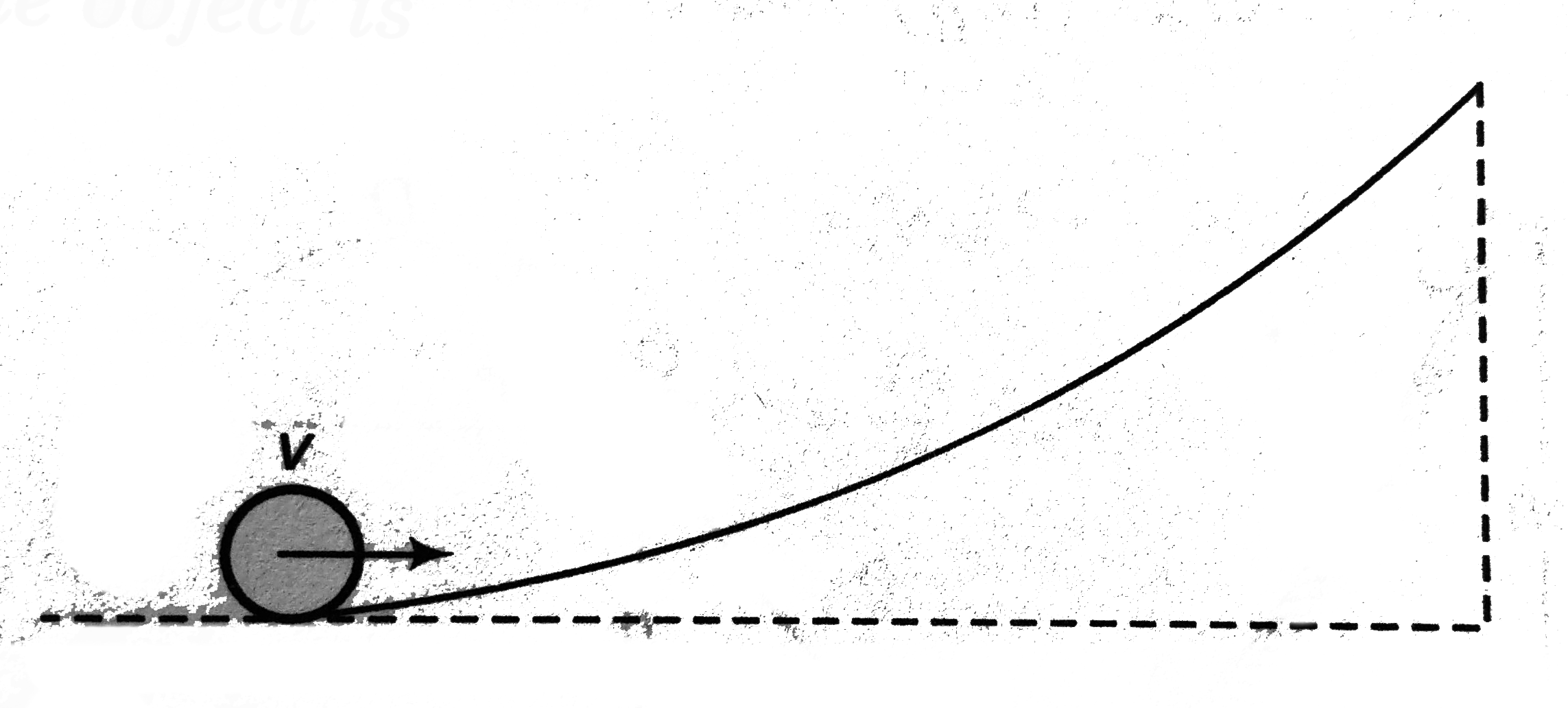
A small object of uniform density rolls up a curved surface with an initial velocity `v`. It reaches up to a maximum height of `(3v^(2))/(4g)` with respect to the initial position. The object is
(a). Ring
(b). solid sphere
(c). hollow sphere
(d). disc
View SolutionA solid cylinder of mass `50 kg` and radius `0.5 m` is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other end hanging freely. Tension in the string required to produce an angular acceleration of `2` revolution `s^(-2)` is
View SolutionThe ratio of the accelerations for a solid sphere (mass `m, and radius R`) rolling down an incline of angle `theta` without slipping, and slipping down the incline without rolling is
View SolutionPoint masses `m_(1)` and `m_(2)` are placed at the opposite ends of a rigid rod of a length L and negligible mass . The rod is to be set rotating about an axis perpendicualr to it . The position of point P on this rod through which the axis should pass, so that the work required to set the rod rotating with angular velocity `omega_(0)` is minimum , is given by

View SolutionA force `vec F = prop hat i + 3 hat j + 6 hat k` is acting at a point `vec r = 2 hat i - 6 hat j - 12 hat k`. The value of `prop` for which angular momentum about origin is conserved is.
View SolutionA rod of weight `w` is supported by two parallel knife edges `A` and `B` and is in equilibrium in a horizontal position. The knives are at a distance `d` from each other. The centre of mass of the rod is at a distance `x` from `A`.
View SolutionA mass m moves in a circle on a smooth horizontal plane with velocity `v_(0)` at a radius `R_(0)` . The mass is attached to a string which passes through a smooth hole in the plane as shown .

The tension in the string increased gradually and finally m moves in a circle of radius `(R_(0))/2` . The final value of the kinetic energy is
View SolutionThree identical spherical shells , each of mass m and radius r are placed as hsown in figure . Consider an axis `XX^(-1)` , which is touching to two shells and passing through diameter of third shell . Moment of inertia of the system consisting of these spherical shells about `XX^(1)` axis is

View SolutionFrom a disc of radius R and mass m, a circular hole of diamter R, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc about a perpendicular axis, passing through the centre?
View SolutionA uniform circular disc of radius 50 cm at rest is free to turn about an axis which is perpendicular to its plane and passes through its centre. It is subjected to a torque which produces a constant angular acceleration of 2 rad `s^(-1)`. Its net acceleration in `ms^(-2)` at the end of 2 s is approximately
View SolutionA disc and a solid sphere of same radius but different masses roll off on two inclined planes of the same altitude and length. Which one of the two objects gets to the bottom of the plane first ?
View SolutionIn the given figure , `a = 15 m//s^(2)` represents the total acceleration of a particle moving in the clockwise drection in a circle of radius R = 2.5 m at a given instant of time . The speed of the particle is

View SolutionTwo rotating bodies `A` and `B` of masses `m` and `2m` with moments of inertia `I_(A)` and `I_(B) (I_(B) gt I_(A))` have equal kinetic energy of rotation. If `L_(A)` and `L_(B)` be their angular momenta respectively, then
View SolutionA solid sphere of mass `m` and radius `R` is rotating about its diameter. A solid cylinder of the same mass and same radius is also rotating about its geometrical axis with an angular speed twice that of the sphere. The ratio of their kinetic emergies of rotation `(E_("sphere")//E_("cylinder"))` will be.
View SolutionA light rod of length `l` has two masses `m_1` and `m_2` attached to its two ends. The moment of inertia of the system about an axis perpendicular to the rod and passing through the centre of mass is.
View Solution
EXERCISE - III questions and complete solutions for chapter SYSTEM OF PARTICLES AND ROTATIONAL MOTION of NARAYANA PHYSICS (HINGLISH) of Class 11