Complete Solutions to Exercise of chapter ELECTROSTATICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | ELECTROSTATICS |
| RESONANCE | Exercise |
| Number Of Questions | 52 |
| Academic Year | 2023-24 |
On moving a charge of 20 C by 2 cm, 2 J of work is done, then the potential differ rence between the point is :
View SolutionA charged particle q is placed at the centre O of cube of length L(ABCDEFGH). Another same charge q is placed at a distance L from O.Then, the electric flux through ABCD is :
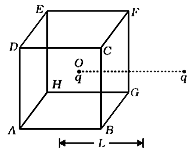
View SolutionIf the electric flux entering and leaving an enclosed surface respectively, is `phi_(1)` and `phi_(2)`, then the electric charge inside the surface will be
View SolutionThe number of electrons to be put on a spherical conductor of radius `0.1m` to produce an electric field of `0.036N//C` just above its surface is
View SolutionA charged particle is shot with speed V towards another ifxed charged particle Q .It approaches Q up to a closest distnce r and then returns.If q were given a speed 2V, the closest distance of approach would be
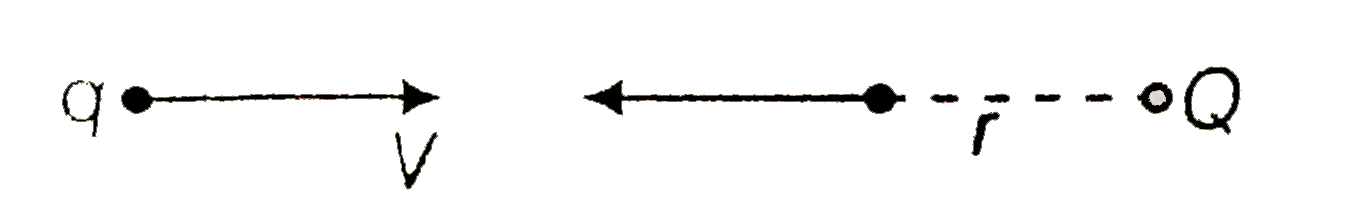
View SolutionA point charge +Q is placed at the centroid of an equilateral triangle. When a second charge +Q is placed at a vertex of the triangle, the magnitude of the electrostatic force on the central charge is 8 N. The magnitude of the net force on the central charge when a third charge +Q is placed at another vertex of the triangle is
View SolutionFour positive charges `(2sqrt2-1)Q` are arranged at the four corners of a square. Another charge q is placed at the centre of the square. Resulting force acting on each corner charge is zero if q is
View SolutionPoint charges `+4q, -q` and `+4q` are kept on the x-axis at points `x=0,x=a` and `x=2a` respectively, then
View SolutionA simple pendulum of mass m and charge +q is suspended vertically by a massless thread of length l. At the point of suspension, a point charge +q is also fixed. If the pendulum is displaced slightly from equilibrium position, its time period will be
View SolutionThe diagram shows the arrangement of three small unifromly charged spheres A, B and C. The arrows indicate the direction of the electrostatic force acting between the spheres (for example, the left arrow on sphere A indicates the electrostatic force on shphere A due to sphere B). At least two of the spheres are positively charged. Which sphere, if any, could be negatively charged?
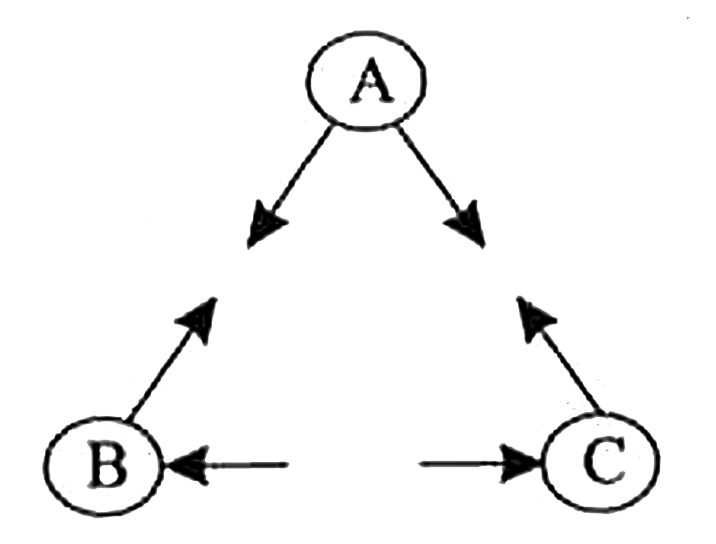
View SolutionFigure, shown above, shows three situations involving a charged particle and a uniformly charged spherical shell. The charges and radii of the shells are indicated in the figure. If `F_(1),F_(2)` and `F_(3)` are the magnitudes of the force on the particle due to the shell in situations (I),(II) and (III) then
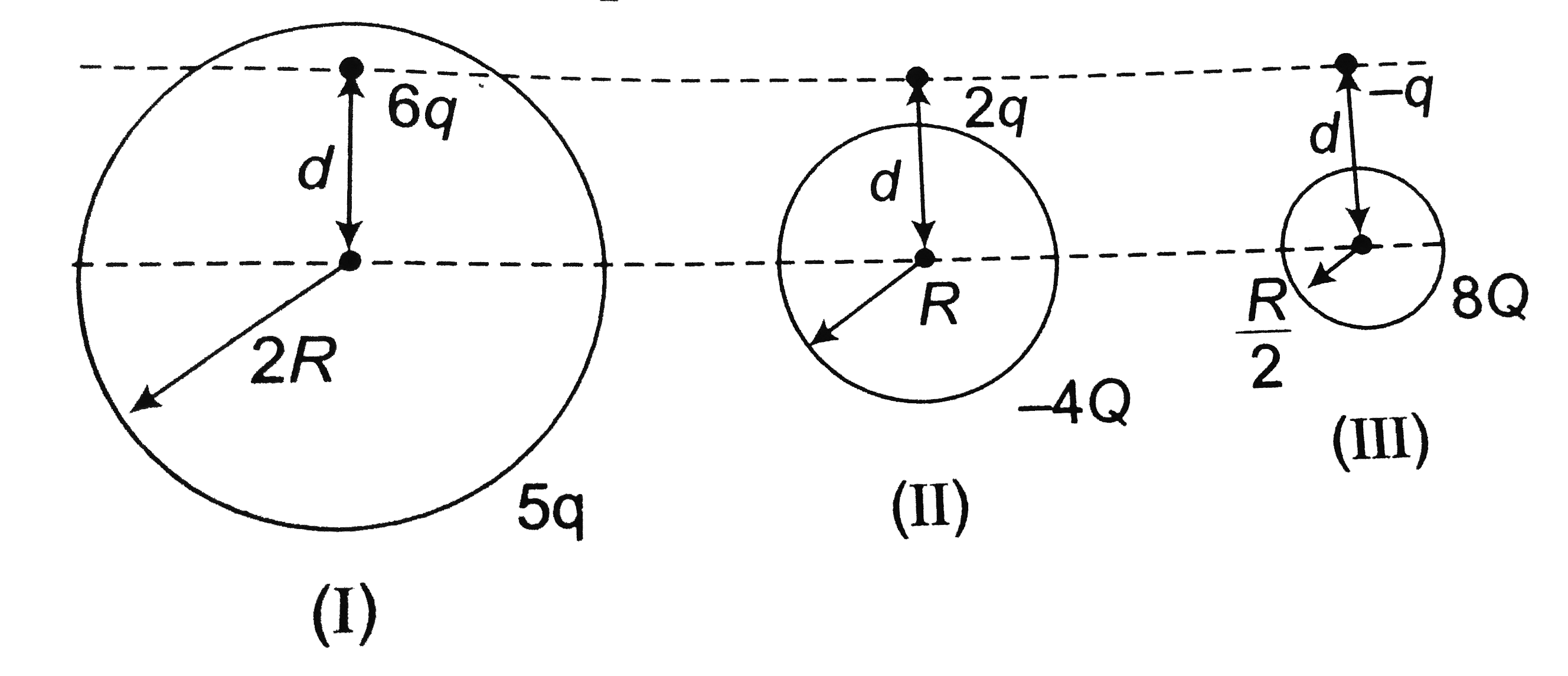
View SolutionThere are three concentric thin spheres of radius `a,b,c (agtbgtc)`. The total surface charge densities on their surfaces are `sigma,-sigma,sigma` respectively. The magnitude of the electric field at `r` (distance from centre) such that `agtrgtb` is:
A. 0
B. `(sigma)/(epsilon_(0) r^(2))(b^(2)-c^(2))`
C. `(sigma)/(epsilon_(0)r)(a^(2)+b^(2))`
D. none of these
View SolutionA particle of charge `-q` and mass m moves in a circle of radius r around an infinitely long line charge of linear charge density `+lamda`. Then, time period will be
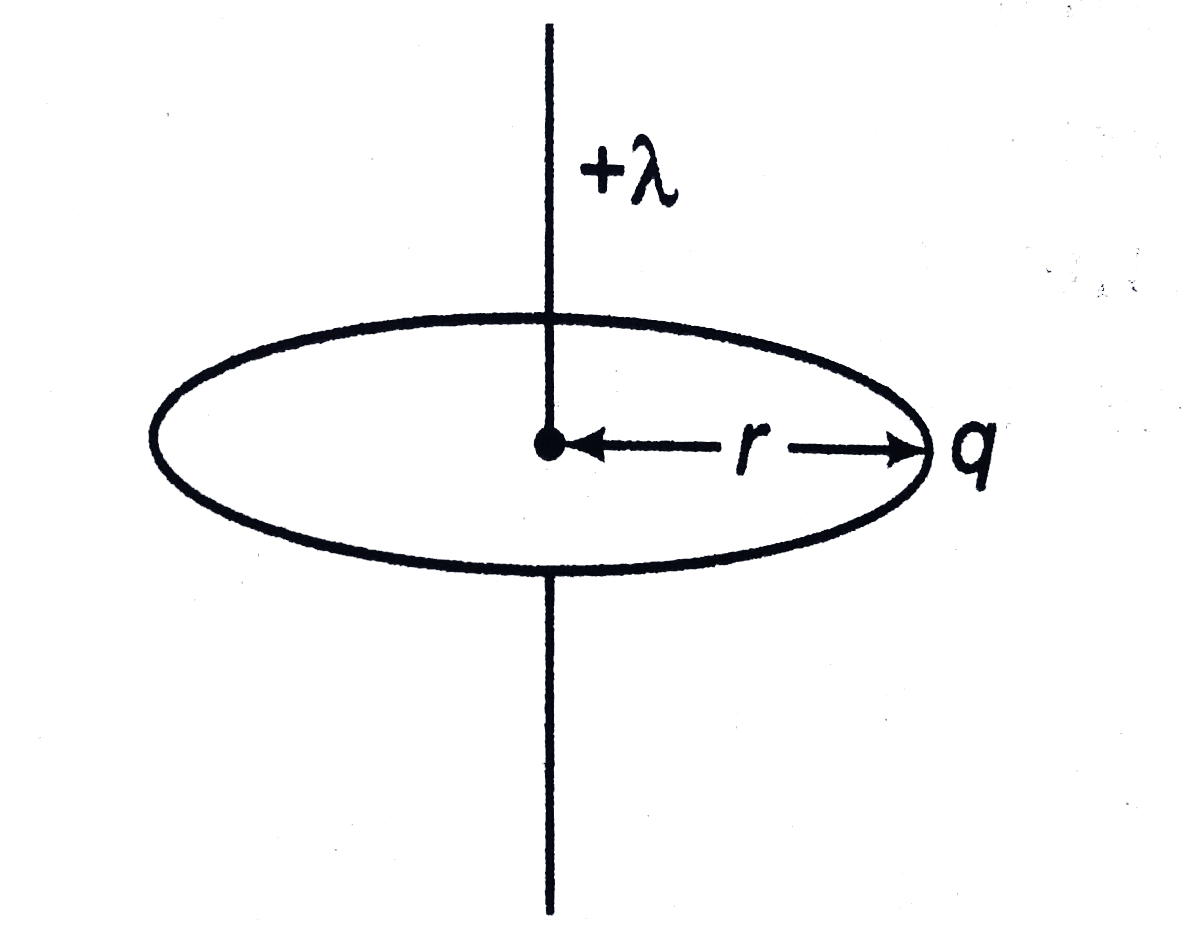 where , `k=1/4piepsilon_0)`
where , `k=1/4piepsilon_0)`
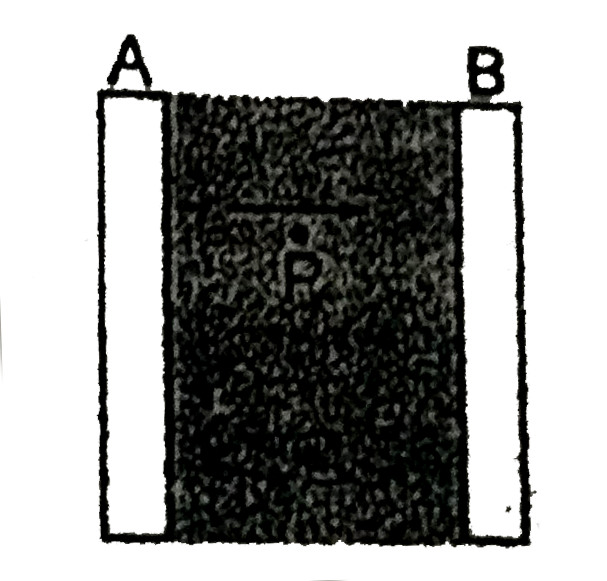
View SolutionThe figure shown represents the electric field between two large metal plates. Which of the graphs given in figure represents the force on a positve charge as it moves from point P towards plate B?
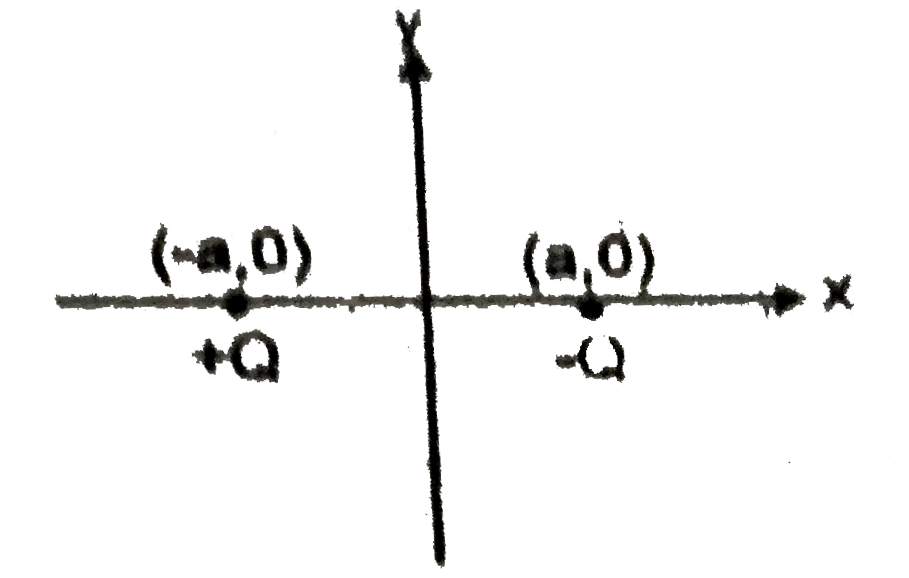
View SolutionThe variation of electric field on the y-axis as a function of 'y' is best represented by [for the given figure]:
A.
B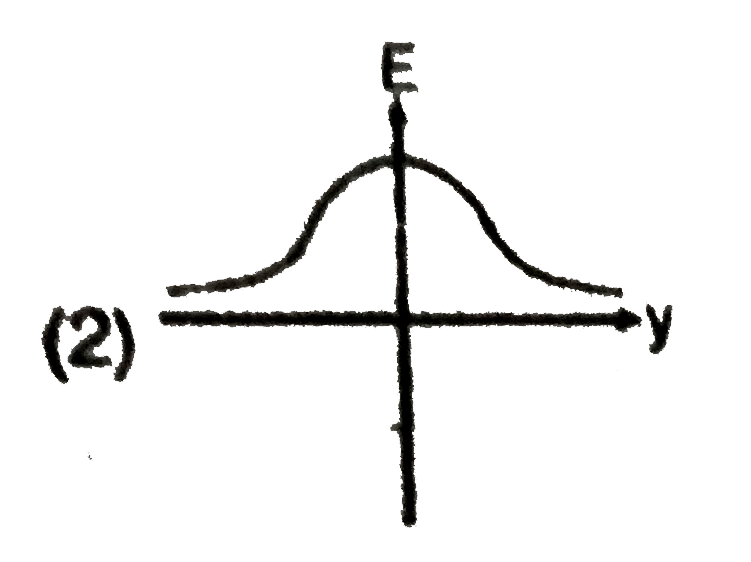
C.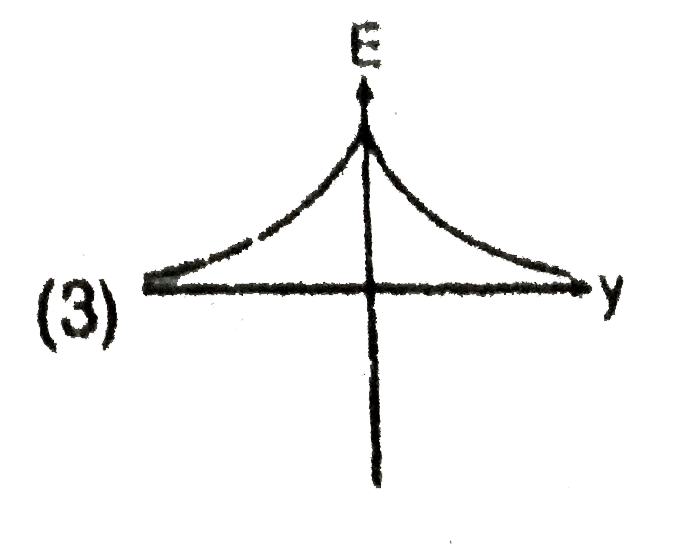
D.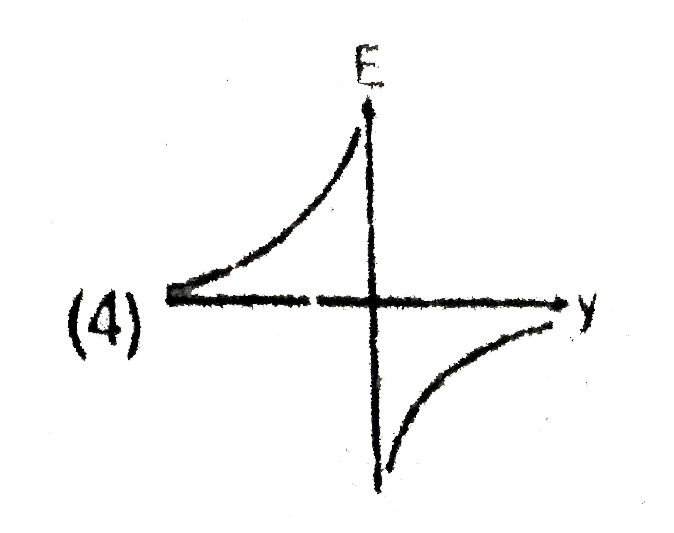
View SolutionA ring carries a uniform linear charge density on one half and the linear charge density of same magnitude but opposite sign on the other half.
View SolutionTwo very large thin conducting plates having same cross sectional area are placed as shown in figure. They are carrying chaerges `Q` and `3Q`, respectivley. The variation of electric field as as function at x(for `x=0` to `x=3d`) will be best represented as by
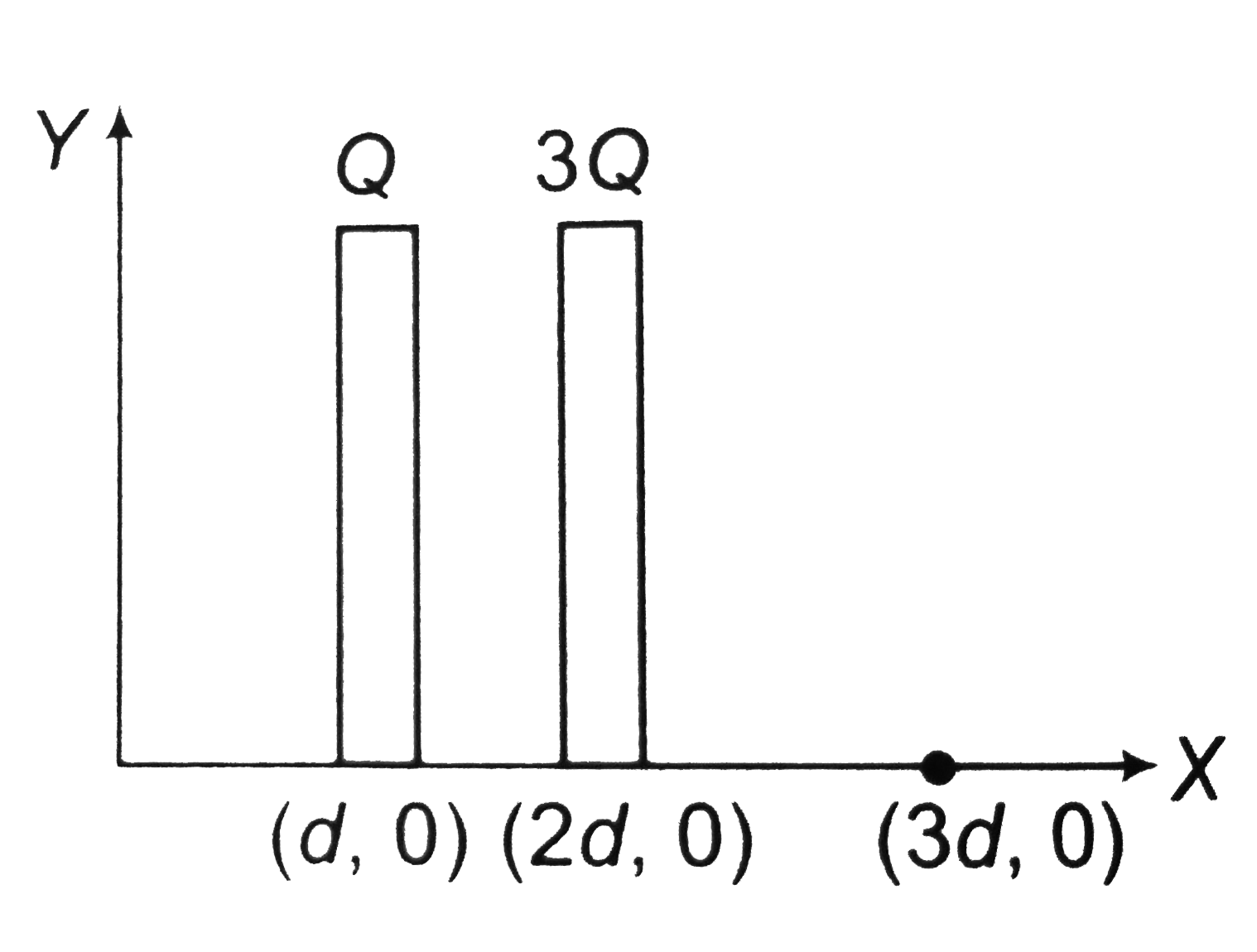
A.
B.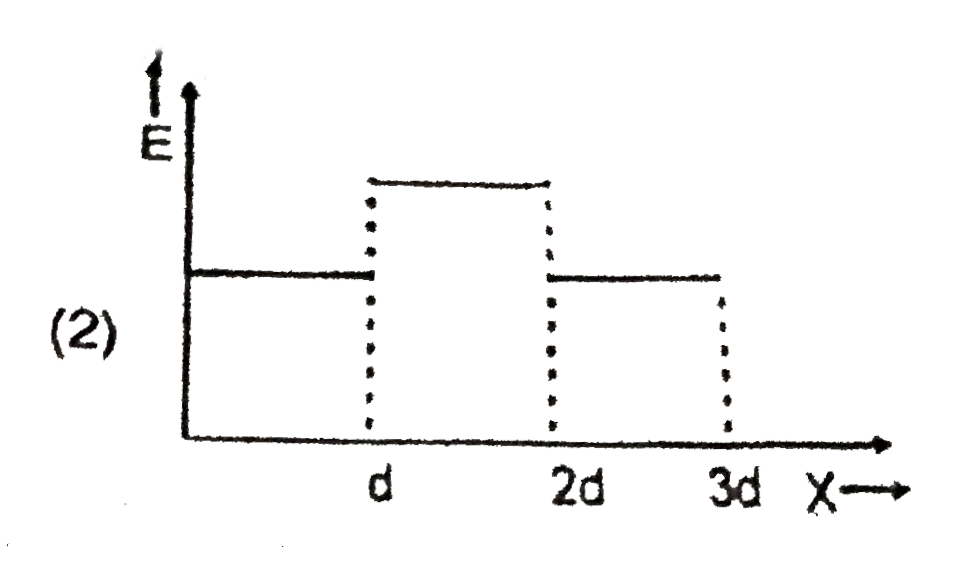
C.
D.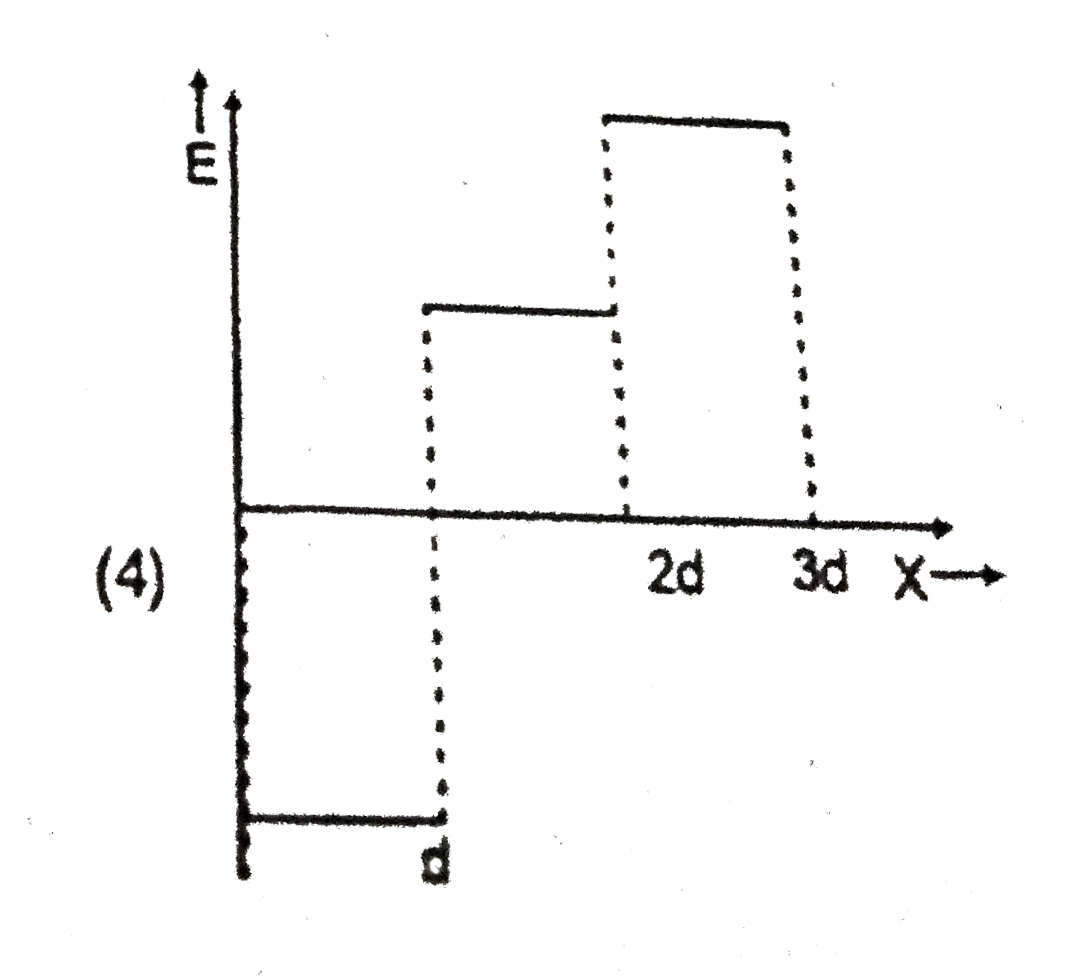
View SolutionTwo semicircular rings lying in same plane, of uniform linear charge density `lambda` have radius r and 2r. They are joined using two straight uniformly charged wires of linear charge density `lambda` and length r as shown in figure. The magnitude of electric field at common centre of semi circular rings is -

View SolutionA nonuniformly charged ring is kept near an uncharged conducting solid sphere. The distance between their centres (which are on the same line normal to the plane of the ring) is 3m and their radius is 4m. If total charge on the ring is `1muC`, then the potential of the sphere will be
View SolutionA mercury drop of water has potential 'V' on its surface. 1000 such drops combine to form a new drop. Find the potential on the surface of the new drop.
View SolutionA point charge of 5C is placed at point P (as shown in figure). A unit positive charge is taken from A to B along the circular path shown, then the net work done by electrostatic force is : [O is the centre of the circular path] [Where `k=9xx10^9 Nm^2//C^2`]
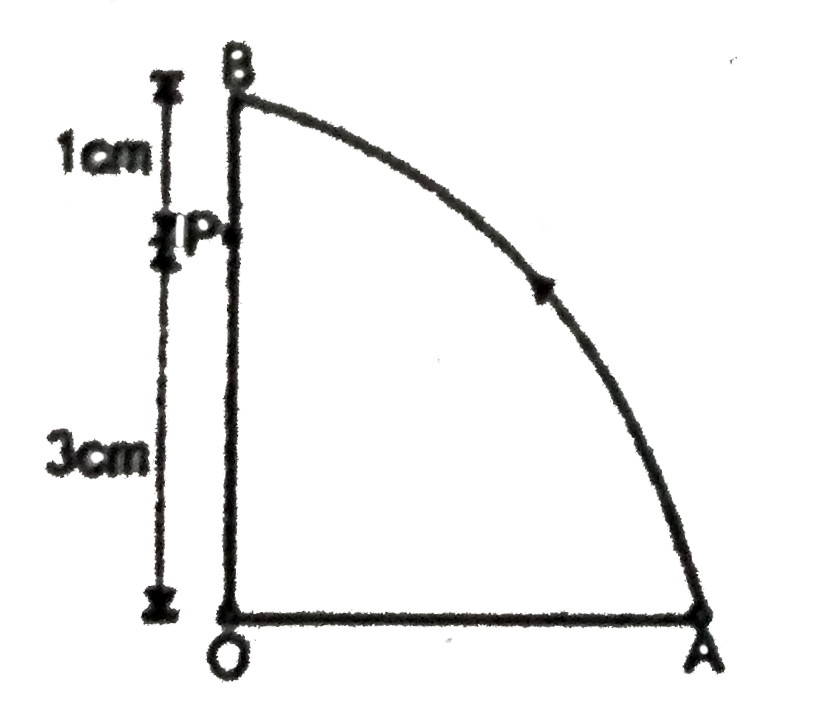
View SolutionAn electron is revolving around a proton. The total work done in one revolution by electric force on the electron will be zero if the trajectory of the electron is
View SolutionThree equal charges `Q` are placed at the three vertices of an equilateral triangle. What should be the va,ue of a charge, that when placed at the centroid, reduces the interaction energy of the system to zero ?
View SolutionTotal electric force on an electric dipole placed in an electric field of a point charge is :
View SolutionElectric potential due to a dipole at a position `vec r` from its centre is: where `(K = 1/(4pi epsilon_0)`
View SolutionThe magnitude of electric field intensity at point `B (2 , 0, 0)` due to a dipole of dipole moment `vec(P) = hati + sqrt(3)hatj` kept at origin is (assum that the point B is at a large distance from the dipole end `K = 1/(4 pi epsilon_0)`)
View SolutionConsider the four field patterns shown. Assuming there are no charges in the regions shown, which of the patterns represents a possible electrostatic field:
View SolutionIf the net electric field flux passing through a closed surface is zero, then the electric field at the surface will be
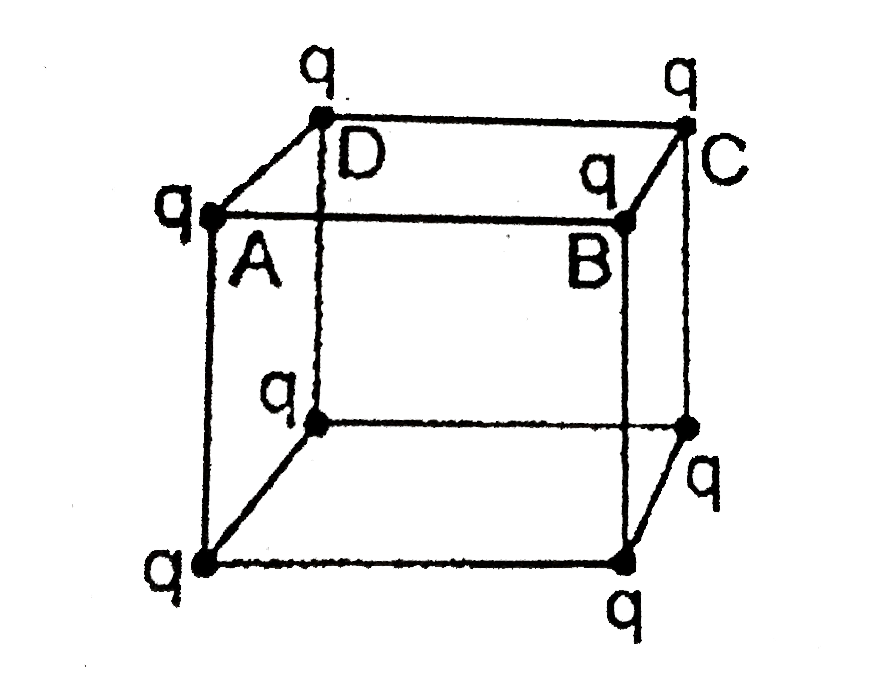
View SolutionEight point charges (can be assumed as small spheres uniformly charged and their centres at the corner of the cube) having values q each are fixed at vertices of a cube. The electric flux through square surface ABCD of the cube is
View SolutionFigure above shows a closed Gaussian surface in the shape of a cube of edge length 3.0 m. There exists an electric field given by `vec(E) = [(2.0xx +4.0)i+ 8.0 j+ 3.0 k]N//C`, where x is in metres in the region is which it lies. The net charge in coulombs enclosed by the cube is equal to

View SolutionElectrical potential `V` in space as a function of co-ordinates is given by `,V=(1)/(x)+(1)/(y)+(1)/(z)`
then the electric field intensity at `(1,1,1)` is given by:
View Solution`S_1`: In a metallic body total number of electrons is very large in comparison with non metallic solid body of same mass.
`S_2`: An uncharged conductor kept near a charged body is attracted by that body whether the charge on the body is positve or negative.
`S_3`: The electric field produced by an infinitely large sheet is same on both sides.
View Solution`S_1`: When a positively charged particle is released in an electric field , in its subsequent motion,it may or may not move along the electric field line passing through the point it has been released.
`S_2`: In electrostatic, conductors are always equipotential surfaces.
`S_3`: The flux through a closed Gaussian surface is non-zero. The electric field at some point on the Gaussian surface may be zero.
View SolutionA point charge `q` is located at the centre fo a thin ring of radius `R` with uniformly distributed charge `-q`, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance `x` from its centre, if `x gt gt R`.
View SolutionTwo small balls having equal positive charge Q (coulumb) on each suspended by two insulating strings of equal length L (metre) from a hook fixed to a stand. The whole set-up is taken in a satellite into space where there is no gravity (state of weightlessness). Then tension (newtons) in each string is:
View SolutionA point charge q moves from point P to pont S along the path PQRS (fig.) in a uniform electric field E pointing parallel to the poistive direction of the X-axis. The coordinates of the points P, Q, R and S are(a, b, O), (2a, O, O)(a, -b,`ulO`) and `(O, O, O) respectively. The work done by the field in the above process is given by the expresison............
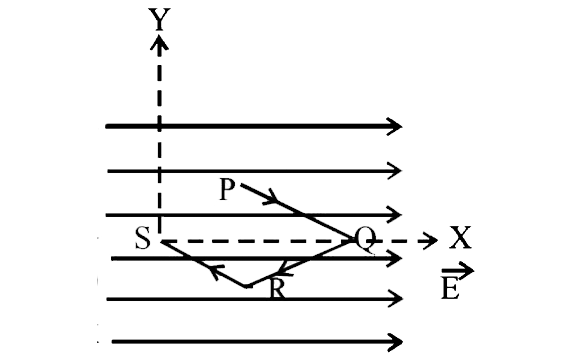
View SolutionA large nonconducting sheet M is given a uniform charge density. Two unchared small metal rods A and B are placed near the sheet as shown in figure

View SolutionAt a distance of` 5cm` and `10 cm` from surface of a uniformly charge solid sphere, te potentials are `100V` and `75V` respectivley. Then
View SolutionAn electric dipole is kept in the electric field produced by a point charge
View SolutionThe electric potential decreases uniformly from 180V to 20V as one moves on the X-axis from `x=-2cm ` to `x= +2cm`. The electric field at the origin:
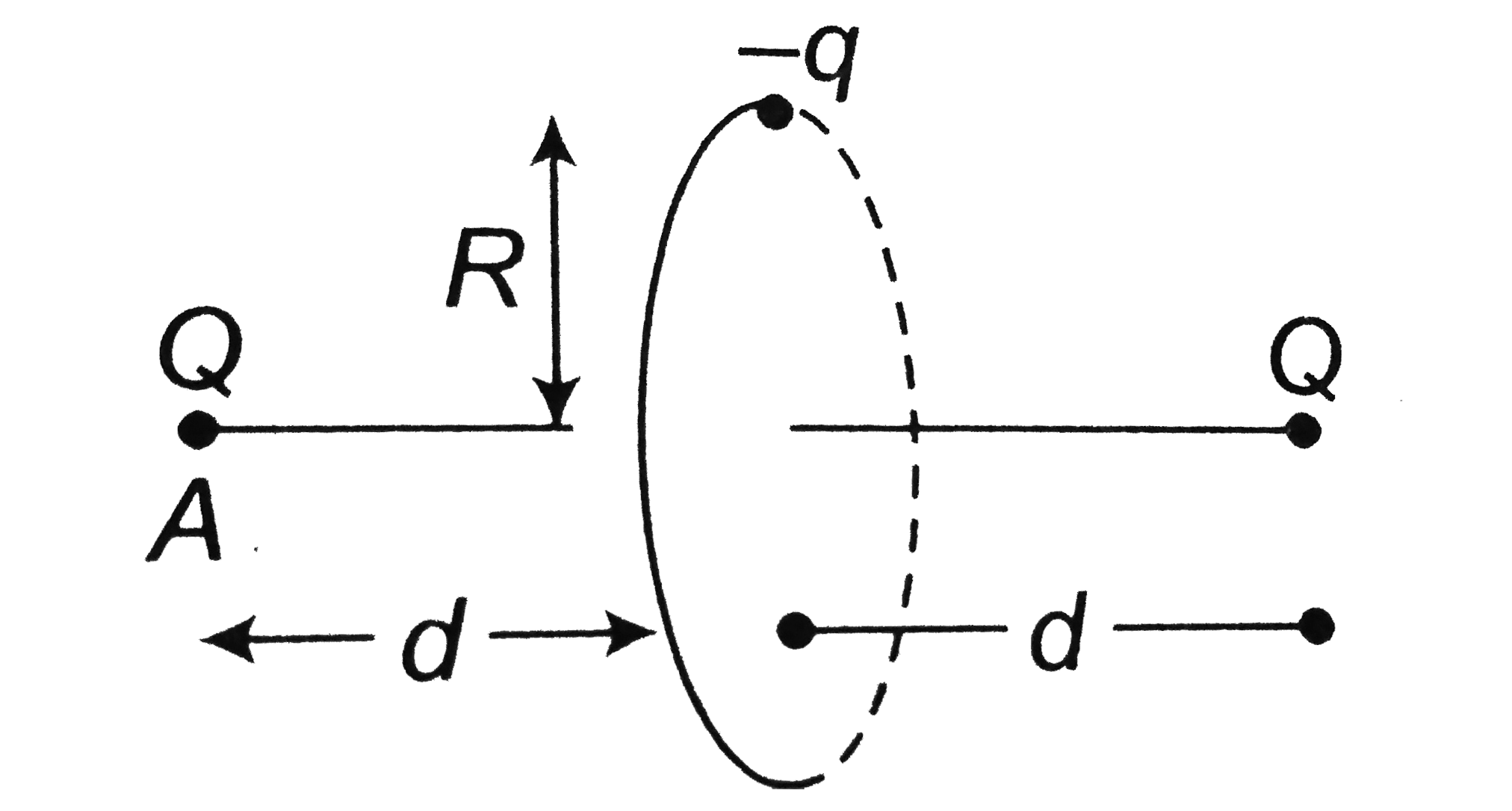
View SolutionTwo positive point charges each of magnitude `10 C` are fixed at positions `A` &`B` at a seperation `2d= 6m`. A negatively charged particle of mass `m= 90gm` and charge of magnitude `10xx10^(-6)C` is revolving in a circular path of radius `4m` in the plane perpendicular to the line `AB` and bisecting the line `AB`. Neglect the effect of gravity. Find the angular velocity of the particle
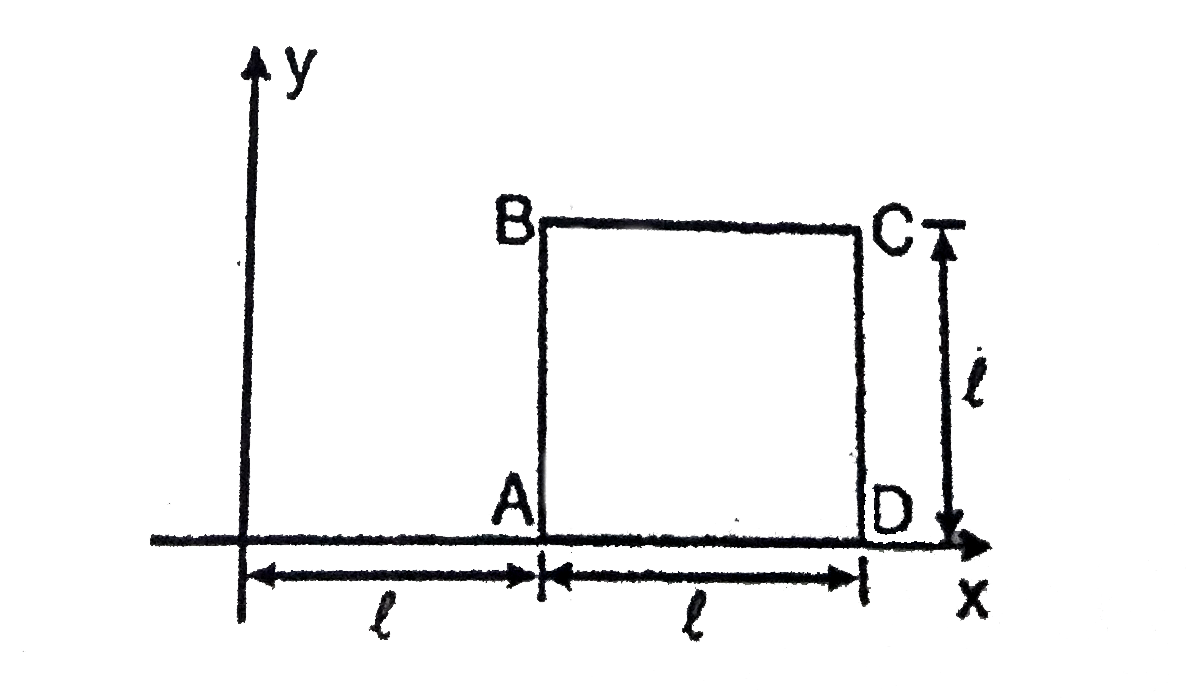
View SolutionA square loop of side 'l' having uniform linear charge density '`lambda`' is placed in 'xy' plane as shown in the figure. There is a non uniform electric field `vec E= a/l (x+l) hat i` where a is a constant. Then resultant electric force on the loop if `l=10cm, a=2N//C` and charge density `lambda = 2muC//m` is:
View SolutionIn the following figure an isolated charged conductor is shown. The correct statement will be -

View SolutionIf the above question, the potential has correct relations as given=
A. `V_(A) gt V_(B) gt V_(C) gt V_(D)`
B. `V_(A) gt V_(B) ge V_(C) gt V_(D)`
C. `V_(D)=V_(C)=V_(B)=V_(A)`
D. `V_(C) lt V_(B) gt V_(A) gt V_(D)`
View SolutionIn the above question, the surface charge densities have the correct relation is-
A. `sigma_(A) gt sigma_(B) gt sigma_(C) gt sigma_(D)`
B. `sigma_(A)=sigma_(B)=sigma_(C)=sigma_(D)`
C. `sigma_(D) gt sigma_(C) gt sigma_(B) gt sigma_(A)`
D. `sigma_(C) lt sigma_(B) gt sigma_(A) gt sigma_(D)`
View SolutionA family of equipotential surface are shown. The direction of the electric field at point A is along-

View SolutionA and B are two concentric spherical shells. If A is given a charge `+q` while B is earthed as shown in figure then
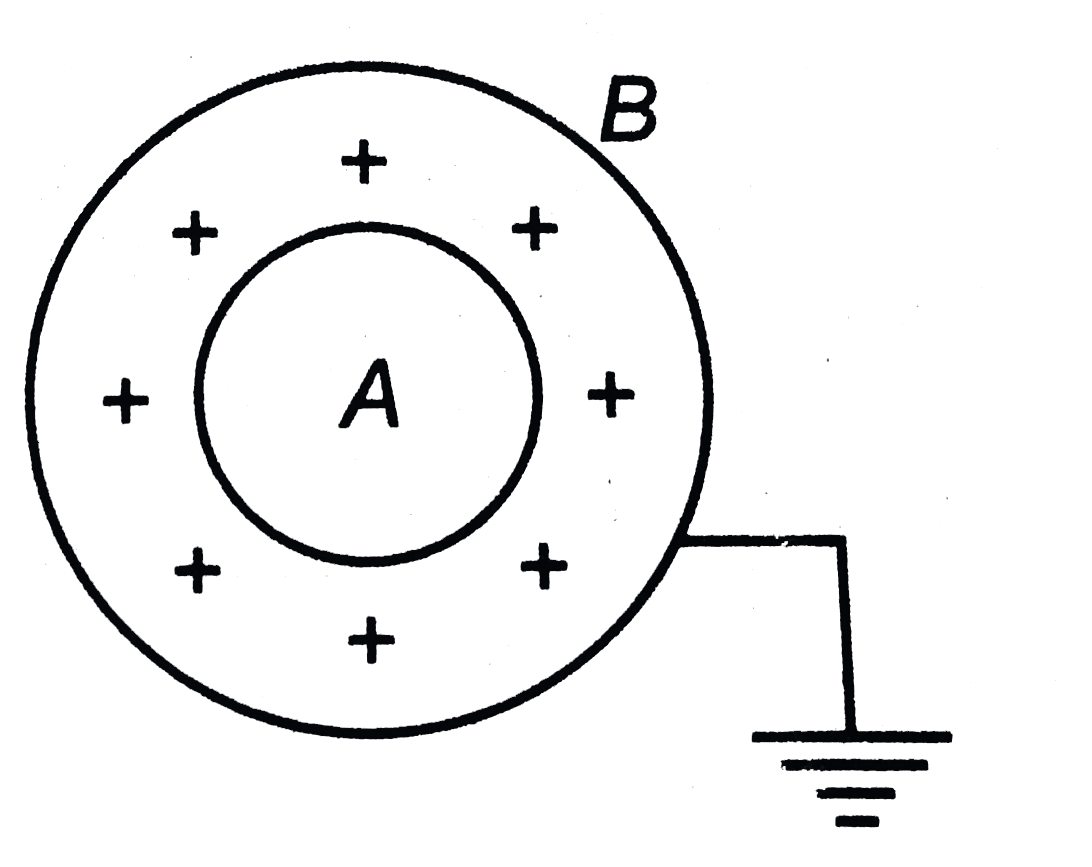
View SolutionMark the wrong statement-
View SolutionA hollow conducting sphere is placed in an electric field produced by a point charge placed at `P` as shown in figure.
Let `V_(A), V_(B), V_(C )` be the potentials at points `A, B and C` respectively. Then
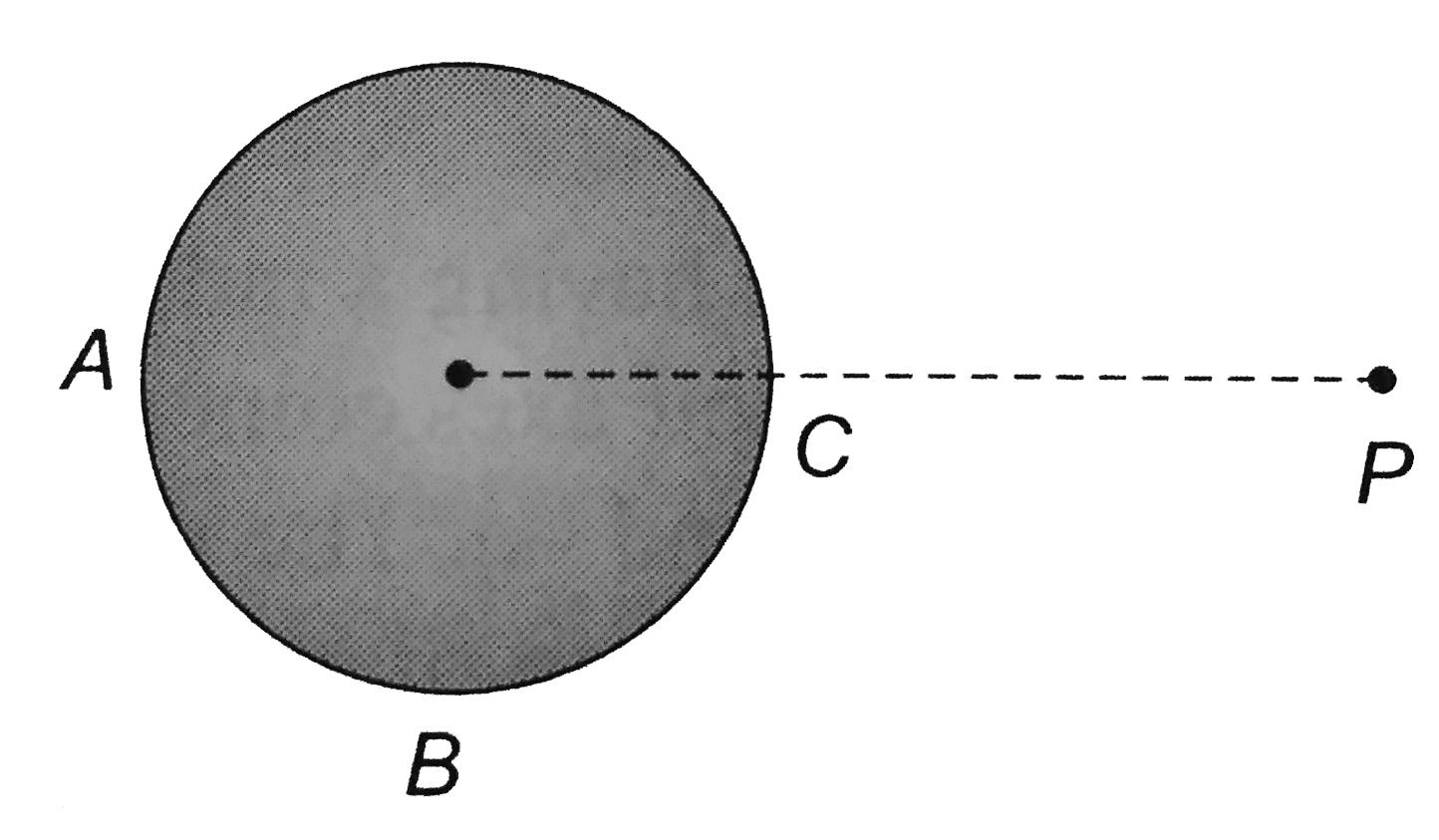
View SolutionA point charge is kept at the centre of a metallic insulated spherical shell. Then
View SolutionSTATEMENT 1: When a negative charge -q is released at a distance R from the centre and along the axis of a uniformly and positvely charged fixed ring of radius R, the negative charge does oscillation but not SHM. STATEMENT 2: The force on negative charge is always towards the centre of the ring but it is not proportional to the displacement from the centre of the ring.
View SolutionAssertion: A positively charged rod is held near a neutral conducting solid sphere as illustrated below. The sphere lies on a insulated stand. The potential of ground (or earth) is zero. The potential at point `A` (point `A` need not be centre of the sphere) is higher compared to potential of gound (earth).
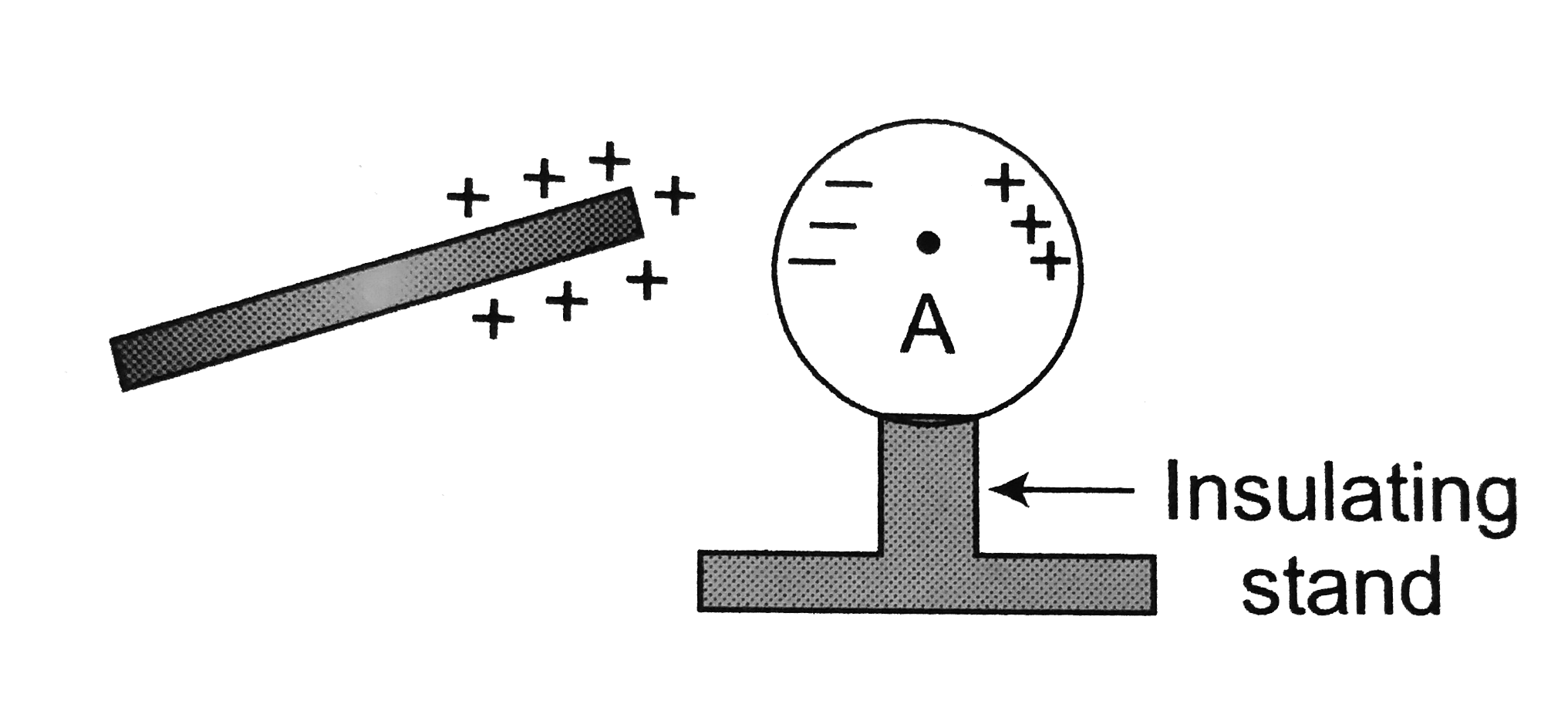
Reason: In this situation of assertion, the potential at the centre of conducting sphere is positive. the solid sphere being conducting, potential at each point in the sphere is same.
View Solution
Exercise questions and complete solutions for chapter ELECTROSTATICS of RESONANCE ENGLISH of Class 11