Complete Solutions to JEE Previous Year of chapter THREE-DIMENSIONAL GEOMETRY of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | THREE-DIMENSIONAL GEOMETRY |
| CENGAGE NEW | JEE Previous Year |
| Number Of Questions | 26 |
| Academic Year | 2023-24 |
(i) Find the equation of the plane passing through the points`(2,1,0),(5,0,1)a n d(4,11)dot` (ii) If `P` s the point `(2,1,6),` then the find the point `Q` such that `P Q` is perpendicular to the plane in (i) and the midpoint of `P Q` lies on it.
04:43View Solutionabout to only mathematics
02:58View SolutionA parallelepiped `S` has base points `A ,B ,Ca n dD` and upper face points `A^(prime),B^(prime),C^(prime),a n dD '` . The parallelepiped is compressed by upper face `A ' B ' C ' D '` to form a new parallepiped `T` having upper face points `A",B",C"a n dD"` . The volume of parallelepiped `T` is 90 percent of the volume of parallelepiped `Sdot` Prove that the locus of `A"` is a plane.
06:31View Solutionabout to only mathematics
04:19View SolutionA line with positive direction cosines passes through the point P(2, – 1, 2) and makes equal angles with the coordinate axes. The line meets the plane 2x + y + z = 9 at point Q. The length of the line segment PQ equals
03:24View SolutionThe value of `k` such that `(x-4)/1=(y-2)/1=(z-k)/2` lies in the plane `2x-4y=z=7` is a. 7 b. -7 c. no real value d. 4
01:54View SolutionIf the two lines `(x-1)/(2)=(y+1)/(3)=(z-1)/(4)and(x-3)/(1)=(y-m)/(2)=z` intersect at a point, find the value of m.
04:35View Solutionabout to only mathematics
03:50View SolutionA plane passes through (1,-2,1) and is perpendicualr to two planes` 2x-2y+z=0" and "x-y+2z=4,` then the distance of the plane from the point (1,2,2) is
03:46View SolutionLet `P(3,2,6)` be a point in space and `Q` be a point on line ` vec r=( hat i- hat j+2 hat k)+mu(-3 hat i+ hat j+5 hat k)dot` Then the value of `mu` for which the vector ` vec P Q` is parallel to the plane `x-4y+3z=1` is a. 1/4 b. -1/4 c. 1/8 d. -1/8
02:56View Solutionabout to only mathematics
03:25View SolutionIf the distance of the point `P(1,-2,1)` from the plane `x+2y-2z=alpha,w h e r ealpha>0,i s5,` then the foot of the perpendicular from `P` to the place is a. `(8/3,4/3,-7/3)` b. `(4/3,-4/3,1/3)` c. `(1/3,2/3,(10)/3)` d. `(2/3,-1/3,-5/3)`
04:24View Solutionabout to only mathematics
05:36View Solutionabout to only mathematics
03:41View SolutionTwo lines `L_1x=5, y/(3-alpha)=z/(-2)a n dL_2: x=alphay/(-1)=z/(2-alpha)` are coplanar. Then `alpha` can take value (s) a. `1` b. `2` c. `3` d. `4`
03:58View Solutionabout to only mathematics
02:42View SolutionIn `R^(3)` let L be straight line passing through the origin. Suppose that all the points on L are at a constant distance from the two planes `P_(1):x+2y-z+1=0 and P_(2) : 2x-y+z-1=0` Let M be the locus of the feet of the perpendiculars drawn from the points on L to the plane `P_(1)` . which of the following points lie (s) on M?
12:01View SolutionIn `R_(3) , ` consider the planes `P_(1):y=0and P_(2) : x+z=1. Let P_(3)` be a plane , different from `P_(1) and P_(2)` , which passes through the interesection of `P_(1) and P_(2) `I fhte distance of the (0,1,0) from `p_(3)` is 1 and the distance of a point `(alpha , beta , gamma )` from `P_(3)` is 2, then which of the following relations is ( are ) true ?
09:48View SolutionConsider the planes `3x-6y-2z=15a n d2x+y-2z=5.` Statement 1:The parametric equations of the line intersection of the given planes are `x=3+14 t ,y=2t ,z=15 tdot` Statement 2: The vector `14 hat i+2 hat j+15 hat k` is parallel to the line of intersection of the given planes.
03:11View Solutionabout to only mathematics
03:27View SolutionConsider the line ` L 1 : (x +1)/3 = (y +2)/1 = (z+ 1)/2, L2 : (x-2)/1 = (y+2)/2 = (z-3)/3 `
02:47View SolutionFind the direction cosines of the vector ` 3hati+2hat j+hat k`
03:23View SolutionIf `f(x)=3x-2` and `g(x)=2x+a` and if `fog=gof`, then find the value of a
02:15View SolutionConsider the linear equations `ax+by+cz=0, bx+cy+az=0 and cx+ay+bz=0`.
Match the conditions/expressions in Column I with statements in Column II.
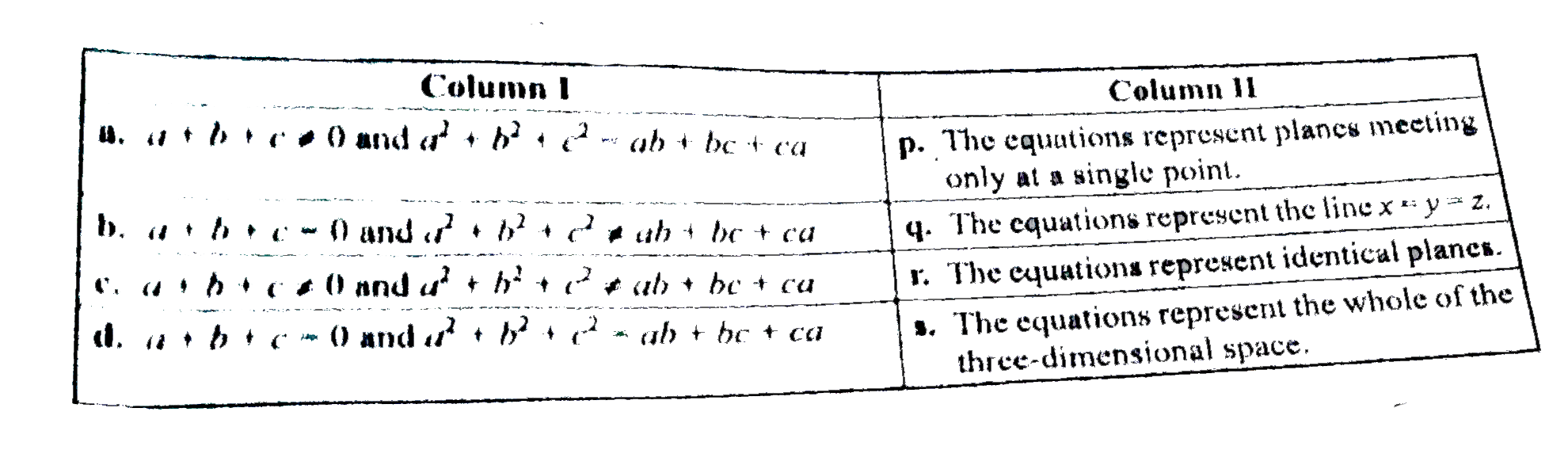 04:57View Solution
04:57View SolutionLet `f:R→R:f(x)=x^2 and g: R→R: g(x)=(x+1)`. Show that `(gof)≠(fog)`.
02:21View SolutionIf the distance between the plane Ax 2y + z = d and the plane containing the lines 2 1x = 3 2y = 4 3z and 3 2x = 4 3y = 5 4z is 6 , then |d| is
02:35View Solution
JEE Previous Year questions and complete solutions for chapter THREE-DIMENSIONAL GEOMETRY of CENGAGE of Class 12
