Complete Solutions to Illustrations of chapter GRAPHICAL TRANSFORMATIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | GRAPHICAL TRANSFORMATIONS |
| CENGAGE NEW | Illustrations |
| Number Of Questions | 56 |
| Academic Year | 2023-24 |
Plot `y=|x|,y+x-2|,a n dy=|x|2|`
02:39View SolutionDraw the graph of `y=sin^(-1)(x-3)`.
01:33View SolutionDraw the graph of `y="log"_(e)(x+3)`,
01:05View SolutionDraw the graph of `y=cot^(-1)x+sec^(-1)x+cosec^(-1)x`.
01:41View SolutionDraw the graph of `y=|x-3|+1`.
02:02View SolutionSolve `|x-1|-|x+3|lt6` graphically
03:53View SolutionFor `alt=0` , determine all real roots of the equation (1986, 5M) `x^2-2a|x-a|-3a^2=0`
03:05View SolutionConsider the function `f(x)={{:(x-[x]-(1)/(2),x !in),(0, "x inI):}` where [.] denotes the fractional integral function and I is the set of integers. Then find `g(x)max.[x^(2),f(x),|x|},-2lexle2.`
05:43View SolutionDraw the graph of `y=log_(e)3x` and compare with `y=log_(e)x`.
01:21View SolutionDraw the graph of `y=cos^(-1)(x//4)` and compare with `y=cos^(-1)x`.
01:23View SolutionDraw the graph of `y=0.5(x-1)^(2)` and compare with `y=(x-1)^(2)`.
03:15View SolutionPlot `y=sinxa n dy=sin2xdot`
02:30View SolutionIf `nsinx=log_(e)x` has exactly 1 root, then find the possible value of `n(ninN)`.
View SolutionPlot the curve `y=(log)_e(-x)dot`
01:25View SolutionDraw the graph of `y=2^(-x)`.
01:33View SolutionDraw the graph of `y=cot^(-1)(-x)`.
01:00View SolutionDraw the graph of `y=-cot^(-1)x`.
01:13View SolutionDraw the graph of `y=-log_(e)x`.
02:01View SolutionDraw the graph of `y=2-|x-1|`.
01:44View SolutionDraw the graph of `y=sin^(-1)(cosx)`.
02:32View SolutionGiven the graph of `y=f(x)`.

Draw the graphs of the followin.
(a) `y=f(1-x)` (b) `y=-2f(x)`
(c) `y=f(2x)` (d) `y=1-f(x)`03:09View SolutionDraw the graph of `y=|logx|`.
00:46View SolutionDraw the graph of `y=|sinx|` and hence the graph of `y=sin^(-1)|sinx|`.
02:38View SolutionDraw the graph of `y=f(x)=sqrt(1-cosx)`
01:15View SolutionDrew the graph of `y=|sinx-0.5|`.
02:19View SolutionThe number of solution of `2cosx=|sinx|` where `x in [0.4pi]` is/are
02:31View SolutionSolve `|x^2+4x+3|+2x+5=0.`
06:26View SolutionSolve `cos2xgt|sinx|,x in(-(pi)/(2),pi)`
07:24View SolutionProve that the equation `2 sin x=|x|+a` has no solution for `a in ((3sqrt(3)-pi)/3, oo)`.
04:55View SolutionSolve `|x^(2)-1|+|x^(2)-4|lt6` graphically.
02:20View SolutionDiscuss the differentiability of `f(x)=mim.{|x|,|x-2|,2-|x-1|}`.
02:18View SolutionIf the equation `|x^2+b x+c|=k` has four real roots, then `b^2-4c >0a n d00a n dk >(4c-b^2)/4` none of these
03:29View SolutionSketch the curve `y="log"|x|`
01:55View SolutionDraw the graph of `y=sin|x|`.
01:15View SolutionDraw the graph of `y=[|x|]`, where `[.]` denotes the greatest integer function.
02:52View SolutionDraw the graph of `y=sin^(-1)|x|`.
01:48View SolutionDraw the graph of `y=tan^(-1)|x|`.
01:24View SolutionDraw the graph of `y={|x|}`, where `{.}` represents the fractional part function.
02:53View SolutionIf `y = x^3-3x + 2`, then draw the graph of the followings (i) `y=|x^3-3x + 2|`
02:02View SolutionDraw the graph of `y=|1-(1)/(|x|-2)|`.
01:33View SolutionDraw the graph of `|y|=|2^|x|-3|`.
02:23View SolutionFind the total number of solutions to `sinpix=|In|x||`.
03:29View SolutionFind the number of solutions to `7^|x|(|1-|x||)=1`.
01:45View SolutionThe graph of the function `y=f(x)` is shown.

Find the number of solutions to the equation `||f(x)|-1|=(1)/(2)`.02:47View SolutionConsider the function `f(x)=x^(2)+bx+c,` where `D=b^(2)-4cgt0`, then match the follwoing columns.
 View Solution
View SolutionDraw the graph of `|y|=sinx`.
01:13View SolutionDraw the graph of `|y|=tanx`.
01:19View SolutionSketch the curve `|y|=(x-1)(x-2)dot`
03:21View SolutionDraw the graph of `|y|={x}`, where `{.}` represents the fractional part function.
01:27View SolutionDraw the graph of `|x|+|y|=1+x`.
02:35View SolutionDraw the graph of `|x|-|y|=2` using graphical transformation.
03:24View SolutionDraw the graph of `|y|=|2^|x|-3|`.
02:23View SolutionThe graph of the function `y=f(x)` is as shown in the figure.
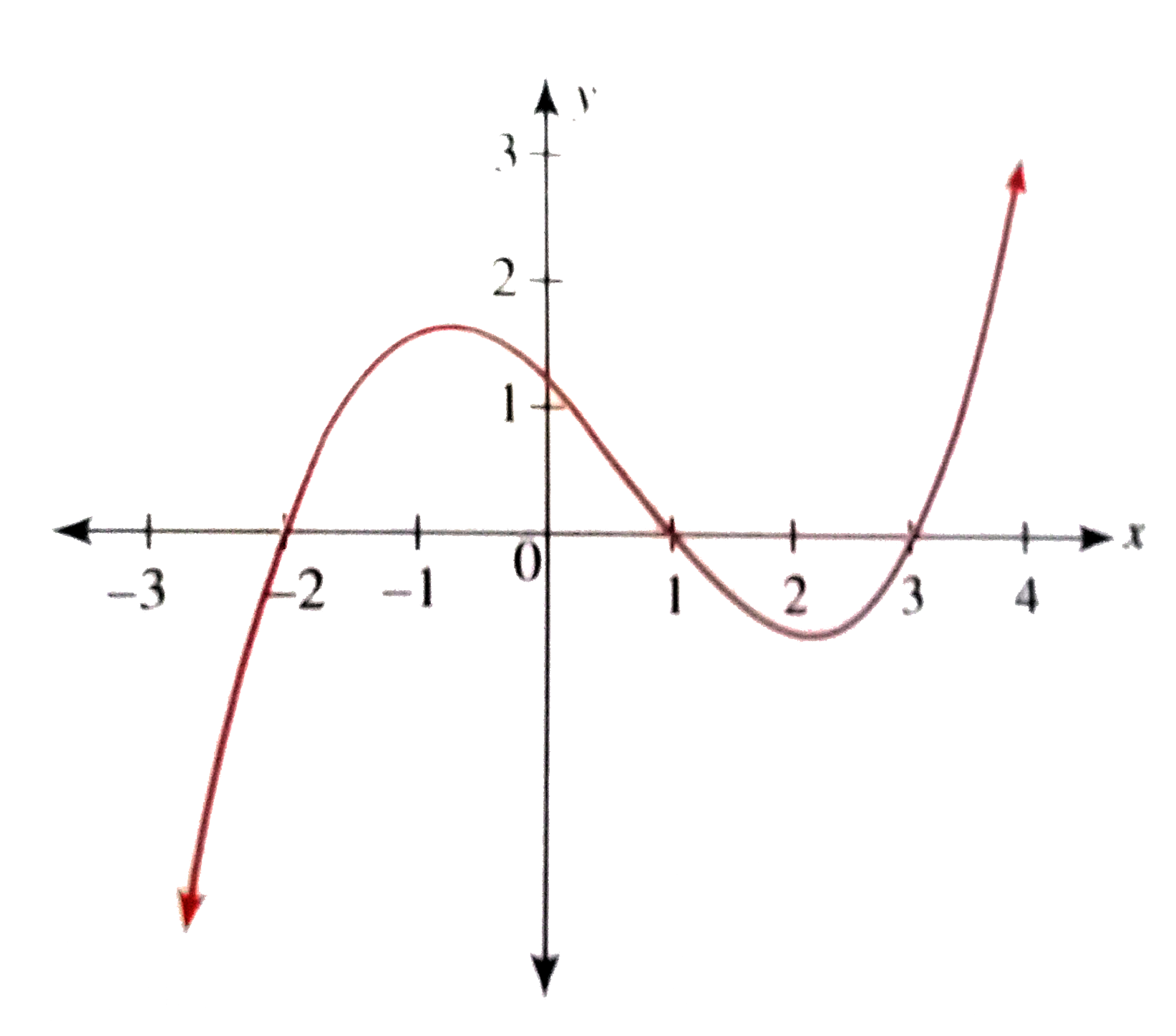
Then draw the graphs of
(i) `|y|=sgn(f(x))` (ii) `|y|=|f(x|)|`
(iii) `y=x^(sgn)(f(x))`View SolutionDraw the graph and find the points of discontinuity `f(x) = [2cos x]` , `x in [0, 2pi]` . ([.] represents the greatest integer function.)
04:48View SolutionDraw the graph and discuss the continuity of `f (x) = [sin x + cos x], x in [0, 2pi),` where [.] represents the greatest integer function.
06:27View SolutionDraw the graph and find the points of discontinuity for `f(x)=[x^(2)-x-1],x in[-1,2]` ([.] represents the greatest integer function).
06:10View Solution
Illustrations questions and complete solutions for chapter GRAPHICAL TRANSFORMATIONS of CENGAGE MATHS (HINGLISH) of Class 12
