Complete Solutions to NCERT SECTION (Exercise 7.1) of chapter TRIANGLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | TRIANGLES |
| MTG IIT JEE FOUNDATION | NCERT SECTION (Exercise 7.1) |
| Number Of Questions | 14 |
| Academic Year | 2023-24 |
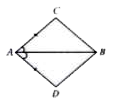
In quadrilateral ABCD, AC=AD and AB bisects `angleA` (see figure). Show that `DeltaABC~=DeltaABD`. What can you say about BC and BD ?
View SolutionABCD is quadrilateral in which `AD=BC and angleDAB= angleCBA` (see figure). Prove that
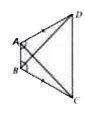
`DeltaABD~=DeltaBAC`
View SolutionABCD is quadrilateral in which `AD=BC and angleDAB= angleCBA` (see figure). Prove that

`BD=AC`
View SolutionABCD is quadrilateral in which `AD=BC and angleDAB= angleCBA` (see figure). Prove that
`angleADB=angleBCA`.
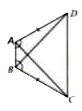
View SolutionAD and BC are equal perpendiculars to a line segment AB (see Fig. 7.18). Show that CD bisects AB.
View Solutionl and m are two parallel lines intersected by another pair of parallel lines p and q (see Fig. 7.19). Show that `DeltaA B C~=DeltaC D A`.
View SolutionLine l is the bisector of an `angleA` and B is any point on I. BP and BQ are perpendiculars from B to the arms of `angleA` (see figure). Show that:
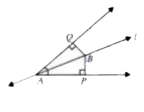
`DeltaAPB~=DeltaAQB`
View SolutionLine l is the bisector of an `angleA` and B is any point on I. BP and BQ are perpendiculars from B to the arms of `angleA` (see figure). Show that:
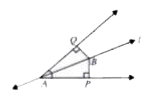
BP=BQ or B is equidistant from the arms of `angleA`.
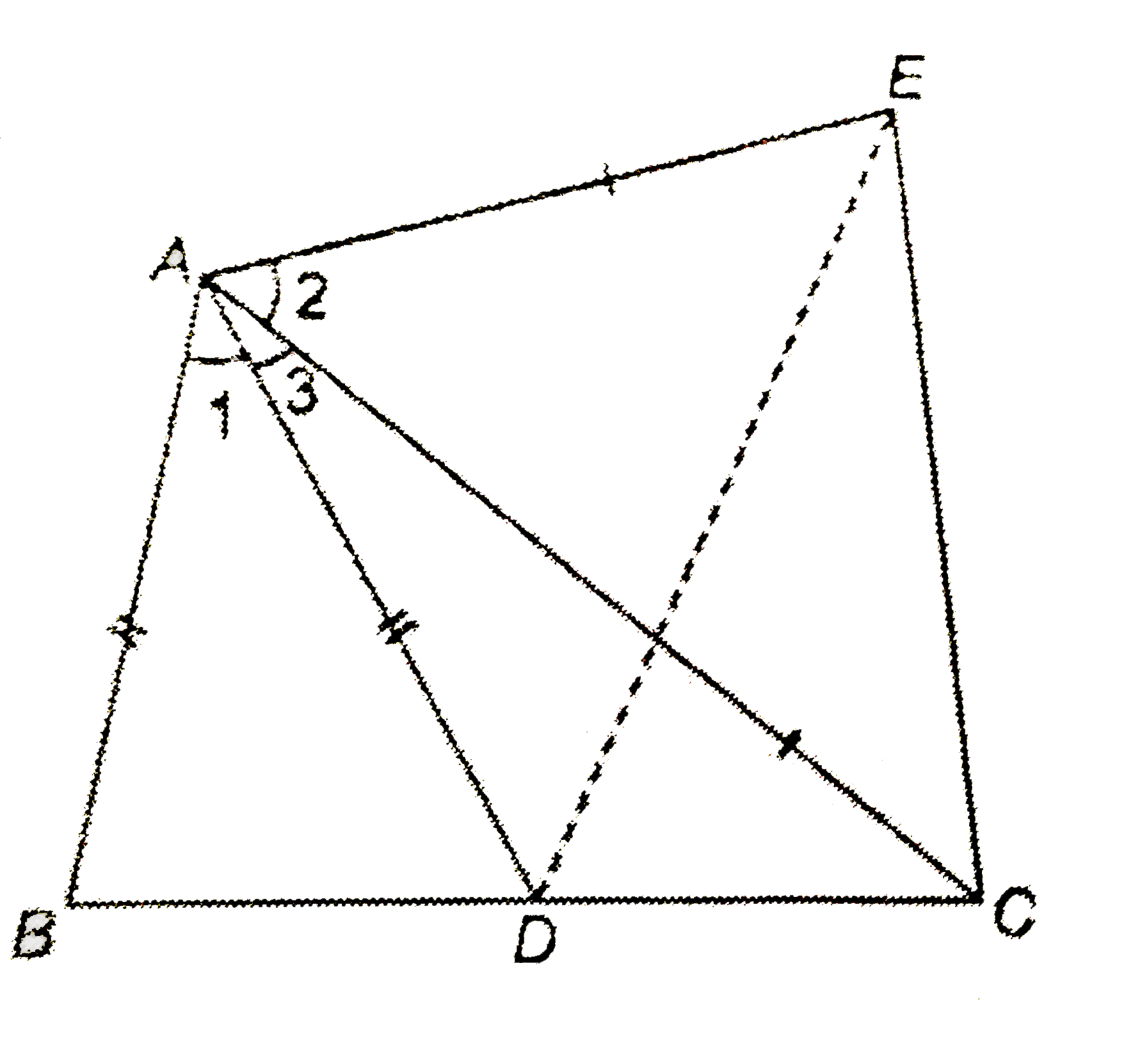
View SolutionIn the given figure, `AC=AE, AB=AD` and `angleBAD=angleEAC`. Prove that `BC=DE`.
View SolutionAB is a line segment D and P is its mid-point. D and E are points on the same side of AB such that `angleBAD = angleABE and angleEPA = angleDPB` (see figure). Show that

`DeltaDAP~=DeltaEBP`
View SolutionAB is a line segment and P is its mid-point. D and E are points on the same side of AB such that `angleBAD = angleABE and angleEPA = angleDPB` (see figure). Show that

`AD=BE`
View SolutionIn right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

`DeltaAMC~=DeltaBMD`
View SolutionIn right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

`angleDBC` is right angle.
View SolutionIn right triangle ABC, right angled at C, Mis the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see figure). Show that

`DeltaDBC~=DeltaACB`
View Solution
NCERT SECTION (Exercise 7.1) questions and complete solutions for chapter TRIANGLES of MTG IIT JEE FOUNDATION of Class 9
All Exercises from MTG IIT JEE FOUNDATION Class 9 MATHS TRIANGLES
- EXERCISE (Multiple Choice Questions) (ASSERTION & REASON TYPE)
- EXERCISE (Multiple Choice Questions) (COMPREHENSION TYPE)
- EXERCISE (Multiple Choice Questions) (LEVEL-1 )
- EXERCISE (Multiple Choice Questions) (LEVEL-2 )
- EXERCISE (Multiple Choice Questions) (MATCH THE FOLLOWING)
- EXERCISE (Multiple Choice Questions) (PASSAGE)
- EXERCISE (Subjective Problems) ( Integer/Numerical Value Type)
- EXERCISE (Subjective Problems) ( Long Answer Type)
- EXERCISE (Subjective Problems) ( Short Answer Type)
- EXERCISE (Subjective Problems) (Very Short Answer Type)
- ILLUSTRATIONS
- NCERT SECTION (Exercise 7.1)
- NCERT SECTION (Exercise 7.2)
- NCERT SECTION (Exercise 7.3)
- NCERT SECTION (Exercise 7.4)
- NCERT SECTION (Exercise 7.5)
- OLYMPAID/ HOTS CORNER
- SOLVED EXAMPLES