S is the centre of the circumcircle of ΔABC and if AD⊥BC, prove that ∠BAD=∠SAC. Solution in Bengali
S is the centre of the circum-circle of ΔABC.
∴SA=SB≠SC.
Also, AD⊥BC,∠ADB=90∘,∴∠ABD+∠BAD=90∘
or, ∠ABD−90∘−∠BAD........(1)
Now, in the circle with centre S, the central angle produced by the chord AD =∠ASC and angle in circle ∠ABC
∴ASC=2∠ABC.......(2)
But SA=SC[∴ radii of same circle]
∴∠SAC=∠SCA.......(3)
Then, ∠ASC+∠SAC+∠SCA=180∘
or, ∠ASC+∠SAC+∠SAC=180∘[from(3)]
or ∠ASC+2∠SAC=180∘
or, 2∠ABC+2∠SAC=180∘ [ by (2)]
or, ∠ABC+∠SAC=90∘ [ dividing by 2]
or, ∠ABD+∠SAC=90∘[∴∠ABCand∠ABD are same angle]
or,90∘−∠∠BAD+∠SAC=90∘ [ from (1)]
or, ∠SAC−∠BAD=0, or ∠SAC=∠BAD
Hence ∠ABD=∠SAC

Topper's Solved these Questions
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (True or False)4videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short-Answer Type Questions) MCQs5videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short Answer Type Questions)5videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)12videos
THEOREMS RELATED TO CYCLIC QUADRILATERAL
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.223videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
Similar Questions
In the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
07:32View SolutionIn the adjoining figure, O is the centre of the circle AC is a diameter and chord DE is parallel to the diameter AC. If ∠CBD=60∘ , find the value of ∠CDE
03:49View SolutionIn the adjacent figure O is the centre of the circle. If ∠OAB=30∘,∠ABC=120∘,∠BCO=y∘and∠COA=x∘, then the find value sof x and y.
03:04View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
02:56View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
03:58View SolutionIn any triangle ABC, if the angle bisector of ∠Aand perpendicular bisector of BCintersect, prove that they intersect on the circumcircle of the triangle ABC
07:41View SolutionA Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
04:39View SolutionΔABC is right-angled at A and AD⊥BC. If BC=13cmandAC=5cm, find the ratio of areas of ΔABCandΔADC.
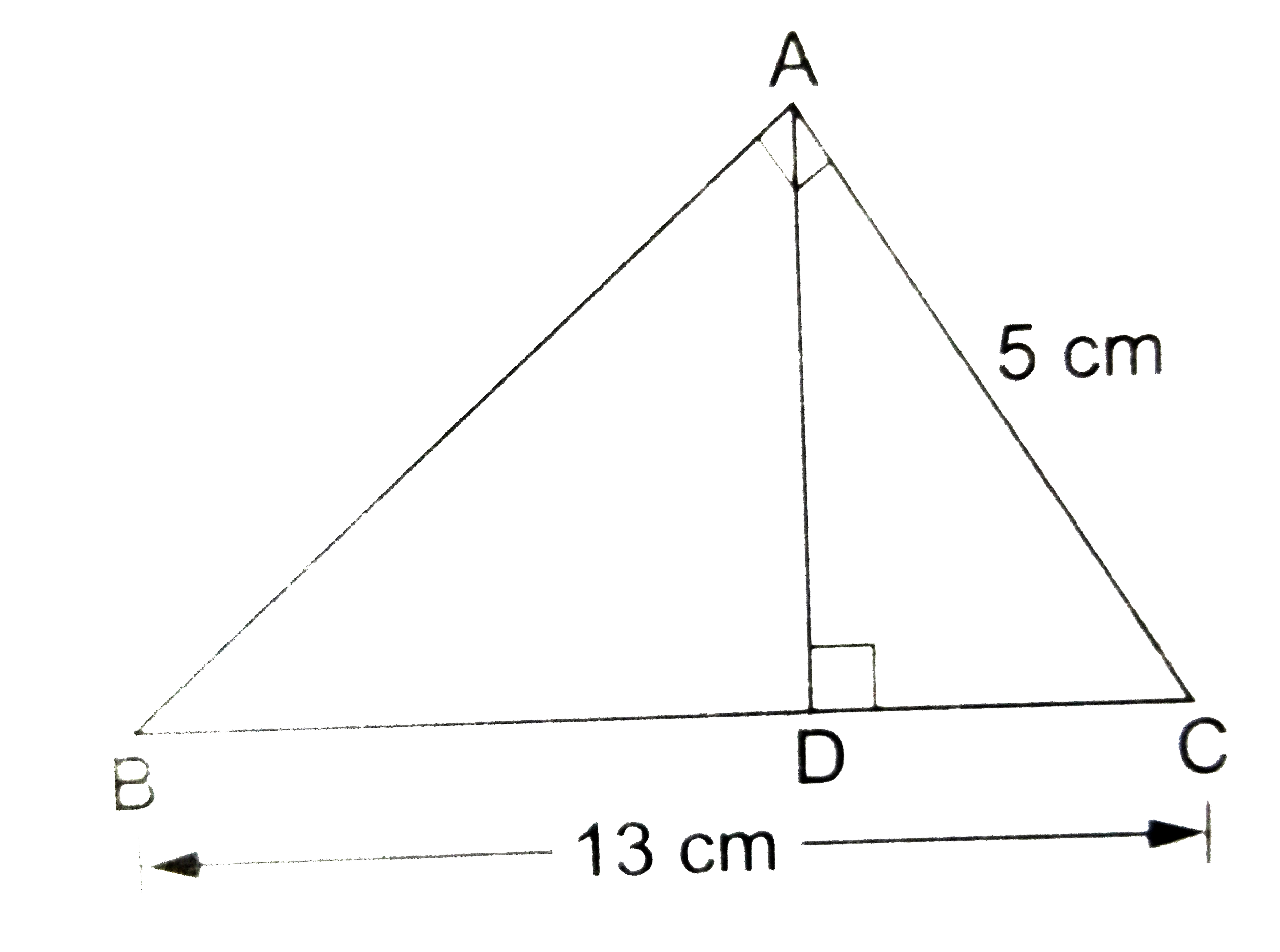 03:43View Solution
03:43View Solutionएक △ABC में, AD⊥BC तथा AD2=BD×CD है तो सिद्ध कीजिए कि △ABC का समकोण त्रिभुज है |
03:11View Solution△ABC एक समकोण त्रिभुज है जिसका ∠Aसमकोण है तथा AD⊥BC है तो सिद्ध कीजिए कि BDAD=ABAC
04:22View SolutionO is the orthocentre of ΔABCandAD⊥BC. If AD is produced it interect the circumcircle of ΔABC at the point G. Prove that OD=DG.
09:37View SolutionO is the circumcentre of ΔABCandOD⊥BC. Prove that ∠BOD=∠BAC
04:59View Solutionत्रिभुज ABC में, यदि AD⊥BC तथा AD2=BD×DC हो, तो सिद्ध करो की ∠BAC=90∘|
02:49View Solutionचित्र में यदि AD⊥BC और BDDA=DADC हो, तो सिद्ध करो की ΔABC एक समकोण त्रिभुज है|
11:15View Solutionयदि ABC का समकोण त्रिभुज है जिसका कोण A समकोण है तथा AD⊥BC है, तो BDDC=
03:57View Solution

