A plane light wave with wavelength λ=0.65μm falls normally on a large glass plate whose opposite side has a large and an opaque strip of width a=0.30mm (Fig.) A screen is placed at a distance b=110cm from the plate. The height h of the ledge is such that the intensity of light at point 2 of the screen is the heighest possible. making use of Fig. find the ratio of intensities at point 1 and 2.
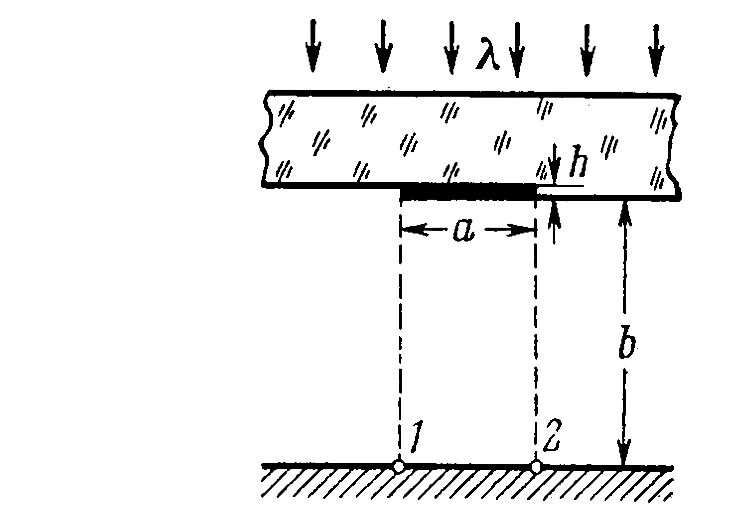
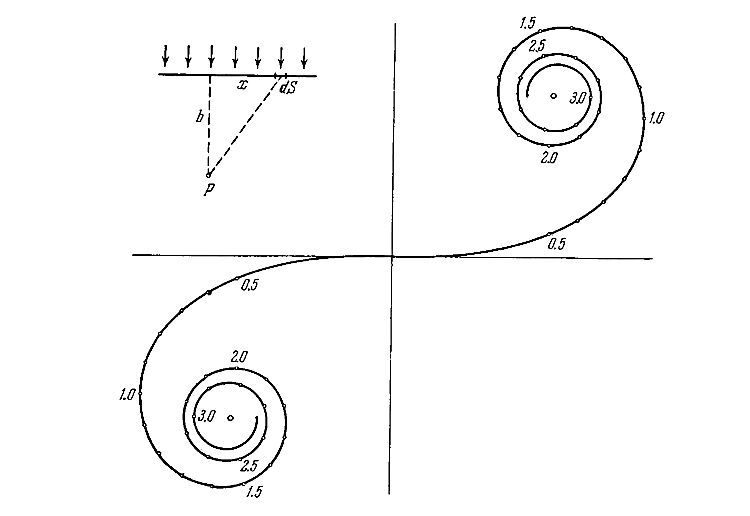

Using the method of problem 5.103 we can immediately write down the amplitudes as 1 and 2. We get:
At 1 amplitude A1~const⎡⎢⎣0∫−∞e−iπu2/2du+e−Iδ∞∫ve−iπu2/2du⎤⎥⎦
At 2 amplitude A2~const⎡⎢⎣0∫−∞e−iπu2/2du+e−Iδ∞∫0e−iπu2/2du⎤⎥⎦
where v=a√2bλ
is the parameter of Cornu's spiral and consatnt factor is common to 1 and 2.
With the sual notation
C=C(v)=v∫0cos.πu22du
S=S(v)=v∫0πu22du
and the result ∞∫0cos.πu22du=∞∫0sin.πu22du=12
We find the ratio of intensituies as
I2I1=∣∣
∣
∣∣(12−C)−i(12−S)+e−iδ(1−i)21−i2+e−iδ{(12−C)−i(12−S)}∣∣
∣
∣∣2
(The constant in A1 and A2 must be the same by symmetry)
In our csse, a=0.30mm,λ=0.65μm,b=1.1m
v=0.30×√21.1×0.65=0.50
C(0.50)=0.48S(0.50)=0.06
I2I1=∣∣
∣
∣∣0.02−0.44i+e−iδ(1−i)21−i2eiδ+0.02−0.44i∣∣
∣
∣∣2=∣∣
∣∣1+(0.02−0.44i)√2eiδ+iπ41+(0.02−0.44i)√2e−iδ+iπ4∣∣
∣∣2
But 0.02−0.44i=0.44eiα,α=1.525rad(≈87.4∘)
So I2I1=∣∣∣1+0.44×√2×ei(δ−0.740)1+0.44×√2×e−i(δ+0.740)∣∣∣2=1+2(0.44)2+2√2×0.44cos(δ−0.740)1+2(0.44)2+2√2×0.44cos(δ+0.740)
i2 is maximum when δ−0.740=0 (modulo 2π)
Thus in that case I2I1=1.387+1.2451.387+1.245cos(1.48)=2.6321.5≈1.75
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light
43 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Dispersion And Absorption Of Light
24 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Interference Of Light
33 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
A plane light wave with wavelength λ=0.65μm falls normally on a large glass plate whose opposite side has a longrectangular recess 0.60mm wide. Using Fig. find the depth H of the recess at which the diffraction pattern on the screen 77cm away from the plate has the maximum illuminance at its centre.
View SolutionA plane light wave with wavelength λ=0.60μm falls normally on a sufficiently large glass plate having a round recess on the oppiste side (Fig.) For the observation point P that recess correcponds to the first one and and a half Fresnel zones. Find the depth h of the recess at which the intensity of light at the point P is
(a) maximum,
(b) minimum,
(c ) equal to the intensity of incident light.
View Solution
Knowledge Check
A screen is placed at a distance of 1m from a point of source of light . What will be the effect on the intensity (I) of light observed on the screen , if the screen is kept at 150 cm from the same point source ?
A1 increases by 50%B1 decreases by 40 %C1 decreases by 55.55%D1 increases by 55.55%Light d of wavelength 5000Å is incident normally on a slite. The first minimum of the distance of 5 mm from the central maximum on a screen placed at a distance of 2 m from the slit. The width of slit is
A0.1mmB0.2mmC0.3mmD0.4mm
A plane light wave wityh wavelength 0.60μm falls normally on a long opaque strip 0.70mm wide. Behind it a screen is placed at a distance 100cm. Using Fig. find the ratio of intensities of light in the middle of the diffraction pattern and at the edge of the geometrical shadow.
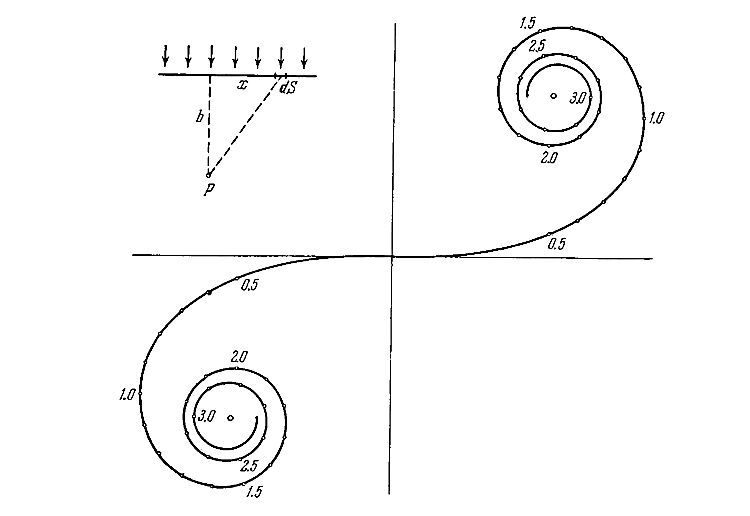
View SolutionA plane light wave with wavelength λ and intensity I0 falls normally on a large glass plate whose opposite side serves as an opaque screen with a round aperture equal to the first Fresnel zone for the observation point P. In the middle of the aperture there is a round recess equal to half the Fresnel zone. What must the depth h of that recess be for the intensity of light at the point P to be the highest? What is this intensity equal to?
View SolutionLight waves from two coherent source arrive at two points on a screen with path difference 0andλ/2. Find the ratio of intensities at the points.
View SolutionA plane light wave with wavelength λ=0.60μm and intensity I0 falls normally on a large glass plate whose side view is shown in Fig. At what height h of the ledge will be intensity of light at points located directly below be
(a) minimum,
(b) twice as low as I0 (the losses due to reflection are to be neglected).
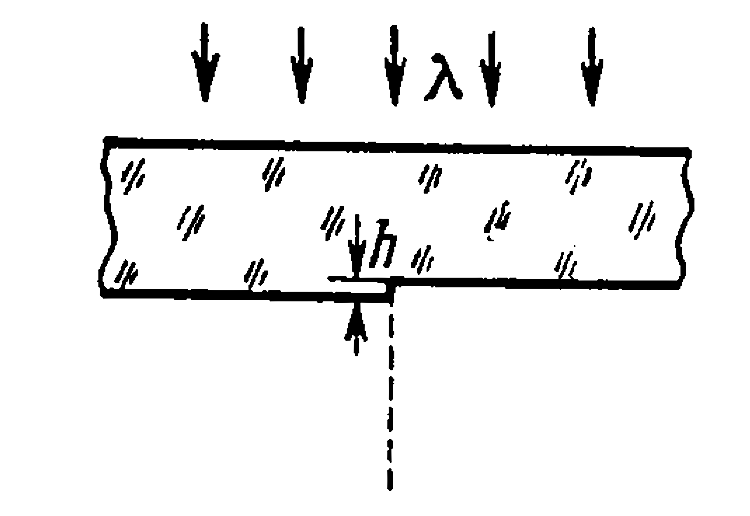
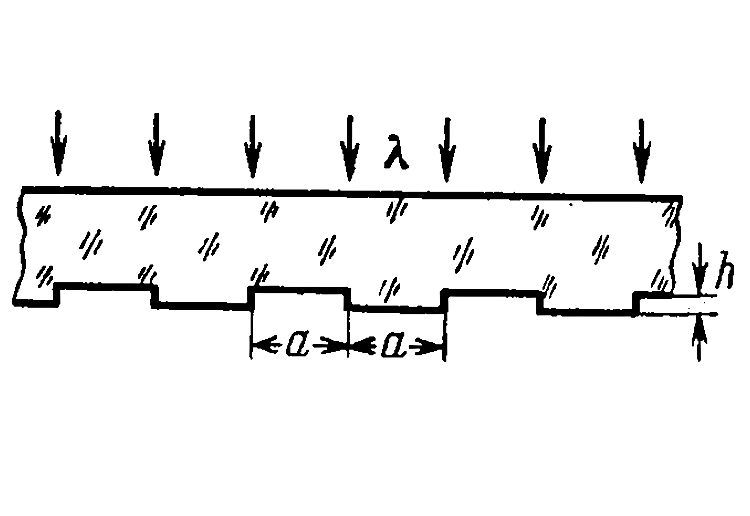
View SolutionA plane light wave with wavelength λ falls normally on a phase diffraction grating whose side view is shown in Fig. The grating is cut on a glass plate with refractive index n. Find the depth h of the lines at which the intensity of the central Fraunhofer maximum is equal to zero. what is in this case the diffraction angle corresponding to the first maximum?
View SolutionA plane light wave falls normally on a diaphragm with round aperature opening the first N Fresnel zones for a point P on a screen located at a distance b form the diaphragm. The wavelength of light is equal to λ. Find the intensity of light I0 in front of the diaphragm if the distribution of intensity of light I(r) on the screen is knows. Here r is the distance from the point P.
View Solution
