When 'n' number of particles of masses m are at distances x1=a,x2=ar,x3=ar2…xn=arn ,units from origin on the X-axis, then find the distance of centre of mass of the system from origin.
To find the distance of the center of mass of the system from the origin, we can follow these steps:
Step 1: Identify the positions of the particles
The positions of the particles are given as:
- x1=a
- x2=ar
- x3=ar2
- ...
- xn=arn−1
Step 2: Write the formula for the center of mass
The formula for the center of mass xcm of a system of particles is given by:
xcm=∑ni=1ximi∑ni=1mi
where xi is the position of the i-th particle and mi is the mass of the i-th particle.
Step 3: Substitute the values into the formula
Since all particles have the same mass m, we can substitute the positions and masses into the formula:
xcm=∑n−1i=0xim∑n−1i=0m
This simplifies to:
xcm=m∑n−1i=0arin⋅m
The mass m cancels out:
xcm=∑n−1i=0arin
Step 4: Calculate the sum of the geometric series
The sum n−1∑i=0ari is a geometric series. The formula for the sum of a geometric series is:
Sn=a1−rn1−r
In our case, the first term a=a and the common ratio r=r:
n−1∑i=0ari=a1−rn1−r
Step 5: Substitute the sum back into the center of mass formula
Now, substituting this sum back into the center of mass formula:
xcm=a1−rn1−rn
Step 6: Simplify the expression
Thus, the distance of the center of mass from the origin is:
xcm=a(1−rn)n(1−r)
Final Answer
The distance of the center of mass of the system from the origin is:
xcm=a(1−rn)n(1−r)
The positions of the particles are given as:
- x1=a
- x2=ar
- x3=ar2
- ...
- xn=arn−1
The formula for the center of mass xcm of a system of particles is given by:
xcm=∑ni=1ximi∑ni=1mi
where xi is the position of the i-th particle and mi is the mass of the i-th particle.
Since all particles have the same mass m, we can substitute the positions and masses into the formula:
xcm=∑n−1i=0xim∑n−1i=0m
This simplifies to:
xcm=m∑n−1i=0arin⋅m
The mass m cancels out:
xcm=∑n−1i=0arin
The sum n−1∑i=0ari is a geometric series. The formula for the sum of a geometric series is:
Sn=a1−rn1−r
In our case, the first term a=a and the common ratio r=r:
n−1∑i=0ari=a1−rn1−r
Now, substituting this sum back into the center of mass formula:
xcm=a1−rn1−rn
Thus, the distance of the center of mass from the origin is:
xcm=a(1−rn)n(1−r)
The distance of the center of mass of the system from the origin is:
xcm=a(1−rn)n(1−r)
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:C.U.Q.
Explore 83 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-1(C.W.)
Explore 64 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
When 'n' number of particles of masses m,2m,3m,….nm are at distances x1=1,x2=2,x3=3…xn=n units respectively from origin on the X-axis, then find the distance of centre of mass of the system from origin.
View SolutionWhen 'n' number of particles of masses m,2m,3m,….nm are at distances x1=1,x2=4,x3=9…xn=n2 units respectively from origin on the X-axis, then find the distance of centre of mass of the system from origin.
View Solution
Knowledge Check
Two points masses of 2 kg and 3 kg lie at 5 m and 9 m away from origin O respectively. Find out the distance of centre of mass of these point masses from the origin.
A14.2 mB7.4 mC20 mD6 mA large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
A=RB≤RC>RD≥RA number of particles each of mass 0.75kg are placed at distances 1m, 2m, 4m, 8m etc. from origin along positive X axis. the intensity of gravitational field at the origin will be -
AGB34GC12GDzero
A large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
View SolutionFor a group of particles of different masses,if all the masses are placed at different points at equal distance R from the origin, then the distance of the centre of mass from the origin will be
View SolutionO is the origin and B is a point on the x-axis at a distance of 2 units from the origin. Match the following lists.
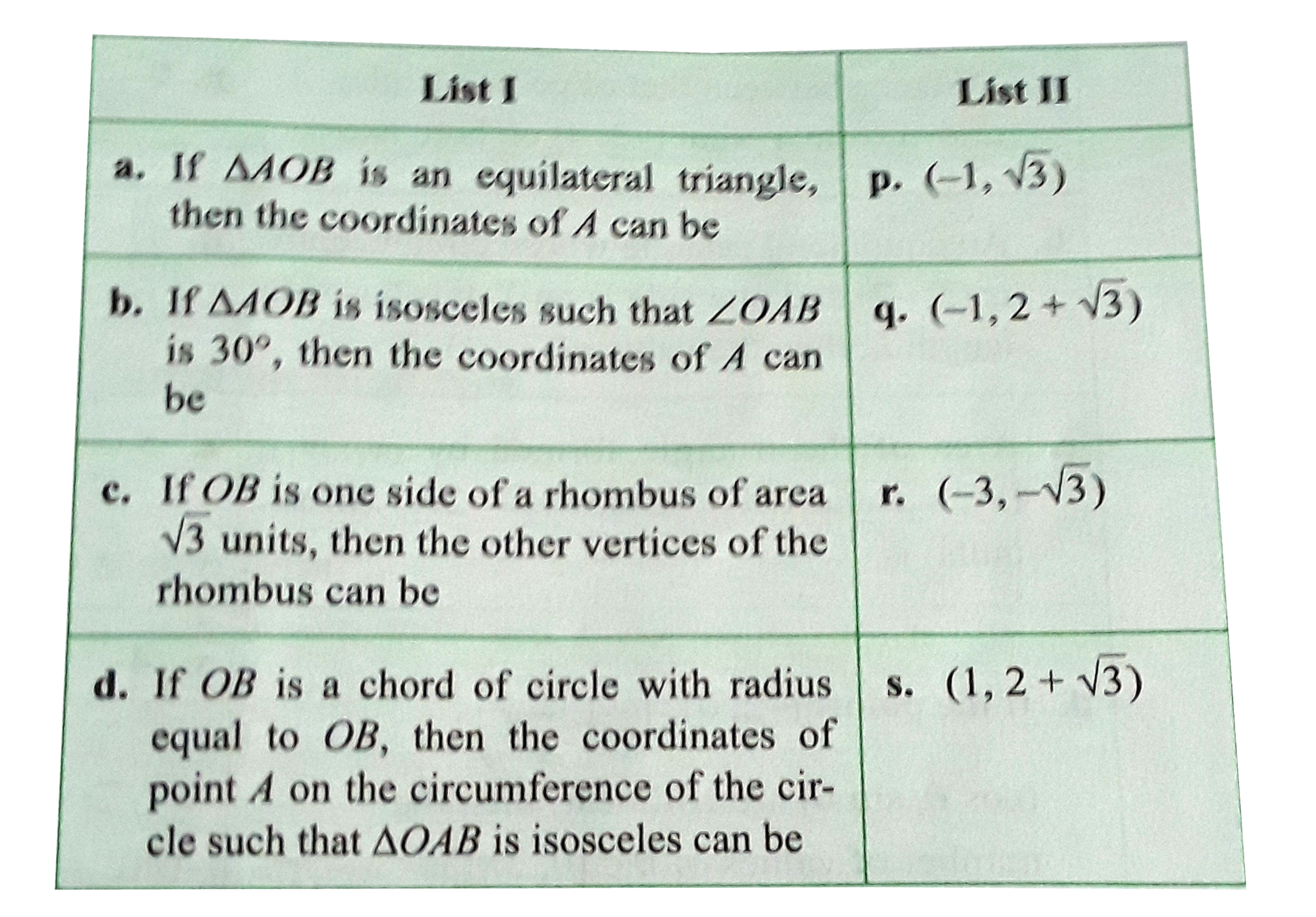
View SolutionProve that the point (x,√1−x2) is at a distance of 1 unit from the origin .
View SolutionAll the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
View Solution
