ABCD is a trapezium in which ABDC. If ar(ABD)=24 cm2and AB=8 cm, then height of ABC is 3 cm (b) 4 cm (c) 6 cm (d) 8 cm
Given: ABCD is a trapezium in which AB=8 cm and ar(△ABD)=24cm2
Solution: Here we can see that, In △ADB and △ACB are on same base.
Hence, ar(△ACB)=ar(△ADB)
ar(△ACB)=24
12(8×h)=24
h=6cm
Hence, option(c) is correct.
Topper's Solved these Questions
Similar Questions
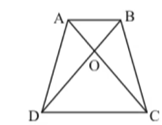
In Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
View SolutionIn Fig.42, ABCD is a parallelogram in which AD=21 cm, DH=18 cm and DK=27 cm. The length of side AB is 63 cm (b) 63.5 cm 31.5 cm (d) 31 cm
View SolutionIn Figure, ABCD is a parallelogram. If AB=12cm,AE=7.5cm,CF=15cm, then AD=
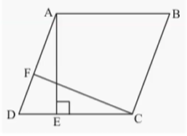
(a) 3 cm
(b) 6 cm
(c) 8 cm
(d) 10.5 cm
View SolutionIn Fig.42, ABCD is a parallelogram in which AD=21 cm, DH=18 cm and DK=27 cm. The perimeter of the parallelogram is 105 cm (b) 84.5 cm 169 cm (d) 52.5 cm
View SolutionIn an isosceles triangle ABC , if AB=AC=25cm and BC=14cm , then the measure of altitude from A on BC is (a) 20cm (b) 22cm (c) 18cm (d) 24cm
View SolutionABCPQR such that ar(ABC)=4ar(PQR). If BC=12cm , then QR= (a) 9cm (b) 10cm (c) 6cm (d) 8cm
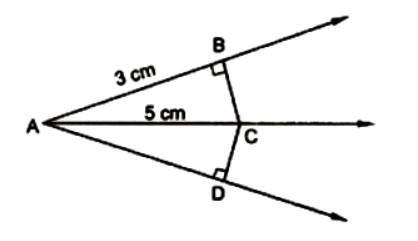
View SolutionIn Figure, if AC is bisector fo ∠BAD such that AB=3 cm and AC=5 cm, then CD= 2 cm (b) 3 cm (c) 4 cm (d) 5 cm
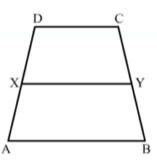
View SolutionIn Figure, ABCD is a trapezium in which AB,DCandDC=40cmandAB=60cm If XandY are, respectively, the mid-points of ADandBC, prove that: ar(traptDCYX)=911,ar(trap(XYBA)
View SolutionIf ABCDEF such that DE=3cm , EF=2cm , DF=2.5cm , BC=4cm , then perimeter of ABC is (a) 18cm (b) 20cm (c) 12cm (d) 15cm
View SolutionABCD is a trapezium in which AB∣∣DC , M and N are the mid-points of AD and BC respectively. If AB=12cm, MN=14cm, then CD= 10cm (b) 12cm (c) 14cm (d) 16cm
View Solution