Figure shows a thin rod (mass M, length L) suspended in a vertical plane by tying ideal strings at ends A and B. Initially, rod makes an angle 30∘ with horizontal and is at rest. Now, the string connected to the end B is cut. Let T be the tension in the string connected to A and aA be the magitude of acceleration of point A just after the cutting event. Pick correct option (s):

T=3mg13
aA=3g13
T=4mg13
aA=3√3g13
The correct Answer is:C, D
mg−T=ma
Tl2cosθ=ml212α
By constraints,
a=αl2cosθ
Thus, T=(mg).(1+3cos2θ) and aA=3gsinθcosθ1+3cos2θ

Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
The system shown in figure is initially in equilibrium. What is the acceleration of B just after the string connecting B to ground is cut ?
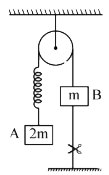
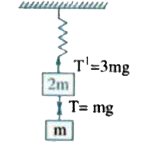
View SolutionThe system shown in the figure is in equilibrium at rest. The spring and string are massless Now the string is cut. Find the acceleration of the blocks just after the string is cut.
View Solution
Knowledge Check
The system shown in figure is initially in equilibrium. What is the acceleration of B just after the string connecting B to ground is cut ?
 Ag downwardsBg3 upwardsCzeroDg upwards
Ag downwardsBg3 upwardsCzeroDg upwardsA uniform rod of mass m and length l is hanged by two stirngs as shown in figure. Suddenly one string is cut. The tension in other string at this instant is
 A4MgBMg6CMg3DMg4
A4MgBMg6CMg3DMg4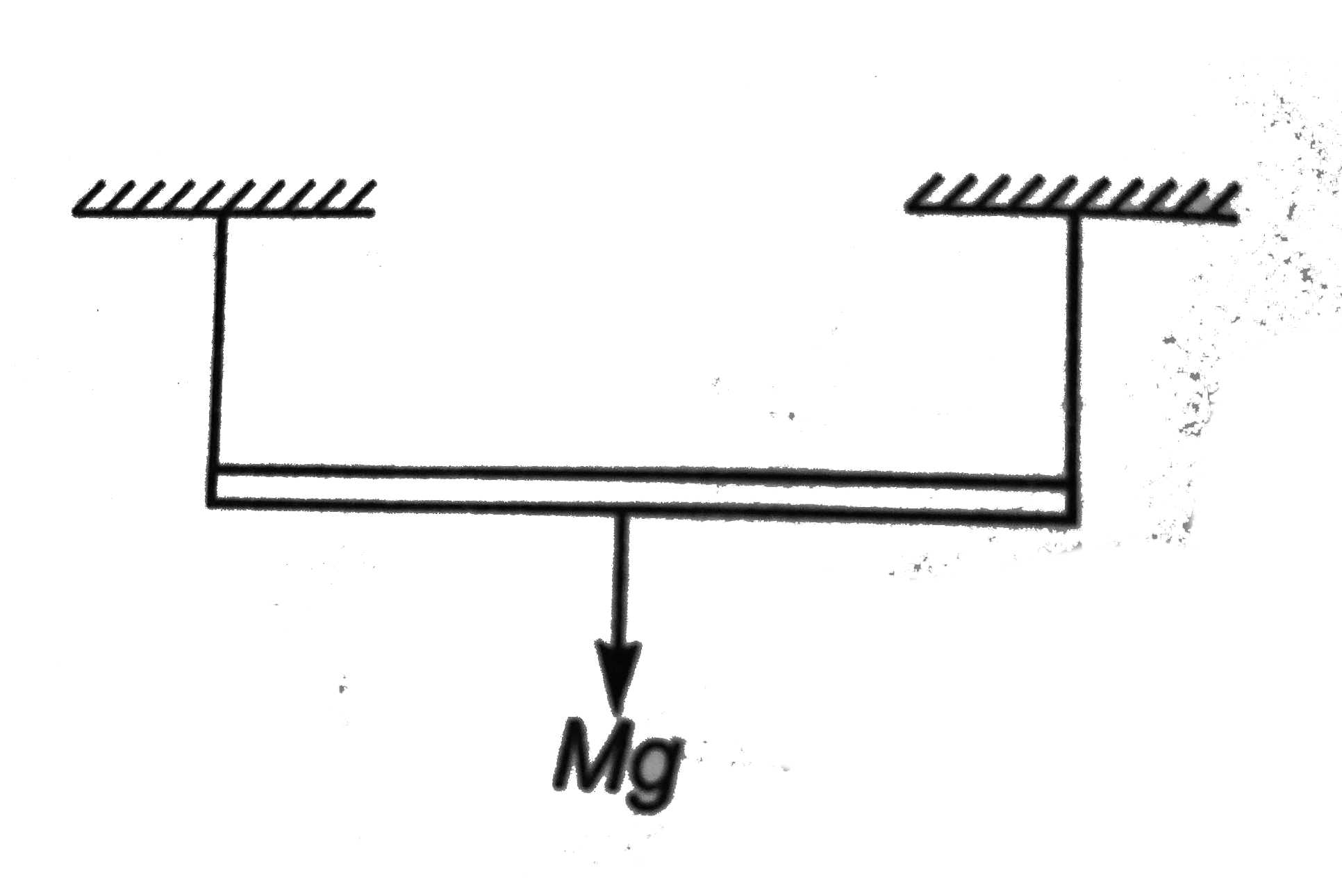
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.Aremains unaffectedBincreaseCdecreaseDbecome equal to weight of the rod.

A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.
View SolutionA particle of mass m=2 kg is suspended in a vertical plane by two strings - one horizontal and the other inclined to the vertical by α=30∘. Find the tension of the inclined string (a) an instant before the horizontal one is cut, (b) an instant after the horizontal one is cut.
View SolutionA uniform rod of mass m length l is attached to smooth hinge at end A and to a string at end B as shown in figure. It is at rest. The angular acceleration of the rod just after the string is cut is :

View SolutionA uniform rod of mass m and length L is kept on a horizontal table with L4 length on the table as shown in figure. As the string attached to the end B is cut, the angular acceleration of the rod about point C will be
_PHY_E05_242_Q01.png)
View SolutionA uniform rod of mass m length l is attached to smooth hinge at end A and to a string at end B as shown in figure. It is at rest. The angular acceleration of the rod just after the string is cut is :

View Solution
