Standing waves are established on a string of length such that is a node and is an immediate anti node. Oscillation amplitude of point is . Let be the oscillation amplitude for point . Pick the suitable option (s) for correct value of and the possible equation (s) for standing waves on the string.

The correct Answer is:C, D
and
Also:
Topper's Solved these Questions
- TEST PAPERSBook:FIITJEEChapter:TEST PAPERSExercise:PHYSICS
Explore 747 Videos
- SIMPLE HARMONIC MOTIONBook:FIITJEEChapter:SIMPLE HARMONIC MOTIONExercise:Assignment Problems (Objective) (Level-II)
Explore 20 Videos
- WORK, ENERGY AND POWER Book:FIITJEEChapter:WORK, ENERGY AND POWER Exercise:COMPREHENSIONS ( Comprehension-II)
Explore 5 Videos
Similar Questions
A standing wave is created on a string of length 120m and it is vibrating in 6th harmonic. Maximum possible amplitude of any particle is 10cm and maximum possible velocity will be 10cm/s. Choose the correct statement.
View SolutionTwo waves are generated on a string of length 4.0 m to produce a three-loop standing wave with an amplitude of 1.0 cm.The wave speed is 100 m/s. Let the equation for one of the waves be of the form y(x,t)=ymsin(kx+ωt). In the equation for the other wave, what are (a) ym (b) k, (c) ω and (d) the sign in front of w!
View Solution
Knowledge Check
A standing wave is created on a string of length 120m and it is vibrating in 6th harmonic. Maximum possible amplitude of any particle is 10cm and maximum possible velocity will be 10cm/s. Choose the correct statement.
AAngular wave number of two waves will be π20BTime period of any particle's SHM will be 4πsec.CAny particle will have same kinetic energy as potential energyDAmplitude of interfering waves are 10cm each.A wave in a string has an amplitude of 2cm. The wave travels in the +ve direction of x axis with a speed of 128ms−1 and it is noted that 5 complete waves fit in 4m length of the string. The equation describing the wave is
Ay=(0.02)msin(15.7x−2010t)By=(0.02)msin(15.7+2010t)Cy=(0.02)msin(7.85x−1005t)Dy=(0.02)msin(7.85x+1005t)Two strings made of same material, same cross-section but different lengths are fixed at both ends and excited in their respective fundamental modes such that total oscillation modes such that total oscillation energy is the same in both cases. Let f1,f2 be the oscillation frequencies and A1,A2 be the amplitudes of oscillation of the antinodes in the two cases. Assuming both strings to be under same tension, pick the correct option (s)
 Af1f2=14BA1A2=12Cf1f2=41DA1A2=21
Af1f2=14BA1A2=12Cf1f2=41DA1A2=21
Figure shows the standing waves pattern in a string at t = 0. Find out the equation of the standing wave where the amplitude of antinode is 2A.
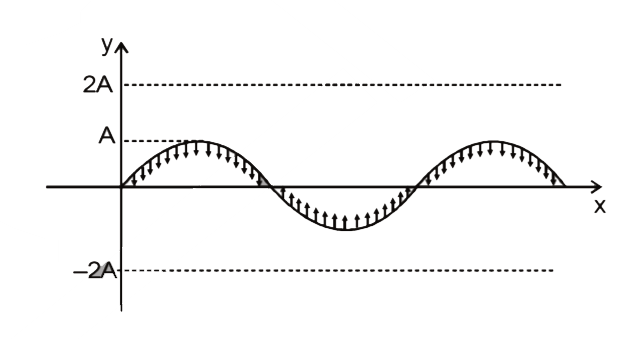
View SolutionAdjacent antinodes of a standing wave on a string are 15.0 cm apart. A particle at an antinode oscillates in simple harmonic motion with amplitude 0.850 cm and period 0.0750 s. The string lies along the +x-axis and is fixed at x=0.
(a) find the displacement of a point on the string as a function of position and time.
(b) Find the speed of propagation of a transverse wave in the string.
(c) Find the amplitude at a point 3.0 cm to the right of an antinode.
View SolutionAdjacent antinodes of a standing wave on a string are 15.0 cm apart. A particle at an antinode oscillates in simple harmonic motion with amplitude 0.850 cm and period 0.0750 s. The string lies along the +x-axis and is fixed at x=0.
(a) find the displacement of a point on the string as a function of position and time.
(b) Find the speed of propagation of a transverse wave in the string.
(c) Find the amplitude at a point 3.0 cm to the right of an antinode.
View SolutionIn Fig. 16-45, a string, tied to a sinusoidal oscillator at P and running over a support at Q, is stretched by a block of mass m. The separation L between P and Q is 1.20 m, and the frequency f of the oscillator is fixed at 120 Hz. The amplitude of the motion at P is small enough for that point to be considered a node. A node also exists at Q. A standing wave appears when the mass of the hanging block is 286.1 g or 4470g, but not for any intermediate mass. What is the linear density of the string?
View SolutionA standing wave arises on a string when two waves of equal amplitude , frequency and wavelength travelling in opposite superimose. If the frequency of oscillation of the standing waves
View Solution
