Question

Two Earth's satellites move in a common plane along circular obrits. The orbital radius of one satellite is r while that of the other satellite is ${\displaystyle r-\Delta r(\text{Here}\Delta r<<r)}$

Options

A. Time interval separates the periodic approaches of the satellites to each other over the minimum distance is `(4pir^(5//2))/(3(GM)^(1//2)Deltar)`
B. Time interval separates the periodic approaches of the satellites to each other over the minimum distance is `(2pi^(5//2))/(3(GM)^(1//2)Deltar)`
C. Angular velocity of approach between two setellites is `(3(GM)^(1//2)Deltar)/(r^(5//2))`
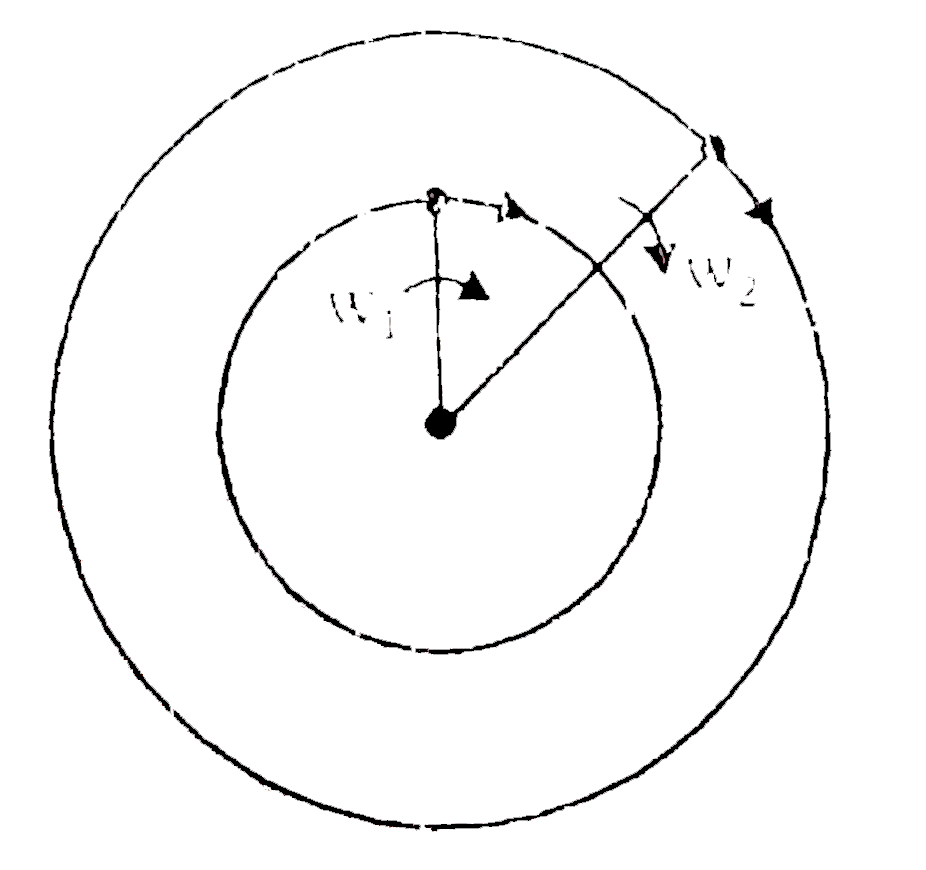
D. Angular velocity of approach between two setellites is `(3(GM)^(1//2)Deltar)/(2r^(5//2))`

Answer

A::D

Explanation

`w_(1)=(GM)^(1//2)/(r-Deltar)^(3//2) " " w_(2)=(GM)^(1//2)/(r^(3//2))`

`r_(2)=r`
`r_(1)=r-Deltar`
Angular velocity of approach `=w_(1)-w_(2)`
`=(GM)^(1//2)[(r^(3//2)-(r-Deltar)^(3//2))/(r^(3//2)(r-Deltar)^(3//2))]`
`=(GM)^(1//2)xx([1-(1-(3Delta r)/(2r))])/(r^(3//2)(1-(Delta r)/(r ))^(3//2))`
`=(3(GM)^(1//2)Deltar)/(2r^(5//2))`
The period of approach
`= (2pi)/(w_(1)-w_(2)) = (4pir^(5//2))/(3(GM)^(1//2)Deltar)`