Construct a parallelogram PQRS such that PQ=5.2cm, PR=6.8cm and QS=8.2cm.
To construct the parallelogram PQRS with the given dimensions PQ=5.2cm, PR=6.8cm, and QS=8.2cm, follow these steps:
Step-by-Step Construction:
1. Draw the Base:
- Draw a line segment QS measuring 8.2cm.
- Label the endpoints as Q and S.
Hint: Use a ruler to ensure the line segment is straight and accurately measures 8.2cm.
2. Draw an Arc from Point Q:
- With point Q as the center, draw an arc with a radius of 5.2cm.
- This arc will represent all possible locations for point P.
Hint: Keep the compass point at Q and adjust the other end to 5.2cm before drawing the arc.
3. Draw an Arc from Point S:
- With point S as the center, draw another arc with the same radius of 5.2cm.
- This arc will intersect the previous arc.
Hint: Make sure the compass remains at S and adjust it to 5.2cm again before drawing.
4. Locate Point P:
- Mark the intersection point of the two arcs as point P.
Hint: Ensure that the intersection is clear and marked accurately.
5. Draw an Arc from Point P:
- With point P as the center, draw an arc with a radius of 6.8cm.
- This arc will help locate point R.
Hint: Measure 6.8cm carefully on the compass before drawing the arc.
6. Draw an Arc from Point Q:
- With point Q as the center, draw another arc with a radius of 6.8cm.
- This arc will intersect the previous arc drawn from point P.
Hint: Ensure the compass is set to 6.8cm before drawing the arc from Q.
7. Locate Point R:
- Mark the intersection of the two arcs as point R.
Hint: Confirm that the intersection point is distinct and labeled clearly.
8. Complete the Parallelogram:
- Finally, connect points P to R and S to R to complete the parallelogram PQRS.
Hint: Use a ruler to draw straight lines between the points to ensure accuracy.
Final Result:
You have successfully constructed the parallelogram PQRS with the specified dimensions.
- Draw a line segment QS measuring 8.2cm.
- Label the endpoints as Q and S.
- With point Q as the center, draw an arc with a radius of 5.2cm.
- This arc will represent all possible locations for point P.
- With point S as the center, draw another arc with the same radius of 5.2cm.
- This arc will intersect the previous arc.
- Mark the intersection point of the two arcs as point P.
- With point P as the center, draw an arc with a radius of 6.8cm.
- This arc will help locate point R.
- With point Q as the center, draw another arc with a radius of 6.8cm.
- This arc will intersect the previous arc drawn from point P.
- Mark the intersection of the two arcs as point R.
- Finally, connect points P to R and S to R to complete the parallelogram PQRS.
You have successfully constructed the parallelogram PQRS with the specified dimensions.
Topper's Solved these Questions
Similar Questions
A, B, C, D are mid-points of sides of a parallelogram PQRS. If ar (PQRS)=36cm2, then ar(ABCD)= 24 cm2 (b) 18 cm2 (c) 30 cm2 (d) 36 cm2
View SolutionIn PQR , M and N are points on sides PQ and PR respectively such that PM=15cm and NR=8cm . If PQ=25cm and PR=20cm state whether MNQR .
View SolutionIn Fig. 4.238, S and T are points on the sides PQ and PR respectively of PQR such that PT=2cm , TR=4cm and ST is parallel to QR . Find the ratio of the areas of PST and PQR . (FIGURE)
View SolutionABCD is a parallelogram. P is a point on AD such that AP=13 AD and Q is a point on BC such that: CQ=13BC. Prove that AQCP is a parallelogram.
View SolutionIn Figure, PSDA is a parallelogram in which PQ=QR=RS and APBQCR. Prove that ar ( PQE)= ar ( CFD).
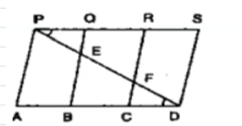 View Solution
View SolutionM and N are points on the sides PQ and PR respectively of a PQR . For each of the following cases, state whether MNQR : PM=4cm, QM=4.5cm, PN=4cm, NR=4.5cm (ii) PQ=1.28cm, PR=2.56cm, PM=0.16cm, PN=0.32cm
View SolutionPQ and RS are two parallel chords of a circle whose centre is O and radius is 10 cm. If PQ=16 cm and RS=12 cm, find the distance between PQ and RS, if they lie: on opposite side of the centre O
View SolutionPQ and RS are two parallel chords of a circle whose centre is O and radius is 10 cm. If PQ=16 cm and RS=12 cm, find the distance between PQ and RS, if they lie: on the same side of centre O
View SolutionIn Fig. 4.177, ACBAPQ . If BC=10cm, PQ=5cm, BA=6.5cm and AP=2.8cm find CA and AQ . Also, find the area (ACB):area (APQ) . (FIGURE)
View SolutionPQRS is a parallelogram. PXandQY are respectively, the perpendiculars from PandQ to SRandRS produced. Prove that PX=QY
View Solution

