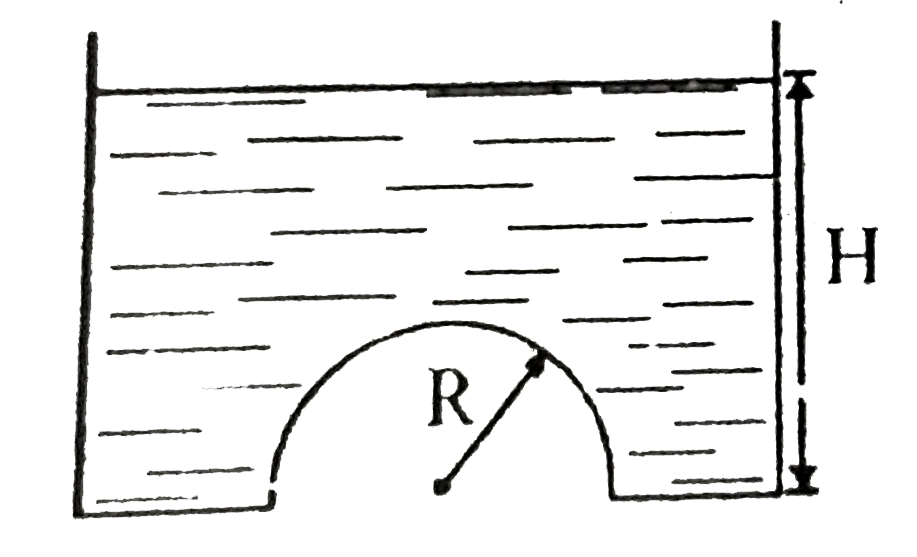
A hole in the horizontal bottom of the container is closed by a hemispherical cap of radius R (see figure) The vessel is filled with liquid of density d. Bottom is at a depth of H. Find the force with which the cap presses down on the bottom of the vessel. Neglect atmospheric pressure. If the force is api N, fill 'a' in the OMR sheet (Take d=103kg/m3,H=1m,R=30cm)
The correct Answer is:0720
(πR2H−23πR3)×d×g
πR2[H−23R]×104
=π×0.09×104[1−23×0.3]
π×900×0.8=720π
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic70videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective131videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
The manifestation of band structure in solids is due to
03:11View SolutionThe quantity// quantities that does //do not have mass in its// their dimentions (when we take standard 7 quantities as fundmental) is//are:
01:27View SolutionA cylinder of height 20m is completely filled with water. The velocity of effux of water (∈ms−1) through a small hole on the side wall of the cylinder near its bottom is
09:27View SolutionHow does the width of the depletion layer of a p-n junction diode change with decrease in reverse bias?
04:56View SolutionTo what height h should a cylindrical vessel of diameter d be filled with a liquid so that the total force on the vertical surface of the vessel be equal to the force on the bottom-
02:38View SolutionSome liquid is filled in a cylindrical vessel of radius R. Let F_(1) be the force applied by the liquid on the bottom of the cylinder. Now the same liquid is poured into a vessel of uniform square cross-section of side R. Let F_(2) be the force applied by the liquid on the bottom of this new vessel. (Neglect atmosphere pressure). Then
View Solutionएक बेलनाकार पात्र को समांग (homogeneous) द्रव्य से कितनी ऊँचाई तक भरें कि जिस बल से द्रव्य बल से द्रव्य पात्र की दीवारों को दबाता है, उतने ही बल से पात्र की तली को दबाये ?
04:03View SolutionA cylindrical vessel of radius r is filled with a homogeneous liquid upto a height h. It is found that the force exerted by the liquid column on the bottom of the cylinder is equal to the force exerted by the liquid on the sides of the cylinder. What is the relation between r and h?
04:07View SolutionThe bottom of a cylindrical vessel has a circlular hole of radius r and at depth h below the water] level. If the diameter of the vessel is D, the find then speed with which the water level in the] vessel drops.
02:50View SolutionThe radius of a funnel-shaped vessel r | As the container is filled with liquid up to h height, the force at the bottom of the container and the force applied at the sides are equal. In this case
02:43View SolutionThe height of which a cylindrical vessel of radius R be filled with a homogeneous liquid to make the force with which the liquid presses on the side of the vessel equal to the force exerted by the liquid on the bottom of vessel is
01:37View SolutionTo what height should a cylindrical vessel be filled with a homogenous liquid to make to force on bottom equal to force on side? (R = radius of vessel )
02:18View SolutionIf a cylindrical vessel with a hole of r radius in the bottom is placed at a depth h above the water level. If the diameter of the vessel is D then the speed of the water level falling in the vessel will be
04:00View SolutionSome liquid is filled in a cylindrical vessel of radius R. Let F1 be the force applied by the liquid on the bottom of the cylinder. Now the same liquid is poured into a vessel of uniform square cross-section of side R. Let F2 be the force applied by the liquid on the bottom of this new vessel. (Neglect atmosphere pressure). Then
View SolutionA similar shield vessel is placed as per the picture. The vessel is filled with a liquid whose density is 900 kg / m. There will be a force due to the fluid on the bottom of the vessel.
01:47View Solution

