A small coin is placed on a stationery horizontal disc at a distance r from its centre. The disc starts rotating about a fixed vertical axis through its centre with a constant angular acceleration α. Determine the number of revolutions N, accomplished by the disc before the coin starts slipping on the disc. Find the coefficient of static friction between the coin and the disc is μ9.
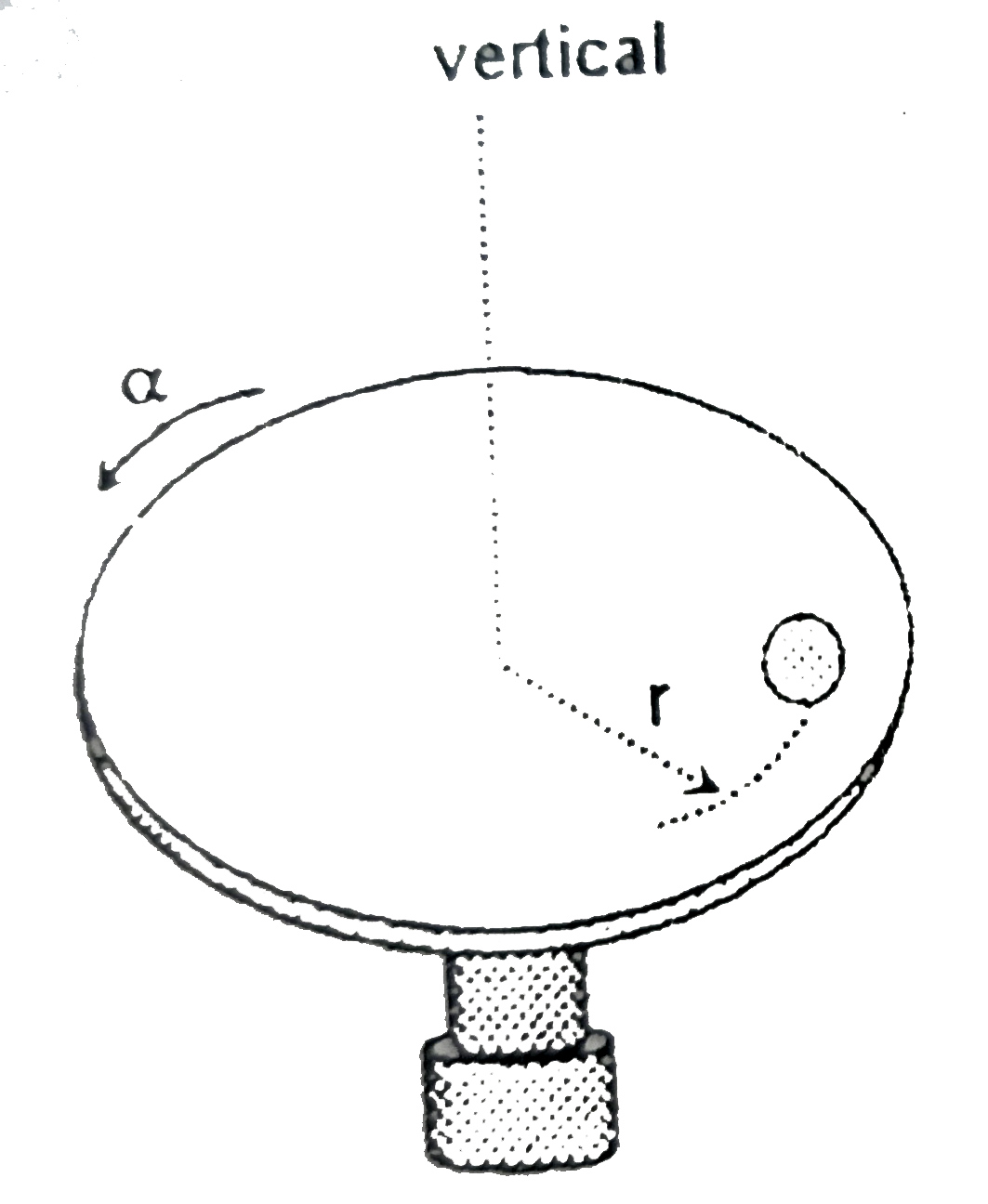
we draw the FBD of the coin
fT= tangential component of frictional force on coin
fR= radial component of frictional force on coin s
fT=mαr
fr=mω2fr
√f2R+f2T≤μgmg
2πN=ω2f2α
Solving the equations, we get
N=(μggr−α2)1/24πα
Topper's Solved these Questions
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Objective problems
Explore 215 Videos
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Assertion & Reason
Explore 15 Videos
- GENERAL PHYSICSBook:FIITJEEChapter:GENERAL PHYSICSExercise:MATRIX MATCH TYPE
Explore 3 Videos
- GRAVITATIONBook:FIITJEEChapter:GRAVITATIONExercise:Numerical based Question
Explore 2 Videos
Similar Questions
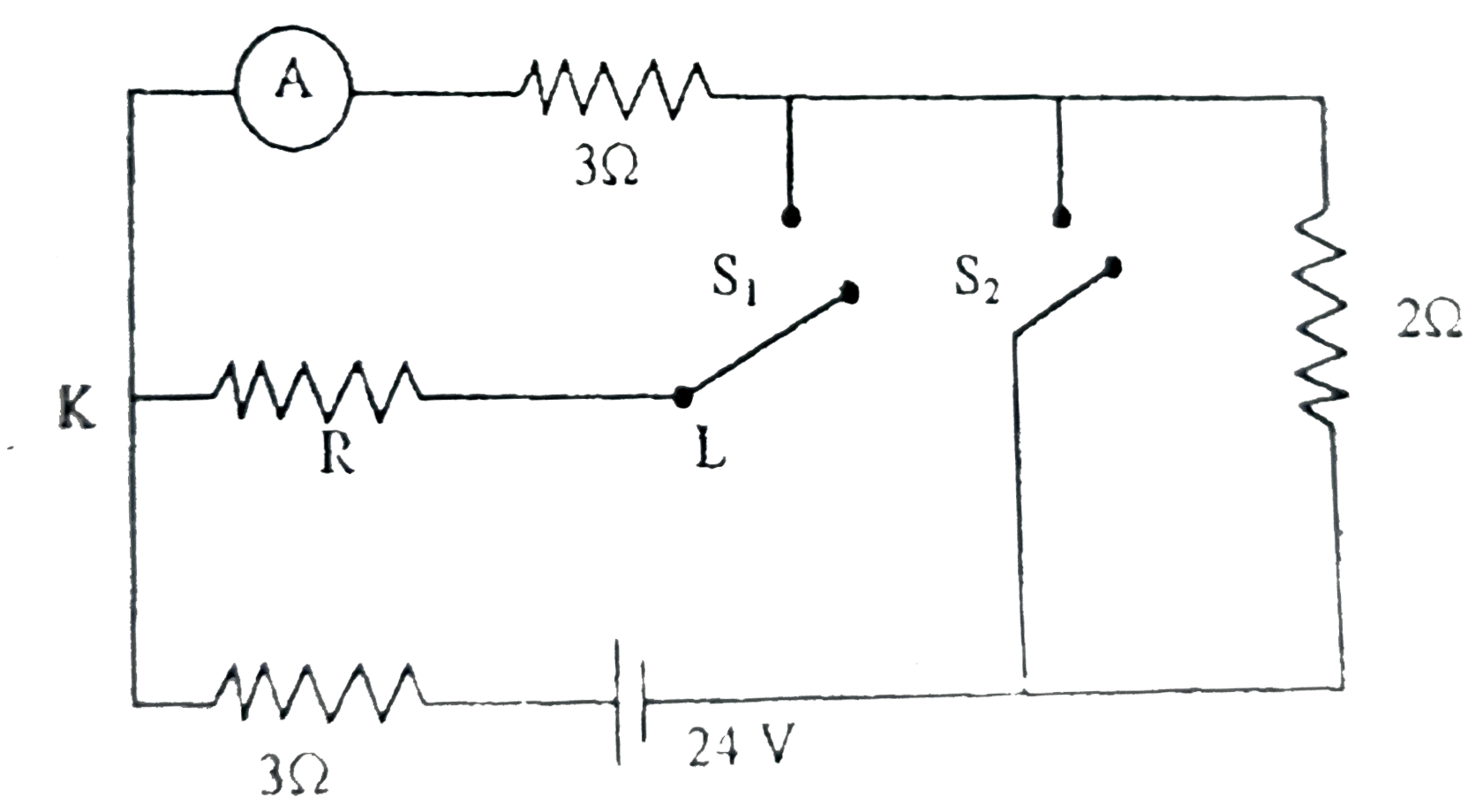
In the circuit shown in the figure, the ideal ammeter reading for current is taken
(a) with both switches open
(b) with both switches closed.
The readings are same in the cases. The value of resistance R is
View SolutionStatement-1 : When an ideal gas is freely expanded change in internal energy of gas is zero, where as when real gas is expanded freely it's internal energy decreases.
Statement-2 : There is no intermolecular force of attraction in ideal gas.
View SolutionTwo resistors along with errors are kown as
R1=(30±3)Ω
R2=(60±3)Ω
column - I lists four alagebratic functions of R1R2 column -II lists corresponding errors where as column -III lisits the absolute errors

pick the correct option
View SolutionA particle is projected radially outwards from the surface of a planet with an initial speed of 4.0km/s. Maximum height attained by the particle is h=900km. Find the radius of the planet taking g=10m/s2.
View SolutionA disc of radius R rotates from rest about a vertical axis with a constant angular acceleration such that a coin placed with a tangenitial between a as shown in figure If the coefficient of static friction between the coin and disc is mu_(s) .Find the velocity of the before it start sliding relative as the disc
View SolutionA small coin of mass 80g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration alpha=2rad//s^(2) . The coefficient of static friction between the coin and the disc is mu_(s)=3//4 and cofficient of kinetic friction is mu_(k)=0.5 . The coin is placed at a distance r=1m from the centre of the disc. The magnitude of the resultant force on the coin exerted by the disc just before it starts slipping on the disc is
View SolutionA coin is placed on the horizontal surface of a rotation disc. The distance of the coin from the axis is 1 m and coefficient of friction is 0.5. If the disc starts from rest and is given an angular acceleration 1√2 rad /sec2, the number of revolutions through which the disc turns before the coin slips is
View SolutionA coin placed on a horizontal rotating disc, with its centre at 10 cm from the centre of the disc, is about to slip off when the disc performs 60 rpm. Find the coefficient of friction between the coin and the disc.
View SolutionA coins kept on a horizontal rotating disc has its centre at a distance of 0.25 m from the axis of rotation of the disc .If μis0.2, then the angular velocity of the disc at which the coion will slip off, (g=9.8m/s2)
View SolutionA coin is placed at the edge of a horizontal disc rotating about a vertical axis through its axis with a uniform angular speed 2 rad s^(-1) . The radius of the disc is 50 cm. Find the minimum coefficient of friction between disc and coin so that the coin does not slip. (Take g = 10 m//s^(2) )
View SolutionA small coin of mass 40 g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration alpha="2 rad s"^(-2) . The coefficient of static friction between the coin and the disc is mu_(s)=3//4 and the coefficient of kinetic friction is mu_(k)=0.5. The coin is placed at a distance r=1m from the centre of the disc. The magnitude of hte resultant force on the coin exerted by the disc just before it starts slipping on the disc is :
View SolutionA coin kept on a horizontal rotating disc has its Centre at a distance of 0.1 m from the axis of the rotation of the disc. If the coefficient of friction between the coin and disc is 0.25, find the angular speed of the disc at which the coin would be about is slip off.
View SolutionA coin kept on a horizontal rotating disc has its centre at a distance of 0.25 cm from the axis of rotation of the disc. If mu is 0.2. What is the angular velocity of the disc at which the coin is about to slip off ?
View SolutionA disc of radius R rotates from rest about a vertical axis with a constant angular acceleration such that a coin placed with a tangenitial between a as shown in figure If the coefficient of static friction between the coin and disc is mu_(s) .Find the velocity of the before it start sliding relative as the disc
View SolutionA disc of radius R rotates from rest about a vertical axis with a constant angular acceleration such that a coin placed with a tangenitial between a as shown in figure If the coefficient of static friction between the coin and disc is μs .Find the velocity of the before it start sliding relative as the disc

View Solution
