Any two points on the circumference of uniform circular conductor are connected to the terminals of a cell. Show that, the resultant magnetic field at the centre of the circle is zero.Solution in Bengali
In the figure a circular conductor of radius a is shown.
There are two points, Z,Y on the circumference and O is centre of the circle .
Let I1andI2 be the currents flowing through the parts XMY and XNY respectively of the circular conductor. Let XMY and XNY subtend angles θ1andθ2 respectively at the centre.
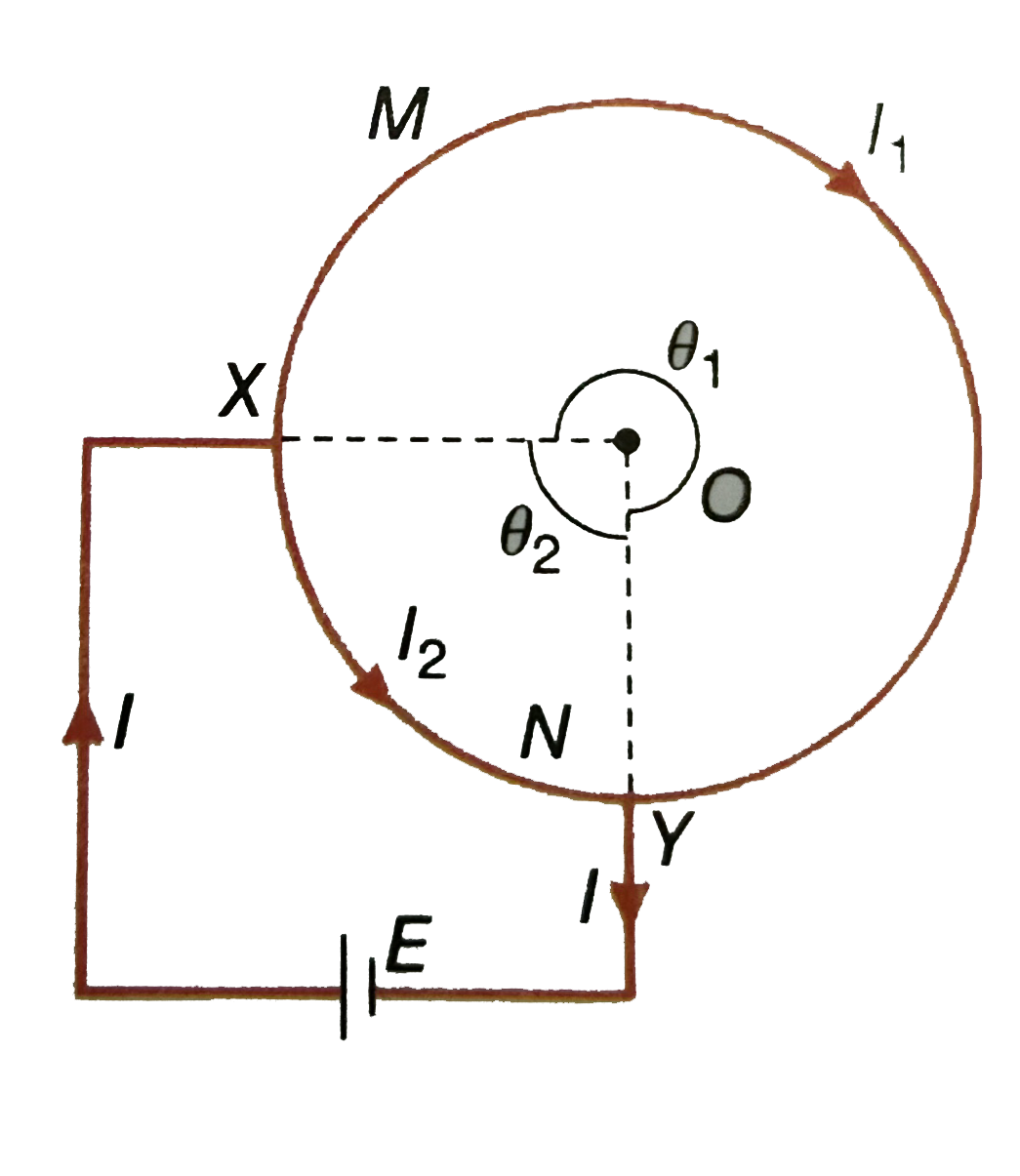
Now magnetic field intensity at O due to part XMY,
BXMY=μ04π⋅I1aθ1 ....(1)
ans similarly for the part XNY,
BNXY=μ04π⋅I2aθ2 ....(2)
BMXY is directed into the plane of the conductor, while BXNY is directed out of the plane of the conductor.
If r resistance per unit lenght of the conductor pern unit lenght of the conductor then resistance of the part XMY and XNY are given by R1=(aθ1)randR2=(aθ2)r resepctively.
As potential differences across R1andR2 are equal
∴VXMY=VXNY
or I1R1=I2R2or,I1(aθ1)r=I2(aθ2)r
∴I1θ1=I2θ2
From equation (1) and (2) if follows that magnetic fields B1andB2 due to the two parts of the circular conductor are equal and opposite. Hence the magnetic field at the centre of the circular conductor is zero.
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETISM EXERCISE - NCERT TEXTBOOK QUESTIONS WITH ANSWER HINT25videos
ELECTROMAGNETISM
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETISM EXERCISE - NCERT EXAMPLAR QUESTIONS WITH ANSWER HINT (MCQ-1)2videos
ELECTROMAGNETISM
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETISM EXERCISE - SECTION RELATED QUESTIONS64videos
ELECTROMAGNETISM
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - CBSE SCANNER17videos
ELECTROMAGNETIC WAVES
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELEMENTARY PHENOMENA OF ELECTROSTATICS EXERCISE - EXAMINATION ARCHIVE2videos
ELEMENTARY PHENOMENA OF ELECTROSTATICS
Similar Questions
Two moving coils meters M1andM2 have the following particulars :
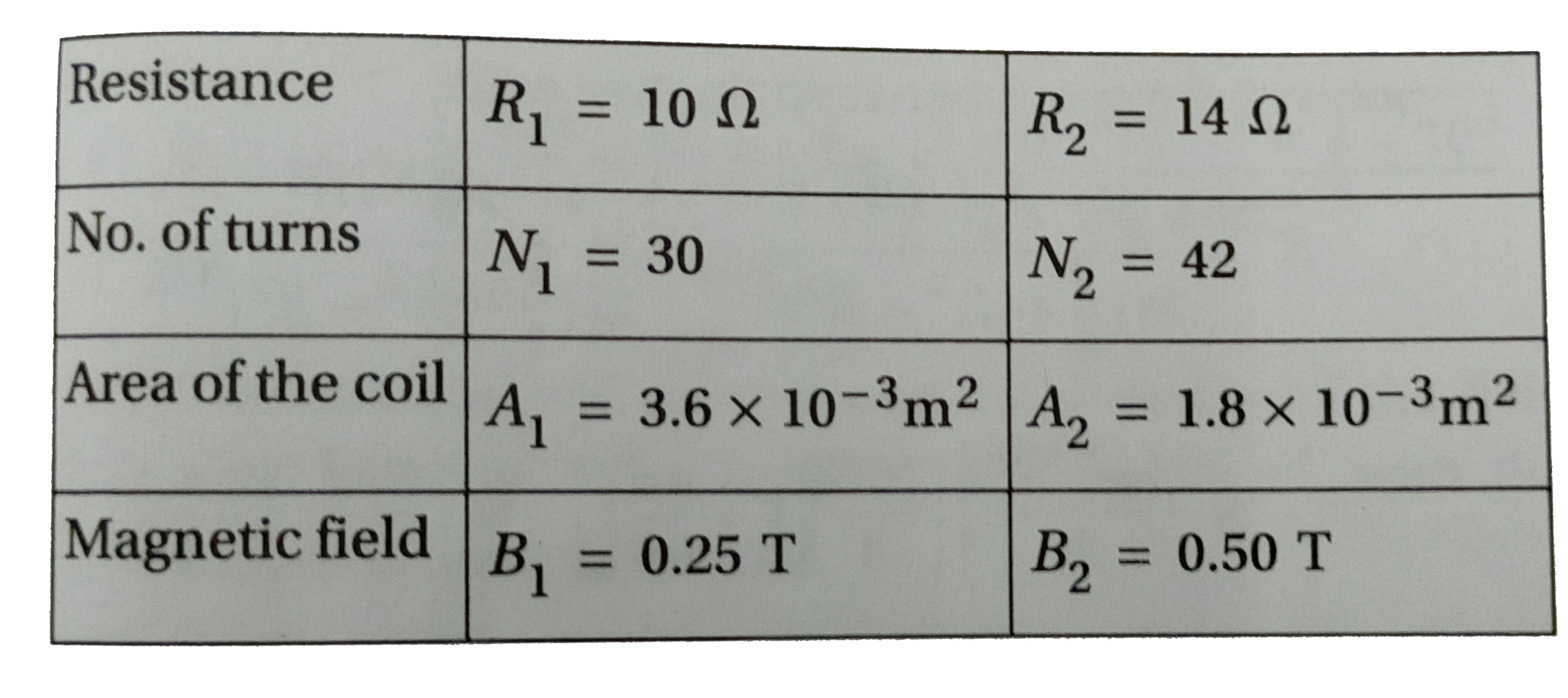
(The spring constancs are identical for the two meters ).02:54View SolutionTwo charged particles traverses indentical helical paths in a completely opposite sense in a uniform magnetic field, →B=B0ˆK.
05:44View SolutionWhat are the fundamental properties of a magnet ?
04:05View SolutionWhat are the directions of electric and magnetic field vectors relative to each other and relative to the direction of propagation of electromagnetic waves?
View SolutionDefine surface density of electric charge
06:50View SolutionAn electron moves in a circular orbit with a uniform speed v.It produces a magnetic field B at the centre of the circle. The radius of the circle is proportional to
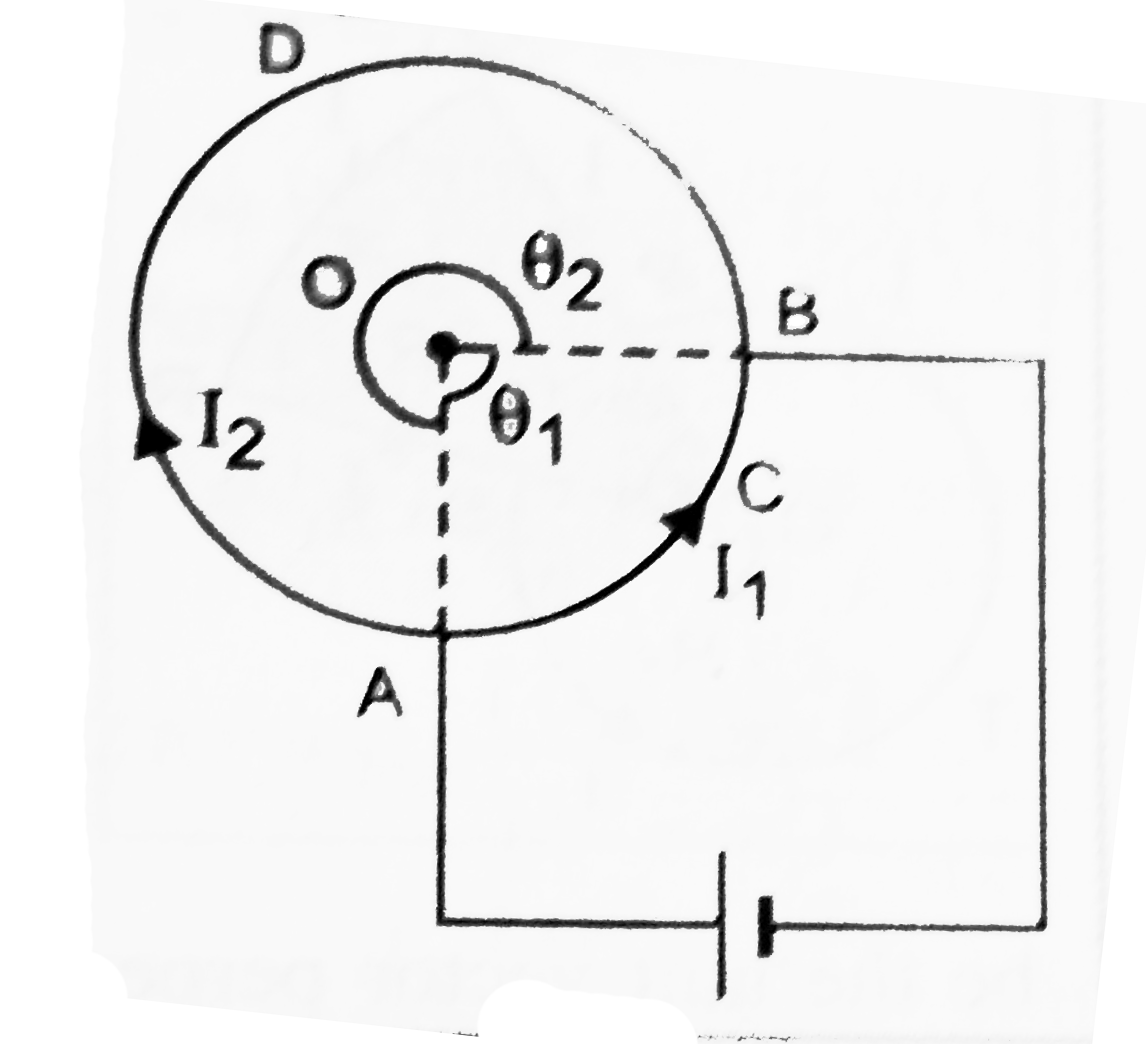
07:47View SolutionAs shown in figure a cell is connected across two points A and B of a uniform circular conductor of radius r. Prove that the magnetic field induction at its centre O will be zero.
 08:53View Solution
08:53View SolutionA cell is connected across two points P and Q of a uniform circular conductor, figure. Prove that the magnetic field at its centre O will be zero.
03:33View SolutionTwo long straight conductors are connected radially to two arbitary points A and B of a circular conductor. Calculate the magnetic field at the center of the coil.
05:44View SolutionA circular conductor of uniform resistance per unit length, is connected to a battery of 4 V. The total resistance of the conductor is 4ω. The net magnetic field at the centre of the conductor is
 View Solution
View Solutionएक समान वृतीय चालक के किन्ही दो बिन्दयों A व B कोई एक सेल से जोड़ा गया है सिद्ध किजिए की केंद्र O पर चुम्कीय क्षेत्र शून्य होगा।
View Solutionएक वृत्तीय चालक की परिधि के किंही दो बिंदुओं के बीच एक सेल जोड़ दिया जाता है । चालक के केंद्र पर चुम्बकीय क्षेत्र की गणना कीजिए ।
View SolutionCompare the ratio of magnetic field produced at the centre of a circular conductor and a point on the axis of a conductor.
06:35View SolutionAs shown in figure a cell is connected across two points A and B of a uniform circular conductor of radius r. Prove that the magnetic field induction at its centre O will be zero.
 04:32View Solution
04:32View SolutionA cell is connected between two points of a uniformly thick circular conductor and i1andi2 are the currents flowing in two parts of the circular conductor of radius a The magnetic field at the centre of the loop will be
02:10View Solution

