What impulse need to be given to a body of mass m, released from the surface of earth along a straight tunnel passing through centre of earth, at the centre of earth, to bring it to rest. (Mass of earth M, radius of earth R)

m√GMR
√GMmR
m√GM2R
zero
The correct Answer is:A
Conservation of mechanical energy gives,
12mv2=m[−GMR+3GM2R]=GMm2R
∵ increase in KE = decrease in PE) or v=√GMR
Momentum, mv=m√GMR
∴ Impulse required =√GMR.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (B) Chapter Exercises31videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Check Point 10.620videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE11videos
KINEMATICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The mass of a body at the centre of the earth is
AinfiniteBzeroCsame as at a other placesDgreater than at poles - Question 2 - Select One
The kinetic energy needed to project a body of mass m from the surface of earth (radius R ) to infinity is
AmgR4B2mgRCmgRDmgR4 - Question 3 - Select One
A body of mass m rises to a height h=R/5 from the surface of earth. If g is the acceleration due to gravity at the surface of earth, the increase in potential energy is (R = radius of earth)
A45mgRB56mgRC67mgRDmgR
The height from the surface of earth at which the gravitational potential energy of a ball of mass m is half of that at the centre of earth is (where R is the radius of earth)
View SolutionA body of mass m is lifted from the surface of earth of height equal to R/3 where R is the radius of earth, potential energy of the body increases by
View SolutionA body of mass m is lifted up from the surface of earth to a height three times the radius of the earth R. The change in potential energy of the body is
View SolutionIf 'g' is the potential energy of an object of mass 'm' raised from the surface of the earth to a height equal to the radius 'R' of the earth is
View SolutionA ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
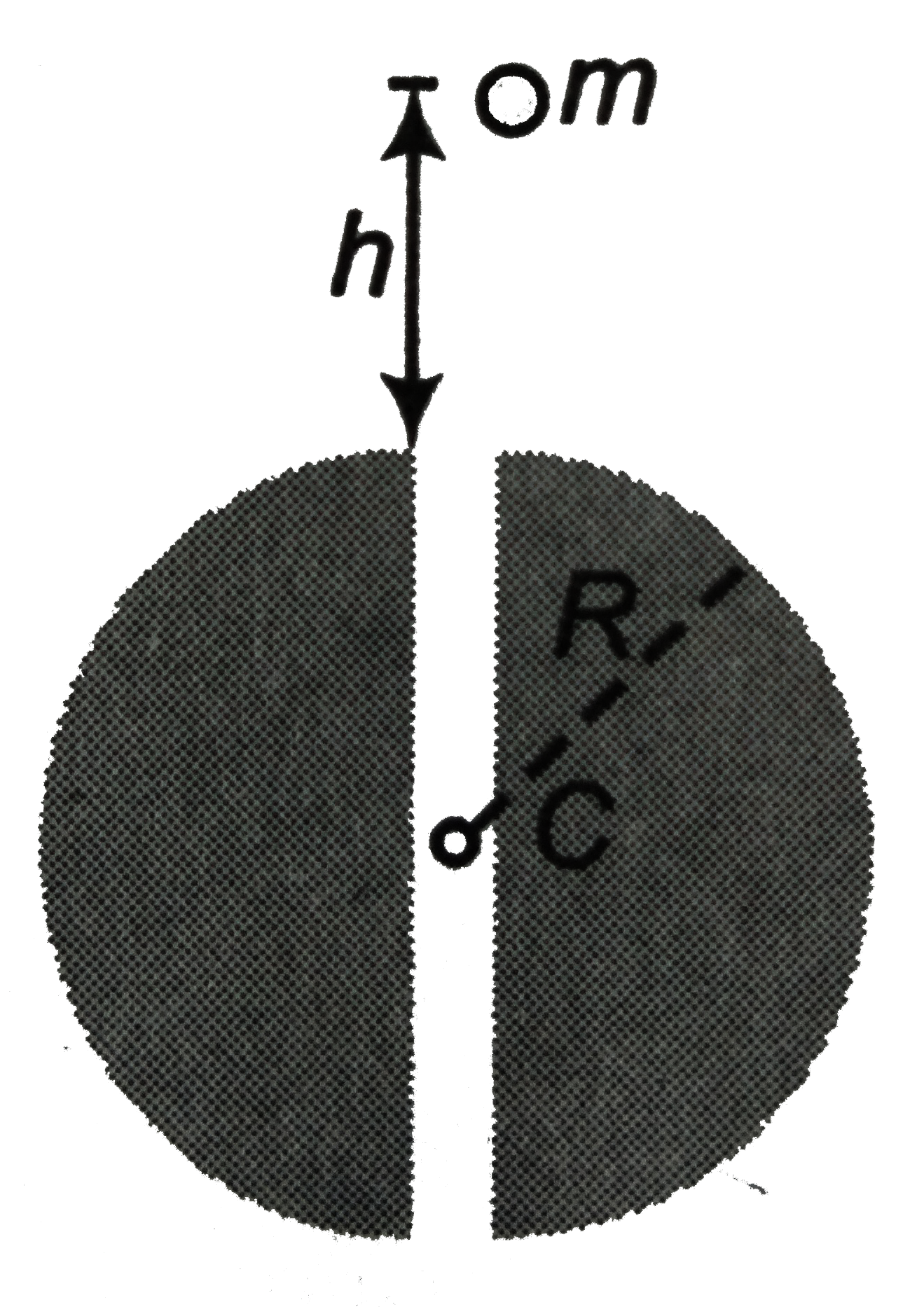 View Solution
View Solution

