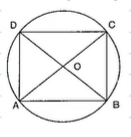
ACandBD are chords of a circle which bisect each other. Prove that (i) ACandBD are diameters (ii) ABCD is a rectangle.
(i) It is given that AB and CD are the two chords of a circle
Let the point of intersection be O.
Join AB,BC,CD and AD.
In triangles AOB and COD
,
∠AOB=∠COD ...(Vertically opposite angles)
OB=OD ....(O is the mid-point of BD)
OA=OC ....(O is the mid-point of AC)
△AOB≅△COD ....SAS test of congruence
∴AB=CD ....c.s.c.t.
Similarly, we can prove
△AOD≅△BOC,
then we get
AD=BC ....c.s.c.t.
So, □ABCD is a parallelogram, since opposite sides are equal in length.
So, opposite angles are equal as well.
So, ∠A=∠C
Also, for a cyclic quadrilateral opposite angles add up to 180∘So,
∠A+∠C=180∘
∠A+∠A=180∘
∠A=90∘
So, BD is the diameter. Similarly, AC is also the diameter.
(ii) Since AC and BD are diameters,
∴∠A=∠B=∠C=∠D=90∘ ...Angle inscribed in a semi circle is a right angle
Hence, parallelogram ABCD is a rectangle.
Topper's Solved these Questions
Similar Questions
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters (ii) ABCD is a rectangle
View SolutionACandBD are chords of a circle which bisect each other. Prove that (i) ACandBD are diameters (ii) ABCD is a rectangle.
View SolutionAC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD arediameters, (ii) ABCD is a rectangle
View SolutionACandBD are chords of a circle that bisect each other. Prove that:AC and BD are diameter. ABCD is a rectangle
 View Solution
View SolutionIf a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
View SolutionIf a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
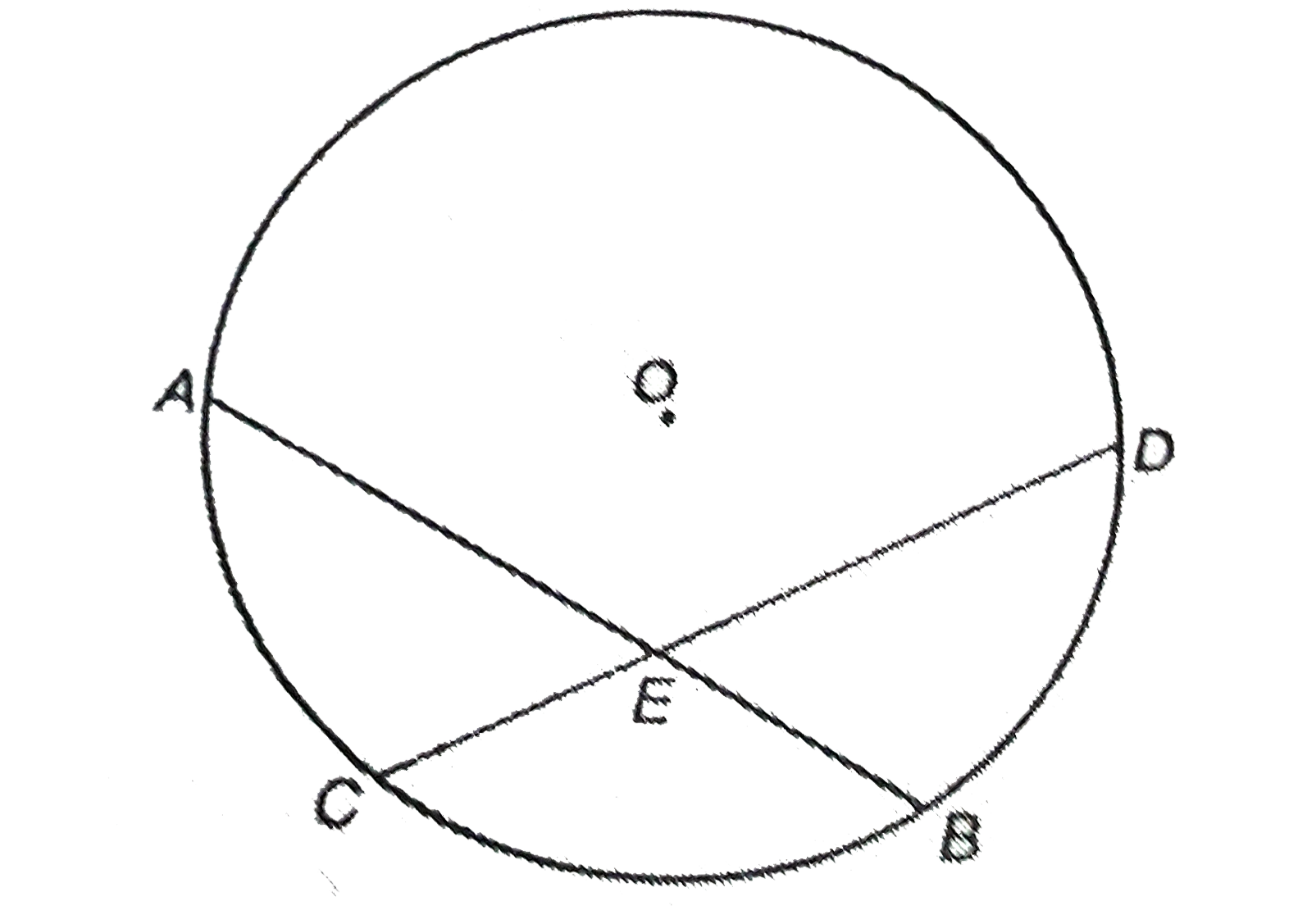
View SolutionIn the adjoining figure. If two equal chords AB and CD of a circle intersect each other at E. then prove that chords AC and DB are equal.
 View Solution
View SolutionIn Figure, two chords AB and CD intersect each other at the point P. Prove that:
(i) ΔAPC~ΔDPB
(ii) AP⋅PB=CP⋅DP
 View Solution
View SolutionProve that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
View SolutionIf AB, BC and CD are equal chords of a circle with O as centre and AD diameter, then ∠AOB= 600 (b) 900 (c) 1200 (d) none of these
View Solution

