A straight conductor is split into indentical semicircular turns by tearing it into two parts from within. A current enters at one end and after passing through two parts leaves at the other, the field at the centre of the loop of radius r is :
To find the magnetic field at the center of a loop formed by splitting a straight conductor into two identical semicircular turns, we can follow these steps:
1. Understanding the Configuration:
- We have a straight conductor that is split into two identical semicircular arcs.
- A current I enters at one end and exits at the other end after passing through both arcs.
2. Identifying the Magnetic Field Contribution from Each Semicircle:
- The magnetic field at the center of a semicircular wire carrying current can be calculated using the formula:
B=μ0I4R
where R is the radius of the semicircle and μ0 is the permeability of free space.
3. Calculating the Magnetic Field from Each Semicircle:
- For the first semicircle (let's call it arc 1), the current flows in an anticlockwise direction. According to the right-hand rule, the magnetic field B1 at the center due to arc 1 will be directed out of the plane.
B1=μ0I4R
- For the second semicircle (arc 2), the current flows in a clockwise direction. Again, using the right-hand rule, the magnetic field B2 at the center due to arc 2 will be directed into the plane.
B2=μ0I4R
4. Determining the Net Magnetic Field:
- Since B1 is directed out of the plane and B2 is directed into the plane, they will oppose each other.
- The net magnetic field B at the center can be calculated as:
B=B1−B2
- Substituting the values we found:
B=μ0I4R−μ0I4R=0
5. Conclusion:
- The total magnetic field at the center of the loop formed by the two semicircular arcs is:
B=0
- We have a straight conductor that is split into two identical semicircular arcs.
- A current I enters at one end and exits at the other end after passing through both arcs.
- The magnetic field at the center of a semicircular wire carrying current can be calculated using the formula:
B=μ0I4R
where R is the radius of the semicircle and μ0 is the permeability of free space.
- For the first semicircle (let's call it arc 1), the current flows in an anticlockwise direction. According to the right-hand rule, the magnetic field B1 at the center due to arc 1 will be directed out of the plane.
B1=μ0I4R
- For the second semicircle (arc 2), the current flows in a clockwise direction. Again, using the right-hand rule, the magnetic field B2 at the center due to arc 2 will be directed into the plane.
B2=μ0I4R
- Since B1 is directed out of the plane and B2 is directed into the plane, they will oppose each other.
- The net magnetic field B at the center can be calculated as:
B=B1−B2
- Substituting the values we found:
B=μ0I4R−μ0I4R=0
- The total magnetic field at the center of the loop formed by the two semicircular arcs is:
B=0
Topper's Solved these Questions
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-3 (Level-II)
23 Videos
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-4 (LEVEL-I)
33 Videos
- MAGNETISM BOOK - MOTIONCHAPTER - MAGNETISM EXERCISE - EXERCISE-2 (Level-II)
15 Videos
- LOGIC GATESBOOK - MOTIONCHAPTER - LOGIC GATESEXERCISE - EXERCISE - 2
10 Videos
- MAGNETISM -1BOOK - MOTIONCHAPTER - MAGNETISM -1EXERCISE - Exercise - 3 SECTION -A
56 Videos
Similar Questions
A square frame of side l carries a current produces a field B at its centre. The same current is passed through a circular loop having same perimeter as the square. The field at its centre is B', the ratio of B/B' is
View SolutionA charged particle of mass m and charge q travels on a circular path of radius r that is perpendicular to a magnetic field B. The time takeen by the particle to complete one revolution is
View SolutionConsider the magnetic field produced by a finitely long current carrying wire .
View SolutionOut of the following, universal gate is :-
View SolutionAn electron moves in a circular orbit with a uniform speed v.It produces a magnetic field B at the centre of the circle. The radius of the circle is proportional to
View SolutionA current loop consists of two identical semicircular parts each of radius R, one lying in the x-y plane and the other in x-y plane. If the current in the loop is i, the resultant magnetic field due to two semicircular parts at their common centre is
View SolutionA circular conducting loop of radius R carries a current I . Another straight infinite conductor carrying current I passes through the diameter of this loop as shown in the figure. The magnitude of force exerted by the straight conductor on the loop is
View SolutionA current of 10A is passing through a long wire which has semicircular loop of the radius 20cm as shown in the figure. Magnetic field produced at the centre of the loop is

View SolutionA current carrying conductor of length l is bent into two loops one by one. First loop has one turn of wire and the second loop has two turns of wire. Compare the magnetic fields at the centre of the loops
View SolutionIn the figure, the current l enters the circular loop of uniform wire of radius r at A and leaves it at B. The magnetic field of the centre of circular loop is
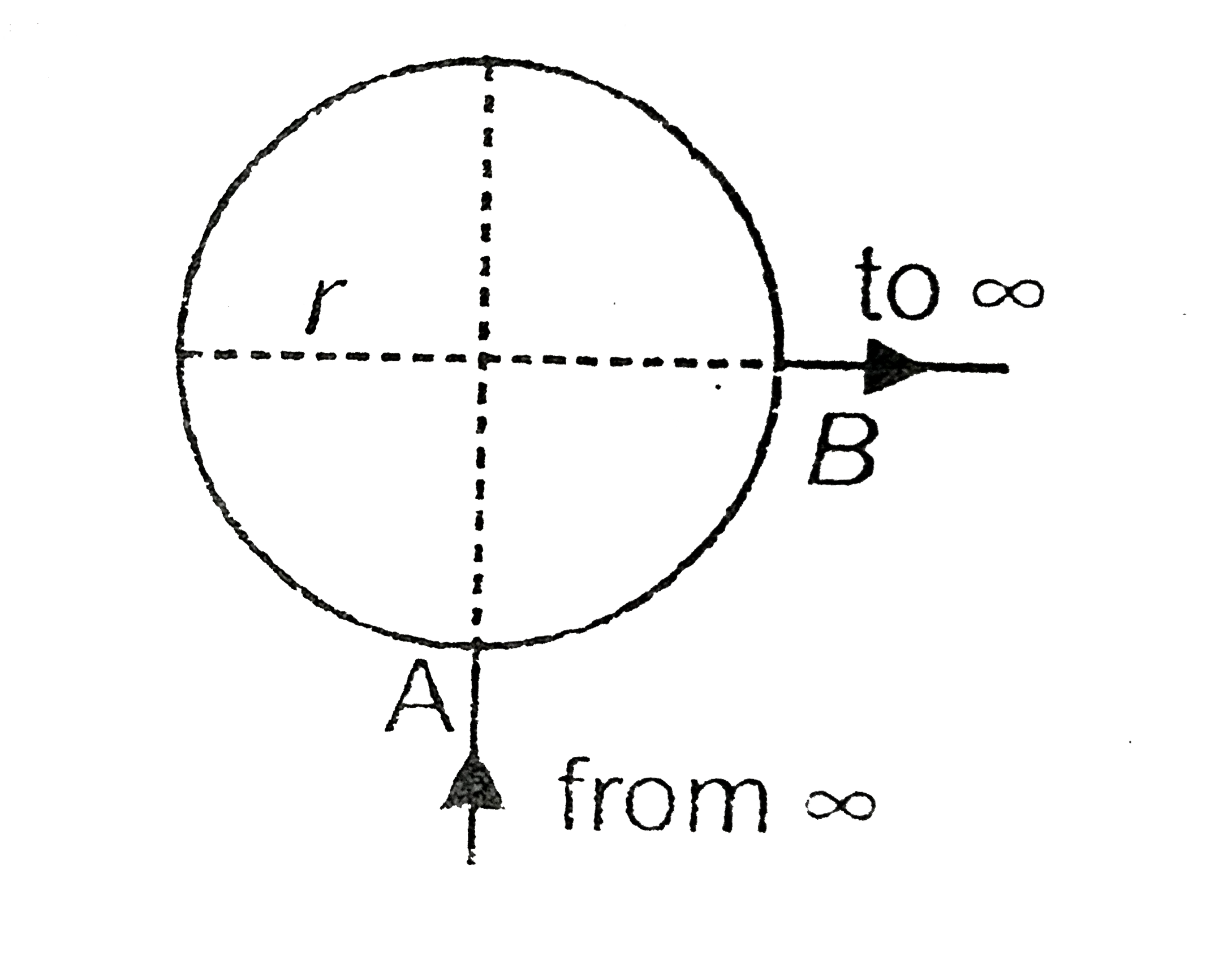
View SolutionA straight conductor carrying a current I, is split into a circular loop of radius r as shown in the figure. The fied at the centre O of the circle, in tesla is

View SolutionIn the shown figure a current 2i is flowing in a straight conductor and entering along the diameter of the circular loop of similar conductor through the point A. The current is leaving the loop along another similar semi-infinite conductor parallel to the plane of the loop through the point D. what is the magnetic field at the centre of the loop P ?
View SolutionA cell is connected between two points of a uniformly thick circular conductor and i1andi2 are the currents flowing in two parts of the circular conductor of radius a The magnetic field at the centre of the loop will be
View SolutionA current loop consists of two identical semicircular parts each of radius R, one lying in the x-y plane and the other in x-z plane. If the current in the loop is 'i' the resultant magnetic field due to the two semicircular parts at their common centre is
View SolutionA current carrying conductor of length l is bent into two loops one by one. First loop has one turn of wire and the second loop has two turns of wire. Compare the magnetic fields at the centre of the loops
View Solution
