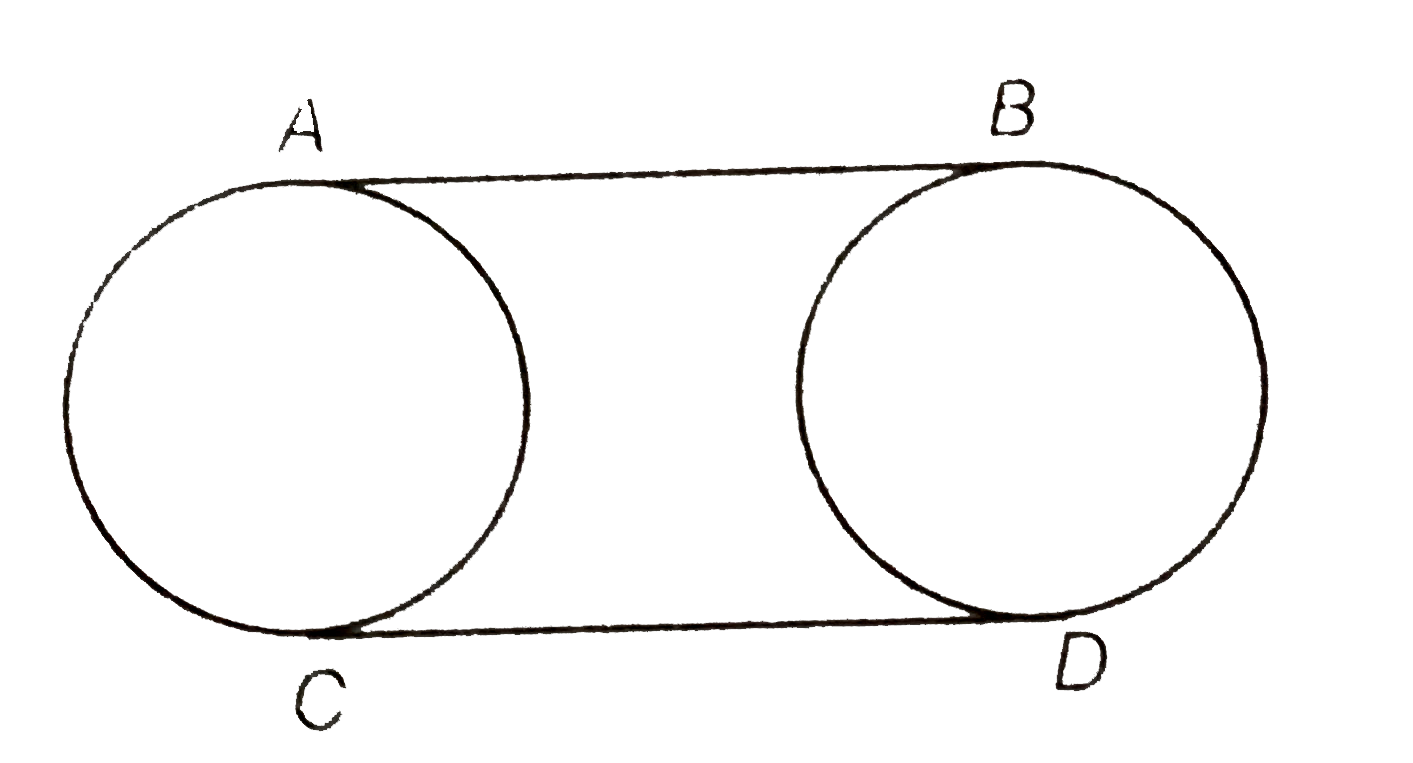
In figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB=CD
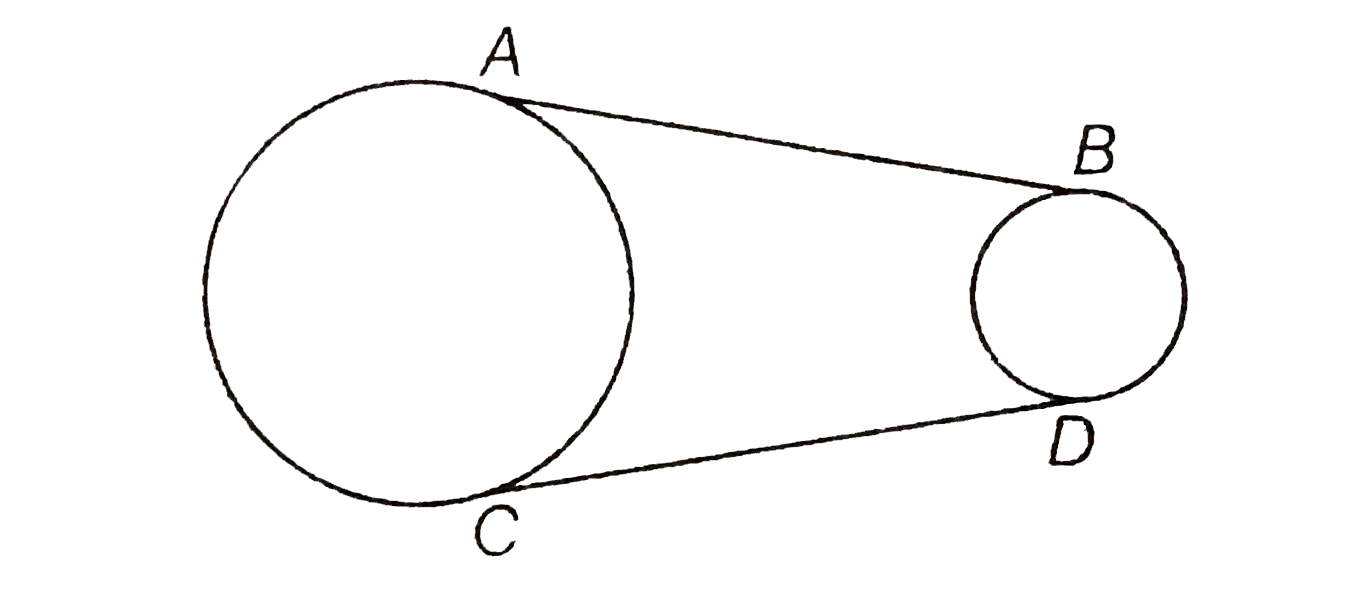
Video Solution
Text Solution
Given AB and CD are common tangent to two circles of unequal radius
To prove AB=CD
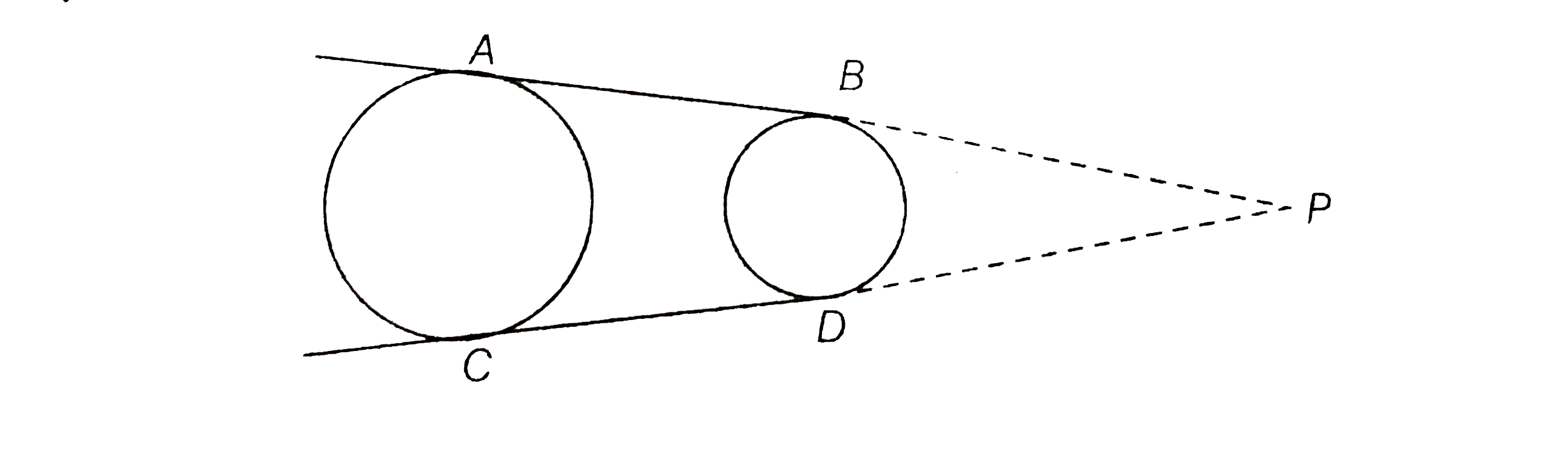
Construction Produce AB and CD, to intersect at P.
Proof PA=PC
[the length of tangents drawn from an internal point to a circle are equal]
Also, PB=PD
[the lengths of tangents drawn from an internal point to a circle are equal]
AB=CD Hence proved.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, AB||CD then
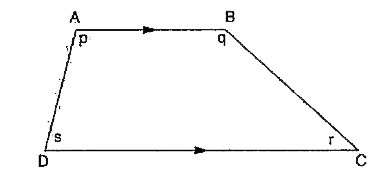 Ap+r=q+sBp−r=q−sCp+s=q+rDp−q=s−r
Ap+r=q+sBp−r=q−sCp+s=q+rDp−q=s−rIn the given figure, AB and CD are two common to the two touching circle. If CD=6cm, then AB is equal to
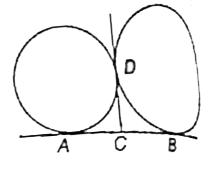 A9 cmB15 cmC12 cmDnone of the above
A9 cmB15 cmC12 cmDnone of the above
In the adjoining figure, common tangents AB and CD to two circles intersect at P.
Prove that AB=CD.

View SolutionIn figure, common tangents AB and CD to two circles intersect at E. Prove that AB=CD.
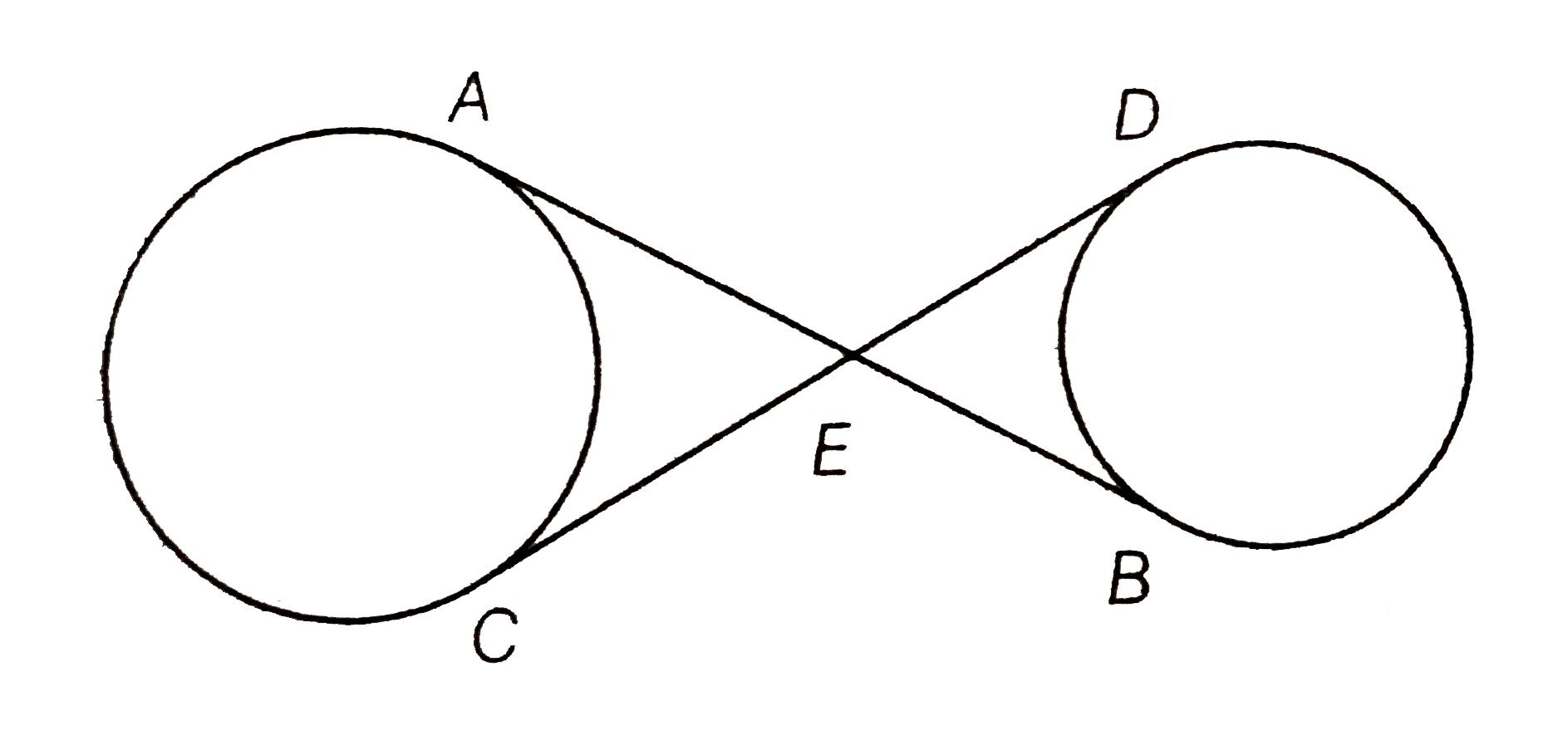
View SolutionAB and CD are common tangents to two circles which intersect each other at C as shown in the figure IF AB = 6cm , find CD.

View SolutionAB and CD are two parallel chords of a circle whose diameter is AC . Prove that AB=CD.
View SolutionAB and CD are two parallel chords of a circle whose diameter is AC . Prove that AB=CD
View SolutionIn the given figure,AB and CDare equal chords of a circle with centre O .Prove that ∠AOB≅∠COD and ∠AOB=∠COD
View Solution