An object is project with a speed 10ms−1 at an angle of 30∘ with the horizontal. It breaks into n equal fragments during its motion . One fragment strikes the ground at a distance of √3m from the point of projection. The centre of mass of the remaining fragments strikes the ground at a distance of 7√3m from the point of projection . If all fragments strike the ground at the same time , Find the value of n.
2
3
4
525
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the object and the behavior of the fragments after it breaks apart.
Step 1: Determine the initial parameters
The object is projected with a speed u=10m/s at an angle θ=30∘ with the horizontal.
Step 2: Calculate the horizontal range of the projectile
The formula for the range R of a projectile is given by:
R=u2sin(2θ)g
Where g≈9.81m/s2.
Calculating sin(60∘):
sin(60∘)=√32
Now substituting the values:
R=(10)2⋅√329.81=100⋅√329.81≈50√39.81
Step 3: Analyze the fragments
Let the object break into n equal fragments. The mass of each fragment is mn.
One fragment strikes the ground at a distance of √3m from the point of projection.
Step 4: Center of mass of the remaining fragments
The center of mass of the remaining n−1 fragments strikes the ground at a distance of 7√3m.
Step 5: Set up the equation for the center of mass
The center of mass of the system can be calculated using the formula:
Center of mass=m1x1+m2x2+…+mnxnm1+m2+…+mn
For our case:
- m1=mn (the fragment that lands at √3)
- m2=(n−1)mn (the remaining fragments that land at 7√3)
Setting up the equation:
mn⋅√3+(n−1)mn⋅7√3m=Center of mass position
Step 6: Simplify the equation
Since the total mass m cancels out:
√3n+(n−1)⋅7√3n=5√3
Step 7: Multiply through by n to eliminate the denominator
√3+(n−1)⋅7√3=5n√3
Step 8: Factor out √3
√3+7(n−1)√3=5n√3
Dividing through by √3:
1+7(n−1)=5n
Step 9: Solve for n
Expanding and rearranging gives:
1+7n−7=5n⟹2n=6⟹n=3
Conclusion
The value of n is 3. Therefore, the object breaks into 3 equal fragments.
---
The object is projected with a speed u=10m/s at an angle θ=30∘ with the horizontal.
The formula for the range R of a projectile is given by:
R=u2sin(2θ)g
Where g≈9.81m/s2.
sin(60∘)=√32
R=(10)2⋅√329.81=100⋅√329.81≈50√39.81
Let the object break into n equal fragments. The mass of each fragment is mn.
The center of mass of the remaining n−1 fragments strikes the ground at a distance of 7√3m.
The center of mass of the system can be calculated using the formula:
Center of mass=m1x1+m2x2+…+mnxnm1+m2+…+mn
For our case:
- m1=mn (the fragment that lands at √3)
- m2=(n−1)mn (the remaining fragments that land at 7√3)
mn⋅√3+(n−1)mn⋅7√3m=Center of mass position
Since the total mass m cancels out:
√3n+(n−1)⋅7√3n=5√3
√3+(n−1)⋅7√3=5n√3
√3+7(n−1)√3=5n√3
Dividing through by √3:
1+7(n−1)=5n
Expanding and rearranging gives:
1+7n−7=5n⟹2n=6⟹n=3
The value of n is 3. Therefore, the object breaks into 3 equal fragments.
Topper's Solved these Questions
Similar Questions
A body is projected with a speed 160 m/s at an angle 53∘ with the horizontal, At the highest point, body explodes into two pieces with mass ratio 1:3. Smaller piece comes to rest immediately after explosion. Find the distance of point from point of projection where heavier piece strikes the horizontal surface.
View SolutionA bomb is projected with speed 10 m/s at an angle 30 with the horizontal. In the mid-air bomb explodes in different fragments and they all move in different directions. At the time when all fragments reach at ground, the displacement of the center of mass of bomb from initial point will be (g=10ms2)
View Solution
Knowledge Check
A particle just clear a wall of height b at a distance a and strikes the ground at a distance from the point of projection, the angle of projection is :
Atan−1(bac)B45∘Ctan−1(bca(c−1))Dtan−1(bca)A ball is projected at an angle 45∘ with horizontal. It passes through a wall of height h at horizontal distance d1 from the point of projection and strikes the ground at a horizontal distance (d1+d2) from the point of projection, then
Ah=2dd_(2)d+d2Bh=d1d2d1+d2Ch=√2d1d2d1+d2Dh=d1d22(d1+d2)A body of mass m is projected at an angle of 45∘ with the horizontal. If air resistance is negligible, then total change in momentum when it strikes the ground is
A2mvB√mvCmvDmv/√2
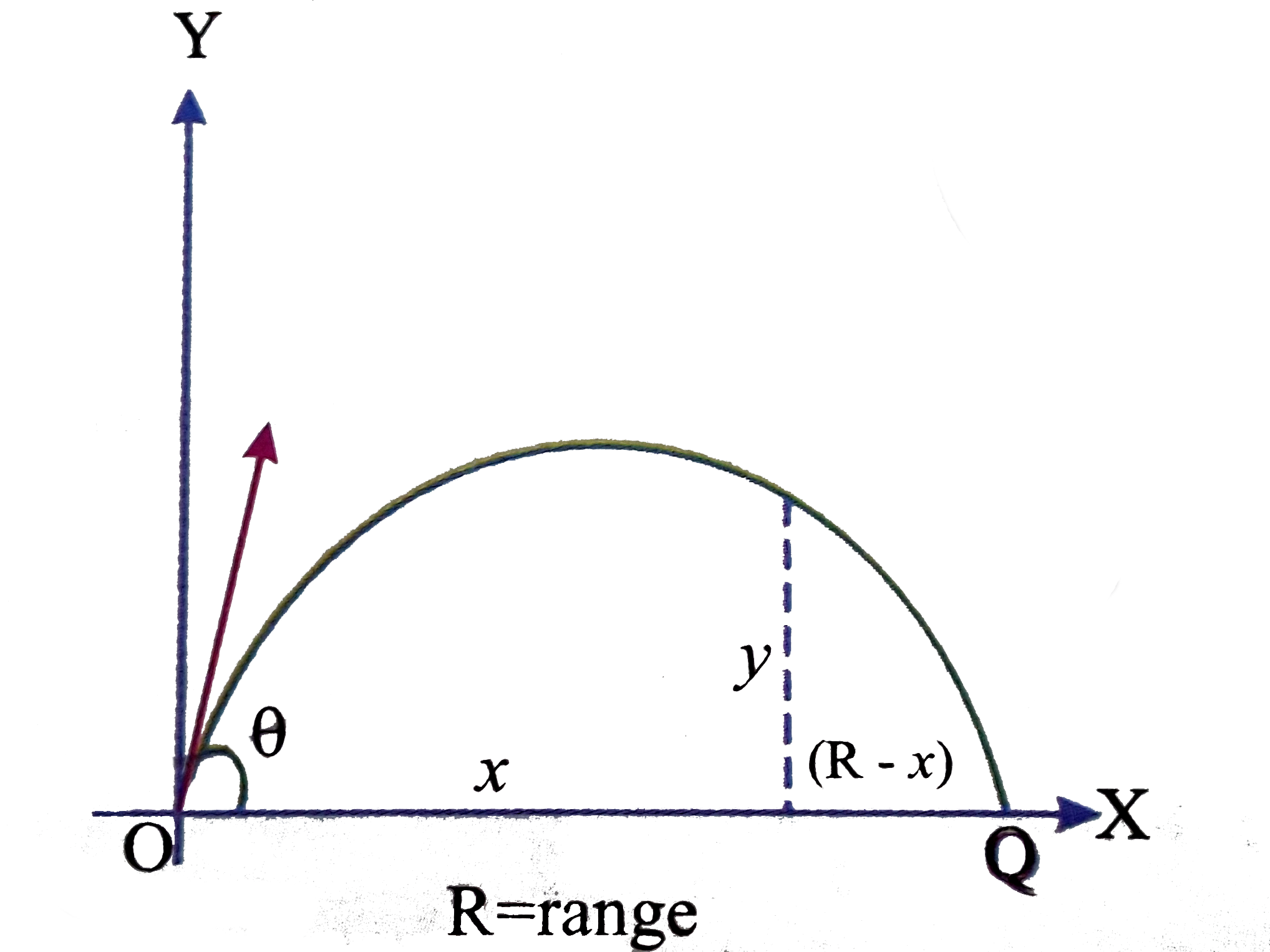
A body projected from a point O at an angle θ, just crosses a wall y m high at a distance. x m from the point of projection and strikes the ground at Q beyond the wall as shown, then find height of the wall
View SolutionA particle is projected from ground with some initial velocity making an angle of 45∘ with the horizontal. It reaches a height of 7.5m above the ground while it travels a horizontal distance of 10m from the point of projection. The initial speed of the projection is
View SolutionA particle of mass 1kg is projected with an initial velocity 10ms−1 at an angle of projection 45∘ with the horizontal. The average torque acting on the projectile and the time at which it strikes the ground about the point of projection in newton meter is
View SolutionA bomb is projected with speed 10 m/s at an angle 30o with the horizontal. In the mid-air bomb explodes in different fragments and they all move in different directions. At the time when all fragments reach at ground, the displacement of the center of mass of bomb from initial point will be (g = 10 m/s2)
View SolutionAn object is projected from ground with speed 20 m/s at angle 30∘ with horizontal. Its centripetal acceleration one second after the projection is
[ Take g = 10 m/s2 ]
View Solution
