f(x)=x15 at x=0 has inflection point. y D.N.E. at x=0
Step by step video & image solution for f(x)=x^(1/5) at x=0 has inflection point. y"\ DdotNdotEdot\ at x=0 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Concavity and convexity :
if f''(x)>0∀x∈(a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and iff''(x)<0∀x∈(a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b)

Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined)
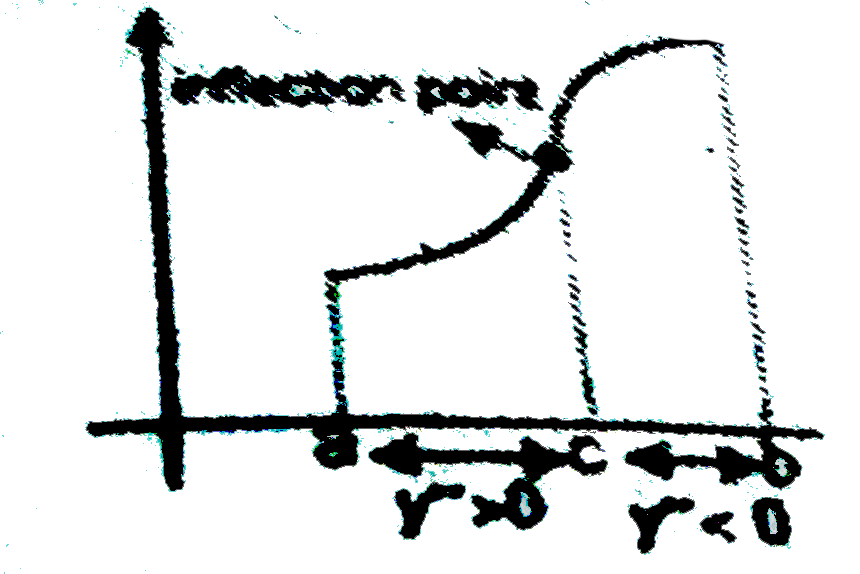
Number of point of inflection for f(x)=(x−1)3(x−2)2 is :A1B2C3D4Concavity and convexity :
if f''(x)>0∀x∈(a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and iff''(x)<0∀x∈(a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b)
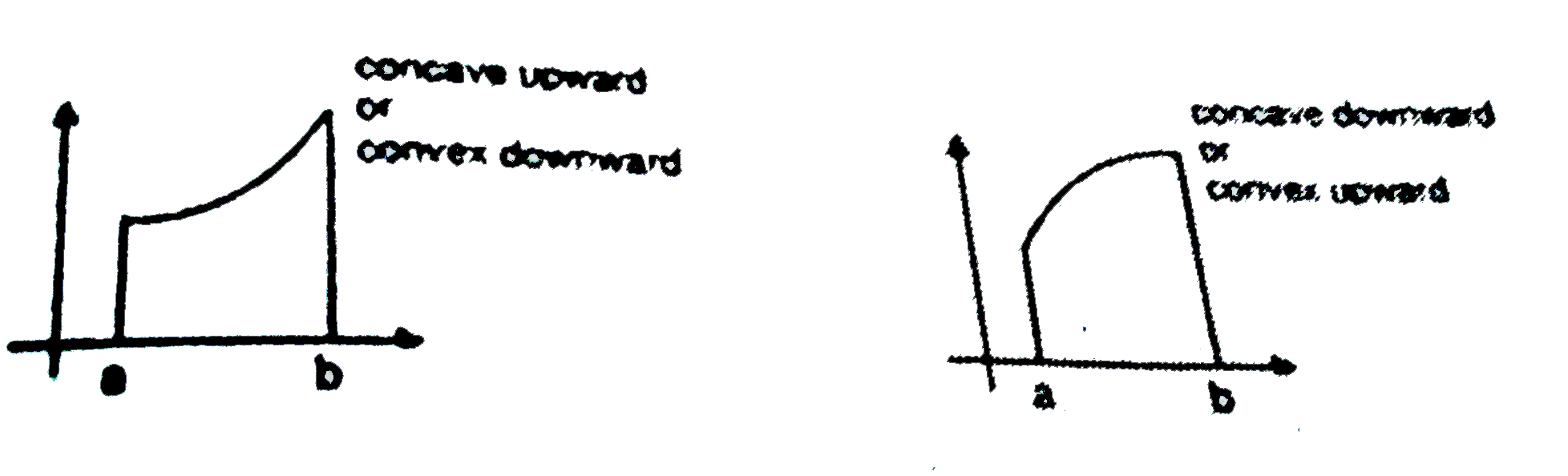
Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined)

Exhaustive set of values of 'a' for which the function f(x)=x4+ax3+3x22+1 will be concave upward along the entire real line is :A[−1,1]B[−2,2]C[0,2]D[0,4]
f(x)=∣∣x2−1∣∣ has no inflection point in its domain as no tangent can be drawn at these points.
View SolutionThe function f(x)=x13(x−1) has two inflection points has one point of extremum is non-differentiable has range [−3x2−83,∞)
View SolutionThe graph of the derivative f of a continuous function f is shown with f(0)=0

If f(x) has local minima at x=x1 and x=x2,f has inflection point at x=k, number of critical points of y=f(x) is w then x1+x2−k−w is...........
View SolutionThe curve f(x)=x23 ,at the point with x=0 ,has
View SolutionThe diagram shows the graph of the derivative of a functin f(x) for 0≤x≤4 with f(0) = 0. Which of the following could be correct statements for y = f(x)?
(##CENGRAC01S01021_Q01.png width=80%><br>(a)Tan≥ntl∈e→y=f(x)atx=0makesan∠of sec^(-1) sqrt 5 with the x - axis.
(b) f is increasing in (0, 3).
(c) x = 1 is both an inflection point and the point of local extremum.
(d) Number of critical point on y = f(x) is two.
View SolutionA function y−f(x) has a second-order derivative fx=6(x−1). It its graph passes through the point (2,1) and at that point tangent to the graph is y=3x−5, then the value of f(0) is 1 (b) −1 (c) 2 (d) 0
View Solution
