If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
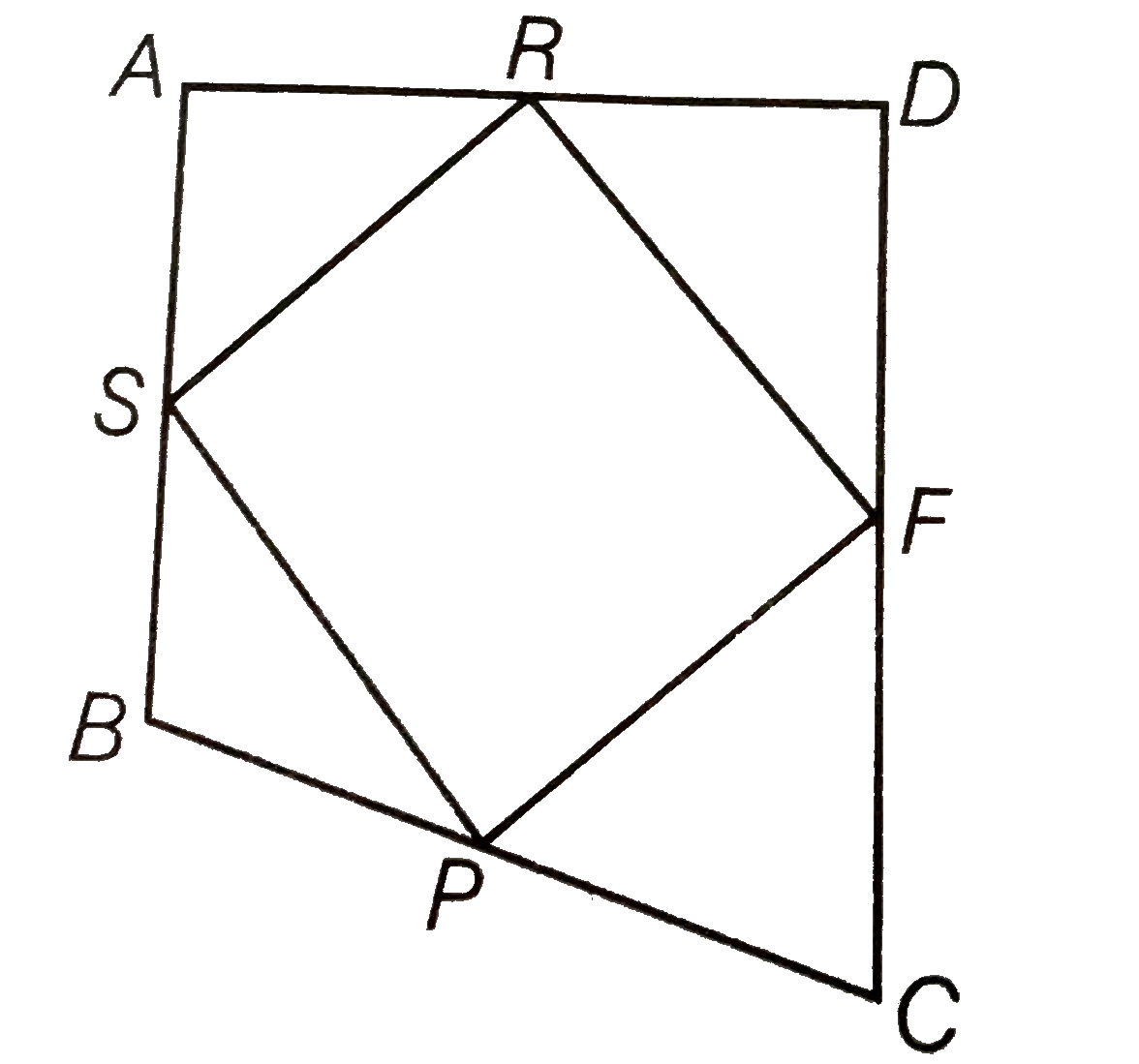
Given: A quadrilateral ABCD in which the mid-points of the sides of it are joined in order to form parallelogram PQRS.
TO prove: ar(∣∣gmPQRS)=12ar(□ABCD)
Consturction : Join BD and draw perpendicular from A on BD which intersect SR and BD at X and Y respectively.
Proof: In ΔABD, S and R are the mid-points of sides AB and AD respectively
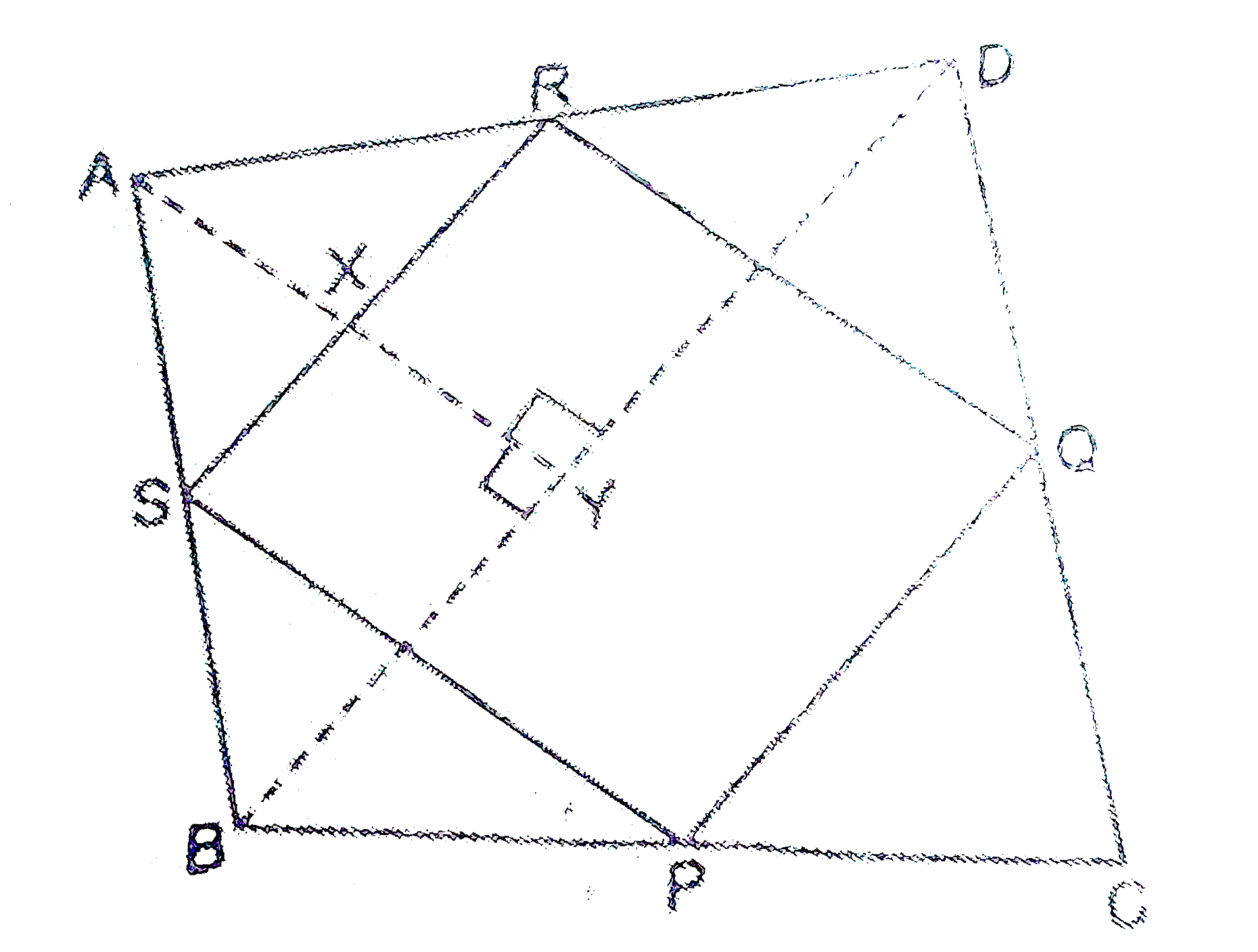
∴SR∣∣BD
⇒SX∣∣BY
⇒ X is the mid-point of AY (converse of mid-point theorem)
⇒AX=XY ...(1)
and SR=12BD ( ∵ mid-point theorem)..(2)
Now, ar(ΔABCD)=12×BD×AY
and ar(ΔASR)=12×SR×AX
⇒ar(ΔASR)=12×(12BD)×(12AY) [using (1) and (2)]
⇒ar(ΔASR)=14×(12BD×AY)
⇒ar(ΔASR)=14ar(ΔABD)...(3)
Similarly,
ar(ΔCPQ)=14ar(ΔCBD)...(4)
ar(ΔBPS)=14ar(ΔBAC)...(5)
ar(ΔDRQ)=14ar(ΔDAC)...(6)
Adding eqs. (3), (4), (5) and (6), we get
ar(ΔASR)+ar(ΔCPQ)+ar(ΔBPS)+ar(ΔDRQ)
=14ar(ΔABD)+14ar(ΔCBD)+14ar(ΔBAC)+14ar(ΔDAC)
=14[ar(ΔABD)+ar(ΔCBD)+ar(ΔBAC)+ar(ΔDAC)]
=14[ar(□ABCD)+ar(□ABCD)]=14×2ar(□ABCD)=12ar(□ABCD)
∴ar(ΔASR)+ar(ΔCPQ)+ar(ΔBPS)+ar(ΔDRQ)=12ar(□ABCD)
⇒ar(□ABCD)−ar(∣∣gmPQRS)=12ar(□ABCD)
⇒ar(∣∣gmPQRS)=ar(□ABCD)−12ar(□ABCD)
rArr ar (||gm PQRS) = (1)/(2) ar (square ABCD)
Topper's Solved these Questions
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise
34 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)
10 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CIRCLE BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Revision Exercise (long Answer Questions )
5 Videos
Similar Questions
Knowledge Check
In the adjoining figure, the area of quadrilateral ABCD is
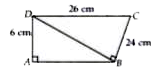 A148 cm2B144cm2C120 cm2D122 cm2
A148 cm2B144cm2C120 cm2D122 cm2The figure formed by joining the mid-points of the sides of a quadrilateral taken in order is
AparallelogramBrectangeCsquareDnoneThe figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only, if
AABCD is a rhombusBdiagnols of ABCD are equalCdiagonals of ABCD are equal and perpendicularDdiagonals of ABCD are perpendicular
If the mid points of consecutive of a quadrilateral connected by straight lines prove that the resulting quadrilateral is a parallelogram.
View SolutionThe midpoints of the sides AB,BC,CDandDA of a quadrilateral ABCD are joined to form a quadrilateral. If AC=BDandAC⊥BD then prove that the quadrilateral formed is a square.
View SolutionArea of the quadrilaterals formed by joining the mid points of the adjacent sides of a quadrilateral is________ the area of given quadrilateral.
View SolutionConsider the - following statements in respect of a quadrilateral :
1. The line segments joining the midpoints of the two pairs of opposite sides bisect each other at the point of intersection.
2. The area of the quadrilateral formed by joining the midpoints of the four adjacent sides is half of the total area of the quadrilateral.
Which of the statements given above is/are correct ?
View SolutionThe figure formed by joining the mid-points of the sides of a quadrilateral ABCD taken in order is a square only, if
View Solution
