In figure, CD∣∣AE and CY∣∣BA. Prove that ar(ΔCBX)=ar(ΔAXY).
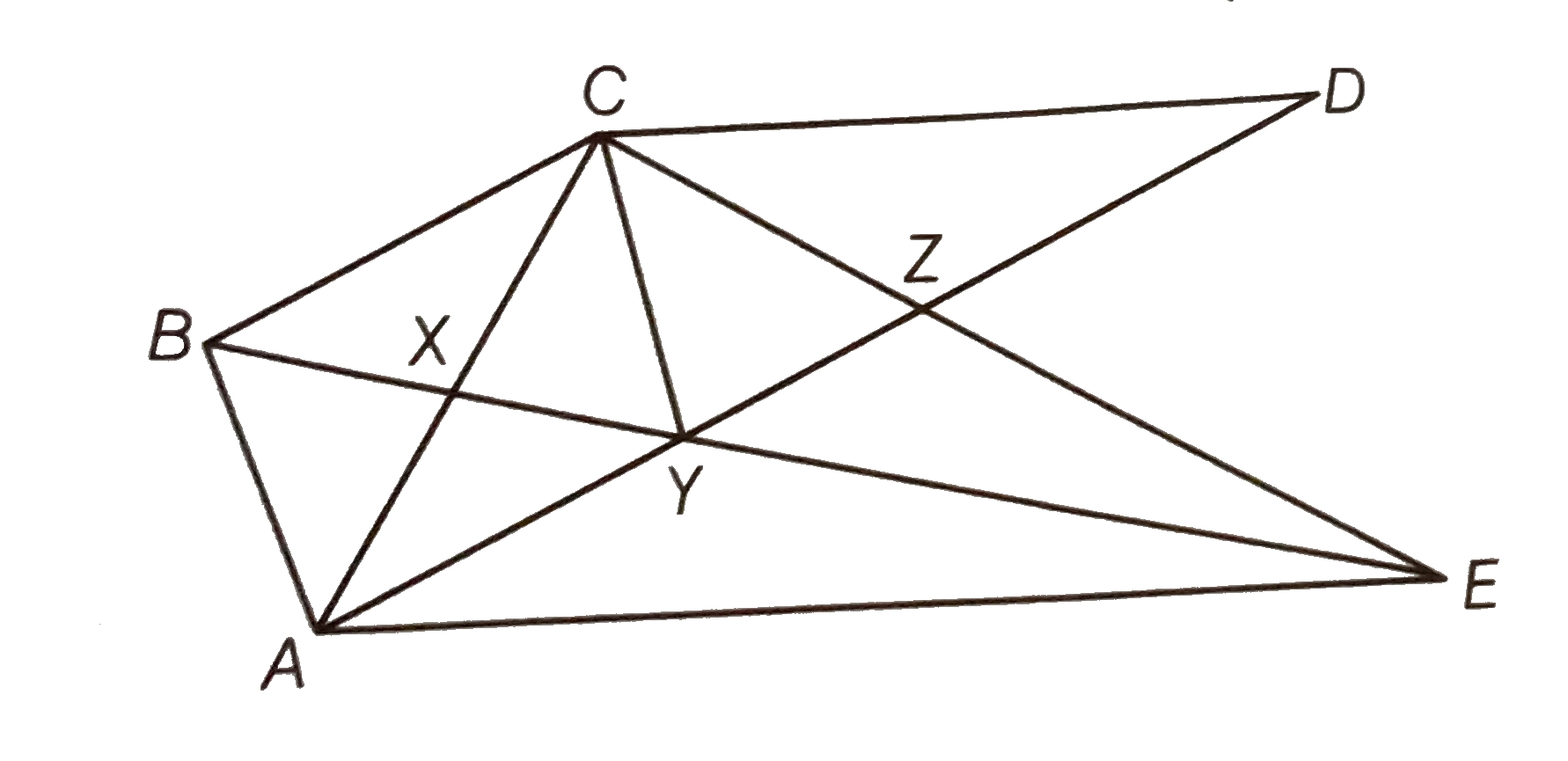
Given : CD||AEandCY||BA
To prove: ar(ΔCBX)=ar(ΔAXY0
Proof: Since, triangle on the same base AB and between the same parallels AB and YC are equal in area, so we have
ar(ΔABC)=ar(ΔABY)
⇒ar(ΔCBX)+ar(ΔABX)=ar(ΔABX)+ar(ΔAXY)
Hence, ar(ΔCBX)=ar(ΔAXY) [cancelling ar(ΔABX) from both sides]
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise34videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)10videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)5videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Revision Exercise (long Answer Questions )5videos
CIRCLE
Similar Questions
In the given figure, AP||BQ||CR. Prove that ar(ΔAQC)=ar(ΔPBR)
 View Solution
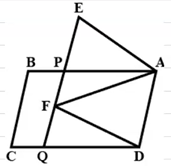
View SolutionIn figure, ABCD and AEFD are two parallelograms. Prove that ar(ΔPEA)=ar(ΔQFD).
 View Solution
View SolutionIn figure, ABCD and AEFD are two parallelograms. Prove that ar(ΔPEA)=ar(ΔQFD).
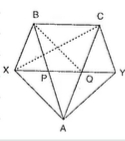
View SolutionIn Figure, BC||XY,BX||CA and AB∣∣YC Prove that: ar(ABX)=ar(ACY)
 View Solution
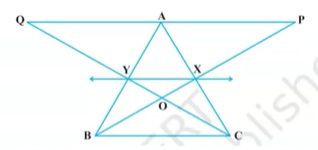
View SolutionIn figure X and Y are the mid-points of AC and AB respectively, QP∣∣BC and CYQ and BXP are straight lines. Prove that ar(ΔABP)=ar(ΔACQ).
 View Solution
View SolutionIn ΔABC , if L and M are the points on AB and AC, respectively such that LM∣∣BC. Prove that ar(ΔLOB)=ar(ΔMOC).
View SolutionA point D is taken on the side BC of a ΔABC such that BD=2DC. Prove that ar(ΔABD)=2ar(ΔADC)
View SolutionIn the given figure, if DE||AQ and DF||AR. Prove that EF||QR.
 View Solution
View SolutionIn figure , if PQ||BCandPR||CD . Prove that ARAD=AQAB .
 View Solution
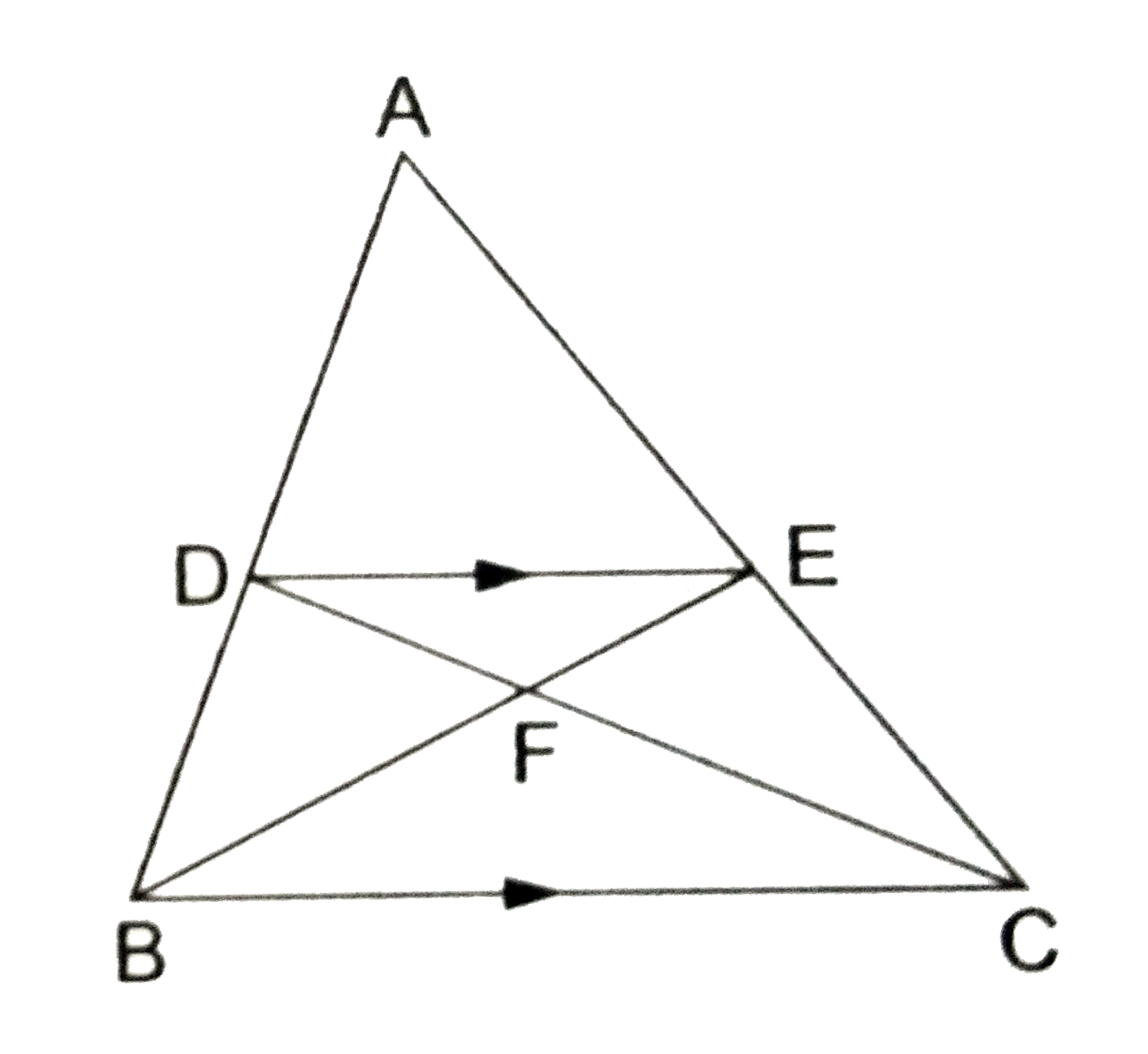
View SolutionIn the given figure DE∣∣BCandAD:DB=5:4
Find the ratio ar(ΔDEF):ar(ΔCFB)
 View Solution
View Solution

