Find the area bounded by the following curve :
(i) $f (x) = sin x, g (x) = {sin}^{2} x, 0 \leq x \leq 2 π$
(ii) $f (x) = sin x, g (x) = {sin}^{4} x, 0 \leq x \leq 2 π$

Text Solution

Verified by Experts

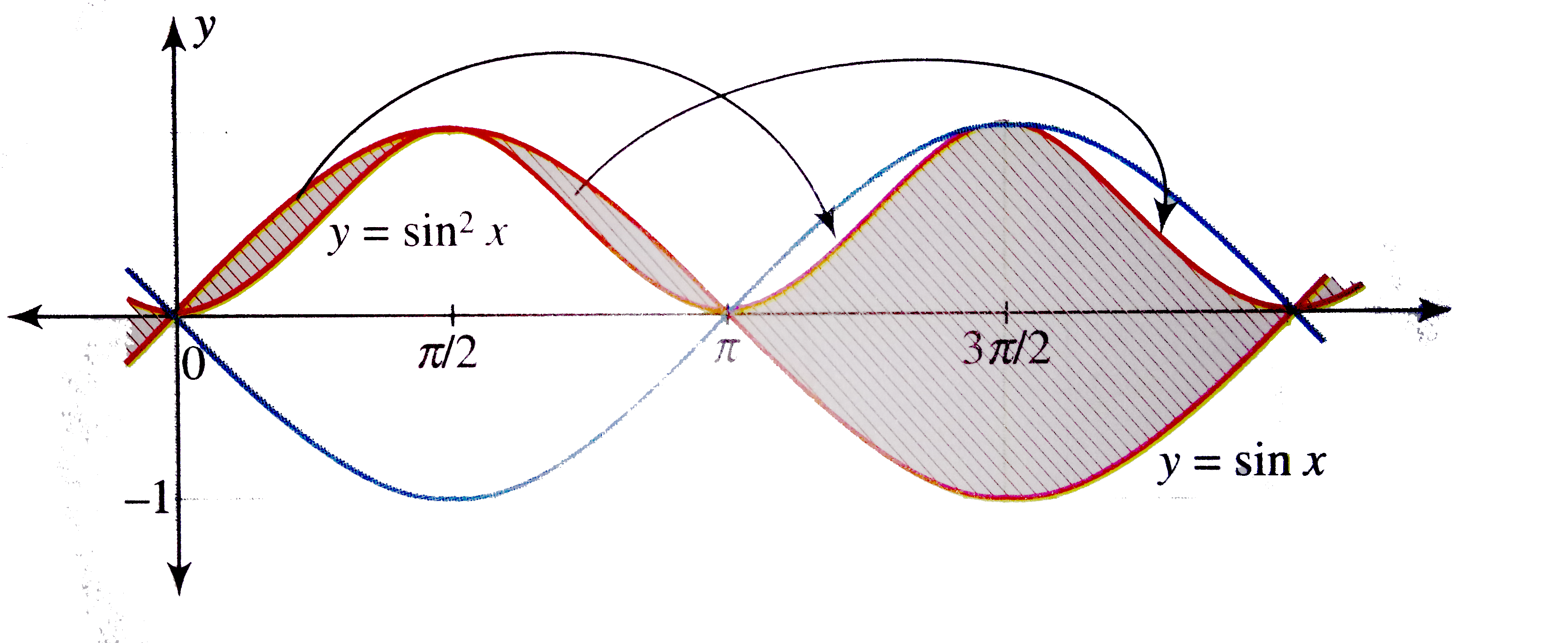
As shown in the figure, the area bounded by $y = sin x$ and $y = {sin}^{2} x$ is equal to twice the area bounded by y = sin x and the x - axis for $x \in [0, π] .$ Now we know that the area bounded by y = sin x and the x axis for $x \in [0, π]$ is $π \int 0 sin x d x = 2 sq. units$
Hence the required area is 4 sq. units.

|

Updated on:21/07/2023

Class 12 MATHS GRAPHS OF TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Let $f (x) = 2 sin x + cos 2 x (0 \leq x \leq 2 π) and g (x) = x + cos x$ then
Ag is a decreasing function
Bf increases on $(0, π / 2)$
Cf increases on $(0, π / 6) \cup (π / 2, 5 π / 6)$
Df decreases on $(0, π / 2)$
Question 2 - Select One
$sin x + 2 sin 2 x = 3 + sin 3 x, 0 \leq x \leq 2 x$ has
A2 solutions in I quadrant
Bone solution in II quadrant
Cno solution in any quadrant
Done solution in each quadrant
Question 3 - Select One
Let $f (x) = min {1, cos x, 1 - sin x}, - π \leq x \leq π$ , Then, f(x) is
Anot continuous at $x = π / 2$
Bcontinuous but not differentiable at x=0
Cneither continuous nor differentiable at $x = π / 2$
Dnone of these

CENGAGE-GRAPHS OF TRIGONOMETRIC FUNCTIONS-Exercise

Home
Ask Doubt
Profile