The given RC circuit has two switches S1andS2 The switch S2 is closed till the capacitor C attains its maximum possible charge 90. Then, Sy is opened and S, is closed simultaneously till the capacitor releases half of its total stored charge go for a time interval ty. Finally S is opened and Sa is closed till the capacitor attains a charge (3//4)qo for a time interval t2. Find the ratio (t.1/t2.
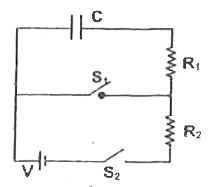
When the switch S2 is closed, the capacitor is charged to a potential V. Now the switch S2 is opened and the switch S1 is closed
The instantaneous charge on the capacitor is
⇒q=q0e1RC
whereq0=CVPuttingt=t1
forq=q−(0)/2,weoba∈et1R1C=12
⇒(t1=R1CIn2
Again, the switch S1 is opened and S2is closed. Therefore, the capacitor starts charging from charge q−(0)/2→34q0
Now, instantaneous charge on the capacitor is
q=q0[1−etR1+(R2)C]+q02etR1+R2C=q0[1−12etR1+R2c]
At,t=t2,q.=3q0/4⇒3q04=(q012et2(R1+R2)C)
⇒et2R1R2C=12⇒t2=(R1+R2)CIn2
∴
The required ratio of the times =(t1t2R1CIn2(R1+R2)CIn2
or,t1t2=R1(R1+R2)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - SOLVED PROBLEMS (OBJECTIVE)
20 Videos
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
9 Videos
- CURRENT ELECTRICITYBOOK - FIITJEECHAPTER - CURRENT ELECTRICITYEXERCISE - Illustration
10 Videos
- COLLISION BOOK - FIITJEECHAPTER - COLLISION EXERCISE - (NUMERICAL BASED QUESTIONS)
4 Videos
- ELASTICITY AND WAVES BOOK - FIITJEECHAPTER - ELASTICITY AND WAVES EXERCISE - Assignment Problems (Objective) Level-II
15 Videos
Similar Questions
The given R-C circuit has two swithes S1andS2 Swithc S2 is clsoed and S1 is open till the capacitor is fully charged to q0 the S2 is opened and S1 is closed simultaneously till the charge on capacitor remains q0/2 for which it takes time t1. Now S1 is again opened, and S2 is closed till chrage on capacitor becomes 3q04. it takes time t2 (see fig for referecne). Find the raito t1/t2
View SolutionThe given R-C circuit has two swithes S1andS2 Swithc S2 is clsoed and S1 is open till the capacitor is fully charged to q0 the S2 is opened and S1 is closed simultaneously till the charge on capacitor remains q0/2 for which it takes time t1. Now S1 is again opened, and S2 is closed till chrage on capacitor becomes 3q04. it takes time t2 (see fig for referecne). Find the raito t1/t2

View Solution
Knowledge Check
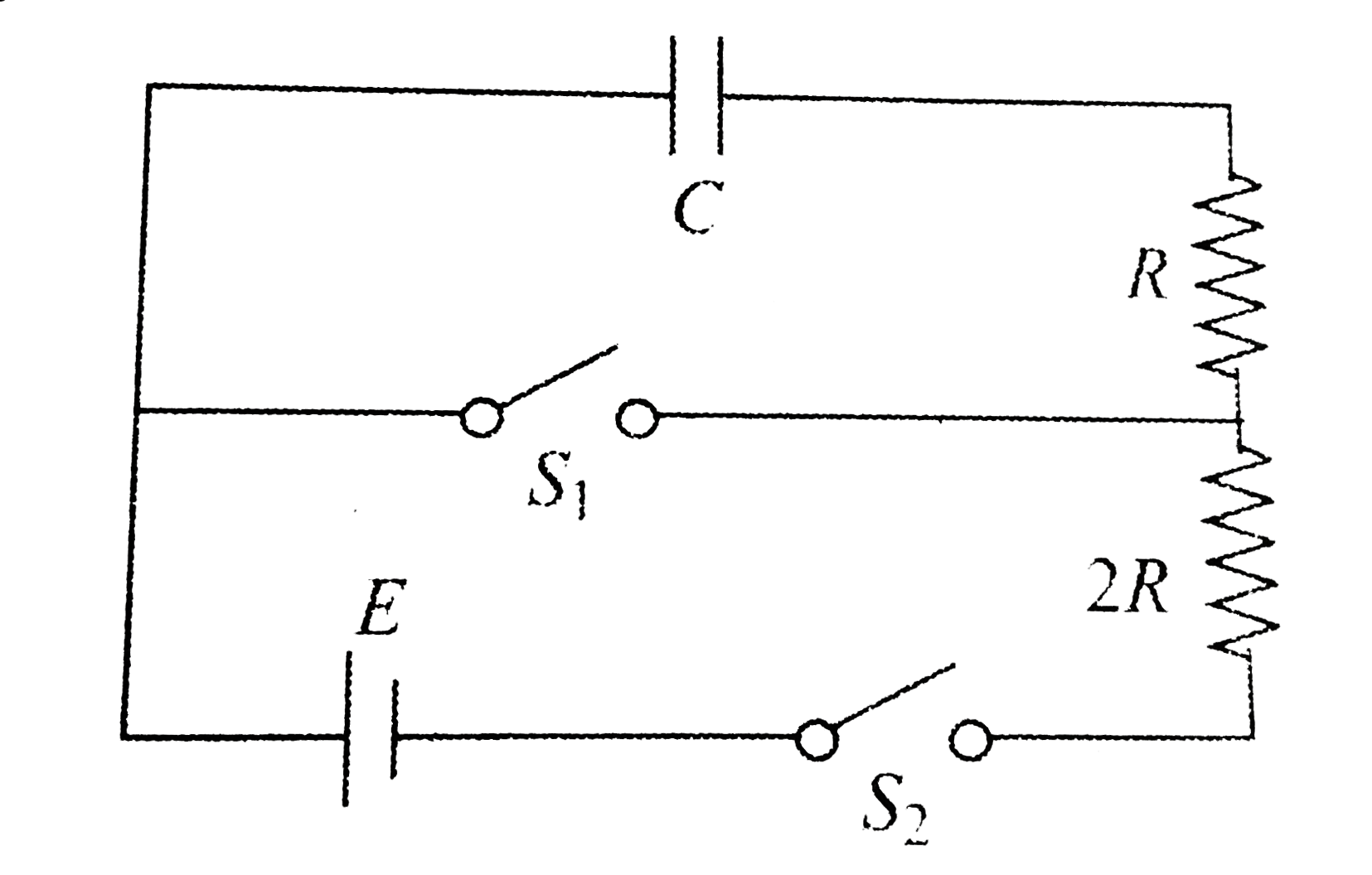
In the circuit shown in fig, if the switch S is closed at t=0 , the capacitor charges with a time constant
 ARCB3RCC23RCDRCIn(23)
ARCB3RCC23RCDRCIn(23)After the capacitor gets fully charged, S1 is opened and S2 is closed so that the inductor is connected in series with the capacitor. Then,
Aat t=0,energy stored in the circuit is purely in the formed of magnetic energy.Bat any time t>0, current in the circuit is in the same directionCat t>0, there is no exchange of energy between the inductor and capacitorDat any timet>0instantaneous current in the circuit mayV√CLThe switch S in the circuit diagram is closed at t = 0. The charge on capacitors at any time is
 Aqt=EC(1−e−2t/3RC)Bqt=EC(1−e−t/2RC)Cqt=EC(1−e−t/3RC)Dqt=EC(1−e−3t/2RC)
Aqt=EC(1−e−2t/3RC)Bqt=EC(1−e−t/2RC)Cqt=EC(1−e−t/3RC)Dqt=EC(1−e−3t/2RC)
In the circuit shown in fig, if the switch S is closed at t=0 , the capacitor charges with a time constant

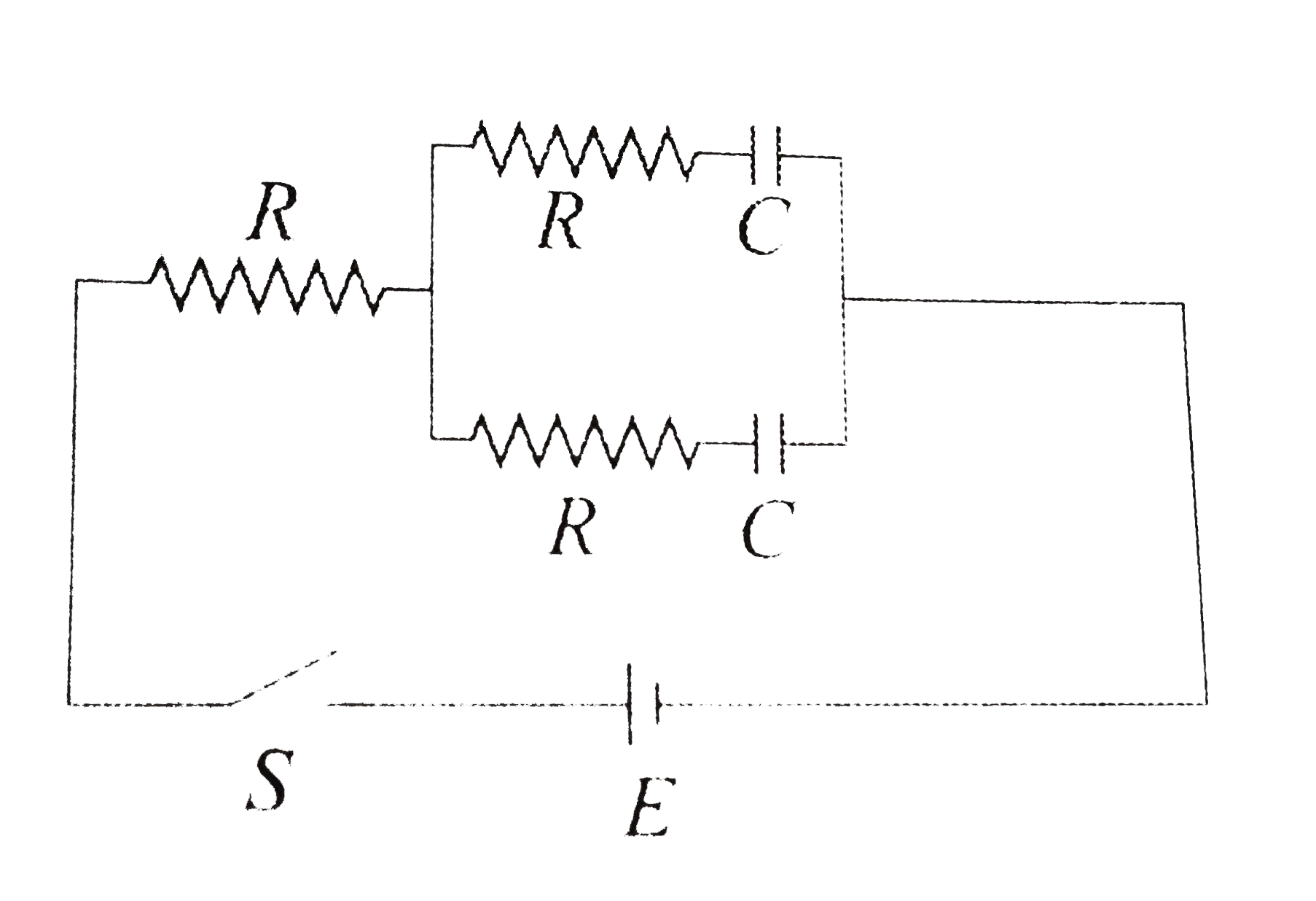
View SolutionAt t =0 switch S1 is closed and remains closed for a long time and S2 remains open. Now S1 is opened and S2 is closed. Find out
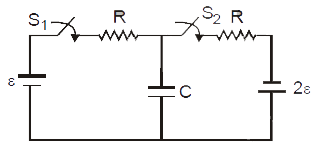
Charge on the capacitor long after that moment.
View SolutionThe switch S in the circuit diagram is closed at t = 0. The charge on capacitors at any time is

View SolutionIn the circuit shown in the figure, if switches S1 and S2 have been closed for a long time, then charge on the capacitor.
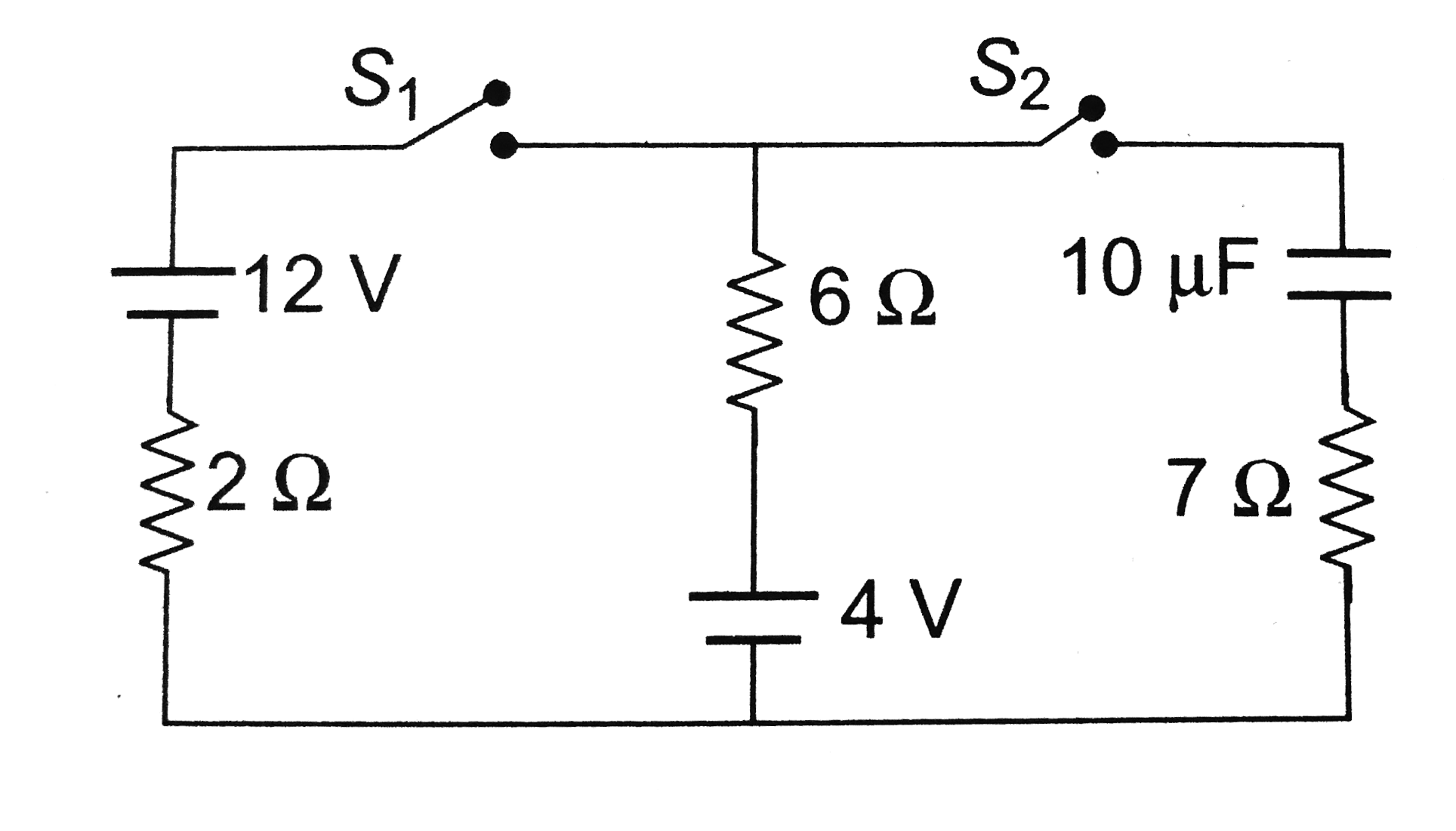
View SolutionIn the circuit shown in the figure, if switches S1 and S2 have been closed for a long time, then charge on the capacitor.
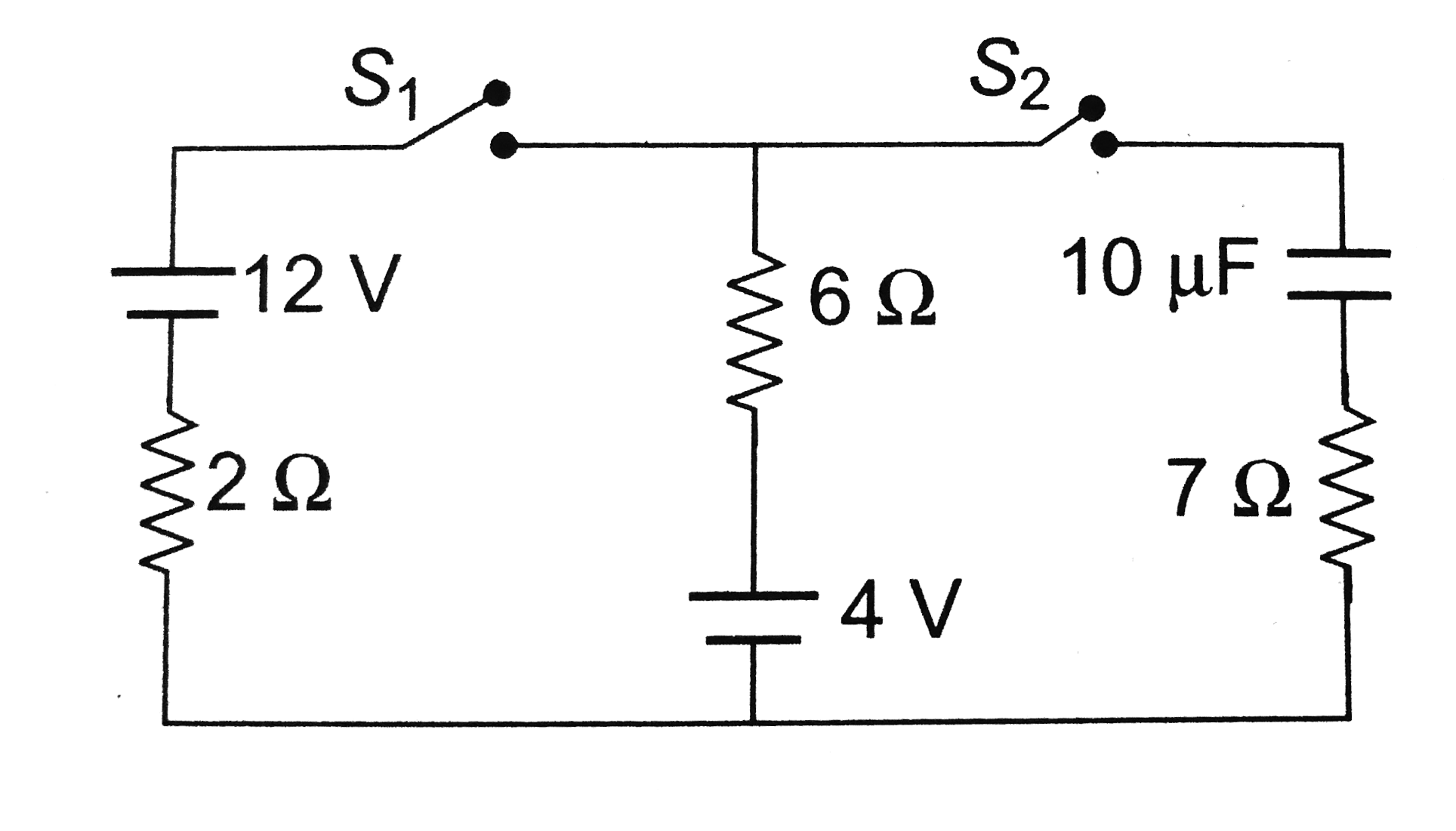
View Solution
