ABC is an equilateral triangle of side 2a. Find each of its altitudes.
a√3
a√2
2a√3
None
The correct Answer is:A
As ΔABC is an equilateral triangle, its altitude will also be the median.
If we draw a median AD to BC. Then,
BD=DCand∠ADB=90∘
We are given,
AB=AC=BC=2a
So,BD=DC=BC2=a
As, ΔABD is a right angle triangle,
AB2=AD2+BD2
AD2=(2a)2−a2=3a2
So,AD=√3a
As, it is an equilateral triangle, all its altitudes will be equal to √3a.
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.316videos
TRIANGLES
- BOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.210videos
TRIANGLES
- BOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.13videos
TRIANGLES
- BOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.39videos
SURFACE AREAS AND VOLUMES
Similar Questions
Construct an equilateral triangle of sides 6 cm each.
View SolutionFind the coordinates of the vertices of an equilateral triangle of side 2a as shown in Figure.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionWrite the negatin of each of the statement :
ABC is an equilateral triangle if and only if it is equiangular.View SolutionABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
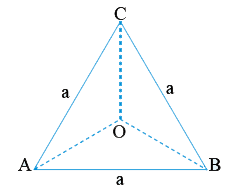
−−→AB+−−→BC+−−→CAView SolutionABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
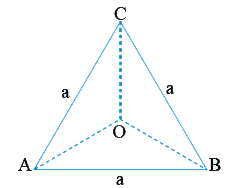
If −−→AB+−−→AC=n−−→AO then n = ?View SolutionABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
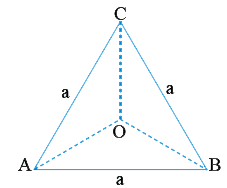
If ∣∣∣−−→AB+−−→BC+−−→AC∣∣∣=na then n = ?View SolutionABC is an equilateral triangle of side 2m. If →E=10NC−1 then VA−VB is
 View Solution
View Solution

