A particle of mass 1 g moves on a straight line. The variation of its velocity with time is shown in fig. Find the force acting on the particle at t = 1 s, 4 s and 7 s.
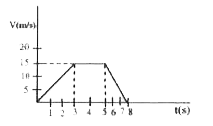
(i) At t = 1 s, acceleration of the particle is equal to the slope of the straight line from 0 to 3s as 1 s is between 0 and 3 s.
Acceleration of the particle at 1 = 1s is
a=vf−vitf−ti=15−03−0=5ms−2
∴ Force acting on the particle
=ma=1×10−3×5=5×10−3N.
(ii) At t = 4 s, acceleration is given by
a = slope of the straight line from t = 3 to 5 s
=vf−vitf−ti=15−155−3=02=0ms−2
∴ Force acting on the particle
=ma=1×10−3×0=0N
(iii) At t = 7 s, acceleration is given by
a = slope of the straight line from t = 5 to 8s
=vf−vitf−ti=0−158−5=153=−5ms−2
∴ Force acting on the particle
=ma=1×10−3×−5=−5×10−3N.
Negative sign indicates that the force is in the opposite direction of the motion of the particle.
Similar Questions
A particle of mass 1 g moves on a straight line. The variation of its velocity with time is shown in fig. Find the force acting on the particle att = 1 s,4s and 7 s.
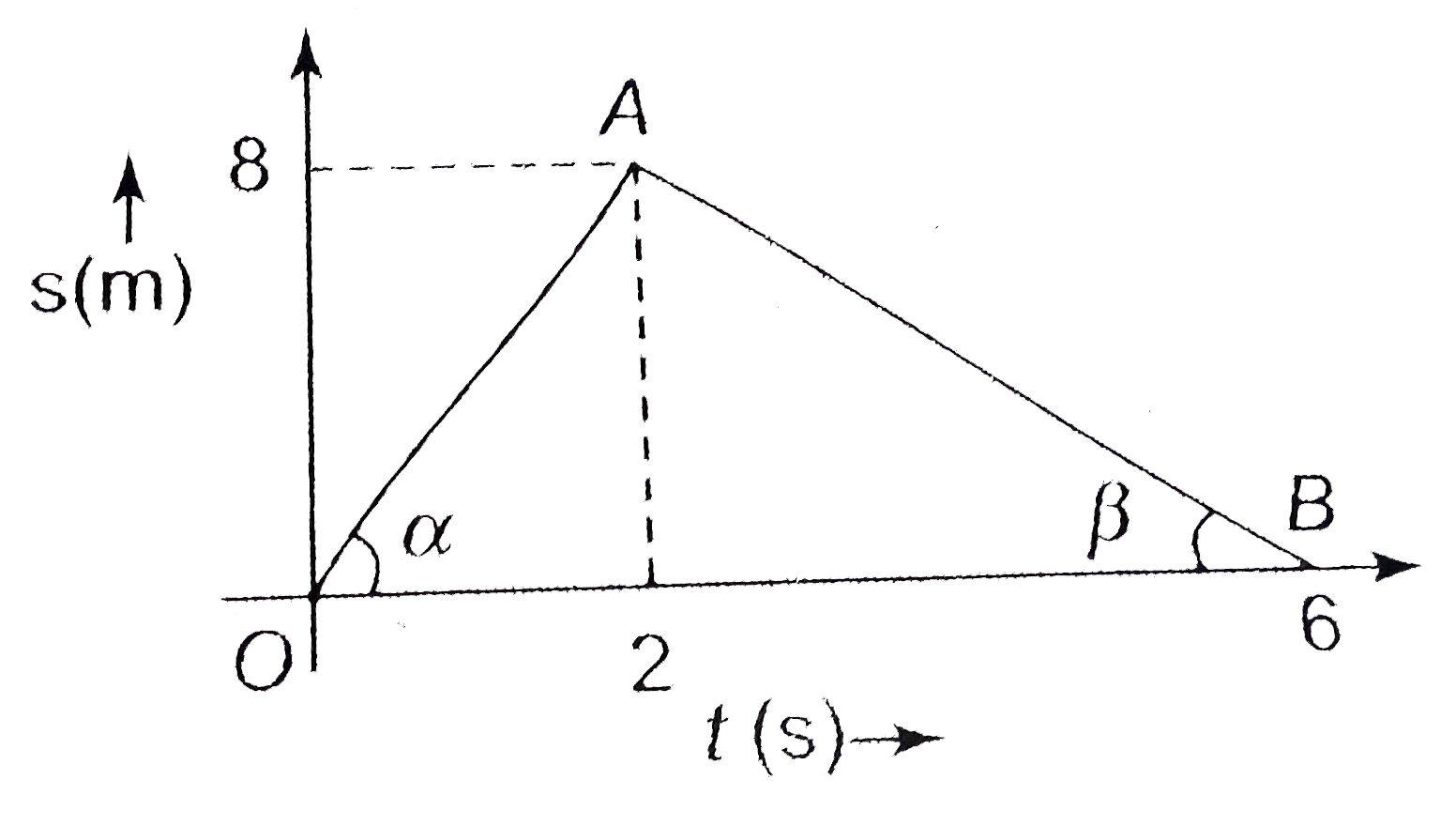
View SolutionFor a particle moving in a straight line, the position-time graph is as shown in the figure. Find the velocity of the particle at time t=1s and t=4s. Also sketch the v-t graph.
View Solution
Knowledge Check
Kinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
Ais constantBis increasingCis decreasingDfirst increase and then decreasesPower of the only force acting on a particle of mass m=1 kg moving in straight line depends on its velocity as P=v2 where v is in m/s and P is in watt. If initial velocity of the particle is 1m/s, then the displacement of the particle in ln2 second will be :
A(ln2−1)mB(ln2)2mC1mD2m
A particle starts motion from rest and moves along a straight line. Its velocity-time graph is shown. Find out speed of particle at t=2s and at t=3s.

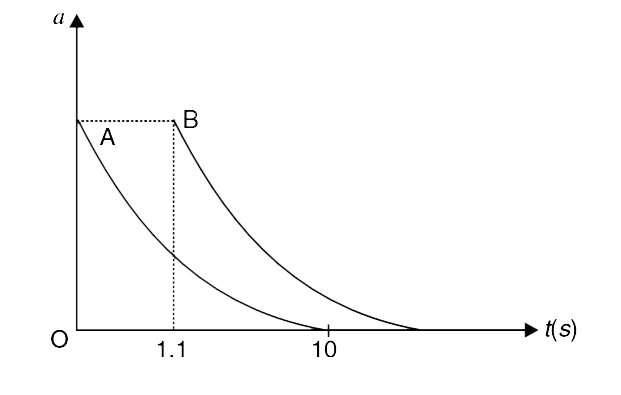
View SolutionParticle A starts from rest and moves along a straight line. Acceleration of the particle varies with time as shown in the graph. In 10 s the velocity of the particle becomes 60m/s and the acceleration drops to zero. Another particle B starts from the same location at time t=1.1s and has acceleration – time relationship identical to A with a delay of 1.1s. Find distance between the particles at time t=15s.
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
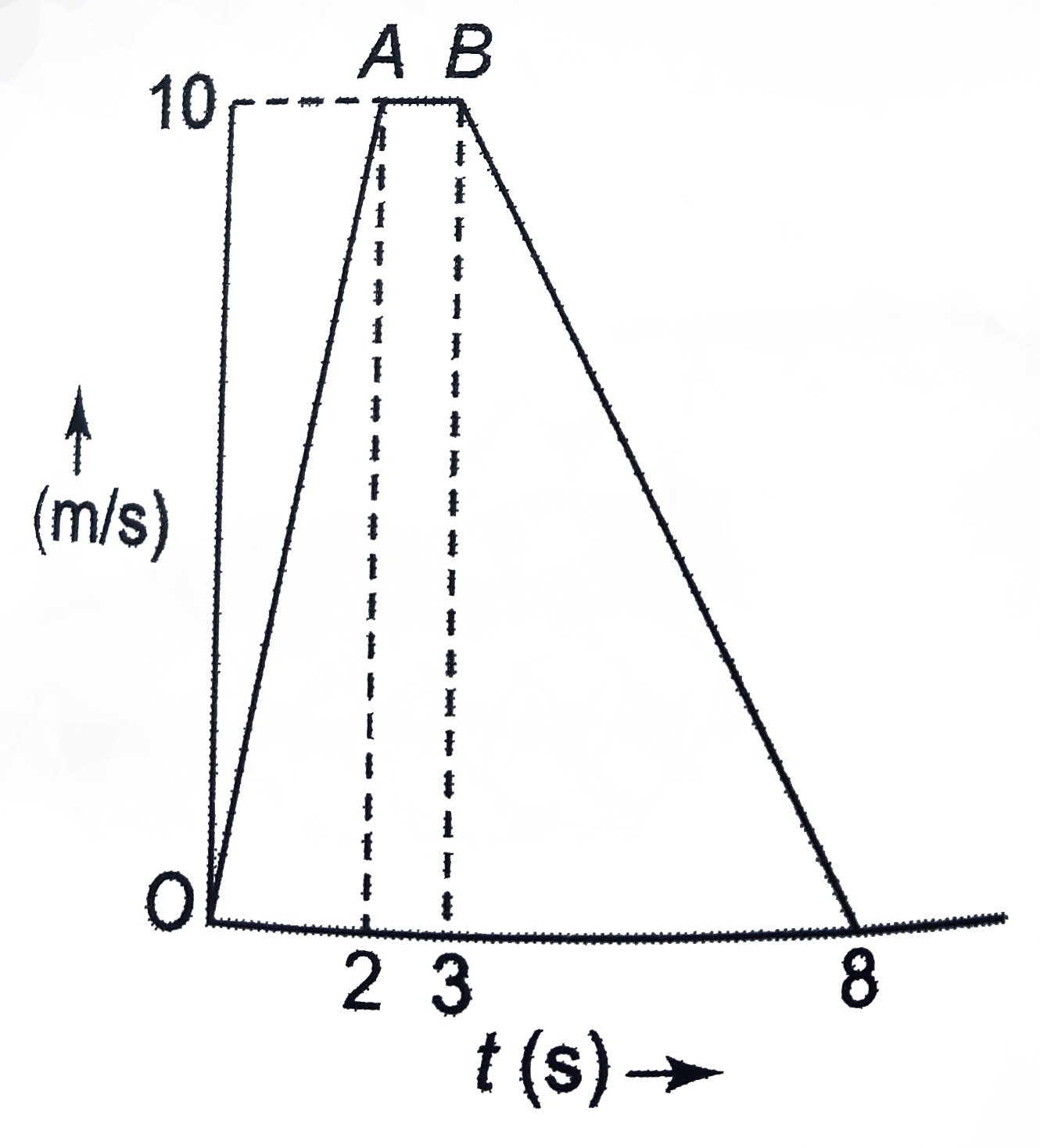
View SolutionFor a particle moving in a straight line, the velocity-time graph is as shown in the figure. Find the acceleration at time t=1s2.5s and 5s. Sketch the acceleration time graph.
View SolutionA particle is moving in a straight line and its acceleration-time graph is shown below. At t=0, the particle is at rest. Find the velocity of the particle at t=3s,6s and 8s. Also sketch the v-s graph.
View SolutionPower of the only force acting on a particle of mass m=1 kg moving in straight line depends on its velocity as P=v2 where v is in m/s and P is in watt. If initial velocity of the particle is 1m/s, then the displacement of the particle in ln2 second will be :
View Solution
