In the given figure, O is the centre of the given circle, and chords AB and CD intersect at a point E inside the circle.
Prove that
∠AOC+∠BOD=2∠AEC
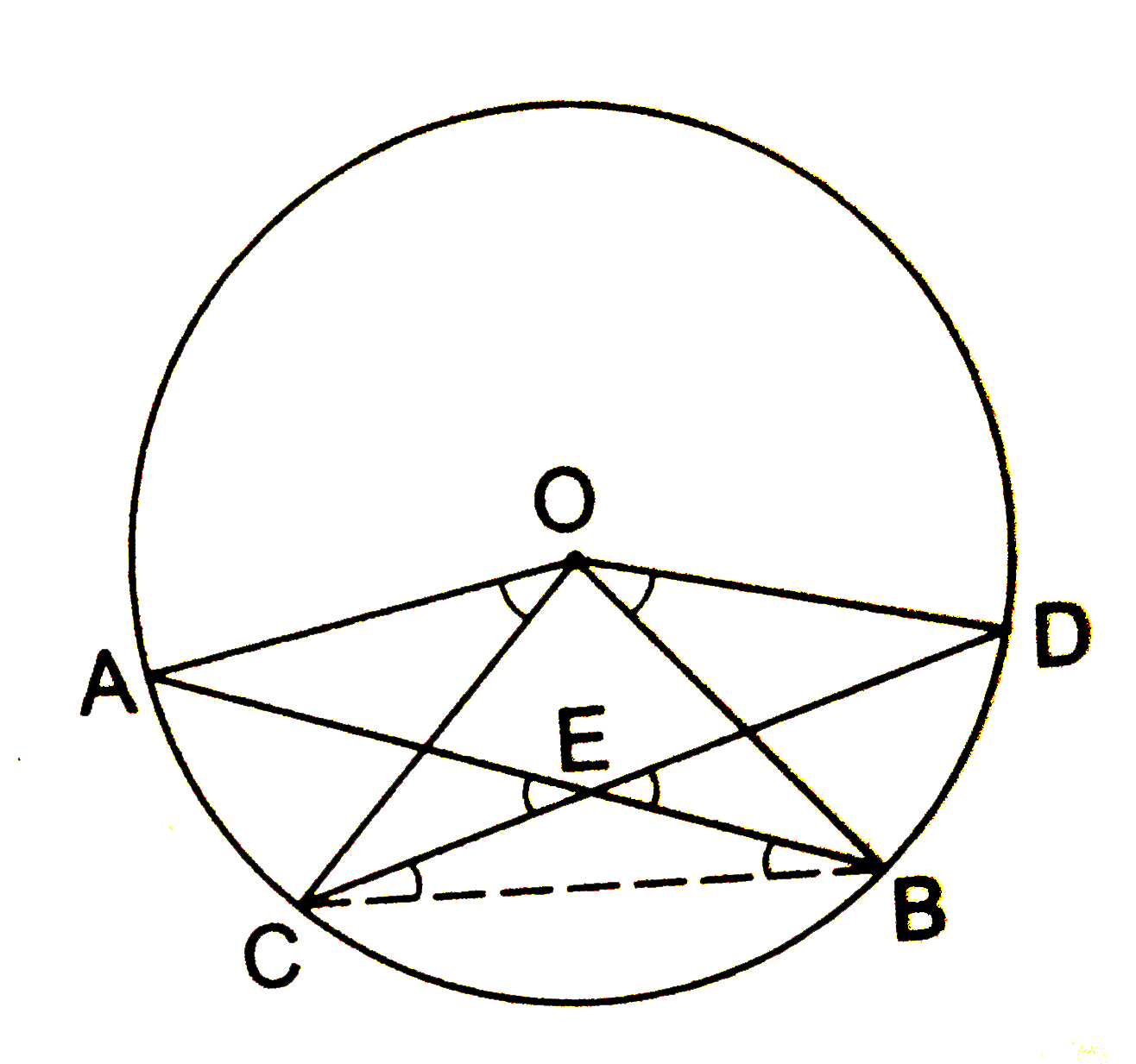
Join CB.
We know that the angle made by ˆAC at the centre O is double the angle made by it at a point B on the remaining part of the circle.
∴∠AOC=2∠ABC. ....(i)
Similarly, the anglemade by ˆBD at the centre O is double the angle made by it at a point C on the remaining part of the circle.
∴∠BOD=2∠BCD ...(ii)
∴∠AOC+∠BOD=2(∠ABE+∠BCD) [adding (i) and (ii) ]
=2(∠EBC+∠BCE)
=2(180∘−∠CEB) [ ∵ sum of ∠s of ΔECB is 180∘ ]
=2[180∘−(180∘−∠AEC)]
=2∠AEC.
Hence, ∠AOC+∠BOD=2∠AEC.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Exercise 12A22videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B18videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B16videos
BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGON
- BOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)20videos
CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLE
Similar Questions
In the given figure, O is the centre of the circle.

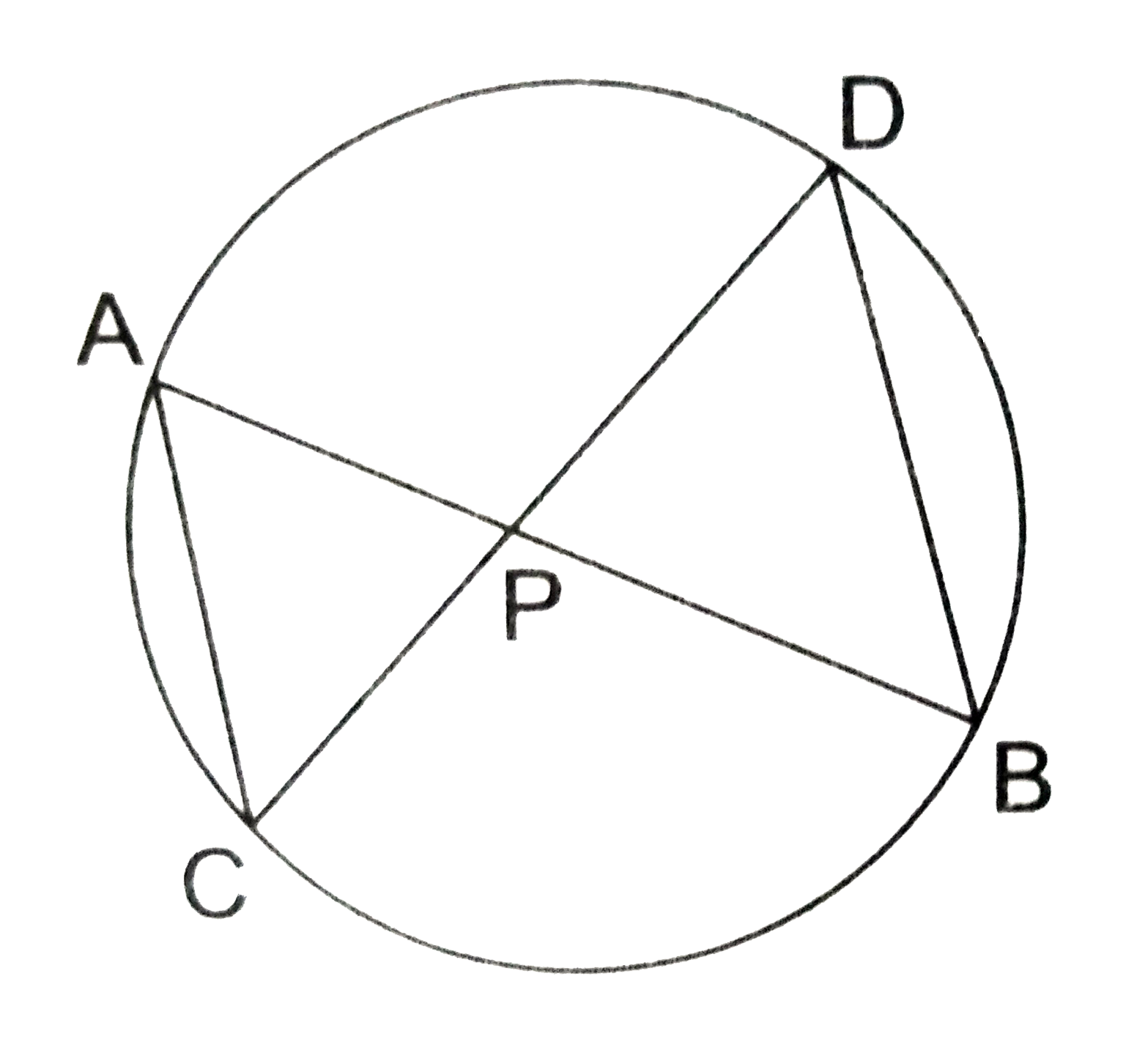
Name all chords of the circle.View SolutionIn a cricle, two chords AB and CD intersect at a point P inside the circle. Prove that
(a)ΔPAC~ΔPDB (b)PA⋅PB=PC⋅PD.
 View Solution
View SolutionIn a circle with centre O, chords ABandCD intersect inside the circumference at E. Prove that ∠AOC+∠BOD=2∠AEC.
View SolutionIn a circle with centre O, chords AB and CD intersect inside the circumference at E. Prove that ∠AOC+ ∠BOD=2 ∠AEC
View SolutionIn a circle with centre O,chords AB and CD intersect inside the circumference at E.Prove that ∠AOC+∠BOD=2∠AEC
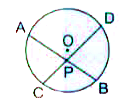
View SolutionIn the given figure, O is the centre of the circle. Its two chords AB and CD intersect each other at the point P within the circle. If AB = 18 cm, PB = 8 cm, CP = 5 cm, then find the length of CD.
 View Solution
View SolutionIn the given figure, O is the centre of the circle. Its two chords AB and CD intersect each other at the point p within the circle. If AB=15cm,PB=9cm, then find the length of PD.
 View Solution
View SolutionIn the given figure, O is the centre of the circle.

Name a chord, which is not the diameter of the circle.View SolutionIn the figure, O is the centre of the circle. Prove ∠AOC=∠AFC+∠AEC.
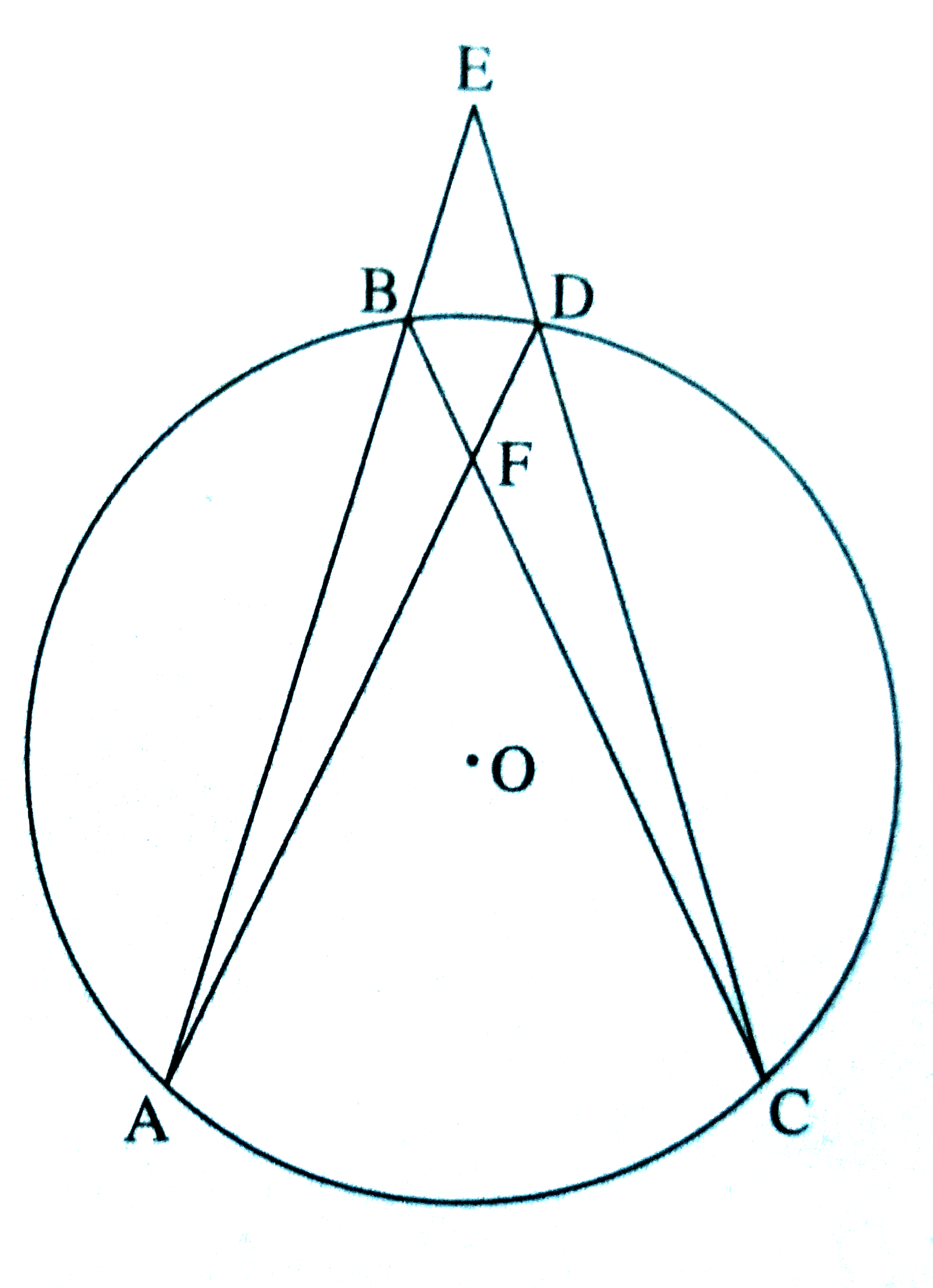 View Solution
View SolutionIn a circle, chords AB and CD intersect at a point R inside the circle. If AR : RB = 1:4 and CR: RD = 4:9, then the ratio AB: CD is
View Solution

