Question

In Figure, $ABandCD$
are respectively the
smallest and longest sides of a quadrilateral $ABCD\stackrel{.}{}$
Show that $\angle A>\angle Cand\angle B>\angle D$

Explanation

Given in quadrilateral ABCD,AB is the smallest and CD is the largest side
To find `angel B gt angle D or angle D gt angle B`
Construction join BD.
Now in `Delta ABD AD gt AB ` [since ,AB is the snallest side in ABCD ]

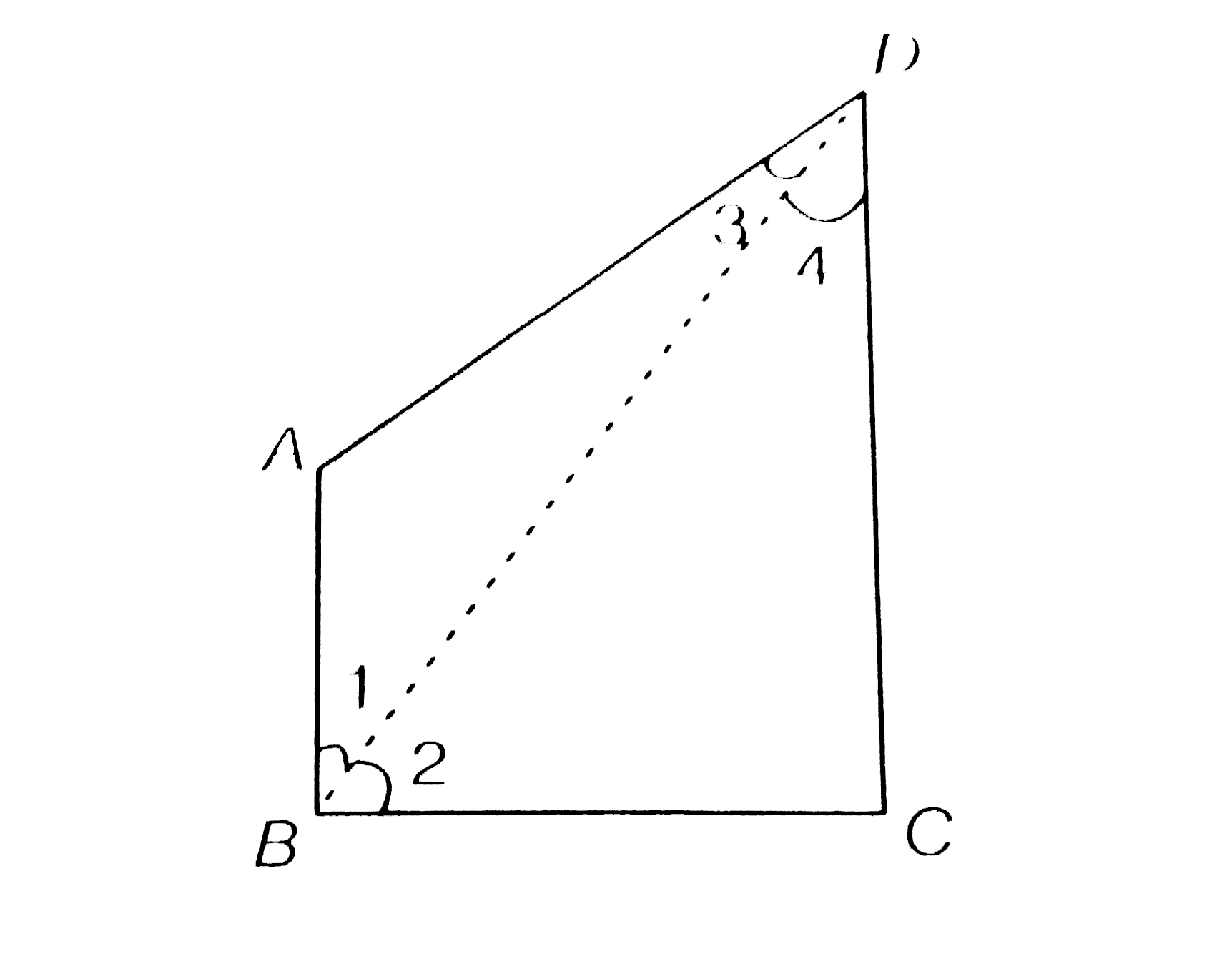
`rArr angle 1 gt angel 3 ` [angle opposite to larger side is greater ] ...(i)
` In Delta ABCD CD gt BC ` [ since ,CD is the largest side is grater ] ..(i)
` rArr angel 2 gt angle 4 ` [angle opposite to larger side is grater ]
On adding Eqs. (i) and (ii) we get
`angel 1 + angle 2 + gt angle 3 + angle 4`
Hence `angle B gt angle D`