Draw the graph of the relation $4 y^{2} = x^{2} (4 - x^{2})$

Video Solution

Text Solution

Verified by Experts

We have $4 y^{2} = x^{2} (4 - x^{2})$
$∴ y = \pm \frac{1}{2} \sqrt{x^{2} (4 - x^{2})}$
$∴ y = \pm \frac{x}{2} \sqrt{4 - x^{2}}$
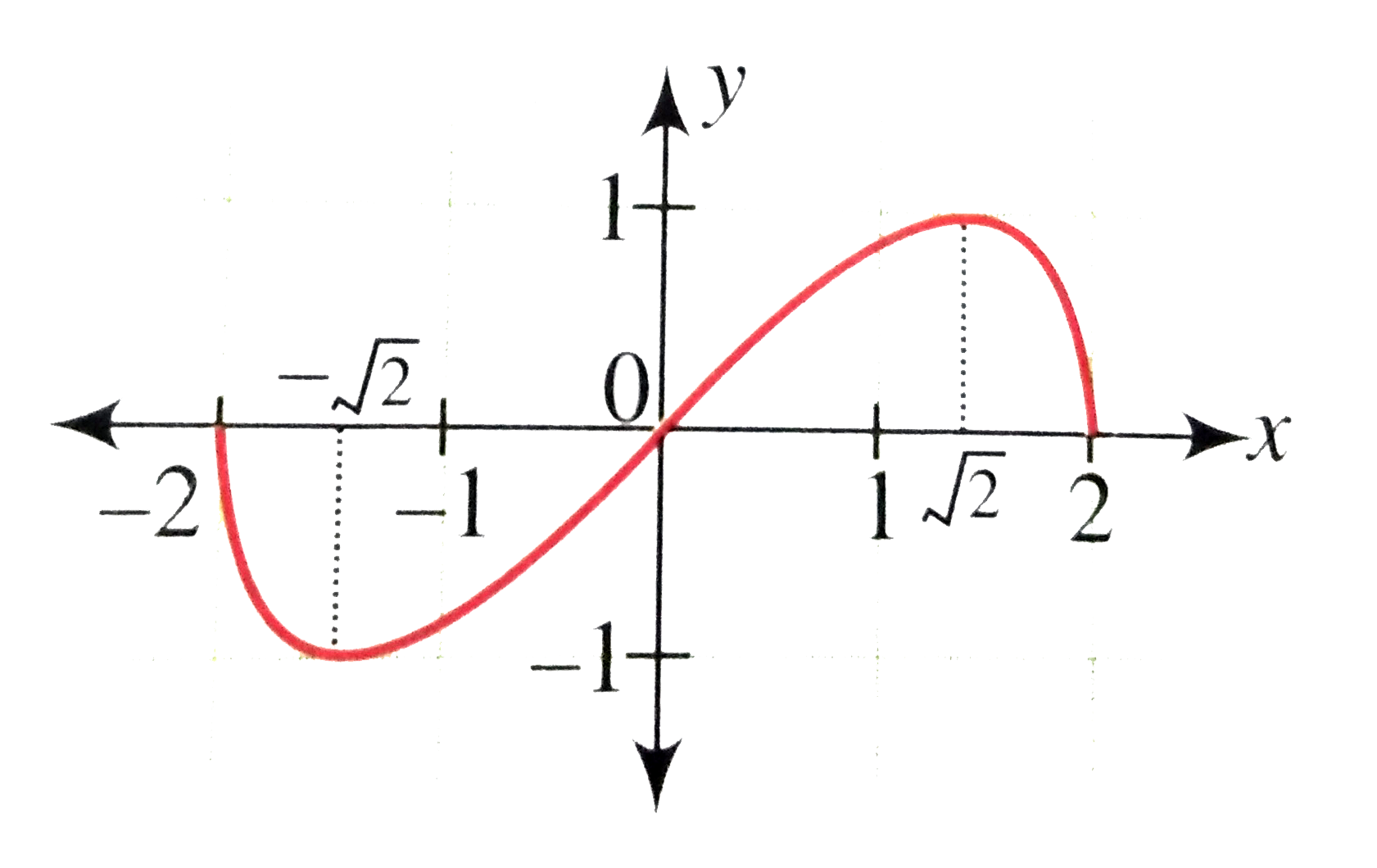
Let us first draw the graph of $y = f (x) = \frac{x}{2} \sqrt{4 - x^{2}}$
Clearly, the domain is $[- 2.2]$
Now, $f (0) = f (- 2) = f (2) = 0$
Also for $x < 0, f (x) < 0$ and for $x > 0, f (x) > 0$
Now $\frac{d y}{d x} = \frac{1}{2} \sqrt{4 - x^{2}} - \frac{x}{2} \frac{x}{\sqrt{4 - x^{2}}}$
$\Rightarrow 4 - x^{2} = x^{2}$
$\Rightarrow x = \pm \sqrt{2}$
Since $f (x) > 0$ for $x < 0$ and $f (0) = f (2) = 0, x = \sqrt{2}$ is the point of maxima and $x = - \sqrt{2}$ is the point of minima.
$f (\sqrt{2}) = 1, f (- \sqrt{2}) = - 1$
Also $f (x)$ is an odd function.
Hence, the graph of the function is as shown in the following figure.

Graph of $y = - \frac{x}{1} \sqrt{4 - x^{2}}$ can be obtained by reflecting the above graph in the x-axis.
Hence, the graph of the relation $4 y^{2} = x^{2} (4 - x^{2})$ is a follows.

Updated on:21/07/2023

|

Class 12 MATHS FUNDAMENTAL OF MATHEMATICS

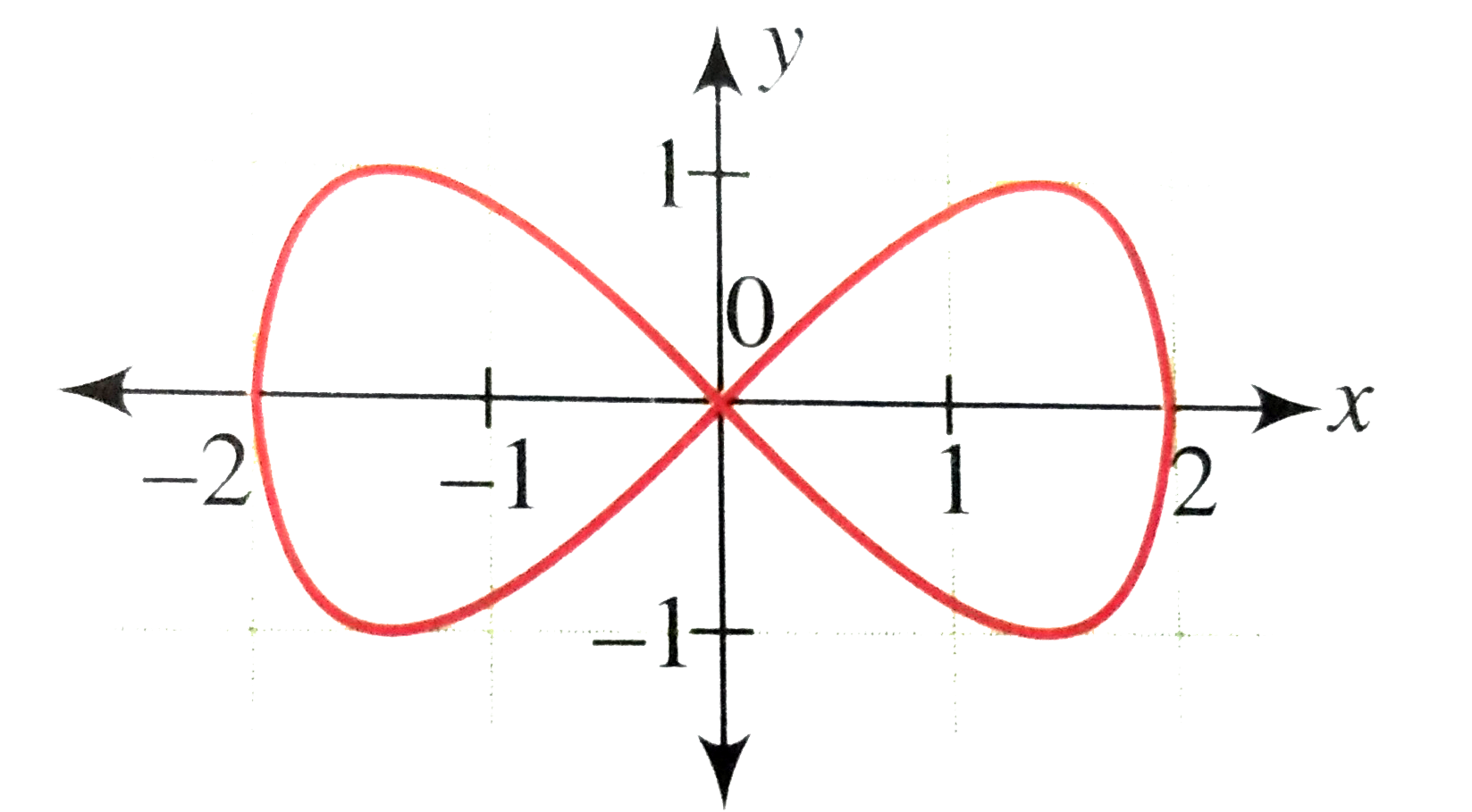
Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-CURVE TRACING-EXERCISES

Home
Ask Doubt
Profile