From an arbitrary point $P$ on the circle $x^{2} + y^{2} = 9$ , tangents are drawn to the circle $x^{2} + y^{2} = 1$ , which meet $x^{2} + y^{2} = 9$ at $A$ and $B$ . The locus of the point of intersection of tangents at $A$ and $B$ to the circle $x^{2} + y^{2} = 9$ is
(a) $x^{2} + y^{2} = {(\frac{27}{7})}^{2}$ (b) $x^{2} - y^{2} {(\frac{27}{7})}^{2}$ $y^{2} - x^{2} = {(\frac{27}{7})}^{2}$ (d) none of these

A

$x^{2} + y^{2} = {(27 / 7)}^{2}$

B

$x^{2} - y^{2} = {(27 / 7)}^{2}$

C

$y^{2} - x^{2} = {(27 / 7)}^{2}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1

Since $Δ P O Q$ and $Δ A O Q$ are congruent,
$∠ P O Q = ∠ Q O A = θ$

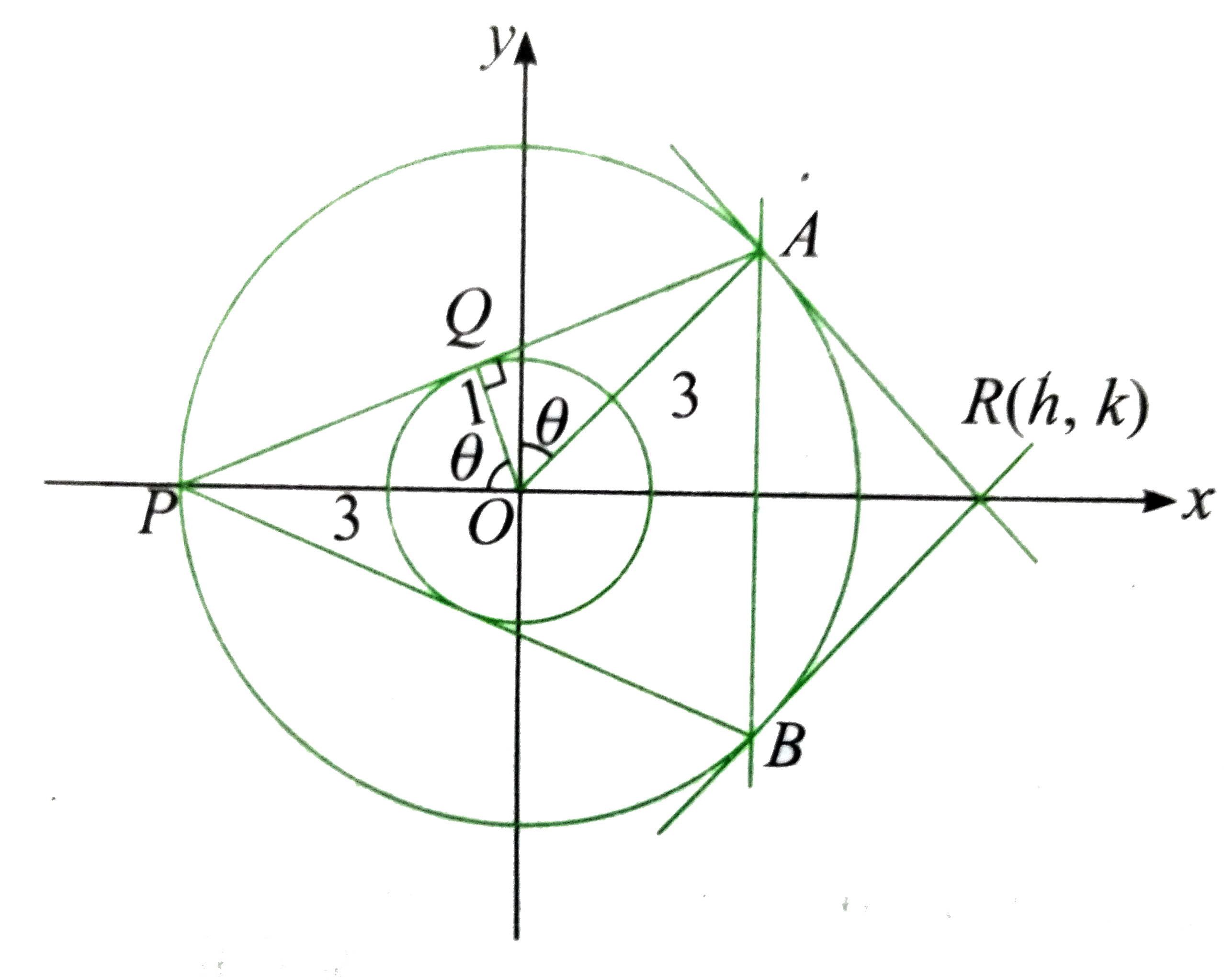
$cos θ = \frac{1}{2},$ since $∠ P O R = π$
$∴ ∠ A O R = π - 2 θ$
Now, in triangle $A O R, ∠ A O R = π - 2 θ$ and $A O = 3$ units
Therefore,
$cos (π - 2 θ) = \frac{O A}{O R} = \frac{3}{\sqrt{h^{2} + k^{2}}}$
or $\sqrt{h^{2} + k^{2}} = \frac{27}{7}$
or $x^{2} + y^{2} = {(\frac{27}{7})}^{2}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-CIRCLE -Excercises (Single Correct Answer Type)

Home
Ask Doubt
Profile