A pendulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road. Find the angle made by the string with the vertical.
To find the angle made by the string of a pendulum with the vertical when the pendulum is hanging from the ceiling of a car that is accelerating with respect to the road, we can follow these steps:
Step 1: Identify the Forces Acting on the Pendulum Bob
The forces acting on the pendulum bob of mass m are:
- The gravitational force →Fg=mg acting downward.
- The tension →T in the string acting along the string.
- A pseudo force →Fp=ma0 acting horizontally in the opposite direction of the car's acceleration a0.
Step 2: Set Up the Coordinate System
We can set up a coordinate system where:
- The vertical direction (y-axis) is directed downward.
- The horizontal direction (x-axis) is directed in the direction of the car's acceleration.
Step 3: Resolve the Tension into Components
The tension T in the string can be resolved into two components:
- A vertical component Tcosθ acting upward.
- A horizontal component Tsinθ acting horizontally.
Step 4: Apply Equilibrium Conditions
Since the pendulum bob is in equilibrium (it is not moving), we can apply the equilibrium conditions for the forces in both the x and y directions.
In the x-direction:
The net force must be zero:
Tsinθ=ma0(1)
In the y-direction:
The net force must also be zero:
Tcosθ=mg(2)
Step 5: Solve for sinθ and cosθ
From equation (1):
sinθ=ma0T(3)
From equation (2):
cosθ=mgT(4)
Step 6: Divide Equation (3) by Equation (4)
Dividing equation (3) by equation (4) gives:
sinθcosθ=ma0mg
This simplifies to:
tanθ=a0g
Step 7: Find the Angle θ
To find the angle θ, we take the inverse tangent:
θ=tan−1(a0g)
Final Answer
The angle made by the string with the vertical is:
θ=tan−1(a0g)
---
The forces acting on the pendulum bob of mass m are:
- The gravitational force →Fg=mg acting downward.
- The tension →T in the string acting along the string.
- A pseudo force →Fp=ma0 acting horizontally in the opposite direction of the car's acceleration a0.
We can set up a coordinate system where:
- The vertical direction (y-axis) is directed downward.
- The horizontal direction (x-axis) is directed in the direction of the car's acceleration.
The tension T in the string can be resolved into two components:
- A vertical component Tcosθ acting upward.
- A horizontal component Tsinθ acting horizontally.
Since the pendulum bob is in equilibrium (it is not moving), we can apply the equilibrium conditions for the forces in both the x and y directions.
The net force must be zero:
Tsinθ=ma0(1)
The net force must also be zero:
Tcosθ=mg(2)
From equation (1):
sinθ=ma0T(3)
cosθ=mgT(4)
Dividing equation (3) by equation (4) gives:
sinθcosθ=ma0mg
This simplifies to:
tanθ=a0g
To find the angle θ, we take the inverse tangent:
θ=tan−1(a0g)
The angle made by the string with the vertical is:
θ=tan−1(a0g)
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - worked out Examples
11 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Objective -1
14 Videos
- LAWS OF THERMODYNAMICSBOOK - HC VERMA ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - All Questions
64 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
Similar Questions
A pendulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road. Find the angle made by the string with vertical at equilibrium. Also find the tension in the sting in this position

View SolutionA simple pendulum is suspended from the ceiling of a car taking a turn of radius 10 m at a speed of 36 km/h. Find the angle made by the string of the pendulum with the vertical if this angle does not change during the turn. Take g=10ms2.
View SolutionA pendulum is hanging from the ceiling of a cage. When the cage is moving up with certain acceleration and when it is moving down with the same acceleration, the tensions in the string are T1 and T2 respectively. When the cage moves horizontally with the same acceleration, the tension in the string is,
View SolutionA pendulum is hanging from the ceiling of a trolley which moves down a smooth inclined plane. Then
View SolutionA simple pendulum is suspended from the ceiling of trolley which is sliding down on a inclined plane of inclination θ Find the angle made by the string with normal to the trolley a) when trolley slides down with uniform velocity b) when the plane is smooth.
View SolutionA small ball is suspended by a string from the ceiling of a car. As the car accelerates at a rate ‘a’ the string makes an angle '/theta' with the vertical in equilibrium. Then the tension in the string is :
View SolutionA small sphere is suspended by a string from the ceiling of a car. If the car beings to move with a constant acceleration a, the inclination of the string to the vertical is:
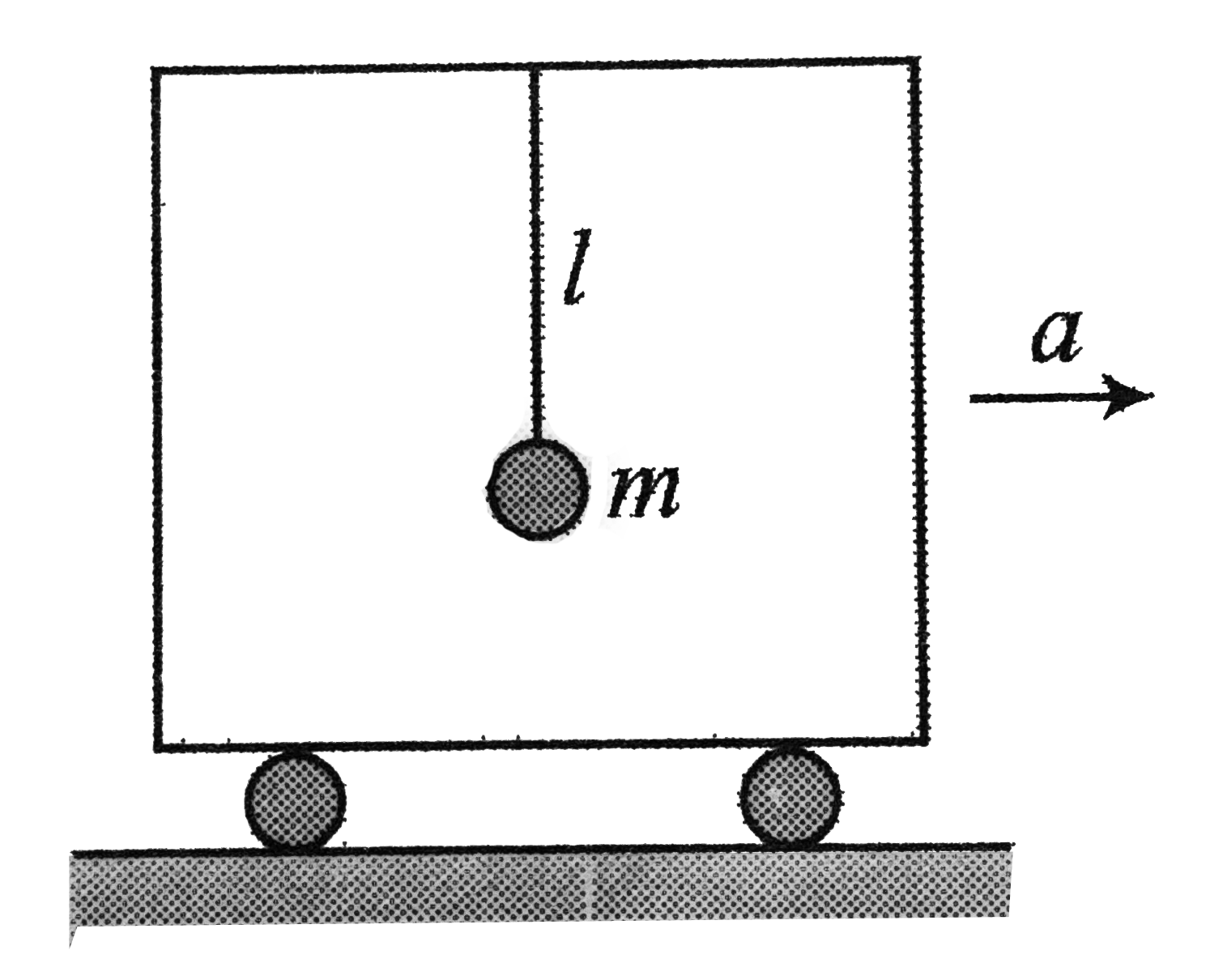
View SolutionA pendulum of mass m and length l is suspended from the ceiling of a trolley which has a constant acceleration a in the maximum deflection θ of the pendulum from the vertical.
View SolutionA bob of mass m attached to an inextensible string of length l is suspended from a verticle support. The bob rotates in a horizontal circle with an angular speed ωrad/s about the verticle. About the point of suspension.
View SolutionA small metallic sphere of mass m is suspended from the ceiling of a car accelerating on a horizontal road with constant acceleration a. The tension in the string attached with metallic sphere is
View Solution
