A heavy particle of mass m falls freely near the earth's surface.Whati si the torque acting on this particle about a point 50 cm east to the lie of motiin? Does this a point 50 cm east to the line of motion? Does this toruque produce any angular accelertio in the particle?
To solve the problem step by step, we will first calculate the torque acting on the particle and then determine if this torque produces any angular acceleration.
Step 1: Identify the Forces Acting on the Particle
The only force acting on the particle as it falls freely is its weight, which is given by:
F=mg
where m is the mass of the particle and g is the acceleration due to gravity (approximately 9.8m/s2).
Step 2: Determine the Distance from the Line of Motion
The problem states that we need to calculate the torque about a point that is 50 cm (or 0.5 m) east of the line of motion. This distance will be denoted as r:
r=0.5m
Step 3: Calculate the Torque
Torque (τ) is calculated using the formula:
τ=r×F
Substituting the values we have:
τ=r×mg
τ=0.5m×mg
Thus, the torque acting on the particle is:
τ=0.5mg
Step 4: Determine if Torque Produces Angular Acceleration
Angular acceleration (α) is given by the formula:
α=τI
where I is the moment of inertia. For a point mass m at a distance r from the axis of rotation, the moment of inertia is:
I=mr2
However, in this case, since the particle is falling straight down, we consider the center of rotation to be at an infinite distance from the particle. Therefore, the moment of inertia becomes:
I=m⋅(∞)2=∞
Step 5: Calculate Angular Acceleration
Substituting the values into the angular acceleration formula:
α=τI=0.5mg∞=0
Conclusion
Thus, the torque acting on the particle about the point 50 cm east to the line of motion is 0.5mg, and this torque does not produce any angular acceleration in the particle.
The only force acting on the particle as it falls freely is its weight, which is given by:
F=mg
where m is the mass of the particle and g is the acceleration due to gravity (approximately 9.8m/s2).
The problem states that we need to calculate the torque about a point that is 50 cm (or 0.5 m) east of the line of motion. This distance will be denoted as r:
r=0.5m
Torque (τ) is calculated using the formula:
τ=r×F
Substituting the values we have:
τ=r×mg
τ=0.5m×mg
Thus, the torque acting on the particle is:
τ=0.5mg
Angular acceleration (α) is given by the formula:
α=τI
where I is the moment of inertia. For a point mass m at a distance r from the axis of rotation, the moment of inertia is:
I=mr2
I=m⋅(∞)2=∞
Substituting the values into the angular acceleration formula:
α=τI=0.5mg∞=0
Thus, the torque acting on the particle about the point 50 cm east to the line of motion is 0.5mg, and this torque does not produce any angular acceleration in the particle.
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
Similar Questions
A particle falls freely near the surface of the earth. Consider a fixed point O (not vertically below the particle) on the ground.
View SolutionA particle of mass m is projected with a speed v at an angle θ with the horizontal. Find the angular momentum of the particle about an axis passing through point of projection and perpendicular to the plane of motion of the particle.
(a) When the particle is at maximum height and
(b) When the particle is just about to collide with the horizontal surface
View SolutionA particle starts moving along a straight line path with velocity 10m/s. After 5s the distance of the particle from the starting point is 50m. Which of the following statements about the nature of motion of the particle are correct?
View SolutionA particle of mass m is released from rest at point A in Fig., falling parallel to the (vertical) y-axis. Find the angular momentum of the particle at any time t with respect to the same origin O.
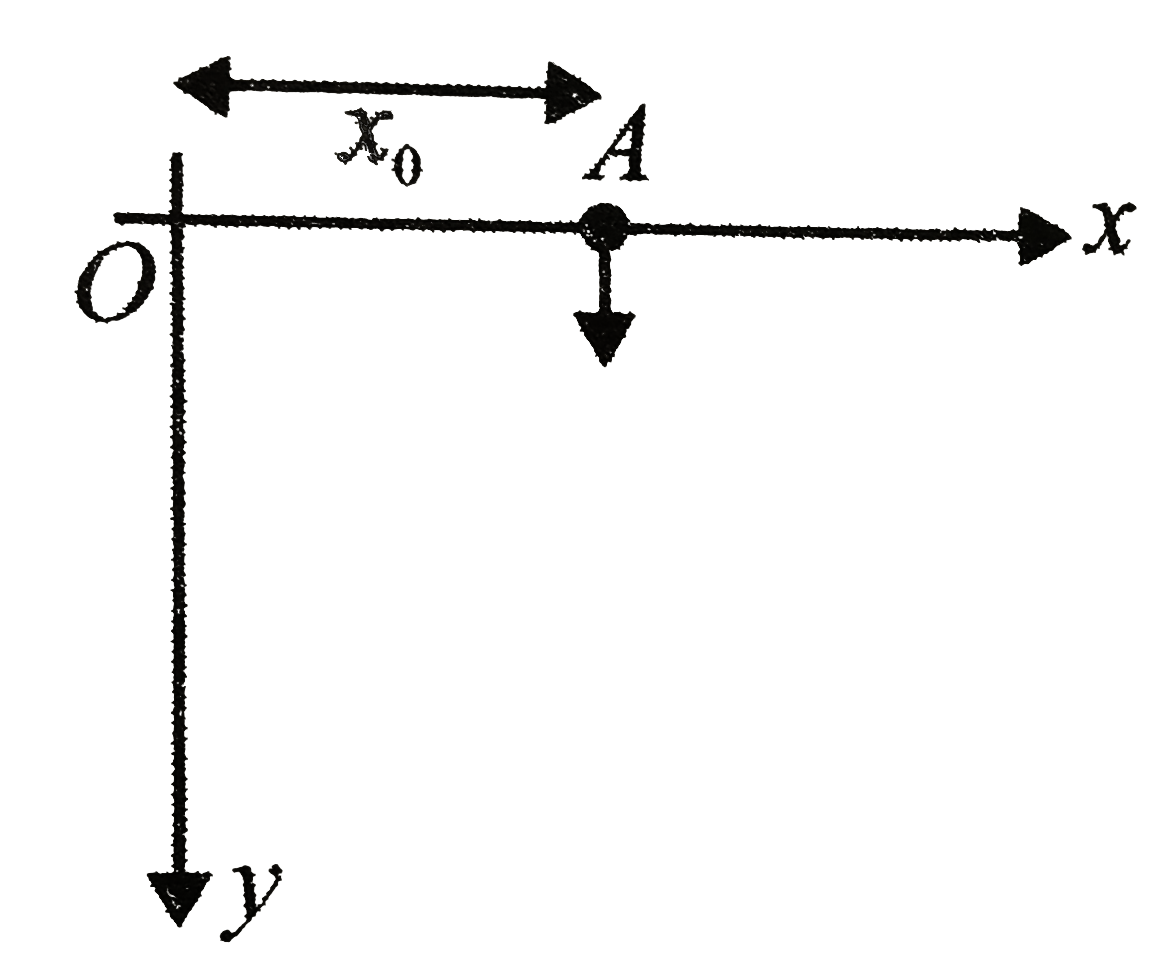
View SolutionA particle moving with uniform acceleration along a straight line ABC rosses point A at t=0 with a velocity 12ms−1.B is 40m away from A andC is 64m away from A. The particle passes B at t=4 s.a.Afterwˆtimewillthepartic≤beatC?b.WˆisitsvelocityatC?c.Wˆdoesthepartic≤reachAaga∈?d.Locatethep∮wherethepartic≤reversesitsdirectionofmotion.e.F∈dthedistancecoveredbythepartic≤∈thefirst15 s.
View SolutionA massive horizontal platform is moving horizontally with a constant acceleration of 10m/s2 as shown in the figure. A particle P of mass m=1 kg is kept at rest on a smooth surface as shown in the figure. The particle is hinged at O with the help of a massless rod OP of length 0.9 m. Hinge O is fixed on the platform and the rod can freely rotate about O. Now the particle P is imparted a velocity in the opposite direction of the platform's acceleration such that it is just able to complete the circular motion about point O. Then the maximum tension (in N) appearing in the rod during the motion is 10 n. Find the value of n

View SolutionA large insulating thick sheet of thickness 2d is charged with a uniform volume charge density p. A particle of mass m, carrying a charge q having a sign opposite to that of the sheet, is released from the surface of the sheet. The sheet does not offer any mechanical resistance to the motion of the particle. Find the oscillation frequency v of the particle inside the sheet
View SolutionA particle of mass 1 g executes an oscillatory motion on the concave surface of a spherical dish of radius 2m placed on a horizontal plane, Figure . If the motion of the particle begins from a point on the disc at a height of 1 cm. from the horizontal plane and coefficient of friction is 0.01 , find the total distance covered by the particle before coming to rest.
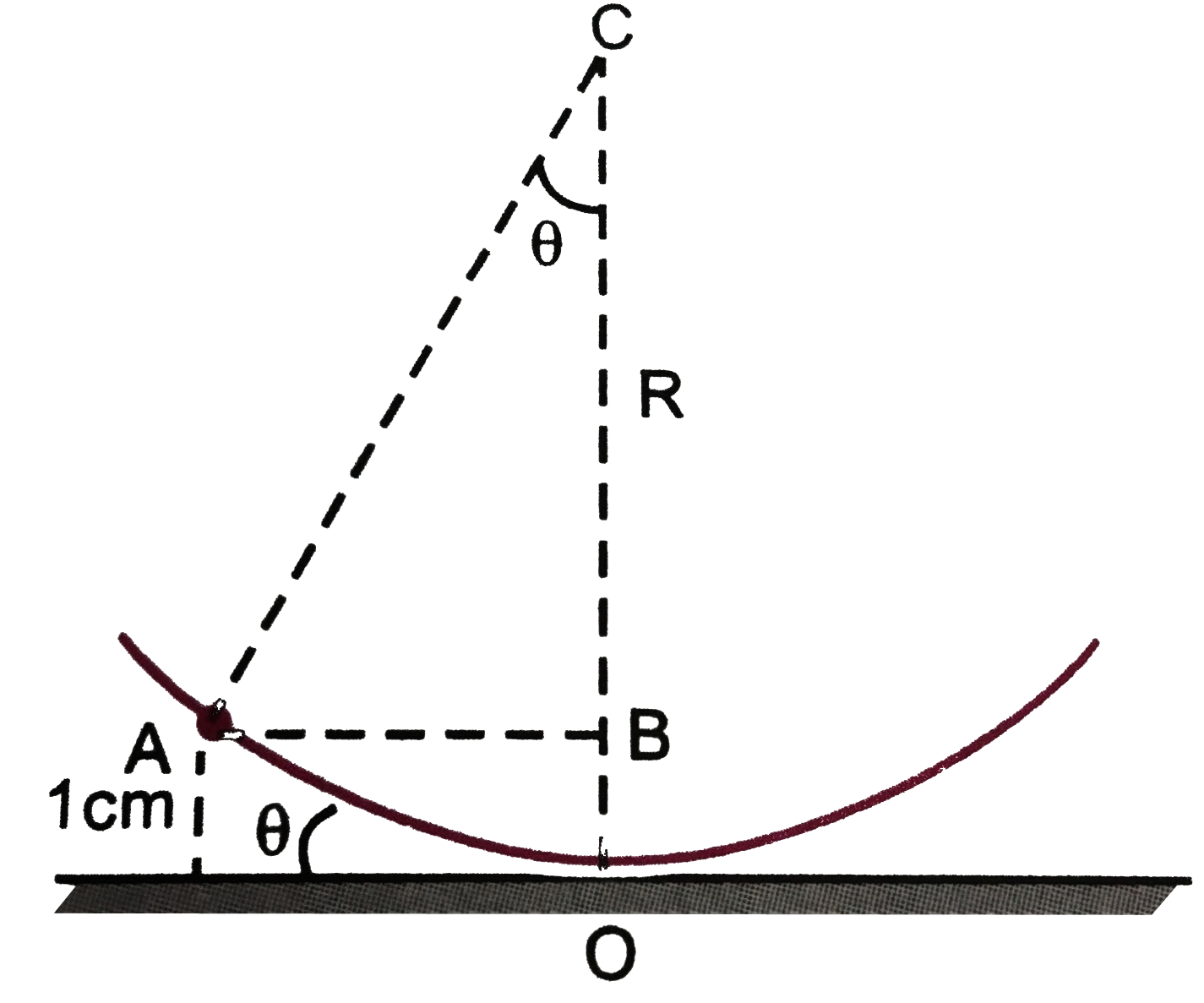
View SolutionA particle of mass m is released in vertical plane from a point P at x=x0 on the x-axis. It falls vertically parallel to the y-axis. Find the torque τ acting on the particle at a time about origin.

View SolutionTwo particles each of mass m and speed v, travel in opposite direction along parallel lines separated by a distance d. Show that the vector angular momentum of this system of particles is the same about any point taken as origin.
View Solution
