Light is incident from glass (μ=1.50) to water (μ=1.33) find the range of the angle of deviation for which there are two angles of incidence.
The correct Answer is:A, C
To solve the problem of finding the range of the angle of deviation for light incident from glass (μ = 1.50) to water (μ = 1.33), we can follow these steps:
Step 1: Identify the refractive indices
We have:
- Refractive index of glass (μ₁) = 1.50 (denser medium)
- Refractive index of water (μ₂) = 1.33 (rarer medium)
Step 2: Determine the critical angle
The critical angle (θ_C) can be found using Snell's law. The formula for the critical angle when light travels from a denser medium to a rarer medium is given by:
sin(θC)=μ2μ1
Substituting the values:
sin(θC)=1.331.50=43⋅11.5=89
Step 3: Calculate the critical angle
Now, we calculate θ_C using the inverse sine function:
θC=sin−1(89)
Calculating this gives:
θC≈62.73∘
Step 4: Calculate the angle of deviation at critical angle
The angle of deviation (Δ) is given by:
Δ=90∘−θC
Substituting the value of θ_C:
Δ=90∘−62.73∘≈27.27∘
Step 5: Determine the range of the angle of deviation
For the range of angles of deviation where there are two angles of incidence, we consider:
- At normal incidence (θ = 0), the deviation is 0°.
- At the critical angle (θ_C), the deviation is approximately 27.27°.
Thus, the range of the angle of deviation for which there are two angles of incidence is:
Δ ranges from 0∘ to 27.27∘
Final Answer
The range of the angle of deviation for which there are two angles of incidence is:
Δ:0∘ to 27.27∘
---
We have:
- Refractive index of glass (μ₁) = 1.50 (denser medium)
- Refractive index of water (μ₂) = 1.33 (rarer medium)
The critical angle (θ_C) can be found using Snell's law. The formula for the critical angle when light travels from a denser medium to a rarer medium is given by:
Now, we calculate θ_C using the inverse sine function:
The angle of deviation (Δ) is given by:
For the range of angles of deviation where there are two angles of incidence, we consider:
- At normal incidence (θ = 0), the deviation is 0°.
- At the critical angle (θ_C), the deviation is approximately 27.27°.
The range of the angle of deviation for which there are two angles of incidence is:
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXAMPLE
9 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
Light is incident from glass (μg=32) to water (μw=43). Find the range of the angle of deviation for refracted light.
View SolutionLight falls from glass (μ=1.5) to air. Find the angle of incidence for which the angle of deviation is 90∘.
View SolutionLight is incident from glass (μ=1.5) to air. Sketch thevariation of the angle of deviation δ with angle of incident i for 0<i<90∘
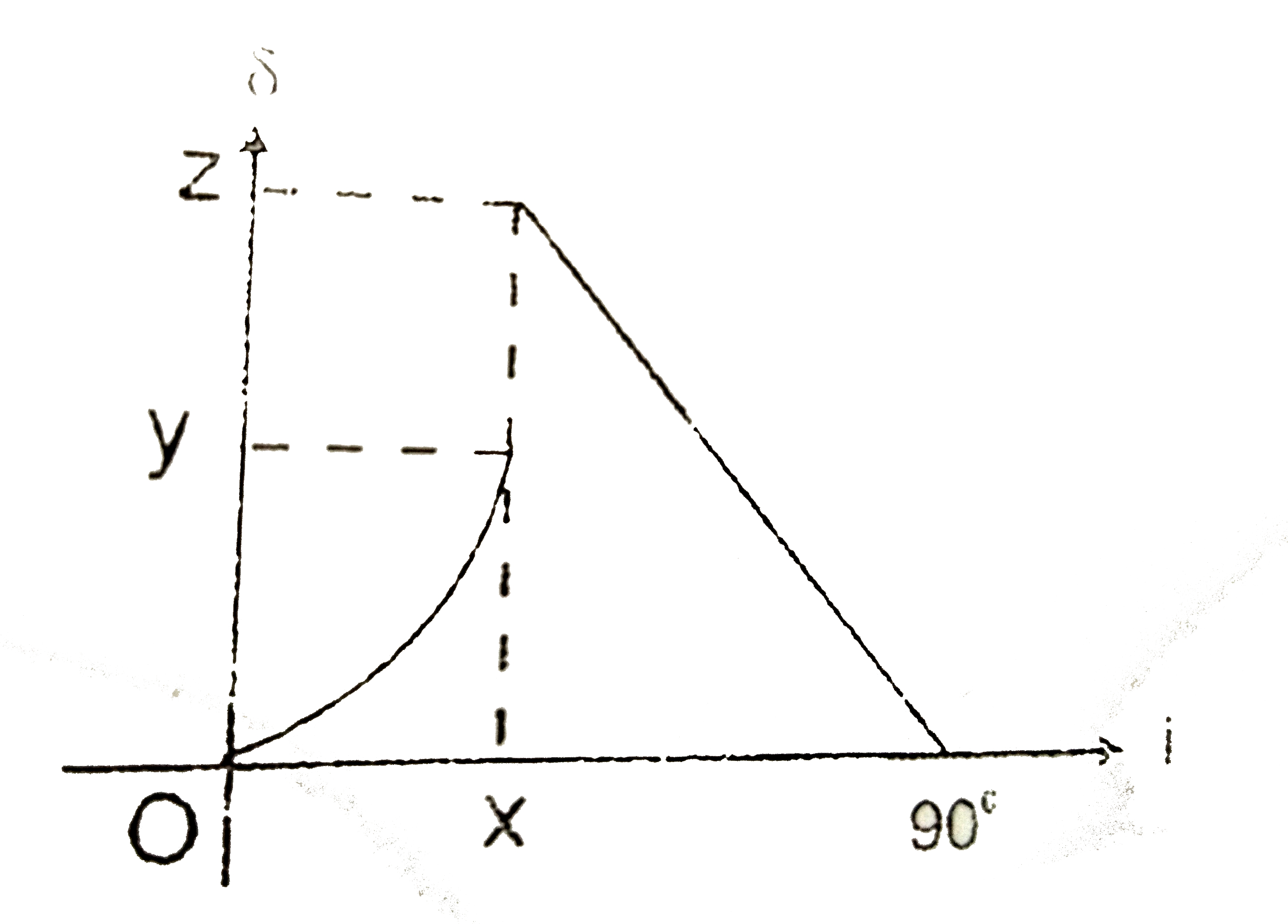
View SolutionLight is incident from glass to are. The variation of the angle of deviation δ with the angle of incidence i for 0<i<90∘ If the value of (x+y+z) is nπ6 then value of n.
View SolutionA ray of light passes from glass, having a refractive index of 1.6, to air. The angle of incidence for which the angle of refraction is twice the angle of incidence is
View SolutionIf refractive index of glass is 1.50 and of water is 1.33, then criticle angle is
View SolutionA ray of light is incident on a rectangular plate at an angle of incidence 60∘. The light ray suffers a deviation which is 25% of the angle of incidence. The refractive index of the glass will be
View SolutionA ray of light is incident normally on a plane glass slab. What will be (i) the angle of refraction, and (ii) the angle of deviation for the ray ?
View SolutionWhen unpolarised light beam is incident from air onto glass (n=1.5) at the polarising angle
View SolutionWhen unpolarised light beam is incident from air onto glass (n=1.5) at the polarising angle
View Solution
