A system can be taken from the initial state to the final state by two different methods, let represent the heat given to the system and the work done by the system. Which of the following must be the same in both the method?
.
The correct Answer is:D
To solve the problem, we need to analyze the relationship between heat (ΔQ), work (ΔW), and internal energy (ΔU) as described by the first law of thermodynamics.
1. Understand the First Law of Thermodynamics:
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (ΔQ) minus the work done by the system (ΔW). This can be expressed mathematically as:
ΔU=ΔQ−ΔW
2. Identify Internal Energy as a State Function:
Internal energy (ΔU) is a state function, meaning it depends only on the initial and final states of the system, not on the path taken to get from one state to another. Therefore, if a system transitions from an initial state (p1, V1) to a final state (p2, V2), the change in internal energy will be the same regardless of the path taken.
3. Apply the Concept to Both Paths:
Since the internal energy change (ΔU) is the same for both paths (let's call them Path 1 and Path 2), we can write:
ΔUPath1=ΔUPath2
4. Relate ΔQ and ΔW for Both Paths:
From the first law, we can express the changes in internal energy for both paths:
ΔUPath1=ΔQPath1−ΔWPath1
ΔUPath2=ΔQPath2−ΔWPath2
Since ΔU is the same for both paths, we can set the two equations equal to each other:
ΔQPath1−ΔWPath1=ΔQPath2−ΔWPath2
5. Conclude About ΔQ and ΔW:
Rearranging the above equation gives us:
ΔQPath1−ΔQPath2=ΔWPath1−ΔWPath2
This indicates that ΔQ and ΔW can vary depending on the path taken, but the difference between the heat added and the work done must remain constant.
6. Final Conclusion:
Therefore, the only quantity that must be the same for both methods is the change in internal energy (ΔU), which can also be expressed as:
ΔU=ΔQ−ΔW
Thus, the correct answer is:
ΔQ−ΔW must be the same for both methods.
Answer:
The quantity that must be the same in both methods is ΔQ−ΔW.
---
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (ΔQ) minus the work done by the system (ΔW). This can be expressed mathematically as:
ΔU=ΔQ−ΔW
Internal energy (ΔU) is a state function, meaning it depends only on the initial and final states of the system, not on the path taken to get from one state to another. Therefore, if a system transitions from an initial state (p1, V1) to a final state (p2, V2), the change in internal energy will be the same regardless of the path taken.
Since the internal energy change (ΔU) is the same for both paths (let's call them Path 1 and Path 2), we can write:
ΔUPath1=ΔUPath2
From the first law, we can express the changes in internal energy for both paths:
ΔUPath1=ΔQPath1−ΔWPath1
ΔUPath2=ΔQPath2−ΔWPath2
Since ΔU is the same for both paths, we can set the two equations equal to each other:
ΔQPath1−ΔWPath1=ΔQPath2−ΔWPath2
Rearranging the above equation gives us:
ΔQPath1−ΔQPath2=ΔWPath1−ΔWPath2
This indicates that ΔQ and ΔW can vary depending on the path taken, but the difference between the heat added and the work done must remain constant.
Therefore, the only quantity that must be the same for both methods is the change in internal energy (ΔU), which can also be expressed as:
ΔU=ΔQ−ΔW
Thus, the correct answer is:
ΔQ−ΔW must be the same for both methods.
The quantity that must be the same in both methods is ΔQ−ΔW.
Topper's Solved these Questions
Similar Questions
An ideal gas can be taken form initial state 1 to final state 2 by two different process. Let ΔQ and W represent the heat given and work done by the system. Then which quantities is/are same in both process (where ΔU= internal energy of gas)
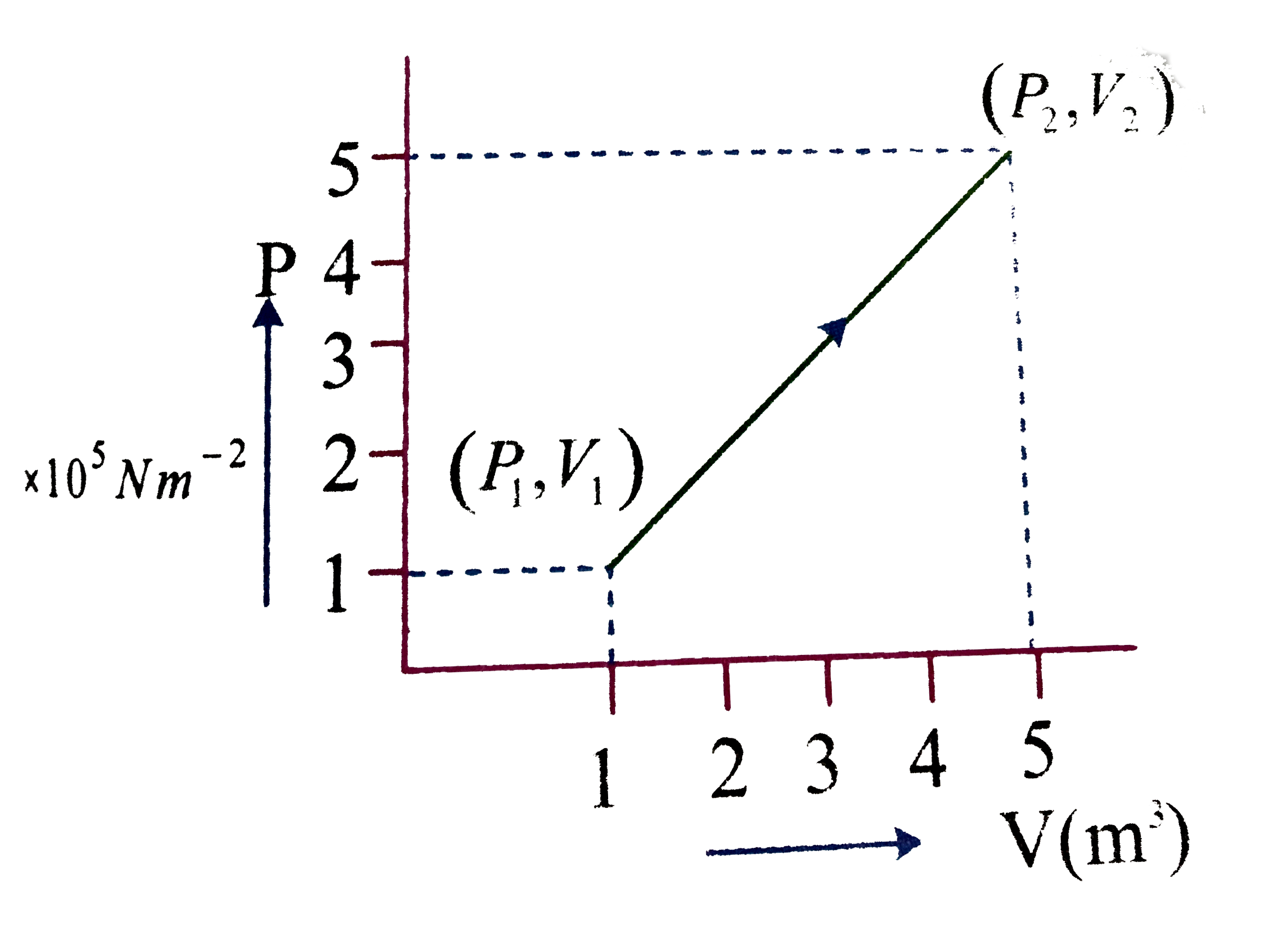
View SolutionA system changes from the state (P1,V1) to (P2,V2) as shown in the diagram. The work done by the system is
 View Solution
View SolutionA gas can be taken from A to B via two different processes ACB and DB.
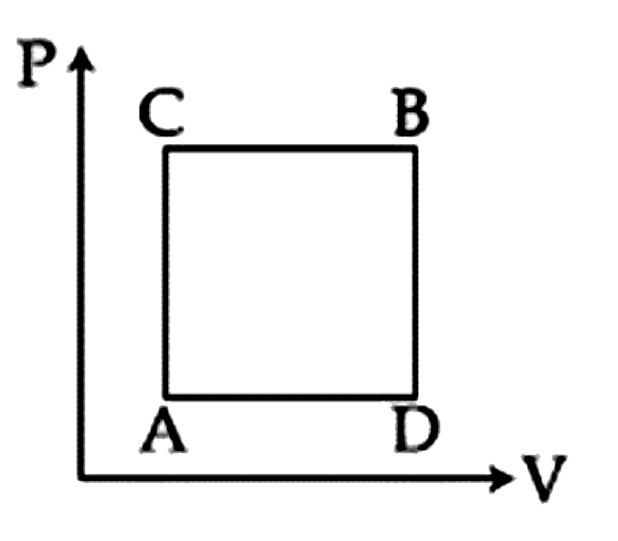
When path ACB is used 60 J of heat flows into the system and 30J of work done by the system is 10 J , the heat flows into the system in the path ADB is :View SolutionA thermo-dynamical system is changed from state (P1,V1) to (P2,V2) by two different process. The quantity which will remain same will be
View SolutionA gas with cpcV=γ goes from an intial state (p1,V1,T1) to a final state (p2,V2,T2) through an adiabatic process. The work done by the gas is
View SolutionIf a system undergoes an adiabatic change from state 1 to state 2, the work done by the gas is:
View SolutionWhen a thermodynamic system is taken from an initial state I to a final state F along the path IAF, as shown in Fig. the heat energy absorbed by the system is Q=55J and work done by the system is W=25J. If the same system is taken along the path IBF, the value of Q=35J.
(a) If W=−15J for the curved path FI, how much heat energy is lost by the system along this path ?
(b) If U1=10J, what is UF ?
(d) If UB=20J, what is Q for the process BF and IB ?View SolutionRefer to figure. Let ΔU1 and ΔU2 be the changes in internal energy in the system in process A+B and ΔW be the net work done by the system in the process A+B,
 View Solution
View SolutionAn ideal gas is initially at P1,V1 is expands to P2,V2 isothermally and then compressed adiabatically to the same volume V1 and pressure P3. If W is the net work done by the gas in complete process which of the following is true.
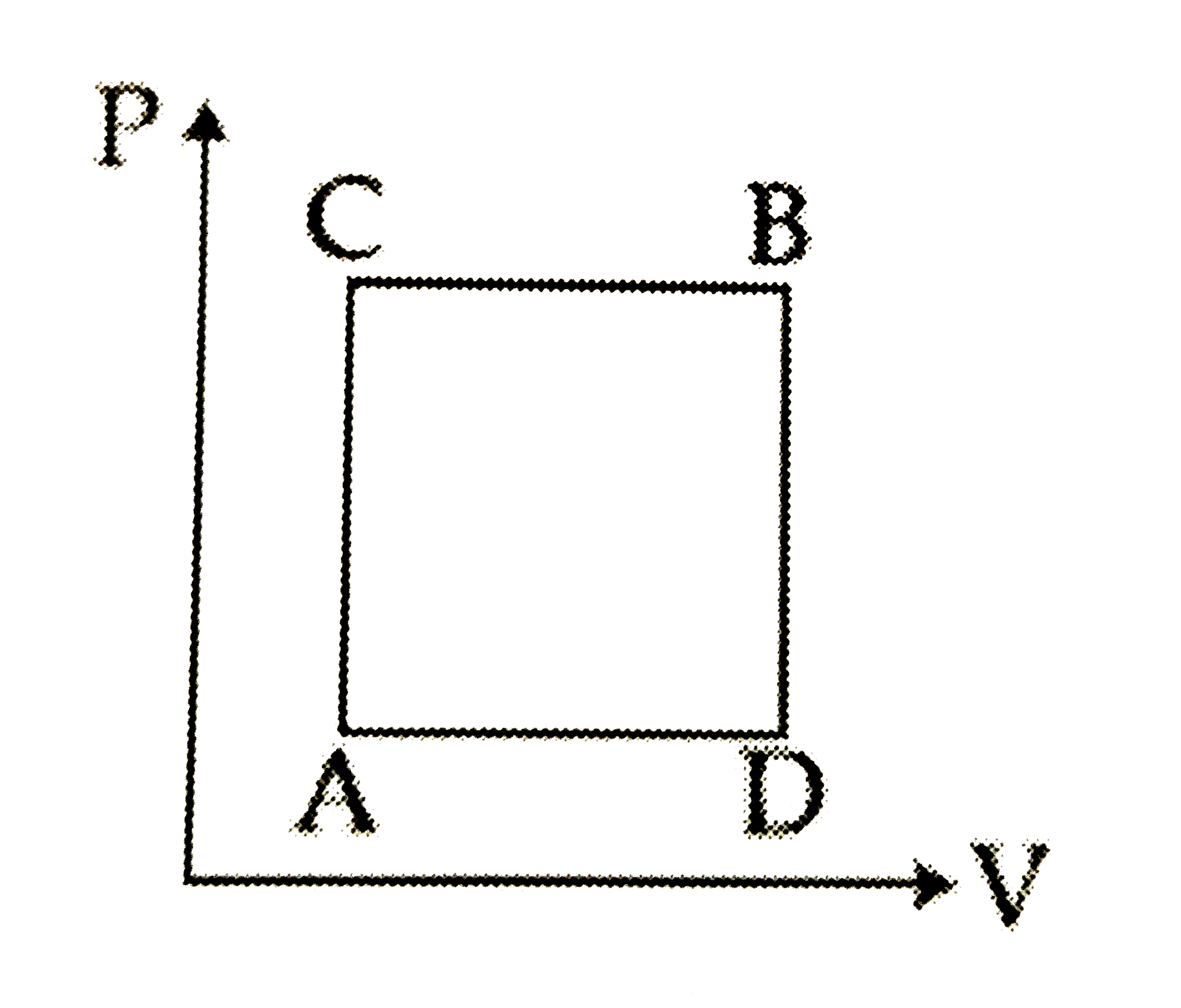
View SolutionA gas can be ken from A to B via two different processes ACB and ADB. When path ACB is used 60 J of heat flows into the system and 30 J of work is done by the system. If path ADB is used work done by the system is 10 J. The heat Flow into the system in path ADB is :
 View Solution
View Solution

