Two identical electric point dipoles have dipole moments −→P1=pˆiand−→P2=pˆi and are held on the x axis at distance 'a' from each other. When released, they move along the x-axis with the direaction of their dipole moments remaining unchanged. If the mass of each dipole is 'm', their speed when they are infinitely for apart is :
Option1 Pa√1π∈0ma
Option2 Pa√12π∈0ma
Option3 Pa√22π∈0ma
Option4 Pa√32π∈0ma
To solve the problem, we need to analyze the motion of two identical electric dipoles that are initially at rest and then released. We will use the principles of electrostatics and energy conservation to find their speed when they are infinitely far apart.
1. Understanding the Dipoles:
- We have two identical electric dipoles with dipole moments →P1=p^i and →P2=p^i.
- They are placed on the x-axis at a distance a from each other.
2. Initial Conditions:
- Initially, both dipoles are at rest, so their initial kinetic energy is zero.
- The potential energy of the system when they are at a distance a can be calculated using the formula for the potential energy of dipoles in an electric field.
3. Electric Field Due to a Dipole:
- The electric field E at a point on the axial line of a dipole is given by:
E=2kpr3
- Here, k is the electrostatic constant, p is the dipole moment, and r is the distance from the dipole.
4. Potential Energy:
- The potential energy U of a dipole in an electric field is given by:
U=−→E⋅→P
- For two dipoles, the potential energy when they are at a distance a can be expressed as:
U=−2kp2a3
5. Final Conditions:
- When the dipoles are infinitely far apart, the potential energy Uf=0.
- The total mechanical energy of the system is conserved. Therefore, the initial potential energy equals the final kinetic energy:
Ui=Kf
- Since both dipoles have mass m, the final kinetic energy of the two dipoles is:
Kf=2×12mv2=mv2
6. Setting Up the Energy Conservation Equation:
- From conservation of energy:
−2kp2a3=mv2
7. Solving for Speed v:
- Rearranging gives:
v2=2kp2ma3
- Taking the square root:
v=√2kp2ma3
8. Substituting for k:
- Recall that k=14πϵ0:
v=
⎷2⋅14πϵ0p2ma3=√p22πϵ0ma3
Final Answer:
The speed of each dipole when they are infinitely far apart is:
v=√p22πϵ0ma3
- We have two identical electric dipoles with dipole moments →P1=p^i and →P2=p^i.
- They are placed on the x-axis at a distance a from each other.
- Initially, both dipoles are at rest, so their initial kinetic energy is zero.
- The potential energy of the system when they are at a distance a can be calculated using the formula for the potential energy of dipoles in an electric field.
- The electric field E at a point on the axial line of a dipole is given by:
E=2kpr3
- Here, k is the electrostatic constant, p is the dipole moment, and r is the distance from the dipole.
- The potential energy U of a dipole in an electric field is given by:
U=−→E⋅→P
- For two dipoles, the potential energy when they are at a distance a can be expressed as:
U=−2kp2a3
- When the dipoles are infinitely far apart, the potential energy Uf=0.
- The total mechanical energy of the system is conserved. Therefore, the initial potential energy equals the final kinetic energy:
Ui=Kf
- Since both dipoles have mass m, the final kinetic energy of the two dipoles is:
Kf=2×12mv2=mv2
- From conservation of energy:
−2kp2a3=mv2
- Rearranging gives:
v2=2kp2ma3
- Taking the square root:
v=√2kp2ma3
- Recall that k=14πϵ0:
v=
⎷2⋅14πϵ0p2ma3=√p22πϵ0ma3
The speed of each dipole when they are infinitely far apart is:
v=√p22πϵ0ma3
Topper's Solved these Questions
Similar Questions
Two electric dipoles, A, B with respective dipole moments −→dA=−4 qa ˆiand−→dB=−2 qa ˆi are placed on the x-axis with a seperation R, as shown in the figure
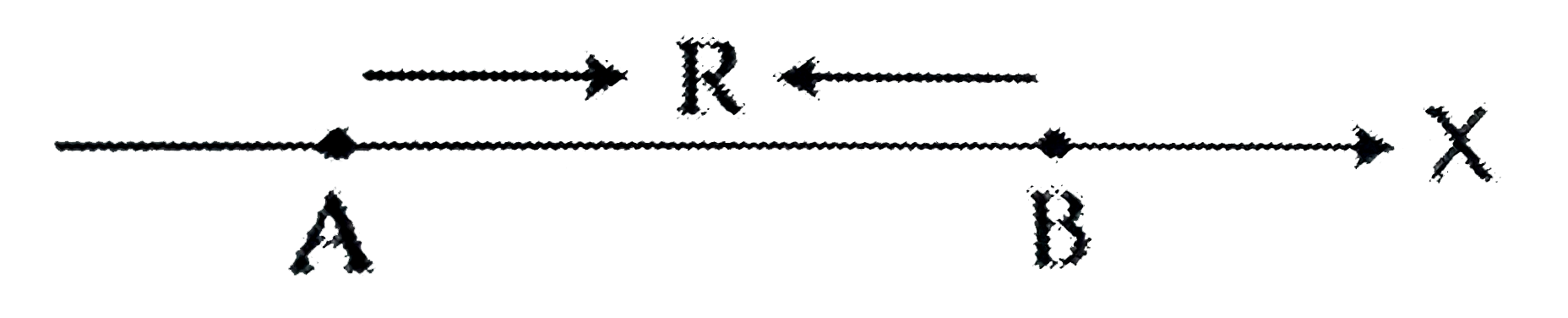
The distance from A at which both of them produce the same potential is :
View SolutionTwo identical electric dipoles of dipole moments p are placed as shown in the figure. The electrostatic force on dipole placed along x-axis is (d << r) (14πε0 )
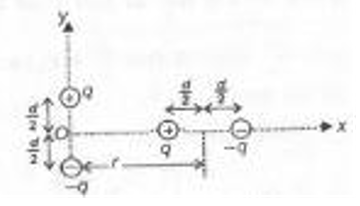
View Solution
Knowledge Check
Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
A√5×10−7TB5×10−7TC√2×10−7TD10−7T
The dipole moment of a short bar magnet is 1.25A−m2. The magnetic field on its axis at a distance of 0.5 metre from the centre of the magnet is
View SolutionA dipole of dipole moment →P is placed in uniform electric field →E, then torque acting on it is given by
View SolutionAn electric dipole is kept on the axis of a uniformly charged ring at distance from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is p, charge of the ring is Q & radius of the ring is R. The force on the dipole is
View SolutionTwo identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
View SolutionAn electric point dipole is placed at the origin O with its dipolemoment along the X-axis . A point A is at a distance r from the origin such that OA makes an angle π/3 with the X -axis if the electric field →E due to the dipole at A makes an angle θ with the positive X-axis, the value of θ is
View SolutionThe electric field intensity →E , , due to an electric dipole of dipole moment →p , at a point on the equatorial line is :
View SolutionTwo electric dipoles of moment P and 64P are placed in opposite direction on a line at a distance of 25cm. The electric field will be zero at point between the dipoles whose distance from the dipole of moment P is
View Solution
