The velocity of a particle moving in the positive direction of the X-axis varies as where K is a positive constant. Draw V-t graph.Solution in Telugu
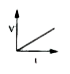
The V-t graph is a straight line passing through the origin.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Exercise 1A62videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Exercise 2A30videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Questions for Descriptive Answers7videos
MOTION IN A STRAIGHT LINE
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Practice Exercise84videos
MOTION IN A PLANE
- BOOK - AAKASH SERIESCHAPTER - NEWTONS LAWS OF MOTION EXERCISE - ADDITIONAL PRACTICE EXERCISE PRACTICE SHEET (ADVANECED) (Matrix Matching Type Questions)1videos
NEWTONS LAWS OF MOTION
Similar Questions
A particle starts from the origin ,goes along the x-axis to the point (20m,0) and then returns along the same line to the point (-20 m,0).The distance and displacement of the particle during the trip are
01:58View Solution(A): Displacement of a body may be zero when distance travelled by it is not zero.
(R) : The displacement is the longest distance between initial and final positions..01:57View SolutionThree tortoises are located at the vertices of an equilateral triangle whose side equals a They all start moving simultaneously with velocity v constant in modulus with the first tortoise heading continually for the second , the second for the third and the third for the first . They will meet after time
02:36View SolutionA person runs along a circular path of radius 5m. Find the magnitude of the displacement if he runs all the way around the circle ?
03:36View SolutionThe system shown in the figure is given an acceleration 'a' towards left. Assume all contact surfaces to be smooth (m: mass of the sphere) The magnitude of the contact force between wedge and sphere between wedge and sphere at A is xmg√3 then 'x' is
 01:44View Solution
01:44View SolutionThe velocity of a particle moving in the positive direction of x-axis veries as v=10√x. Assuming that at t=0, particle was at x=0
02:19View SolutionThe displacement to particle is zero at t=0andiszatt=t. It starts moving in the positive x-direction with a velocity which varies, v=k√x, wher (k) is a constant. Find the relation for variation of velocity with time.
02:43View SolutionThe dispkacement of particle is zero at t= 0 and it is x , at t-t . It starts moving in the positive x-direction with a velocity which varices as v= k sqrt x , where (k) is a constant. Show that velocity is directly proprtional to time.
03:27View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V−t graph.
View SolutionThe velocity of a particle moving in the positive direction of X-axis varies as v=5sqrt(x) . Assuming that at t = 0, particle was at x = 0. What is the acceleration of the particle ?
View SolutionThe velocity v of a particle moving along x - axis varies with ist position (x) as v=alpha sqrtx , where alpha is a constant. Which of the following graph represents the variation of its acceleration (a) with time (t)?
03:08View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V = KsqrtS where K is a positive constant. Draw V-t graph.
02:28View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as v=K√S
Where K is a positive constant.Draw V-t graph .04:08View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=Ksqrt(S) where K is a positive constant. Draw V-t graph.
01:53View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V−t graph.
01:38View Solution

