There are two current carrying planar coils made each from identical wires of length L. C1 is the circular (radius R) and C2 is square (side a). They are so constructed that they have same frequency of oscillation when they are placed in the same uniform →B and carry the same current i. Find a in terms of R.
a=R
a=2R
a=3R
2a=5R
The correct Answer is:C
To find the side length a of the square coil C2 in terms of the radius R of the circular coil C1, we will derive the expressions for the magnetic moments and moments of inertia for both coils, and then equate their angular frequencies since they oscillate with the same frequency.
1. Magnetic Moment of Coil C1:
- The magnetic moment m1 of a coil is given by the formula:
m1=N1IA1
- For the circular coil C1, the number of turns N1 can be expressed as:
N1=L2πR
- The area A1 of the circular coil is:
A1=πR2
- Substituting these into the magnetic moment formula:
m1=(L2πR)I(πR2)=LIR2
2. Moment of Inertia of Coil C1:
- The moment of inertia I1 for a circular coil is:
I1=mR22
- The mass m can be expressed as:
m=L2πR⋅(mass per unit length)
- Therefore:
I1=L2πR⋅(mass per unit length)⋅R22
3. Angular Frequency of Coil C1:
- The angular frequency ω1 is given by:
ω1=√m1BI1
4. Magnetic Moment of Coil C2:
- For the square coil C2:
m2=N2IA2
- The number of turns N2 is:
N2=L4a
- The area A2 of the square coil is:
A2=a2
- Thus:
m2=(L4a)I(a2)=LIa4
5. Moment of Inertia of Coil C2:
- The moment of inertia I2 for a square coil is:
I2=ma212
6. Angular Frequency of Coil C2:
- The angular frequency ω2 is:
ω2=√m2BI2
7. Equating Angular Frequencies:
- Since both coils have the same frequency of oscillation:
ω1=ω2
- This implies:
m1I1=m2I2
8. Substituting Values:
- Substitute m1, I1, m2, and I2 into the equation:
LIR2mR22=LIa4ma212
- Simplifying leads to:
LIRmR2=3LIama2
9. Canceling Common Terms:
- Cancel L and m from both sides:
IRR2=3Ia4a2
- Rearranging gives:
4a2=3R⟹a=3R4
Final Result:
a=3R
- The magnetic moment m1 of a coil is given by the formula:
m1=N1IA1
- For the circular coil C1, the number of turns N1 can be expressed as:
N1=L2πR
- The area A1 of the circular coil is:
A1=πR2
- Substituting these into the magnetic moment formula:
m1=(L2πR)I(πR2)=LIR2
- The moment of inertia I1 for a circular coil is:
I1=mR22
- The mass m can be expressed as:
m=L2πR⋅(mass per unit length)
- Therefore:
I1=L2πR⋅(mass per unit length)⋅R22
- The angular frequency ω1 is given by:
ω1=√m1BI1
- For the square coil C2:
m2=N2IA2
- The number of turns N2 is:
N2=L4a
- The area A2 of the square coil is:
A2=a2
- Thus:
m2=(L4a)I(a2)=LIa4
- The moment of inertia I2 for a square coil is:
I2=ma212
- The angular frequency ω2 is:
ω2=√m2BI2
- Since both coils have the same frequency of oscillation:
ω1=ω2
- This implies:
m1I1=m2I2
- Substitute m1, I1, m2, and I2 into the equation:
LIR2mR22=LIa4ma212
- Simplifying leads to:
LIRmR2=3LIama2
- Cancel L and m from both sides:
IRR2=3Ia4a2
- Rearranging gives:
4a2=3R⟹a=3R4
a=3R
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - EXEMPLAR PROBLEMS5videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSERTION AND REASON15videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - PERMANENT MAGNETS AND ELECTROMAGNETS4videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Assertion And Reason15videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason15videos
MOVING CHARGES AND MAGNETISM
Similar Questions
Two wires of same length are shaped into a square and a circle. If they carry same current, ratio of the magnetic moment is
View SolutionTwo wires of the same length are shaped into a square and a circle. If they carry same current, ratio of the magnetic moment is
View SolutionA semi circular arc of radius r and a straight wire along the diameter, both are carrying same current i find out magnetic force per unit length on the small element P, which is at the centre of curvature.
 View Solution
View SolutionShow that the force on a wire between a and b of arbitrary of figure is the same as force on the straigth wire between the same two points when they carry the same current from a to b and are plassed in the same magnetic field. Also find the force.
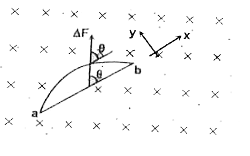 View Solution
View SolutionA length of wire carries a steady current. It is first bent to form a circular coil of one turn. The same length is now bent more sharply to give a loop of two turns of smaller radius. The magentic field at the centre caused by the same current now will be
View SolutionTwo identical circular wires P and Q each of radius R and carrying current 'I' are kept in perpendicular planes such that they have a common centre as shown in the figure. Find the magnitude and direction of the net magnetic field at the common centre of the two coils.
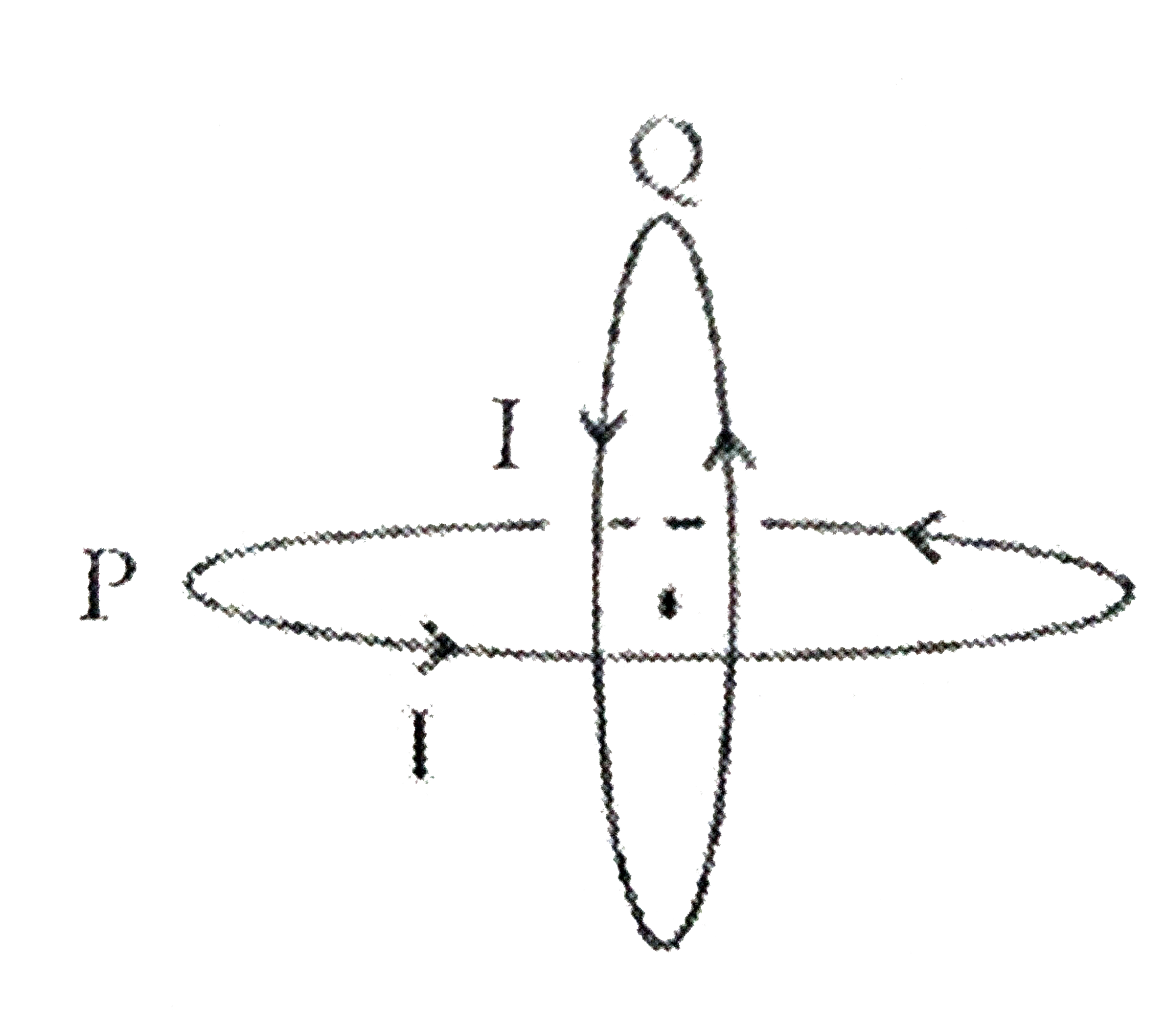 View Solution
View SolutionA circular coil of radius 2R is carrying current 'i' . The ratio of magnetic fields at the centre of the coil and at a point at a distance 6R from the centre of the coil on the axis of the coil is
View SolutionA thin circular wire carrying a current I has a magnetic moment M. The shape of the wire is changed to a square and it carries the same current. It will have a magnetic moment
View SolutionA steady current is flowing in a circular coil of radius R, made up of a thin conducting wire. The magnetic field at the centre of the loop is BL. Now, a circular loop of radius R/n is made from the same wire without changing its length, by unfounding and refolding the loop, and the same current is passed through it. If new magnetic field at the centre of the coil is BC, then the ratio BL/BC is
View SolutionA steady current is flowing in a circular coil of radius R, made up of a thin conducting wire. The magnetic field at the centre of the loop is BL. Now, a circular loop of radius R/n is made from the same wire without changing its length, by unfounding and refolding the loop, and the same current is passed through it. If new magnetic field at the centre of the coil is BC, then the ratio BL/BC is
View Solution

