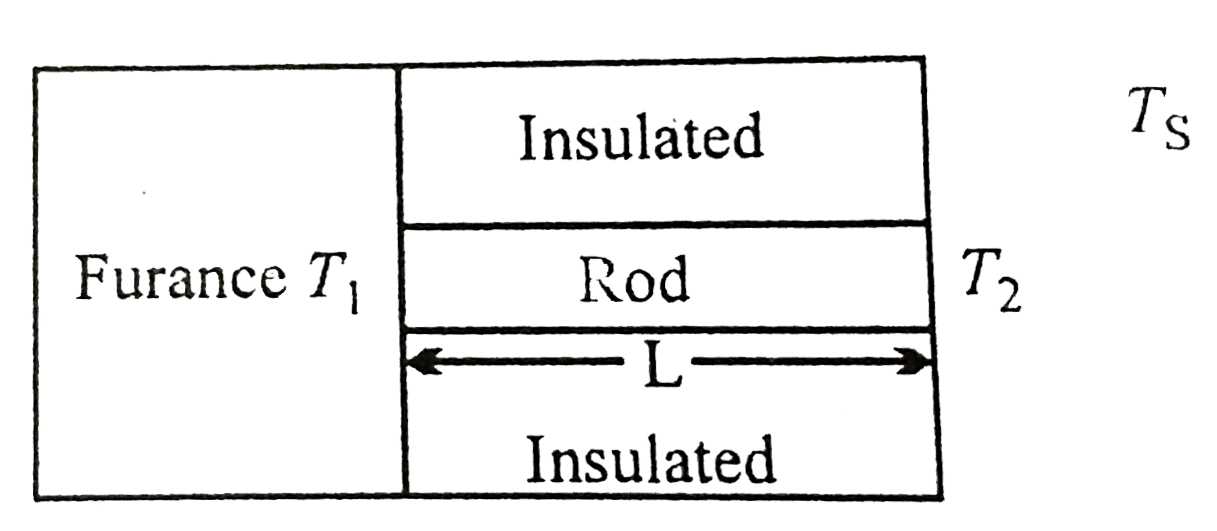
One end of a rod of length L and cross - sectioal area A is kept in a furance of temperature $T_{1}$ . The other end of the rod is kept at a tempearute $T_{2}$ . The thermal conducitivity of the material of the rod is K and emissivity of rod is K and emissivity of rod is e. It is given that $T_{2} = T_{s} + Δ T$ , where $Δ T < < T_{s}, T_{s}$ being the temperature of surroudings. If $Δ T \propto (T_{1} - T_{s})$ , find the proportionality constant. Consider that heat is lost only by radiation at the end where the temperature of the rod is $T_{2}$ ..

Text Solution

Verified by Experts

Rate of Heat gained by rod through conduction , $Q = \frac{K A (T_{1} - T_{2})}{L}$

Rate of Heat lost by rod through radiation , $Q_{2} = e A σ (T_{2}^{4} - T_{s}^{4})$
In steady state $Q_{1} = Q_{2}$
$∴ \frac{K A (T_{1} - T_{2})}{L} = e A σ (T_{2}^{4} - T_{s}^{4}) ... ... ... . (1)$
Given $T_{2} = T_{s} + Δ T ∴ T_{2}^{4} = {(T_{s} + Δ T)}_{s}^{4} = T_{S}^{4} + {(1 + \frac{Δ T}{T_{s}})}^{4}$
As $Δ T < < T_{s}$ , so using bionmial theorem .
$T_{2}^{4} = T_{s}^{4} (1 + \frac{4 Δ T}{T_{s}}) \Rightarrow T_{2}^{4} - T_{s}^{4} = 4 T_{s}^{3} Δ T$
substituting this value in (1) , we get $\frac{K A (T_{1} - T_{2})}{L} = e A σ . (4 T_{s}^{3} Δ T)$ Also $T_{2} = T_{s} + Δ T$
$∴ \frac{K A (T_{1} - T_{s} - Δ T)}{L} = e A σ (4 T_{s}^{3} Δ T) \Rightarrow \frac{K (T_{1} - T_{s})}{L} - \frac{K}{L} Δ T = 4 e σ T_{s}^{3} Δ T$
(or) $\frac{K (T_{1} - T_{s})}{L} = (4 e σ T_{s}^{3} + \frac{K}{L}) Δ T, Δ T = \frac{K (T_{1} - T_{S})}{(4 e σ L T_{s}^{3} + K)}$ But given $Δ T = C (T_{1} - T_{s})$
$∴$ Constant of proportioanlity C is $\frac{K}{K + 4 e σ L T_{s}^{3}}$

Updated on:21/07/2023

One end of a metal rod is kept in a furnce. In steady state, the temperature of the rod
Aincreases
Bdecreases
Cremains constant
Dis non uniform.
The two ends of a rod of length $L$ and a uniform cross-sectional area $A$ are kept at two temperature $T_{1}$ and $T_{2}$ $(T_{1} > T_{2})$ . The rate of heat transfer. $\frac{d Q}{d t}$ , through the rod in a steady state is given by
A $\frac{d Q}{d t} = \frac{K L (T_{1} - T_{2})}{A}$
B $\frac{d Q}{d t} = \frac{K L (T_{1} - T_{2})}{L A}$
C $\frac{d Q}{d t} = K L A (T_{1} - T_{2})$
D $\frac{d Q}{d t} = \frac{K A (T_{1} - T_{2})}{L}$
A metal rod of area of cross section A has length $L$ and coefficient of thermal conductivity $K$ the thermal resistance of the rod is .
A $\frac{L}{K A}$
B $\frac{K L}{L}$
C $\frac{K A}{L}$
D $\frac{A}{K L}$